lec40.ppt
Download as PPT, PDF
0 likes6 views
Complex analysis deals with complex-valued functions of complex variables. Some key concepts covered in the document include: - Complex functions can be expressed in either Cartesian (z = x + iy) or polar (z = rei慮) form. - For a complex function f(z) to be differentiable, it must satisfy the Cauchy-Riemann conditions. - Analytic functions are differentiable at every point in their domain. For example, ez is analytic everywhere while z is analytic nowhere. - Branch cuts arise for multi-valued functions like z, with the line between 慮 = 2 and 慮 = 4 representing one branch cut.
![NPTEL Physics Mathematical Physics - 1
The value of the function at A is () = 12. After a complete revolution,
() =
(1+22
= 12
So it does not return to the same value. So the function is multivalued and
12 is a
value on one of the branches and between 2 4, the function assumes another
branch. The line OB is called the branch cut.
Complex integration Cauchy Goursat Theorem
If () is analytic in a region R and on its boundary , then () = 0 Also known as
cauchys integral theorem.
Cauchys Integral formula
() =
1 ()
2 р
() is analytic inside and on the boundary c of a simply connected region R, excepting
at a point a.
Residue theorem
If () is analytic inside and on a simple closed curve c except for a pole of order m at
= , inside c, prove that
1 1
2
() = 瑞
р ( 1)! ю1
1
[( )()]
If there are two different poles of order 1and 2
1 1
2
() = 瑞
р1 (11)! ю11
11
[( 1)1 ()] +
瑞
р2 (21)! ю21
Joint initiative of IITs and IISc Funded by MHRD Page 55 of 66
1 21
[( )2 ()]
2
Example
Integrate,
=
0 1+3
The integrand I is not even so we cannot extend it up to -.](https://image.slidesharecdn.com/lec40-231023100403-fdfab015/85/lec40-ppt-2-320.jpg)
lec40.ppt
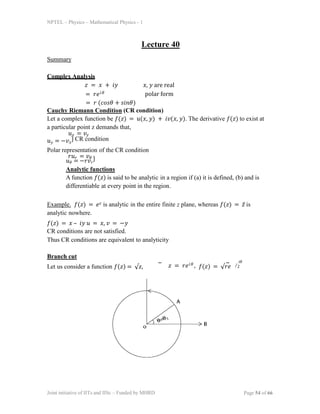
- 1. NPTEL Physics Mathematical Physics - 1 Lecture 40 Summary Complex Analysis = + = = ( + ) , are real polar form Cauchy Riemann Condition (CR condition) Let a complex function be () = (, ) + (, ). The derivative () to exist at a particular point z demands that, = ｐ } CR condition Polar representation of the CR condition = ｐ = ｐ = ｐ } Analytic functions A function () is said to be analytic in a region if (a) it is defined, (b) and is differentiable at every point in the region. Example. () = is analytic in the entire finite z plane, whereas () = is analytic nowhere. () = = , = CR conditions are not satisfied. Thus CR conditions are equivalent to analyticity Branch cut Let us consider a function () = , = , () = 2 Joint initiative of IITs and IISc Funded by MHRD Page 54 of 66
- 2. NPTEL Physics Mathematical Physics - 1 The value of the function at A is () = 12. After a complete revolution, () = (1+22 = 12 So it does not return to the same value. So the function is multivalued and 12 is a value on one of the branches and between 2 4, the function assumes another branch. The line OB is called the branch cut. Complex integration Cauchy Goursat Theorem If () is analytic in a region R and on its boundary , then () = 0 Also known as cauchys integral theorem. Cauchys Integral formula () = 1 () 2 р () is analytic inside and on the boundary c of a simply connected region R, excepting at a point a. Residue theorem If () is analytic inside and on a simple closed curve c except for a pole of order m at = , inside c, prove that 1 1 2 () = 瑞 р ( 1)! ю1 1 [( )()] If there are two different poles of order 1and 2 1 1 2 () = 瑞 р1 (11)! ю11 11 [( 1)1 ()] + 瑞 р2 (21)! ю21 Joint initiative of IITs and IISc Funded by MHRD Page 55 of 66 1 21 [( )2 ()] 2 Example Integrate, = 0 1+3 The integrand I is not even so we cannot extend it up to -.
- 3. NPTEL Physics Mathematical Physics - 1 Let us evaluate the integral 3 = 1 = 1+3 over the Contour below = 3 1 are the poles 3 = = 6 0 0 = 1 + 3 = 1 + 33 = = 1+3 2 + 3 1+3 1+3 0 + 0 So, 0 = + 1+33 = 2 3 = + ( 1 2 3 2 ) 0 1+3 3 = + 2 2 3 2 3 = (1 3) 2 = 1 3 2 2 2 = 2 + 2 = 1 Now has a simple pole at = 3 = 2 3 2 3 = 2 33 Joint initiative of IITs and IISc Funded by MHRD Page 56 of 66