�ݺ�ߣ

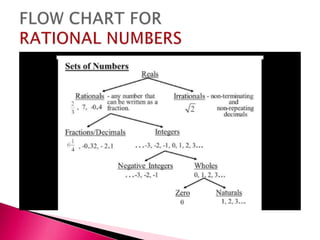
 DEFINITION OF RATIONAL NUMBERS
 COMPARISON OF RATIONAL AND IRRATIONAL
NUMBERS
 PROPERTIES OF RATIONAL NUMBERS
 OPERATIONS ON RATIONAL NUMBERS
 ROLE OF “ 0 “ and “ 1”
 NEGATIVE OF RATIONAL NUMBERS
 RECIPROCAL OF RATIONAL NUMBERS
 REPRESENTATION OF RATIONAL NUMBERS ON
NUMBER LINE
 RATIONAL NUMBERS BETWEEN ANY TWO
RATIONAL NUMBERS

 Def:- Rational numbers :- The numbers are
of the form
i)p/q form
ii)p,q £ Z
iii)q≠ 0
 Rational numbers are indicated by capital “ Q”
(QUOZIENTE---ITALIAN---QUOTIENT)

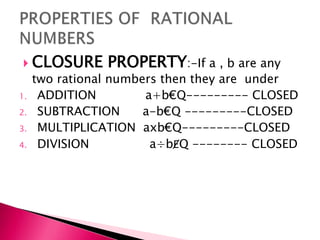
 CLOSURE PROPERTY:-If a , b are any
two rational numbers then they are under
1. ADDITION a+b€Q--------- CLOSED
2. SUBTRACTION a-b€Q ---------CLOSED
3. MULTIPLICATION axb€Q---------CLOSED
4. DIVISION a÷bɆQ -------- CLOSED

If a , b are any two rational numbers then they
are under
1. ADDITION a+b=b+a-------- TRUE
2. SUBTRACTION a-b≠b-a ------ FALSE
3. MULTIPLICATION axb=bxa-------TRUE
4. DIVISION a÷b≠b÷a ------------FALSE

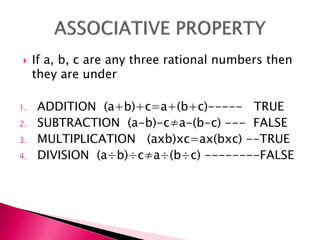
 If a, b, c are any three rational numbers then
they are under
1. ADDITION (a+b)+c=a+(b+c)----- TRUE
2. SUBTRACTION (a-b)-c≠a-(b-c) --- FALSE
3. MULTIPLICATION (axb)xc=ax(bxc) --TRUE
4. DIVISION (a÷b)÷c≠a÷(b÷c) --------FALSE

 When we multiply the given number by the
other number the result is “1”.,then one of
the number is the reciprocal of the other
number.
 then the multiplied number is called”
multiplicative inverse”
 Here “ 1” is called multiplicative identity of
rational numbers.


When a rational number is multiplied with the
reciprocal
or
 multiplicative inverse of its own,
result will be one (1)
 i.e.
 x/y × y/x = 1.
Example: 2/7 × 7/2 = 1; etc.

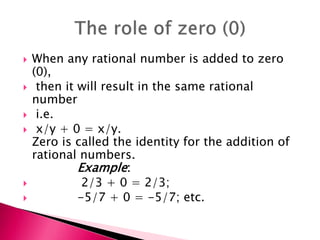
 When any rational number is added to zero
(0),
 then it will result in the same rational
number
 i.e.
 x/y + 0 = x/y.
Zero is called the identity for the addition of
rational numbers.
Example:
 2/3 + 0 = 2/3;
 -5/7 + 0 = -5/7; etc.

 When any rational number is multiplied with
one (1),
 then
 it will result in the same rational number
 i.e. x/y × 1 = x/y.
One is called the multiplicative identity for
rational numbers.
Example: 2/7 × 1 = 2/7;
 -8/3 × 1 = -8/3; etc.

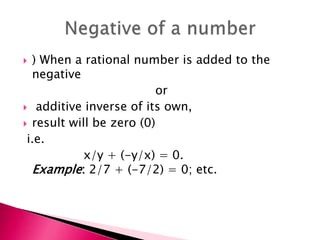
 When we add any number to the given
number the result is “0”.
 Adding number is called” NEGATIVE OF A
GIVEN RATIONAL NUMBER”.
 Then the added number is called a “additive
inverse “.
 Here “0 “ is called additive identity of rational
numbers.

 ) When a rational number is added to the
negative
or
 additive inverse of its own,
 result will be zero (0)
i.e.
x/y + (-y/x) = 0.
Example: 2/7 + (-7/2) = 0; etc.

:
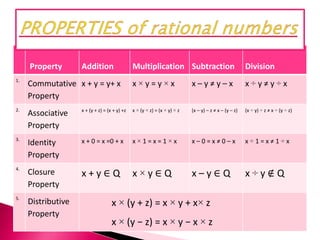
Closure Property
Associative Property
Commutative Property
Distributive Property
Identity Property
Inverse property

Property Addition Multiplication Subtraction Division
1.
Commutative
Property
x + y = y+ x x × y = y × x x – y ≠ y – x x ÷ y ≠ y ÷ x
2.
Associative
Property
x + (y + z) = (x + y) +z x × (y × z) = (x × y) × z (x – y) – z ≠ x – (y – z) (x ÷ y) ÷ z ≠ x ÷ (y ÷ z)
3.
Identity
Property
x + 0 = x =0 + x x × 1 = x = 1 × x x – 0 = x ≠ 0 – x x ÷ 1 = x ≠ 1 ÷ x
4.
Closure
Property
x + y ∈ Q x × y ∈ Q x – y ∈ Q x ÷ y ∉ Q
5.
Distributive
Property
x × (y + z) = x × y + x× z
x × (y − z) = x × y − x × z

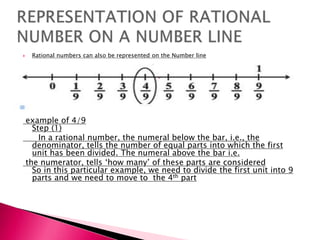
 Rational numbers can also be represented on
the Number line

 Rational numbers can also be represented on the Number line
example of 4/9
Step (1)
In a rational number, the numeral below the bar, i.e., the
denominator, tells the number of equal parts into which the first
unit has been divided. The numeral above the bar i.e.
the numerator, tells ‘how many’ of these parts are considered
So in this particular example, we need to divide the first unit into 9
parts and we need to move to the 4th part

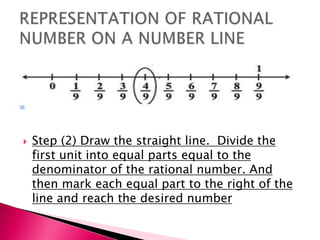
 Step (2) Draw the straight line. Divide the
first unit into equal parts equal to the
denominator of the rational number. And
then mark each equal part to the right of the
line and reach the desired number

�ݺ�ߣ

Rational number class 8th

Recommended

More Related Content

What's hot (11)

Similar to Rational number class 8th (20)

Recently uploaded (20)

Rational number class 8th