04TypeCurves.pptx
Download as PPTX, PDF0 likes8 views
This document provides an overview of Module 4 of the ESS 454 Hydrogeology course on flow to wells. It discusses the following key points in 3 paragraphs: 1) It introduces radial flow and well function, non-dimensional variables, Theis type curves, and the Cooper-Jacob method for analyzing confined aquifers. It also mentions aquifer boundaries and recharge. 2) It lists the learning objectives of understanding how to use the Hantush-Jacob formula to model leaky confined aquifers and use type curves to determine transmissivity, storativity, and boundary effects. 3) It provides an overview of modeling unconfined aquifers using the Neuman
More Related Content
More from Ali Al-naqa (20)
Recently uploaded (20)
04TypeCurves.pptx
- 1. ESS 454 Hydrogeology Module 4 Flow to Wells Preliminaries, Radial Flow and Well Function Non-dimensional Variables, Theis Type curve, and Cooper-Jacob Analysis Aquifer boundaries, Recharge, Thiem equation Other Type curves Well Testing Last Comments Instructor: Michael Brown brown@ess.washington.edu
- 2. Learning Objectives Forward problem: Understand how to use the Hantush- Jacob formula to predict properties of a confined aquifer with leakage Inverse problem: Understand how to use Type curves for a leaky confined aquifer to determine T, S, and B Understand how water flows to a well in an unconfined aquifer o Changes in the nature of flow with time o How to use Type curves
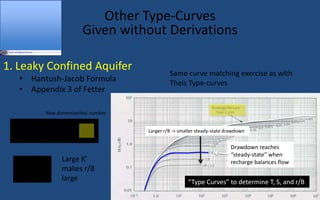
- 3. Other Type-Curves Given without Derivations 1. Leaky Confined Aquifer Hantush-Jacob Formula Appendix 3 of Fetter ho -h = Q 4pT W(u,r B) B = Tb' K ' Type Curves to determine T, S, and r/B Drawdown reaches steady-state when recharge balances flow Larger r/B -> smaller steady-state drawdown Same curve matching exercise as with Theis Type-curves New dimensionless number Large K makes r/B large
- 4. Other Type-curves Given without Derivations 2. Unconfined Aquifer Neuman Formula Appendix 6 of Fetter ho -h = Q 4pT W(uA,uB,G) G = r2 Kv b2 Kh uA = r2 S 4Tt uB = r2 Sy 4Tt Similar to Theis but more complicated: 1. Initial flow from elastic storage - S 2. Late time flow from gravity draining Sy Remember: Sy>>S 3. Vertical and horizontal flow Kv may differ from Kh Three non-dimensional variables Initial flow from Storativity Later flow from gravity draining Difference between vertical and horizontal conductivity is important
- 5. Flow in Unconfined Aquifer surface Flow from elastic storage Vertical flow (gravity draining) Horizontal flow induced by gradient in head 1. Elastic Storage Time order 2. Flow from gravity draining and horizontal head gradient Start Pumping
- 6. Other Type-curves Given without Derivations Two-step curve matching: 1. Fit early time data to A- type curves 2. Fit late time data to B- type curves 2. Unconfined Aquifer Neuman Formula Appendix 6 of Fetter Theis curve using Elastic Storage Theis curve using Specific Yield Transition depends on ratio r2Kv/(Khb2) Sy=104*S Sy=103*S
- 7. The End: Other Type Curves Coming up: Well Testing