1a factorization ok
Download as PPTX, PDF2 likes485 views
This document provides examples of factorizing polynomials using various factorization techniques. It contains 30 questions with step-by-step solutions demonstrating how to factorize expressions involving single variables, binomials, trinomials, and polynomials using formulas like difference of squares, perfect square trinomials, and grouping. The techniques shown include finding common factors, using factor trees, recognizing patterns, and applying factorization identities.
1 of 32
Download to read offline






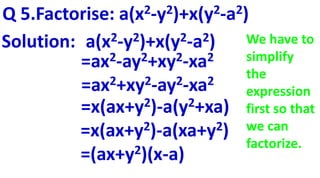






















Recommended
Foil



Foillothomas
Ėý
The document provides instructions for multiplying polynomials using three methods: the distributive property, FOIL (First, Outer, Inner, Last), and the box method. FOIL is only used when multiplying two binomials, while the distributive property and box method can be used for any polynomials. Examples are provided to demonstrate multiplying polynomials of varying complexities using each method. Students are encouraged to practice the methods and choose the one they find easiest.Expand brackets 1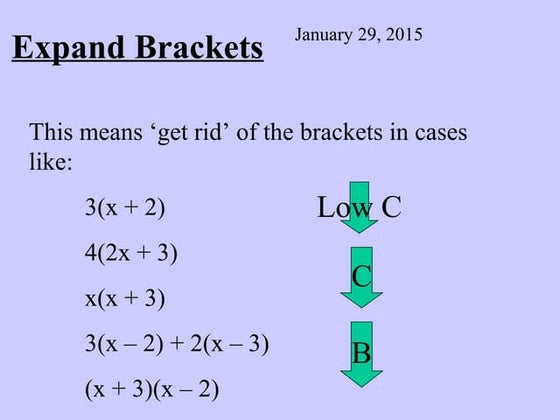



Expand brackets 1Patryk Mamica
Ėý
To expand expressions with brackets, you multiply the terms within the brackets by the number outside. For example, to expand 3(x + 2), you multiply x + 2 by 3, giving 3x + 6. Similarly, to expand 4(2x - 3), you multiply 2x - 3 by 4, giving 8x - 12. In both cases, this removes the brackets by distributing the number outside the brackets to each term inside.Radical and exponents (2)



Radical and exponents (2)Nurul Atiyah
Ėý
The document discusses properties and laws of exponents, radicals, logarithms including the definition of rational exponents, properties of logarithms such as the change of base formula, and examples of simplifying expressions using exponent laws, combining like radicals, and solving logarithmic equations by using properties of logarithms. It also provides sample problems and their step-by-step solutions for simplifying expressions and solving equations involving exponents, radicals, and logarithms.factoring polynomials



factoring polynomialsabigail Dayrit
Ėý
This document outlines key concepts and examples for factoring polynomials. It covers factoring trinomials of the forms x^2 + bx + c, ax^2 + bx + c, and x^2 + bx + c by grouping. Examples are provided to demonstrate finding the greatest common factor of terms, factoring trinomials by finding two numbers whose product and sum meet the given criteria, and checking factoring results using FOIL multiplication. Sections include the greatest common factor, factoring trinomials of the forms x^2 + bx + c and ax^2 + bx + c.Integers and Operation of Integers



Integers and Operation of IntegersMaria Theresa Bicar - Edar
Ėý
This document provides information and examples about integer operations:
- Addition of integers follows the same rules as normal addition, such as 20 + 10 = 30 and -40 + -60 = -100.
- Subtraction of integers is performed similarly to addition, such as -3 - 7 = -10 and 15 - 9 = 6.
- When multiplying integers, the product is positive if the signs are the same and negative if the signs are different, exemplified as -2 Ã 6 = -12 and 2 Ã -3 = -6.
- For division of integers, the quotient is positive if the signs are the same and negative if the signs are different, with examples like 12 ÷ -4 =Lecture 05 b radicals multiplication and division



Lecture 05 b radicals multiplication and divisionHazel Joy Chong
Ėý
This document discusses multiplying and dividing radical expressions. It provides examples of multiplying radicals with the same index by multiplying coefficients and radicands separately. It explains how to divide radicals by dividing coefficients and radicands, and rationalizing denominators by multiplying the numerator and denominator by a number to eliminate radicals in the denominator. The document also demonstrates multiplying radicals with different indices by applying the distributive property and keeping track of indices.Quadratic Equations in One Variables.pptx



Quadratic Equations in One Variables.pptxpandavlogsbyJM
Ėý
The document discusses solving quadratic equations in one variable of the form ax^2 + bx + c = 0. It provides examples of quadratic equations and shows how to rewrite them in standard form. It then covers methods for solving quadratic equations, including extracting square roots, factoring, completing the square, and using the quadratic formula. It also discusses the nature of the roots based on the discriminant and provides rules for determining the sum and product of the roots.Lesson 1.9 a adding and subtracting rational numbers



Lesson 1.9 a adding and subtracting rational numbersJohnnyBallecer
Ėý
To add or subtract fractions with the same denominator:
1. Add or subtract the numerators
2. Keep the original denominator
3. Simplify if possible
To add or subtract fractions with different denominators:
1. Find the least common denominator (LCD)
2. Convert all fractions to equivalent fractions with the LCD as the denominator
3. Add or subtract the numerators
4. Keep the LCD as the denominatorMultiplying Polynomials



Multiplying PolynomialsMary Ann Villanueva
Ėý
Multiplying polynomials can be done using three methods:
1. The distributive property, which involves multiplying each term of one polynomial with each term of the other.
2. FOIL (First, Outer, Inner, Last), which is a shortcut used for multiplying two binomials by multiplying the first, outer, inner, and last terms.
3. The box method, which involves drawing a box and writing one polynomial above and beside the box before distributing the multiplication similar to the distributive property.Understanding negative numbers



Understanding negative numbersWorserbay
Ėý
The document discusses negative numbers and how they relate to temperature scales. It provides examples of number lines that extend to the left of zero to demonstrate negative numbers. It then shows vertical and horizontal temperature scales and asks questions about finding missing numbers and comparing temperatures on the scales. Finally, it asks the reader to order a set of numbers from coldest to warmest based on their position on the temperature scale.PEMDAS - The Proper Order of Mathematical Operations



PEMDAS - The Proper Order of Mathematical OperationsMark (Mong) Montances
Ėý
The document discusses the proper order of mathematical operations known as PEMDAS. It covers absolute values, addition and subtraction of signed numbers, multiplication and division of signed numbers, and the order of operations using PEMDAS. Examples are provided to illustrate how to use PEMDAS to simplify expressions involving multiple operations. Exercises with answers are included to help readers practice applying these concepts.Quadratic Formula Presentation



Quadratic Formula Presentationanjuli1580
Ėý
The document introduces two methods for solving quadratic equations - factoring and graphing. It provides examples of equations that cannot be solved using these methods. It then introduces the quadratic formula as the method to use for equations that cannot be factored or graphed easily. It walks through identifying the a, b, and c coefficients needed for the quadratic formula. It provides examples of using the formula and encourages practicing with a worksheet.Quadratic Trinomial where a is not equal 1



Quadratic Trinomial where a is not equal 1Lorie Jane Letada
Ėý
This document provides instructions on factoring quadratic trinomials where the leading coefficient a is not equal to 1. It reviews the steps for factoring when a=1, which are to find the factors of the first term, list number pairs with the product of the last term and matching sum to the middle term, and write the factors. For aâ 1, the steps are to multiply the leading coefficient a and constant c, list number pairs with this product and matching sum to the middle term, and write the factors including the leading coefficient. An example demonstrates factoring 2x^2 - 9x + 4. The key difference when aâ 1 is accounting for the leading coefficient in the factors.Find the percentage of a number



Find the percentage of a numberSherina Isolica
Ėý
This document provides two methods for calculating percentages of numbers and examples of word problems involving percentages. Method 1 is to convert the percentage to a decimal and multiply it by the original number. Method 2 is to convert the percentage to a fraction with the percentage over 100 and multiply that by the original number. Several word problems follow that require calculating percentages of original amounts to determine final costs.Area of triangle



Area of triangleBruce Lightner
Ėý
This document discusses three methods for calculating the area of triangles: using the base and height, using two sides and the included angle, and using all three sides. It provides the formulas for each method and works through examples of calculating the area. Practice problems are included at the end to allow additional practice applying the different area calculation methods.Slope of a Line



Slope of a Linekaren wagoner
Ėý
This document contains a lesson on slope of a line from a mathematics course. It includes examples of calculating slope given two points on a line, identifying whether graphs represent constant or variable rates of change, and word problems applying slope to real-world contexts like cost of fruit and gas. The key points are that slope is defined as the ratio of rise over run, or change in y over change in x, and represents the constant rate of change for linear equations and functions.First Quarter - Chapter 2 - Quadratic Equation



First Quarter - Chapter 2 - Quadratic EquationVer Louie Gautani
Ėý
Okay, let's think through this with the new information:
* The equation modeling the height is: h = -16t^2 + vt + c
* The initial height (c) is still 2 feet
* The initial velocity (v) is now 20 feet/second
* The target height (h) is still 20 feet
So the equation is:
20 = -16t^2 + 20t + 2
0 = -16t^2 + 20t + 18 (subtract 20 from both sides)
Evaluating the discriminant:
(20)^2 - 4(-16)(-18) = 400 - 288 = 112
Since the discriminant is positiveIndex laws ppt



Index laws pptEdTechonGC Mallett
Ėý
Here are the solutions to the more difficult examples:
1) 43 x 47 / 46 = 43+7-6 = 44
2) 6p8 x 3p3 / 9p4 x p7 = 6p8+3-4-7 = 6p0 = 1
3) 83 / 85 = 83-5 = 78
4) (4-1)3 = (-1)3 = -1Rearranging Formulas



Rearranging FormulasPassy World
Ėý
The document discusses the "onion skin" method for transposing (rearranging) algebra formula equations. It explains that with this method, you draw concentric "skins" or circles around the equation, starting with the variable you want to isolate. You then work inward by applying the opposite operations to each term or part of the equation until the desired variable is alone on one side. It provides examples of using this method to transpose different types of equations, including ones with fractions, exponents, multiple variables, and square roots.Factoring and Box Method



Factoring and Box Methodswartzje
Ėý
This document discusses various techniques for factoring polynomials, including:
1. Factoring using the greatest common factor (GCF).
2. Factoring polynomials with 4 or more terms by grouping.
3. Factoring trinomials using factors that add up to the coefficient of the middle term.
4. Using the "box method" to factor trinomials where the coefficient of the x^2 term is not 1.
5. Factoring the difference of two squares using the formula a^2 - b^2 = (a + b)(a - b).The remainder theorem powerpoint



The remainder theorem powerpointJuwileene Soriano
Ėý
The document explains the Remainder Theorem in multiple ways using different examples and proofs. It states that the Remainder Theorem provides a test to determine if a polynomial f(x) is divisible by a polynomial of the form x-c. It proves that the remainder obtained when dividing f(x) by x-c is equal to the value of f(x) when x is substituted with c. It provides multiple examples working through applying the Remainder Theorem to determine if various polynomials are divisible.10.1 Distance and Midpoint Formulas



10.1 Distance and Midpoint Formulasswartzje
Ėý
1. The document discusses the distance and midpoint formulas in coordinate geometry.
2. It provides the formulas for finding the distance between two points and the midpoint of a line segment.
3. Examples are given of using the formulas to find distances and midpoints, as well as classifying triangles and finding endpoint coordinates given a midpoint.Odd and even functions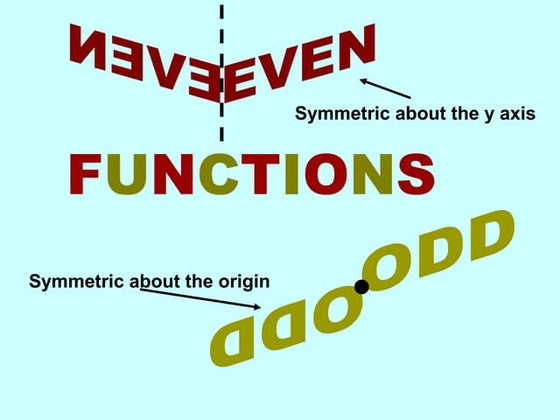



Odd and even functionsDebra Wallace
Ėý
The document defines even, odd, and neither functions based on their symmetry properties. An even function is symmetric about the y-axis, such that f(-x) = f(x). An odd function is symmetric about the origin, such that f(-x) = -f(x). A function is neither even nor odd if it does not satisfy those properties. Examples are provided to demonstrate how to determine if a given function is even, odd, or neither.Expanding two brackets



Expanding two bracketsPatryk Mamica
Ėý
The document discusses expanding and simplifying expressions within brackets. It provides examples of multiplying out terms within brackets for expressions like (2a + 3)(4a + 2) and (3b + 2)(4b - 6). The key steps are to multiply out each corresponding term, then collect like terms. Distributing negatives requires being careful about the signs of multiplied terms. The document encourages practicing expanding several example expressions within time limits to check understanding.Expansion and Factorisation of Algebraic Expressions 2.pptx



Expansion and Factorisation of Algebraic Expressions 2.pptxMitaDurenSawit
Ėý
This document discusses expanding and factorizing algebraic expressions. It begins by defining key terms like variables, constants, terms, coefficients, expressions and quadratic expressions. It then discusses how to add, subtract, expand and simplify algebraic expressions using the distributive law. Examples are provided to demonstrate expanding expressions using FOIL method and squaring a binomial. The document concludes with practice problems for students to expand different algebraic expressions.Factorising



Factorisingmathsteacher101
Ėý
The document provides examples of factorizing algebraic expressions. It begins with expanding simple expressions like 3g + 4 and finding the common factors of terms like 12 and 20. The next section provides more examples of factorizing expressions like x2 + 2x by finding the highest common factor. It emphasizes factorizing completely, like writing 3abc(ab4 + 9) instead of 9abc(ab4 + 9). The document concludes with an exercise for students to practice factorizing expressions and an extension with more challenging examples. It provides the opportunity for a 10 minute practice session to reinforce the steps of factorizing algebraic expressions.5 4 function notation



5 4 function notationhisema01
Ėý
This document introduces function notation and how to evaluate functions. It explains that f(x) represents the output of the function f for a given input x. Examples show different functions defined using this notation, such as f(x) = 2x + 1. The document also demonstrates how to evaluate functions for given x-values, find x-values for which a function is a certain amount, and graph functions using this notation. It compares translating and shifting graphs of functions.Solving equations



Solving equationsRussell Shaw
Ėý
This document discusses how to solve equations and inequalities. It covers topics such as: balancing equations, combining like terms, expanding brackets, rearranging formulas to change the subject, representing inequalities on number lines, and solving one-step and two-step inequalities. Examples are provided to demonstrate each concept and practice problems are included for readers to try.Solving Equations Transformable to Quadratic Equation Including Rational Alge...



Solving Equations Transformable to Quadratic Equation Including Rational Alge...Cipriano De Leon
Ėý
Provides examples and solutions on how to solve equations that is transformable to quadratic equation and rational algebraic equations.More Related Content
What's hot (20)
Multiplying Polynomials



Multiplying PolynomialsMary Ann Villanueva
Ėý
Multiplying polynomials can be done using three methods:
1. The distributive property, which involves multiplying each term of one polynomial with each term of the other.
2. FOIL (First, Outer, Inner, Last), which is a shortcut used for multiplying two binomials by multiplying the first, outer, inner, and last terms.
3. The box method, which involves drawing a box and writing one polynomial above and beside the box before distributing the multiplication similar to the distributive property.Understanding negative numbers



Understanding negative numbersWorserbay
Ėý
The document discusses negative numbers and how they relate to temperature scales. It provides examples of number lines that extend to the left of zero to demonstrate negative numbers. It then shows vertical and horizontal temperature scales and asks questions about finding missing numbers and comparing temperatures on the scales. Finally, it asks the reader to order a set of numbers from coldest to warmest based on their position on the temperature scale.PEMDAS - The Proper Order of Mathematical Operations



PEMDAS - The Proper Order of Mathematical OperationsMark (Mong) Montances
Ėý
The document discusses the proper order of mathematical operations known as PEMDAS. It covers absolute values, addition and subtraction of signed numbers, multiplication and division of signed numbers, and the order of operations using PEMDAS. Examples are provided to illustrate how to use PEMDAS to simplify expressions involving multiple operations. Exercises with answers are included to help readers practice applying these concepts.Quadratic Formula Presentation



Quadratic Formula Presentationanjuli1580
Ėý
The document introduces two methods for solving quadratic equations - factoring and graphing. It provides examples of equations that cannot be solved using these methods. It then introduces the quadratic formula as the method to use for equations that cannot be factored or graphed easily. It walks through identifying the a, b, and c coefficients needed for the quadratic formula. It provides examples of using the formula and encourages practicing with a worksheet.Quadratic Trinomial where a is not equal 1



Quadratic Trinomial where a is not equal 1Lorie Jane Letada
Ėý
This document provides instructions on factoring quadratic trinomials where the leading coefficient a is not equal to 1. It reviews the steps for factoring when a=1, which are to find the factors of the first term, list number pairs with the product of the last term and matching sum to the middle term, and write the factors. For aâ 1, the steps are to multiply the leading coefficient a and constant c, list number pairs with this product and matching sum to the middle term, and write the factors including the leading coefficient. An example demonstrates factoring 2x^2 - 9x + 4. The key difference when aâ 1 is accounting for the leading coefficient in the factors.Find the percentage of a number



Find the percentage of a numberSherina Isolica
Ėý
This document provides two methods for calculating percentages of numbers and examples of word problems involving percentages. Method 1 is to convert the percentage to a decimal and multiply it by the original number. Method 2 is to convert the percentage to a fraction with the percentage over 100 and multiply that by the original number. Several word problems follow that require calculating percentages of original amounts to determine final costs.Area of triangle



Area of triangleBruce Lightner
Ėý
This document discusses three methods for calculating the area of triangles: using the base and height, using two sides and the included angle, and using all three sides. It provides the formulas for each method and works through examples of calculating the area. Practice problems are included at the end to allow additional practice applying the different area calculation methods.Slope of a Line



Slope of a Linekaren wagoner
Ėý
This document contains a lesson on slope of a line from a mathematics course. It includes examples of calculating slope given two points on a line, identifying whether graphs represent constant or variable rates of change, and word problems applying slope to real-world contexts like cost of fruit and gas. The key points are that slope is defined as the ratio of rise over run, or change in y over change in x, and represents the constant rate of change for linear equations and functions.First Quarter - Chapter 2 - Quadratic Equation



First Quarter - Chapter 2 - Quadratic EquationVer Louie Gautani
Ėý
Okay, let's think through this with the new information:
* The equation modeling the height is: h = -16t^2 + vt + c
* The initial height (c) is still 2 feet
* The initial velocity (v) is now 20 feet/second
* The target height (h) is still 20 feet
So the equation is:
20 = -16t^2 + 20t + 2
0 = -16t^2 + 20t + 18 (subtract 20 from both sides)
Evaluating the discriminant:
(20)^2 - 4(-16)(-18) = 400 - 288 = 112
Since the discriminant is positiveIndex laws ppt



Index laws pptEdTechonGC Mallett
Ėý
Here are the solutions to the more difficult examples:
1) 43 x 47 / 46 = 43+7-6 = 44
2) 6p8 x 3p3 / 9p4 x p7 = 6p8+3-4-7 = 6p0 = 1
3) 83 / 85 = 83-5 = 78
4) (4-1)3 = (-1)3 = -1Rearranging Formulas



Rearranging FormulasPassy World
Ėý
The document discusses the "onion skin" method for transposing (rearranging) algebra formula equations. It explains that with this method, you draw concentric "skins" or circles around the equation, starting with the variable you want to isolate. You then work inward by applying the opposite operations to each term or part of the equation until the desired variable is alone on one side. It provides examples of using this method to transpose different types of equations, including ones with fractions, exponents, multiple variables, and square roots.Factoring and Box Method



Factoring and Box Methodswartzje
Ėý
This document discusses various techniques for factoring polynomials, including:
1. Factoring using the greatest common factor (GCF).
2. Factoring polynomials with 4 or more terms by grouping.
3. Factoring trinomials using factors that add up to the coefficient of the middle term.
4. Using the "box method" to factor trinomials where the coefficient of the x^2 term is not 1.
5. Factoring the difference of two squares using the formula a^2 - b^2 = (a + b)(a - b).The remainder theorem powerpoint



The remainder theorem powerpointJuwileene Soriano
Ėý
The document explains the Remainder Theorem in multiple ways using different examples and proofs. It states that the Remainder Theorem provides a test to determine if a polynomial f(x) is divisible by a polynomial of the form x-c. It proves that the remainder obtained when dividing f(x) by x-c is equal to the value of f(x) when x is substituted with c. It provides multiple examples working through applying the Remainder Theorem to determine if various polynomials are divisible.10.1 Distance and Midpoint Formulas



10.1 Distance and Midpoint Formulasswartzje
Ėý
1. The document discusses the distance and midpoint formulas in coordinate geometry.
2. It provides the formulas for finding the distance between two points and the midpoint of a line segment.
3. Examples are given of using the formulas to find distances and midpoints, as well as classifying triangles and finding endpoint coordinates given a midpoint.Odd and even functions



Odd and even functionsDebra Wallace
Ėý
The document defines even, odd, and neither functions based on their symmetry properties. An even function is symmetric about the y-axis, such that f(-x) = f(x). An odd function is symmetric about the origin, such that f(-x) = -f(x). A function is neither even nor odd if it does not satisfy those properties. Examples are provided to demonstrate how to determine if a given function is even, odd, or neither.Expanding two brackets



Expanding two bracketsPatryk Mamica
Ėý
The document discusses expanding and simplifying expressions within brackets. It provides examples of multiplying out terms within brackets for expressions like (2a + 3)(4a + 2) and (3b + 2)(4b - 6). The key steps are to multiply out each corresponding term, then collect like terms. Distributing negatives requires being careful about the signs of multiplied terms. The document encourages practicing expanding several example expressions within time limits to check understanding.Expansion and Factorisation of Algebraic Expressions 2.pptx



Expansion and Factorisation of Algebraic Expressions 2.pptxMitaDurenSawit
Ėý
This document discusses expanding and factorizing algebraic expressions. It begins by defining key terms like variables, constants, terms, coefficients, expressions and quadratic expressions. It then discusses how to add, subtract, expand and simplify algebraic expressions using the distributive law. Examples are provided to demonstrate expanding expressions using FOIL method and squaring a binomial. The document concludes with practice problems for students to expand different algebraic expressions.Factorising



Factorisingmathsteacher101
Ėý
The document provides examples of factorizing algebraic expressions. It begins with expanding simple expressions like 3g + 4 and finding the common factors of terms like 12 and 20. The next section provides more examples of factorizing expressions like x2 + 2x by finding the highest common factor. It emphasizes factorizing completely, like writing 3abc(ab4 + 9) instead of 9abc(ab4 + 9). The document concludes with an exercise for students to practice factorizing expressions and an extension with more challenging examples. It provides the opportunity for a 10 minute practice session to reinforce the steps of factorizing algebraic expressions.5 4 function notation



5 4 function notationhisema01
Ėý
This document introduces function notation and how to evaluate functions. It explains that f(x) represents the output of the function f for a given input x. Examples show different functions defined using this notation, such as f(x) = 2x + 1. The document also demonstrates how to evaluate functions for given x-values, find x-values for which a function is a certain amount, and graph functions using this notation. It compares translating and shifting graphs of functions.Solving equations



Solving equationsRussell Shaw
Ėý
This document discusses how to solve equations and inequalities. It covers topics such as: balancing equations, combining like terms, expanding brackets, rearranging formulas to change the subject, representing inequalities on number lines, and solving one-step and two-step inequalities. Examples are provided to demonstrate each concept and practice problems are included for readers to try.Similar to 1a factorization ok (20)
Solving Equations Transformable to Quadratic Equation Including Rational Alge...



Solving Equations Transformable to Quadratic Equation Including Rational Alge...Cipriano De Leon
Ėý
Provides examples and solutions on how to solve equations that is transformable to quadratic equation and rational algebraic equations.rational equation transformable to quadratic equation.pptx



rational equation transformable to quadratic equation.pptxRizaCatli2
Ėý
1. The document provides examples for solving quadratic equations that are not in standard form by transforming them into standard form ax2 + bx + c = 0 and then using methods like factoring or the quadratic formula.
2. It also gives examples for solving rational algebraic equations by multiplying both sides by the least common denominator to obtain a quadratic equation, transforming it into standard form, and then solving.
3. The examples cover topics like solving for the solution set, checking solutions, and using the quadratic formula to solve transformed equations.Diamond and box factoring student version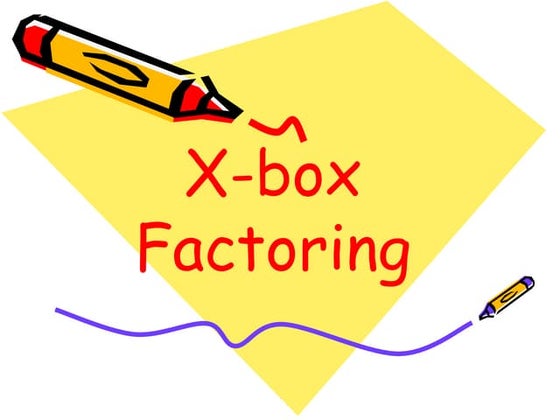



Diamond and box factoring student versionvelmon23
Ėý
The document explains and provides examples of how to factor quadratic expressions using the "x-box" method. This method involves drawing an x-box with the coefficients of the expression and identifying the greatest common factor and two binomial factors that multiply to the original expression. Three examples are provided and worked through step-by-step to demonstrate how to set up and solve factoring problems using the x-box method.Diamond and box factoring student version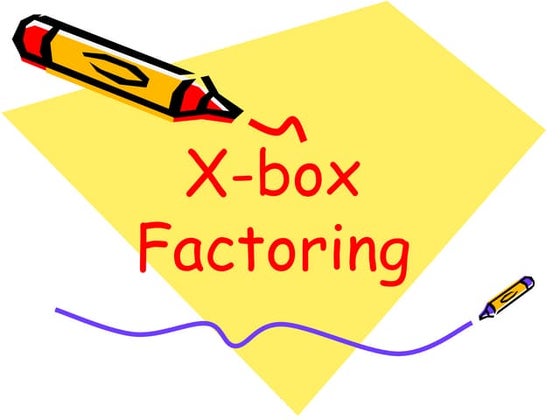



Diamond and box factoring student versionvelmon23
Ėý
The document provides instructions and examples for factoring polynomials using the x-box method. It explains that the x-box method involves writing the polynomial terms in an x-box diagram and identifying the greatest common factor and factor pairs. Three examples of polynomials are then factored using the x-box method.Factoring 15.3 and 15.4 Grouping and Trial and Error



Factoring 15.3 and 15.4 Grouping and Trial and Errorswartzje
Ėý
This document discusses various methods for factoring trinomials of the form Ax^2 + Bx + C. It begins by outlining three main methods: trial and error, factoring by grouping, and the box method. It then provides examples of using the factoring by grouping method, demonstrating how to find two numbers whose product is AC and sum is B. The document also covers special cases like factoring the difference of squares using the form A^2 - B^2 = (A-B)(A+B), and factoring perfect square trinomials using the form A^2 + 2AB + B^2 = (A+B)^2. In all, it thoroughly explains the step-bysim-140907230908-phpapp01.pptx



sim-140907230908-phpapp01.pptxJeffreyEnriquez10
Ėý
This document provides an overview of solving quadratic equations by factoring. It discusses identifying quadratic equations, rewriting them in standard form, factoring trinomials in the form x^2 + bx + c, and determining roots. Several examples of factoring trinomials and solving quadratic equations are shown. Activities include identifying quadratic equations, rewriting equations in standard form, factoring trinomials, and solving equations by factoring. The document provides resources for further learning about quadratic equations and factoring.Solving Quadratic Equations



Solving Quadratic EquationsCipriano De Leon
Ėý
Provides example on how to solve quadratic equations using extracting square roots, factoring, completing the square and quadratic formula.Q1-W1-Factoring Polynomials.pptx



Q1-W1-Factoring Polynomials.pptxTherezaNoble
Ėý
This document provides instruction on factoring polynomials. It covers several factoring methods including common monomial factoring, difference of squares, sum and difference of cubes, perfect square trinomials, and general trinomials. Examples are provided for each method. The objectives are to determine appropriate factoring methods, factor polynomials completely using various techniques, and solve problems involving polynomial factors.Business Math Chapter 3



Business Math Chapter 3Nazrin Nazdri
Ėý
The document covers systems of linear equations, including how to solve them using substitution and elimination methods. It provides examples of solving systems of equations with one solution, no solution, and infinitely many solutions. Quadratic equations are also discussed, including how to solve them by factoring, using the quadratic formula, and identifying the nature of solutions based on the discriminant.Factoring



FactoringMartinGeraldine
Ėý
The document discusses the zero factor theorem and provides examples of using it to solve quadratic equations. The zero factor theorem states that if p and q are algebraic expressions, then pq = 0 if and only if p = 0 or q = 0. This means a quadratic equation can be solved by factoring it into two linear factors and setting each factor equal to zero. Five examples are provided that show factoring quadratic equations, applying the zero factor theorem to set the factors equal to zero, and solving for the roots.Sum and product of the roots of a



Sum and product of the roots of aMartinGeraldine
Ėý
The document discusses how to find the sum and product of the roots of a quadratic equation ax^2 + bx + c = 0. It provides the formulas that the sum of the roots is equal to -b/a and the product of the roots is equal to c/a. It then works through two examples of using these formulas to find the sum and product of the roots of equations 3x^2 + 4x - 5 = 0 and 4x^2 - 29x - 24 = 0. The solutions show the steps of identifying a, b, c and then applying the formulas to determine the sum and product of the roots for each example equation.Algebra 2 Section 3-4



Algebra 2 Section 3-4Jimbo Lamb
Ėý
This document discusses solving quadratic equations by factoring. It begins by defining factored form and the FOIL method. It then provides examples of writing quadratic equations in standard form given roots, factoring quadratics using special products like difference of squares, and solving factored quadratic equations. The examples illustrate each step of solving quadratics by factoring, from rewriting the equation in factored form to setting each factor equal to 0 to obtain the solutions.College algebra real mathematics real people 7th edition larson solutions manual



College algebra real mathematics real people 7th edition larson solutions manualJohnstonTBL
Ėý
This document contains information about the College Algebra Real Mathematics Real People 7th Edition Larson textbook including:
- A link to download the solutions manual and test bank for the textbook
- An overview of the content covered in Chapter 2 on solving equations and inequalities, including linear equations, identities, conditionals, and more.
- 51 example problems from Chapter 2 with step-by-step solutions.Writing quadratic equation



Writing quadratic equationMartinGeraldine
Ėý
The document discusses quadratic equations and their roots. It provides examples of writing the quadratic equation given the roots. Specifically:
1) It shows how to write the quadratic equation x^2 - 8x + 12 = 0 given the roots are 2 and 6.
2) It shows how to write the quadratic equation 2x^2 - 9x + 4 = 0 given the roots are 4 and 1/2.
3) The key steps are finding the sum and product of the roots, and using those values to write the quadratic equation in the form x^2 - (sum of roots)x + (product of roots) = 0.Quadratic equations



Quadratic equationsLenie Zapata
Ėý
Quadratic Equations
In One Variable
1. Quadratic Equation
an equation of the form
ax2 + bx + c = 0
where a, b, and c are real numbers
2.Types of Quadratic Equations
Complete Quadratic
3x2 + 5x + 6 = 0
Incomplete/Pure Quadratic Equation
3x2 - 6 = 0
3.Solving an Incomplete Quadratic
4.Example 1. Solve: x2 â 4 = 0
Solution:
x2 â 4 = 0
x2 = 4
âxÂē = â4
x = Âą 2
5.Example 2. Solve: 5xÂē - 11 = 49
Solution:
5xÂē - 11 = 49
5xÂē = 49 + 11
5xÂē = 60
xÂē = 12
x = Âąâ12
x = Âą2â3
6.Solving Quadratic Equation
7.By Factoring
Place all terms in the left member of the equation, so that the right member is zero.
Factor the left member.
Set each factor that contains the unknown equal to zero.
Solve each of the simple equations thus formed.
Check the answers by substituting them in the original equation.
8.Example: xÂē = 6x - 8
Solution:
xÂē = 6x â 8
xÂē - 6x + 8 = 0
(x â 4)(x â 2) = 0
x â 4 = 0 | x â 2 = 0
x = 4 x = 2
9.By Completing the Square
Write the equation with the variable terms in the left member and the constant term in the right member.
If the coefficient of xÂē is not 1, divide every term by this coefficient so as to make the coefficient of xÂē equal to 1.
Take one-half the coefficient of x, square this quantity, and add the result to both members.
Find the square root of both members, placing a Âą sign before the square root of the right member.
Solve the resulting equation for x.
10.Example: xÂē - 8x + 7 = 0
11.By Quadratic Formula
Example: 3xÂē - 2x - 7 = 0
12.Solve the following:
1. xÂē - 15x â 56 = 0
2. 7xÂē = 2x + 6
3. 9xÂē - 3x + 8 = 0
4. 8xÂē + 9x -144 = 0
5. 2xÂē - 3 + 12x
13.Activity:
Solve the following quadratic formula.
By Factoring By Quadratic Formula
1. xÂē - 5x + 6 = 0 1. xÂē - 7x + 6 = 0
2. 3 xÂē = x + 2 2. 10 xÂē - 13x â 3 = 0
3. 2 xÂē - 11x + 12 = 0 3. x (5x â 4) = 2
By Completing the Square
1. xÂē + 6x + 5 = 0
2. xÂē - 8x + 3 = 0
3. 2 xÂē + 3x â 5 = 0
Recently uploaded (20)
Administrative bodies( D and C Act, 1940



Administrative bodies( D and C Act, 1940P.N.DESHMUKH
Ėý
These presentation include information about administrative bodies such as D.T.A.B
CDL AND DCC, etc.Azure Administrator Interview Questions By ScholarHat



Azure Administrator Interview Questions By ScholarHatScholarhat
Ėý
Azure Administrator Interview Questions By ScholarHatInterim Guidelines for PMES-DM-17-2025-PPT.pptx



Interim Guidelines for PMES-DM-17-2025-PPT.pptxsirjeromemanansala
Ėý
This is the latest issuance on PMES as replacement of RPMS. Kindly message me to gain full access of the presentation. BISNIS BERKAH BERANGKAT KE MEKKAH ISTIKMAL SYARIAH



BISNIS BERKAH BERANGKAT KE MEKKAH ISTIKMAL SYARIAHcoacharyasetiyaki
Ėý
BISNIS BERKAH BERANGKAT KE MEKKAH ISTIKMAL SYARIAHAzure Data Engineer Interview Questions By ScholarHat



Azure Data Engineer Interview Questions By ScholarHatScholarhat
Ėý
Azure Data Engineer Interview Questions By ScholarHatFunctional Muscle Testing of Facial Muscles.pdf



Functional Muscle Testing of Facial Muscles.pdfSamarHosni3
Ėý
Functional Muscle Testing of Facial Muscles.pdfHow to create security group category in Odoo 17



How to create security group category in Odoo 17Celine George
Ėý
This slide will represent the creation of security group category in odoo 17. Security groups are essential for managing user access and permissions across different modules. Creating a security group category helps to organize related user groups and streamline permission settings within a specific module or functionality.Blind spots in AI and Formulation Science, IFPAC 2025.pdf



Blind spots in AI and Formulation Science, IFPAC 2025.pdfAjaz Hussain
Ėý
The intersection of AI and pharmaceutical formulation science highlights significant blind spotsâsystemic gaps in pharmaceutical development, regulatory oversight, quality assurance, and the ethical use of AIâthat could jeopardize patient safety and undermine public trust. To move forward effectively, we must address these normalized blind spots, which may arise from outdated assumptions, errors, gaps in previous knowledge, and biases in language or regulatory inertia. This is essential to ensure that AI and formulation science are developed as tools for patient-centered and ethical healthcare.ASP.NET Interview Questions PDF By ScholarHat



ASP.NET Interview Questions PDF By ScholarHatScholarhat
Ėý
ASP.NET Interview Questions PDF By ScholarHatHow to Configure Deliver Content by Email in Odoo 18 Sales



How to Configure Deliver Content by Email in Odoo 18 SalesCeline George
Ėý
In this slide, weâll discuss on how to configure proforma invoice in Odoo 18 Sales module. A proforma invoice is a preliminary invoice that serves as a commercial document issued by a seller to a buyer.ASP.NET Web API Interview Questions By Scholarhat



ASP.NET Web API Interview Questions By ScholarhatScholarhat
Ėý
ASP.NET Web API Interview Questions By ScholarhatNUTRITIONAL ASSESSMENT AND EDUCATION - 5TH SEM.pdf



NUTRITIONAL ASSESSMENT AND EDUCATION - 5TH SEM.pdfDolisha Warbi
Ėý
NUTRITIONAL ASSESSMENT AND EDUCATION, Introduction, definition, types - macronutrient and micronutrient, food pyramid, meal planning, nutritional assessment of individual, family and community by using appropriate method, nutrition education, nutritional rehabilitation, nutritional deficiency disorder, law/policies regarding nutrition in India, food hygiene, food fortification, food handling and storage, food preservation, food preparation, food purchase, food consumption, food borne diseases, food poisoningEntity Framework Interview Questions PDF By ScholarHat



Entity Framework Interview Questions PDF By ScholarHatScholarhat
Ėý
Entity Framework Interview Questions PDF By ScholarHatEffective Product Variant Management in Odoo 18



Effective Product Variant Management in Odoo 18Celine George
Ėý
In this slide weâll discuss on the effective product variant management in Odoo 18. Odoo concentrates on managing product variations and offers a distinct area for doing so. Product variants provide unique characteristics like size and color to single products, which can be managed at the product template level for all attributes and variants or at the variant level for individual variants.Unit 1 Computer Hardware for Educational Computing.pptx



Unit 1 Computer Hardware for Educational Computing.pptxRomaSmart1
Ėý
Computers have revolutionized various sectors, including education, by enhancing learning experiences and making information more accessible. This presentation, "Computer Hardware for Educational Computing," introduces the fundamental aspects of computers, including their definition, characteristics, classification, and significance in the educational domain. Understanding these concepts helps educators and students leverage technology for more effective learning.Comprehensive Guide to Antibiotics & Beta-Lactam Antibiotics.pptx



Comprehensive Guide to Antibiotics & Beta-Lactam Antibiotics.pptxSamruddhi Khonde
Ėý
ðĒ Comprehensive Guide to Antibiotics & Beta-Lactam Antibiotics
ðŽ Antibiotics have revolutionized medicine, playing a crucial role in combating bacterial infections. Among them, Beta-Lactam antibiotics remain the most widely used class due to their effectiveness against Gram-positive and Gram-negative bacteria. This guide provides a detailed overview of their history, classification, chemical structures, mode of action, resistance mechanisms, SAR, and clinical applications.
ð What Youâll Learn in This Presentation
â
History & Evolution of Antibiotics
â
Cell Wall Structure of Gram-Positive & Gram-Negative Bacteria
â
Beta-Lactam Antibiotics: Classification & Subtypes
â
Penicillins, Cephalosporins, Carbapenems & Monobactams
â
Mode of Action (MOA) & Structure-Activity Relationship (SAR)
â
Beta-Lactamase Inhibitors & Resistance Mechanisms
â
Clinical Applications & Challenges.
ð Why You Should Check This Out?
Essential for pharmacy, medical & life sciences students.
Provides insights into antibiotic resistance & pharmaceutical trends.
Useful for healthcare professionals & researchers in drug discovery.
ð Swipe through & explore the world of antibiotics today!
ð Like, Share & Follow for more in-depth pharma insights!1a factorization ok
- 1. Curriculum on factorization Factorization a2-b2, trinomial with perfect square, trinomial in the form of ax2 ïą bxï ïą c. PRESENTED BY MANINATH NEUPANE CLASS 8
- 3. Q 1.Factorise: 2ax+4ay ax ay a x a y a x a x a y a y a y a y 2a (x+2y)
- 4. Q 1.Factorise: 2ax+4ay =2 . =2.2 2ax + 4aySolution: 2ax 4ay = a . x . a . y ( + ) Rule 1. We have to take common factors as common and remaining factors should be kept inside parenthesis.
- 5. Q 2.Factorise: 4a2b-6ab2+10ab ( 4a2b-6ab2+10ab 4a2b 6ab2 10ab =2. 2. a. a. b 2. 3. a. b. b= 2. 5. a. b= - + ) Solution: = Rule 1. We have to take common factors as common and remaining factors should be kept inside parenthesis.
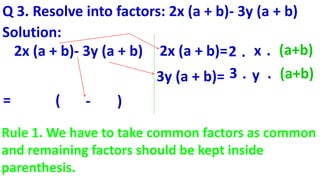
- 6. Q 3. Resolve into factors: 2x (a + b)- 3y (a + b) Solution: 2x (a + b)- 3y (a + b) 2x (a + b)= 3y (a + b)= 2 . x . (a+b) 3 . y . (a+b) = ( - ) Rule 1. We have to take common factors as common and remaining factors should be kept inside parenthesis.
- 7. Q 4.Factorise: a2-ax+ab-bx Solution: a2-ax + ab-bx = ( a2= a . a ax= a . x- ) + b(a - x) =(a-x)(a+b) Rule 2. We have to arrange the terms in groups such that each group has common factor.
- 8. Q 5.Factorise: a(x2-y2)+x(y2-a2) Solution: a(x2-y2)+x(y2-a2) =ax2-ay2+xy2-xa2 =ax2+xy2-ay2-xa2 =x(ax+y2)-a(y2+xa) =x(ax+y2)-a(xa+y2) =(ax+y2)(x-a) We have to simplify the expression first so that we can factorize.
- 9. Q 6.Factorise: x2-9 Solution: x 2 - 9 = x 2 â 3 2 âī x2 â 32 = a 2 â b 2 Let x=a and 3=b = (a â b)(a + b) = ( x â 3 )( x + 3 ) a 2 â b 2 = ( a â b ) ( a + b ) Putting the value of a and b. Rule 3. We have to use the suitable formula.
- 10. Q 6.Factorise: x2-9 Solution: x 2 - 9 = x 2 â 3 2 = ( x â 3 )( x + 3 ) a 2 â b 2 = ( a â b ) ( a + b ) Rule 3. We have to use the suitable formula.
- 11. Q 6.Factorise: x2-9 Solution: x 2 - 9 = x 2 â 3 2 = ( x â 3 )( x + 3 ) a 2 â b 2 = ( a â b ) ( a + b ) Rule 3. We have to use the suitable formula.
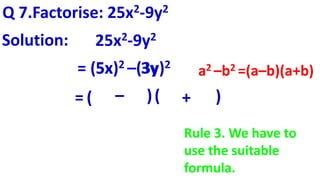
- 12. Q 7.Factorise: 25x2-9y2 Solution: 25x2-9y2 a2 âb2 =(aâb)(a+b)= (5x)2 â(3y)2 = ( 5x â 3y )( 5x + 3y ) Rule 3. We have to use the suitable formula.
- 13. Q 8.Factorise: a4-16 Solution: a4-16 =(a2)2-42 =(a2-4)(a2+4) =(a2-22)(a2+4) =(a-2)(a+2)(a2+4) a2 âb2 =(aâb)(a+b) Rule 3. We have to use the suitable formula.
- 14. Q 9.Factorise: (x2+y2)2-x2y2 Solution: (x2+y2)2-x2y2 =(x2+y2)2-(xy)2 ={(x2+y2)-(xy)}{(x2+y2)+(xy)} =(x2+y2-xy)(x2+y2+xy) =(x2-xy+y2)(x2+xy+y2) a2 âb2 =(aâb)(a+b) Rule 3. We have to use the suitable formula.
- 15. Q 10.Factorise: 4-(a-b)2 Solution: 4-(a-b)2 =22-(a-b)2 ={2-(a-b)}{2+(a-b)} =(2-a+b)(2+a-b) a2 âb2 =(aâb)(a+b) Rule 3. We have to use the suitable formula.
- 16. Q 11. Simplify by factorization process: 722-622. Solution: 722-622 =(72-62)(72+62) =10Ã134 =1340 We have to use the suitable formula.
- 17. Q 12. Simplify by factorization process: 101Ã99 Solution: 101Ã99 = (100+1)(100-1) = (10000-1) = 9999 We have to use the suitable formula.
- 18. Q 13. If a+b=8 and ab =15, find the value of a and b. numbers(a & b) sum(a+b) = 8 product(a.b) =15 3+5=8 1.7=7 âīNumbers are 3 &5. When a=3 then b=5 and when a=5 then b=3. 1 and 7 2 and 6 3 and 5 1+7=8 2+6=8 2.6=12 3.5=15 Solution: We use hit and trial method.
- 19. Q 14. If a+b=-10 and ab=24,find the value of a and b. numbers(a & b) a.b=24 a+b=-10 -3.-8=24 -1-24=-25 âīNumbers are -4 & -6. When a=-4 then b=-6 and when a=-6 then b=-4. -1 and -24 -2 and -12 -3 and -8 -1.-24=24 -2.-12=24 -2-12=-14 -3-8=-11 Solution: -4 and -6 -4.-6=24 -4-6=-10 We use hit and trial method.
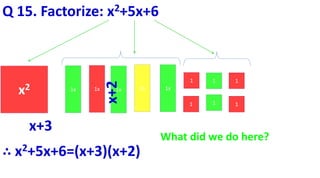
- 20. Q 15. Factorize: x2+5x+6 x2 1x 1x 1x 1x 1x 1 1 1 1 1 1 x+3 x+2 âī x2+5x+6=(x+3)(x+2) What did we do here?
- 21. Q 15. Factorize: x2+5x+6 Solution: x2+5x+6 Rule 4. Method 1. Multiply coefficient of x2 and constant term. 1Ã6=6(product) 2. Find the possible factors of the product 6. 6=1Ã6 6=2Ã3 3. Remember the sign of constant term. = x2+(3+2)x+6 = x2+3x+2x+6 = x(x+3)+2(x+3) = (x+3)(x+2) 4. If sign of constant term is + then choose the pair of factors whose sum is 5 (coefficient of x)
- 22. Q 16. Resolve into factors: x2-9x+20 Rule 4. Method 1. Multiply coefficient of x2 and constant term. 1Ã20=20(product) 2. Find the possible factors of the product 20. 20=1Ã20 20=2Ã10 4. If sign of constant term is + then choose the pair of factors whose sum is 9 (coefficient of x) 20=4Ã5 Solution: x2-9x+20 = x2-(5+4)x+20 = x2-5x-4x+20 = x(x-5)-4(x-5) = (x-5)(x-4) 3. Remember the sign of constant term.
- 23. Q 17. Factorize: x2+3x-18 Rule 4. Method 1. Multiply coefficient of x2 and constant term. 1Ã18=18(product) 2. Find the possible factors of the product 18. 18=1Ã18 18=2Ã9 18=3Ã6 Solution: x2+3x-18 =x2+(6-3)x-18 =x2+6x-3x-18 =x(x+6)-3(x+6) =(x+6)(x-3) 4. If sign of constant term is - then choose the pair of factors whose difference is 3 (coefficient of x) 3. Remember the sign of constant term.
- 24. Q 18. Factorize: x2-5x-14 Rule 4. Method 1. Multiply coefficient of x2 and constant term. 1Ã14=14(product) 2. Find the possible factors of the product 14. 14=1Ã14 14=2Ã7 4. If sign of constant term is - then choose the pair of factors whose difference is 3 (coefficient of x) 3. Remember the sign of constant term. Solution: x2-5x-14 =x2-(7-2)x-14 =x2-7x+2x-14 =x(x-7)+2(x-7) =(x-7)(x+2)
- 25. Q 19. Factorize: 2x2+7x+3 Rule 4. Method 1. Multiply coefficient of x2 and constant term. 2Ã3=6(product) 2. Find the possible factors of the product 6. 6=1Ã6 6=2Ã3 4. If sign of constant term is + then choose the pair of factors whose sum is 7 (coefficient of x) 3. Remember the sign of constant term. Solution: 2x2+7x+3 =2x2+7x+3 =2x2+(6+1)x+3 =2x2+6x+1x+3 =2x(x+3)+1(x+3) =(x+3)(2x+1)
- 26. Q 20. Factorize: x2+x-30 Solution: x2+x-30 Rule 4. Method 1. Multiply coefficient of x2 and constant term. 1Ã30=30(product) 2. Find the possible factors of the product 30. 30=1Ã30 30=2Ã15 4. If sign of constant term is - then choose the pair of factors whose difference is 1 (coefficient of x). 3. Remember the sign of constant term. 30=3Ã10 30=5Ã6 =x2+x-30 =x2+(6-5)x-30 =x2+6x-5x-30 =x(x+6)-5(x+6) =(x+6)(x-5)
- 27. Q 21. Factorize: x2+4x+4 Solution: x2+4x+4 =x2+2.x.2+22 =(x+2)2 Rule 3. We have to use the suitable formula. Rule 4. Or,
- 28. Q 22. Factorize: 4x2-12x+9 Solution: 4x2-12x+9 = (2x)2-2.2x.3+32 = (2x-3)2 Rule 3. We have to use the suitable formula. Rule 4. Or,
- 29. Q 23. Factorize: 4x â 8 Q 24. Factorize: 4x3 - 6x2 + 8x Q 25. Factorize: xm + ym + xa + ya Q 26. Factorize: a( a + 4 ) + 2 ( a + 4 ) Q 27. Factorize: a( a â 3 ) - 4( 3 â a ) Q 28. Factorize: â a â b + 1 + ab Q 29. Factorize: x2y + xy2z + zx + yz2
- 30. Q 30. Factorize: x2 â 1 Q 31. Factorize: 4x2 â 9b2 Q 32. Factorize: 1-36x2 Q 33. Factorize: x2-36 Q 34. Factorize: 16a2 â 25b2 Q 35. Factorize: 72x3 â 50x Q 36. Find the area of shaded part. y y 2 2
- 31. Q 37. Factorize: x2 + 10x +25 Q 38. Factorize: p2 â 24p+144 Q 39. Factorize: 9a2 â 66a + 121 Q 40. Factorize: x2 + 9x +18 Q 41. Factorize: x2 - 9x +18 Q 42. Factorize: x2 + 3x â 18 Q 43. Factorize: x2 - 3x â 18 Q 44. Factorize: 15x2 â x â 2 Q 45. Factorize: 125x3 + 8a3 Q 46. Factorize: (x â y )3 + 8(x + y)3