2-3 Conditional Statements.ppt
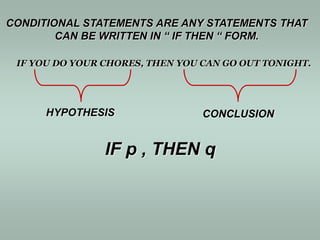
- 2. CONDITIONAL STATEMENTS ARE ANY STATEMENTS THAT CAN BE WRITTEN IN ŌĆ£ IF THEN ŌĆ£ FORM. IF YOU DO YOUR CHORES, THEN YOU CAN GO OUT TONIGHT. HYPOTHESIS CONCLUSION IF p , THEN q
- 3. IF YOU DO YOUR CHORES, THEN YOU CAN GO OUT TONIGHT. IF YOU CAN GO OUT TONIGHT , THEN YOU DID YOUR CHORES. IF YOU CANŌĆÖT GO OUT TONIGHT , THEN YOU DIDNŌĆÖT DO YOUR CHORES . CONDITIONAL STATEMENT: IF p , THEN q ( p q ) CONVERSE : IF q , THEN p ( q p ) INVERSE IF NOT p , THEN NOT q ( ~ p ~ q ) IF YOU DONŌĆÖT DO YOUR CHORES, THEN YOU CANŌĆÖT GO OUT TONIGHT. CONTRAPOSITIVE : IF NOT q , THEN NOT p ( ~ q ~ p )
- 4. WRITING A CONDITIONAL IN ŌĆ£ IF THEN ŌĆ£ FORM SOMETIMES YOU MUST ADD INFORMATION OR CHANGE THE WORDING OF A STATEMENT TO PUT IT IN ŌĆ£ IF THEN ŌĆ£ FORM. AN ANGLE WITH A MEASURE LESS THAN 90 IS ACUTE. IF AN ANGLE HAS A MEASURE LESS THAN 90, THEN IT IS ACUTE PARALLEL LINES NEVER INTERSECT IF 2 LINES ARE PARALLEL, THEN THEY NEVER INTERSECT
- 5. Questions from Glenco Mathematics www.glenco.com Identify the hypothesis of the following if-then statement. If Ralph does his math homework, then he will get a good grade on the quiz. A.He will not get a good grade on the quiz. B.Ralph does his math homework. C.He will get a good grade on the quiz. D.Ralph may do his math homework. Determine the converse of the following if-then statement. If the flowers are yellow, then they are daffodils. A.If the flowers are daffodils, then they are yellow. B.The flowers are not yellow. C.If the flowers are not yellow, then they are not daffodils. D.The flowers are not daffodils. 1 2
- 6. Write the contrapositive of the statement a right angle measures 90 degrees. A.If an angle measures 90 degrees, then it is a right angle. B.If an angle is not a right angle, then its measure is not 90 degrees. C.If an angle does not measure 90 degrees, then it is not a right angle. D.If an angle is a right angle, then it measures 90 degrees. Which statement is the inverse of the statement angles with the same measure are congruent? A.If two angles are congruent, then they have the same measure. B.If two angles have the same measure, then they are congruent. C.If two angles are not congruent, then they do not have the same measure. D.If two angles do not have the same measure, then they are not congruent. If-then statements are also called ______________. A.reasons B.conditionals C.hypothesis D.conclusions 3 4 5