5.2 Strong Induction
- 1. Strong Induction and Well- Ordering Section 5.2 1
- 2. Strong Induction âĒ Strong Induction: To prove that P(n) is true for all positive integers n, where P(n) is a propositional function, complete two steps: â Basis Step: Verify that the proposition P(1) is true. â Inductive Step: Show the conditional statement [P(1) ⧠P(2) â§âââ ⧠P(k)] â P(k + 1) holds for all positive integers k. 2 Strong Induction is sometimes called the second principle of mathematical induction or complete induction.
- 3. Strong Induction and the Infinite Ladder 3 Strong induction tells us that we can reach all rungs if: 1. We can reach the first rung of the ladder. 2. For every integer k, if we can reach the first k rungs, then we can reach the (k + 1)st rung. To conclude that we can reach every rung by strong induction: âĒ BASIS STEP: P(1) holds âĒ INDUCTIVE STEP: Assume P(1) ⧠P(2) â§âââ ⧠P(k) holds for an arbitrary integer k, and show that P(k + 1) must also hold. We will have then shown by strong induction that for every positive integer n, P(n) holds, i.e., we can reach the nth rung of the ladder.
- 4. Strong Induction and the Infinite Ladder 4 Strong induction tells us that we can reach all rungs if: 1. We can reach the first rung of the ladder. 2. For every integer k, if we can reach the first k rungs, then we can reach the (k + 1)st rung. To conclude that we can reach every rung by strong induction: âĒ BASIS STEP: P(1) holds âĒ INDUCTIVE STEP: Assume P(1) ⧠P(2) â§âââ ⧠P(k) holds for an arbitrary integer k, and show that P(k + 1) must also hold. We will have then shown by strong induction that for every positive integer n, P(n) holds, i.e., we can reach the nth rung of the ladder.
- 5. Which Form of Induction Should Be Used? âĒ We can always use strong induction instead of mathematical induction. But there is no reason to use it if it is simpler to use mathematical induction. âĒ In fact, the principles of mathematical induction, strong induction, and the well-ordering property are all equivalent. âĒ Sometimes it is clear how to proceed using one of the three methods, but not the other two. 5
- 6. Proof using Strong Induction Example: Prove that every amount of postage of 12 cents or more can be formed using just 4-cent and 5-cent stamps.
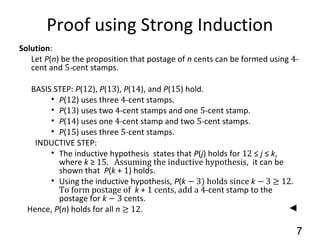
- 7. Proof using Strong Induction Solution: Let P(n) be the proposition that postage of n cents can be formed using 4- cent and 5-cent stamps. BASIS STEP: P(12), P(13), P(14), and P(15) hold. âĒ P(12) uses three 4-cent stamps. âĒ P(13) uses two 4-cent stamps and one 5-cent stamp. âĒ P(14) uses one 4-cent stamp and two 5-cent stamps. âĒ P(15) uses three 5-cent stamps. INDUCTIVE STEP: âĒ The inductive hypothesis states that P(j) holds for 12 âĪ j âĪ k, where k âĨ 15. Assuming the inductive hypothesis, it can be shown that P(k + 1) holds. âĒ Using the inductive hypothesis, P(k â 3) holds since k â 3 âĨ 12. To form postage of k + 1 cents, add a 4-cent stamp to the postage for k â 3 cents. Hence, P(n) holds for all n âĨ 12. 7

![Strong Induction
âĒ Strong Induction: To prove that P(n) is true for
all positive integers n, where P(n) is a
propositional function, complete two steps:
â Basis Step: Verify that the proposition P(1) is true.
â Inductive Step: Show the conditional statement
[P(1) ⧠P(2) â§âââ ⧠P(k)] â P(k + 1) holds for
all positive integers k.
2
Strong Induction is sometimes called the second principle of
mathematical induction or complete induction.](https://image.slidesharecdn.com/5-140404115734-phpapp01/85/5-2-Strong-Induction-2-320.jpg)