6.3 integration by substitution
- 2. Recognizing the ŌĆ£Outside-InsideŌĆØ Pattern Ōł½(x 2 +1) 2x dx 2 From doing derivatives we need to recognize the integrand above is a composite function from the ŌĆ£derivative of the outside times the derivative of the insideŌĆØ (chain rule). 3 1 2 = ( x + 1) + C 3 ŌĆ£+ CŌĆØ since this is an indefinite integral
- 3. Think of this function as 2 functions: f(x) and g(x) f ( x) = x g ( x) = x + 1 2 2 As a composite function then: ( ) f ( g ( x) ) = x + 1 outside 2 2 inside Now look at the original integral: Ōł½(x 2 +1) 2x dx f(g(x)) 2 gŌĆÖ(x)
- 4. Read this as ŌĆ£the antiderivative of the outside function with the inside function plugged inŌĆ”plus CŌĆØ
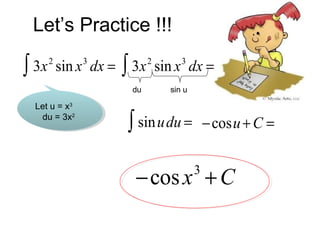
- 6. LetŌĆÖs Practice !!! 3x 2 sin x 3 dx = Ōł½ 3x 2 sin x 3 dx = Ōł½ du Let u = x3 du = 3x2 sin u Ōł½ sin u du = ŌłÆ cosu + C = ŌłÆcos x + C 3
- 7. More Practice !!! Ōł½x 1/4 3 x + 2 dx = 4 Let u = x4 + 2 (x 4 x 4 + 2 x 3 dx = 1 4 u du Ōł½ 1 4 du = 4 x3 4 Ōł½ u du = 3 2 3 2 1u 1 2u Ōł½ u du = 4 3 + C = 4 3 + C = 2 + 2) 6 1 2 3 2 +C = (x 4 + 2) 6 3 +C
- 8. Here are some problems for you to work on!!! dx 1. Ōł½ = 2 1+ 3x ’Ż½1 2 ’ŻČ 2. Ōł½ ’Ż¼ sin ŽĆ x ├Ędx = ’ŻŁx ’ŻĖ 3. Ōł½ sin 2 x cos x dx = e x 4. Ōł½ dx = x 43 5 5. Ōł½ t 3ŌłÆ 5t dt =
- 9. Less Apparent Substitution 1. Ōł½ x 2 x ŌłÆ1 dx = Ōł½ x 2 x ŌłÆ1 dx = (u + 1) Let u = x ŌĆō 1 du = dx x=u+1 x2 = (u + !)2 7 2 Ōł½ ( u +1) Ōł½( 5 2 u 2 du u du = Ōł½ ( u +1) 2 1 2 u du = 3 1’ŻČ ’Ż½ 5 2 ’Ż¼ u 2 + 2u 2 + u 2 ├Ędu = u + 2u +1 u du = Ōł½ ’ŻŁ ’ŻĖ ) 3 2 1 2 7 2 5 2 3 2 2 ( x ŌłÆ1) 4 ( x ŌłÆ1) 2 ( x ŌłÆ1) 2u 2 ├Ś2u 2u + + +C = + + +C 7 5 3 7 5 3
- 10. Less Apparent Substitution 1. Ōł½ x 2 x ŌłÆ1 dx = Ōł½ x 2 x ŌłÆ1 dx = (u + 1) Let u = x ŌĆō 1 du = dx x=u+1 x2 = (u + !)2 7 2 Ōł½ ( u +1) Ōł½( 5 2 u 2 du u du = Ōł½ ( u +1) 2 1 2 u du = 3 1’ŻČ ’Ż½ 5 2 ’Ż¼ u 2 + 2u 2 + u 2 ├Ędu = u + 2u +1 u du = Ōł½ ’ŻŁ ’ŻĖ ) 3 2 1 2 7 2 5 2 3 2 2 ( x ŌłÆ1) 4 ( x ŌłÆ1) 2 ( x ŌłÆ1) 2u 2 ├Ś2u 2u + + +C = + + +C 7 5 3 7 5 3