More Related Content
Similar to 6.5 Logarithmic Properties (20)
More from smiller5 (20)
Recently uploaded (20)
6.5 Logarithmic Properties
- 1. 6.5 Logarithmic Properties Chapter 6 Exponential and Logarithmic Functions
- 2. Concepts & Objectives Objectives for this section are Use the product rule for logarithms. Use the quotient rule for logarithms. Use the power rule for logarithms. Expand logarithmic expressions. Condense logarithmic expressions. Use the change-of-base formula for logarithms.
- 3. Properties of Logarithms Because logarithms are exponents, they have three properties that come directly from the corresponding properties of exponentiation: Exponents Logarithms a b a b x x x + = a a b b x x x = ( ) b a ab x x = ( ) log log log a b a b = + log log log a a b b = log log b a b a =
- 4. Examples 1. Write log224 log28 as a single logarithm of a single argument. 2. Use the Log of a Power Property to solve 0.82x = 0.007.
- 5. Examples 1. Write log224 log28 as a single logarithm of a single argument. 2. Use the Log of a Power Property to solve 0.82x = 0.007. 2 2 2 24 log 24 log 8 log 8 = 2 log 3 = 2 log0.8 log0.007 x = 2 log0.8 log0.007 x = log0.007 11.12 2log0.8 x = = 2 0.8 0.007 x
- 6. Change-of-Base Formula Although the calculators we use in class, as well as Desmos, will calculate a logarithm using any base, it can sometimes be useful to change a logarithm from one base to another. For any positive real numbers M, b, and n, where n 1 and b 1, = log log log n b n M b
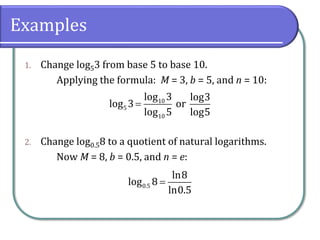
- 7. Examples 1. Change log53 from base 5 to base 10. 2. Change log0.58 to a quotient of natural logarithms.
- 8. Examples 1. Change log53 from base 5 to base 10. Applying the formula: M = 3, b = 5, and n = 10: 2. Change log0.58 to a quotient of natural logarithms. Now M = 8, b = 0.5, and n = e: 10 5 10 log 3 log3 log 3 or log 5 log5 = 0.5 ln8 log 8 ln0.5 =
- 9. Classwork College Algebra 2e 6.5: 4-14 (even); 6.3: 26-40 (even); 6.1: 62-68 (even) 6.5 Classwork Check Quiz 6.3