8-5 Trig Angles of Elev and Depress.ppt
Download as PPT, PDF0 likes56 views
This document defines and provides examples of angles of elevation and depression. It discusses how these angles are formed when viewing an object from a higher or lower position and must have one horizontal side. Several word problems are worked out using trigonometric functions like sine, tangent, and inverse tangent to calculate unknown distances and heights based on a given angle of elevation or depression and one known side length.
1 of 20
Download to read offline

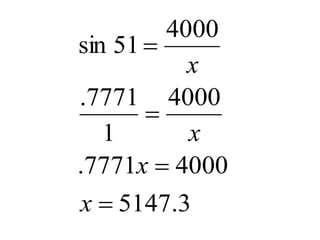
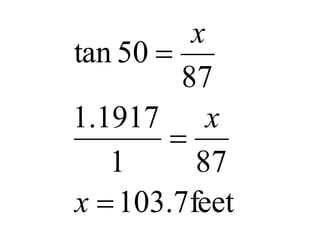
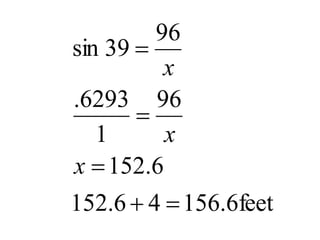
Recommended
Real life application of angle of elevation and depression.pptx



Real life application of angle of elevation and depression.pptxLiciaMc
╠²
A lengthy presentation which is a compilation of several areas of trigonometry. Application to real life, angles of elevation and depression.PLANE-TRIGONOMETRY.pptx



PLANE-TRIGONOMETRY.pptxDanEmilBernardino
╠²
This document provides information on trigonometric functions of right triangles. It defines the sine, cosine, and tangent functions as ratios of sides of a right triangle. It also introduces cosecant, secant, and cotangent as reciprocals of the primary trig functions. Several examples are given to calculate unknown side lengths or angles using trig functions. The document then covers trigonometric identities, angle sum and difference formulas, double and half angle formulas, and techniques for reducing trig powers and converting between sum and product formulas. It concludes with information on measuring angles in different units and introduces the study of oblique triangles, including the Law of Sines and Law of Cosines.ANGLES-OF-ELEVATION-AND.pptx



ANGLES-OF-ELEVATION-AND.pptxMaria685954
╠²
The document discusses angles of elevation and depression. It defines an angle of elevation as the angle from the horizontal to the line of sight of an observer looking at an object above them. An angle of depression is defined as the angle from the horizontal to the line of sight of an observer looking at an object below them. The document provides examples of solving problems involving angles of elevation and depression using trigonometric ratios.Trigonometry, ratios and otheraspec.pptx



Trigonometry, ratios and otheraspec.pptxAbdulwahabSuleimanGi
╠²
This is a compilation of ratios special angles and others found to be useful to the mathematics world4th observation TRIANGLE MATH.pptx



4th observation TRIANGLE MATH.pptxJennyNuevoIpong
╠²
The document discusses finding the area of triangles using the formula Area = 1/2 * base * height. It provides examples of calculating the area of various triangles by measuring their bases and heights. The last example asks what is the area of the Bermuda Triangle, which has a base of 30km and height of 20km.Unit v review



Unit v reviewDavid Wagner
╠²
This document contains a jeopardy-style game with categories and clues. It discusses trigonometric functions including angle measurement, unit circle, graphs, transformations, and solving equations. Key concepts covered are degrees, radians, trig function periods, amplitudes, phase shifts, and relating sine and cosine graphs.trigonometry



trigonometryWeerachat Martluplao
╠²
This document provides information about triangles, squares, and trigonometry. It begins by defining different types of triangles, including isosceles, equilateral, and scalene triangles. It then discusses squares and using the area formula. The document introduces Pythagoras' theorem and provides examples of using it to find missing side lengths in right triangles. It also covers sine, cosine, and tangent ratios. Exercises provide practice applying concepts like finding missing lengths, areas, and using trigonometric functions in right triangles.Prelims-MST.pptx



Prelims-MST.pptxValenTuraray
╠²
* A rope is stretched from the top of a 12m tree to the ground
* The rope is 20m long
* Let's call the distance from the tree to the end of the rope x
* Then we have a right triangle with:
* Hypotenuse (c) = Rope length = 20m
* One leg (a) = Height of tree = 12m
* Other leg (x) = Distance from tree to end of rope
* Using the Pythagorean theorem: a^2 + b^2 = c^2
* x^2 + 12^2 = 20^2
* x^2 + 144 = 400
* x^2 = 256
* x =Ch 3 Two Dimensional Kinematics



Ch 3 Two Dimensional KinematicsScott Thomas
╠²
This document provides a summary of key concepts in two-dimensional kinematics and projectile motion. It begins by defining displacement, velocity, and acceleration in two dimensions. It then discusses solving kinematics problems by resolving vectors into horizontal and vertical components. The document also covers projectile motion, where the horizontal velocity is constant and vertical acceleration is due to gravity. It ends by discussing relative velocity problems involving adding velocities of objects moving relative to each other or to a fixed point.right triangles Application



right triangles Applicationrey castro
╠²
This document contains multiple word problems involving right triangles and trigonometric functions like sine, cosine, and tangent. It provides the problems, drawings of the relevant right triangles, and the step-by-step workings to arrive at the solutions. The problems cover a range of real-world scenarios involving angles of elevation/depression, shadows, ladders, towers, and more.Angle of Elevation and Depression Grade 9



Angle of Elevation and Depression Grade 9CasterBadival1
╠²
Angle of Elevation and Depresion With ExercisesGeneral Physics 1 - Two Dimensional Motion



General Physics 1 - Two Dimensional MotionLyndrianShalomBaclay
╠²
General Physics 1 - Two Dimensional Motion1-5 Angle relationships.ppt



1-5 Angle relationships.pptsmithj91
╠²
1. Adjacent angles have a common vertex and side but no interior points in common. Complementary angles have a sum of 90 degrees. Supplementary angles have a sum of 180 degrees.
2. Vertical angles are formed by two intersecting lines and are congruent. Linear pairs are adjacent angles whose noncommon sides form opposite rays and are supplementary.
3. The document provides examples of finding missing angles based on properties such as being complementary, supplementary, vertical or corresponding angles.1-3 Distance and Midpoint.ppt



1-3 Distance and Midpoint.pptsmithj91
╠²
The document discusses distance and midpoint formulas for finding the distance between two points and the midpoint of a line segment. It provides examples of using the distance formula to find the distance between points and the midpoint formula to find the midpoint. It also explains that if you know one endpoint and the midpoint, you can use the midpoint formula to find the other endpoint.5-4 Triangle Inequalites.pptx



5-4 Triangle Inequalites.pptxsmithj91
╠²
This document discusses the triangle inequality theorem and its applications. It provides 5 examples of potential side lengths of triangles and asks the reader to determine which sets of lengths satisfy the triangle inequality theorem. It also provides 4 examples and asks the reader to determine the range of possible lengths for the third side in each case.Radicals.ppt



Radicals.pptsmithj91
╠²
This document provides information on perfect squares, radicals, and simplifying expressions with radicals. It defines perfect squares as numbers obtained by multiplying a number by itself. It explains radicals as the square root symbol and what it represents. Examples are provided of evaluating radicals and identifying the positive and negative roots of a number. The document outlines rules for simplifying expressions with radicals, such as never leaving a perfect square or fraction under a radical sign, and rationalizing denominators that have radicals.Solving Equations.ppt



Solving Equations.pptsmithj91
╠²
The document provides steps for solving algebraic equations:
1) Simplify both sides of the equal sign. This may require multiple steps.
2) Get all variables on one side of the equal sign by canceling out variables on both sides.
3) Cancel out any addition or subtraction by performing the opposite operation to both sides.
4) Cancel out any multiplication or division by dividing or multiplying both sides.13-3 Probability.ppt



13-3 Probability.pptsmithj91
╠²
This document discusses probability and provides examples of calculating probability using dice rolls, game boards with colored sections, and spinners. The key points are:
- Probability is the number of successful outcomes divided by the total number of possible outcomes.
- For a single die, the probability of rolling a 3 is 1/6 since there is 1 way to roll a 3 out of 6 total possible outcomes.
- For two dice, the probability of rolling a 5 is 4/36 since there are 4 ways to roll a 5 (1-4, 2-3, 3-2, 4-1) out of 36 total possible outcomes combining the two dice.
- For game boards, probability is calculated by taking the12-1 Solids.ppt



12-1 Solids.pptsmithj91
╠²
This document provides formulas and examples for calculating the perimeter, area, surface area, and volume of various shapes including rectangles, circles, prisms, cylinders, pyramids, cones, and spheres. It defines perimeter as the distance around a shape and area as the space inside a shape. Formulas provided include calculating the perimeter and area of rectangles and circles, as well as the lateral area, surface area, and volume of prisms, cylinders, pyramids, cones, and spheres. Worked examples demonstrate applying the formulas to find perimeter, area, surface area, and volume for specific shapes.google earth circles.pptx



google earth circles.pptxsmithj91
╠²
The document provides calculations to find the arc length and sector area of a circle with a central angle of 117 degrees and a radius of 813.42 meters. It first calculates the central angle as 117 degrees, then uses this to find the arc length as 528.7ŽĆ meters and the sector area as 215036.9ŽĆ square meters.11-3 Arc Length, Sectors & Sections.ppt



11-3 Arc Length, Sectors & Sections.pptsmithj91
╠²
This document contains examples of calculating arc lengths, sector areas, and section areas of circles. It demonstrates how to find the arc length or sector area as a fraction of the total circumference or total area of the circle based on the central angle. It also shows how to calculate the area of a section by subtracting the area of a triangle from the area of the corresponding sector. Key formulas used include the circumference formula C=2ŽĆr, the area formula for a circle A=ŽĆr^2, and the area formula for a triangle A=1/2bh.11-1, 11-2 Perimeter & Area of Polygons.ppt



11-1, 11-2 Perimeter & Area of Polygons.pptsmithj91
╠²
This document provides information and formulas for calculating the perimeter and area of various polygons. It includes formulas for finding the perimeter and area of rectangles, parallelograms, triangles, trapezoids, rhombi, kites, and regular polygons. The key points are that perimeter is found by adding all the sides of any polygon, and area formulas vary depending on the specific shape but generally involve multiplying base and height or base and apothem.10-8 Equations of circles.ppt



10-8 Equations of circles.pptsmithj91
╠²
The document discusses finding the equation of a circle given its center and radius, or finding the center and radius given the equation. It provides examples of writing the standard equation of a circle (x - h)2 + (y - k)2 = r2 given the center (h, k) and radius r. It also works through examples of finding the center and radius when given the equation of a circle. Finally, it explains how to find the equation of a circle if given its center and that it passes through a specific point.10-7 Circles - Segments.ppt



10-7 Circles - Segments.pptsmithj91
╠²
This document contains instructions and examples for measuring circles, segments, chords, and tangents. It includes the following examples:
1) Finding the length of a chord using the formula that the product of the parts of one chord equals the product of the parts of the other.
2) Solving a pair of equations involving a segment, radius, and variable part of a chord.
3) Explaining that the diameter of a circle bisects any chord perpendicular to it.
The document provides step-by-step workings for each example and problem.10-4, 10-6 Circles and Angles.ppt



10-4, 10-6 Circles and Angles.pptsmithj91
╠²
This document discusses properties of angles related to circles. It defines central angles, inscribed angles, and their relationships to intercepted arcs. The measure of a central angle is equal to the measure of its intercepted arc. The measure of an inscribed angle is equal to half the measure of its intercepted arc. Examples are given of finding the measures of unknown angles using these properties.10-3_Arcs_and_Chords.ppt



10-3_Arcs_and_Chords.pptsmithj91
╠²
This document discusses properties of chords and arcs in circles. It states that if two chords are congruent, their corresponding arcs are also congruent. It also notes that equal chords are equidistant from the center, and chords get longer as they get closer to the center, with diameters being the longest chords that pass through the center. The document provides an example of how to find the center of a circle by drawing two chords and finding the intersection of the perpendicular bisectors.10-2_Angles_and_Arcs.ppt



10-2_Angles_and_Arcs.pptsmithj91
╠²
This document discusses angles and arcs in circles. An angle formed by two radii is called a central angle. The measure of an arc is equal to the measure of the central angle. There are three types of arcs: minor arcs which are named by their endpoints, major arcs which are named by endpoints and go through another point, and semicircles which are arcs that are equal to 180 degrees. The document also provides an example of using percentages to find the measures of central angles for different sports in a pie chart.10-1 Circles.ppt



10-1 Circles.pptsmithj91
╠²
The document defines and describes the key parts of a circle, including the center, radius, diameter, chord, and circumference. It provides formulas for calculating the radius, diameter, and circumference based on these parts. Examples are given for using the formulas to calculate lengths and circumferences of various circles.More Related Content
Similar to 8-5 Trig Angles of Elev and Depress.ppt (6)
Ch 3 Two Dimensional Kinematics



Ch 3 Two Dimensional KinematicsScott Thomas
╠²
This document provides a summary of key concepts in two-dimensional kinematics and projectile motion. It begins by defining displacement, velocity, and acceleration in two dimensions. It then discusses solving kinematics problems by resolving vectors into horizontal and vertical components. The document also covers projectile motion, where the horizontal velocity is constant and vertical acceleration is due to gravity. It ends by discussing relative velocity problems involving adding velocities of objects moving relative to each other or to a fixed point.right triangles Application



right triangles Applicationrey castro
╠²
This document contains multiple word problems involving right triangles and trigonometric functions like sine, cosine, and tangent. It provides the problems, drawings of the relevant right triangles, and the step-by-step workings to arrive at the solutions. The problems cover a range of real-world scenarios involving angles of elevation/depression, shadows, ladders, towers, and more.Angle of Elevation and Depression Grade 9



Angle of Elevation and Depression Grade 9CasterBadival1
╠²
Angle of Elevation and Depresion With ExercisesGeneral Physics 1 - Two Dimensional Motion



General Physics 1 - Two Dimensional MotionLyndrianShalomBaclay
╠²
General Physics 1 - Two Dimensional MotionMore from smithj91 (20)
1-5 Angle relationships.ppt



1-5 Angle relationships.pptsmithj91
╠²
1. Adjacent angles have a common vertex and side but no interior points in common. Complementary angles have a sum of 90 degrees. Supplementary angles have a sum of 180 degrees.
2. Vertical angles are formed by two intersecting lines and are congruent. Linear pairs are adjacent angles whose noncommon sides form opposite rays and are supplementary.
3. The document provides examples of finding missing angles based on properties such as being complementary, supplementary, vertical or corresponding angles.1-3 Distance and Midpoint.ppt



1-3 Distance and Midpoint.pptsmithj91
╠²
The document discusses distance and midpoint formulas for finding the distance between two points and the midpoint of a line segment. It provides examples of using the distance formula to find the distance between points and the midpoint formula to find the midpoint. It also explains that if you know one endpoint and the midpoint, you can use the midpoint formula to find the other endpoint.5-4 Triangle Inequalites.pptx



5-4 Triangle Inequalites.pptxsmithj91
╠²
This document discusses the triangle inequality theorem and its applications. It provides 5 examples of potential side lengths of triangles and asks the reader to determine which sets of lengths satisfy the triangle inequality theorem. It also provides 4 examples and asks the reader to determine the range of possible lengths for the third side in each case.Radicals.ppt



Radicals.pptsmithj91
╠²
This document provides information on perfect squares, radicals, and simplifying expressions with radicals. It defines perfect squares as numbers obtained by multiplying a number by itself. It explains radicals as the square root symbol and what it represents. Examples are provided of evaluating radicals and identifying the positive and negative roots of a number. The document outlines rules for simplifying expressions with radicals, such as never leaving a perfect square or fraction under a radical sign, and rationalizing denominators that have radicals.Solving Equations.ppt



Solving Equations.pptsmithj91
╠²
The document provides steps for solving algebraic equations:
1) Simplify both sides of the equal sign. This may require multiple steps.
2) Get all variables on one side of the equal sign by canceling out variables on both sides.
3) Cancel out any addition or subtraction by performing the opposite operation to both sides.
4) Cancel out any multiplication or division by dividing or multiplying both sides.13-3 Probability.ppt



13-3 Probability.pptsmithj91
╠²
This document discusses probability and provides examples of calculating probability using dice rolls, game boards with colored sections, and spinners. The key points are:
- Probability is the number of successful outcomes divided by the total number of possible outcomes.
- For a single die, the probability of rolling a 3 is 1/6 since there is 1 way to roll a 3 out of 6 total possible outcomes.
- For two dice, the probability of rolling a 5 is 4/36 since there are 4 ways to roll a 5 (1-4, 2-3, 3-2, 4-1) out of 36 total possible outcomes combining the two dice.
- For game boards, probability is calculated by taking the12-1 Solids.ppt



12-1 Solids.pptsmithj91
╠²
This document provides formulas and examples for calculating the perimeter, area, surface area, and volume of various shapes including rectangles, circles, prisms, cylinders, pyramids, cones, and spheres. It defines perimeter as the distance around a shape and area as the space inside a shape. Formulas provided include calculating the perimeter and area of rectangles and circles, as well as the lateral area, surface area, and volume of prisms, cylinders, pyramids, cones, and spheres. Worked examples demonstrate applying the formulas to find perimeter, area, surface area, and volume for specific shapes.google earth circles.pptx



google earth circles.pptxsmithj91
╠²
The document provides calculations to find the arc length and sector area of a circle with a central angle of 117 degrees and a radius of 813.42 meters. It first calculates the central angle as 117 degrees, then uses this to find the arc length as 528.7ŽĆ meters and the sector area as 215036.9ŽĆ square meters.11-3 Arc Length, Sectors & Sections.ppt



11-3 Arc Length, Sectors & Sections.pptsmithj91
╠²
This document contains examples of calculating arc lengths, sector areas, and section areas of circles. It demonstrates how to find the arc length or sector area as a fraction of the total circumference or total area of the circle based on the central angle. It also shows how to calculate the area of a section by subtracting the area of a triangle from the area of the corresponding sector. Key formulas used include the circumference formula C=2ŽĆr, the area formula for a circle A=ŽĆr^2, and the area formula for a triangle A=1/2bh.11-1, 11-2 Perimeter & Area of Polygons.ppt



11-1, 11-2 Perimeter & Area of Polygons.pptsmithj91
╠²
This document provides information and formulas for calculating the perimeter and area of various polygons. It includes formulas for finding the perimeter and area of rectangles, parallelograms, triangles, trapezoids, rhombi, kites, and regular polygons. The key points are that perimeter is found by adding all the sides of any polygon, and area formulas vary depending on the specific shape but generally involve multiplying base and height or base and apothem.10-8 Equations of circles.ppt



10-8 Equations of circles.pptsmithj91
╠²
The document discusses finding the equation of a circle given its center and radius, or finding the center and radius given the equation. It provides examples of writing the standard equation of a circle (x - h)2 + (y - k)2 = r2 given the center (h, k) and radius r. It also works through examples of finding the center and radius when given the equation of a circle. Finally, it explains how to find the equation of a circle if given its center and that it passes through a specific point.10-7 Circles - Segments.ppt



10-7 Circles - Segments.pptsmithj91
╠²
This document contains instructions and examples for measuring circles, segments, chords, and tangents. It includes the following examples:
1) Finding the length of a chord using the formula that the product of the parts of one chord equals the product of the parts of the other.
2) Solving a pair of equations involving a segment, radius, and variable part of a chord.
3) Explaining that the diameter of a circle bisects any chord perpendicular to it.
The document provides step-by-step workings for each example and problem.10-4, 10-6 Circles and Angles.ppt



10-4, 10-6 Circles and Angles.pptsmithj91
╠²
This document discusses properties of angles related to circles. It defines central angles, inscribed angles, and their relationships to intercepted arcs. The measure of a central angle is equal to the measure of its intercepted arc. The measure of an inscribed angle is equal to half the measure of its intercepted arc. Examples are given of finding the measures of unknown angles using these properties.10-3_Arcs_and_Chords.ppt



10-3_Arcs_and_Chords.pptsmithj91
╠²
This document discusses properties of chords and arcs in circles. It states that if two chords are congruent, their corresponding arcs are also congruent. It also notes that equal chords are equidistant from the center, and chords get longer as they get closer to the center, with diameters being the longest chords that pass through the center. The document provides an example of how to find the center of a circle by drawing two chords and finding the intersection of the perpendicular bisectors.10-2_Angles_and_Arcs.ppt



10-2_Angles_and_Arcs.pptsmithj91
╠²
This document discusses angles and arcs in circles. An angle formed by two radii is called a central angle. The measure of an arc is equal to the measure of the central angle. There are three types of arcs: minor arcs which are named by their endpoints, major arcs which are named by endpoints and go through another point, and semicircles which are arcs that are equal to 180 degrees. The document also provides an example of using percentages to find the measures of central angles for different sports in a pie chart.10-1 Circles.ppt



10-1 Circles.pptsmithj91
╠²
The document defines and describes the key parts of a circle, including the center, radius, diameter, chord, and circumference. It provides formulas for calculating the radius, diameter, and circumference based on these parts. Examples are given for using the formulas to calculate lengths and circumferences of various circles.8-7 Law of Cosines.ppt



8-7 Law of Cosines.pptsmithj91
╠²
The document discusses the Law of Cosines, which can be used to find the angles of a triangle when only the three sides are known. It provides the Law of Cosine formulas for finding the angles opposite sides a, b, and c. Examples are given for finding a missing side with two sides and the included angle (SAS) and for finding a missing angle with all three sides known (SSS). A word problem example finds the distance a golf ball is from the cup using the Law of Cosines.8-6 Law of Sines.ppt



8-6 Law of Sines.pptsmithj91
╠²
The document discusses the Law of Sines and how to use it to solve for missing sides and angles in non-right triangles. It provides examples of using the Law of Sines to find a missing side length given two angles and one side, and to find a missing angle given two sides and the angle between them. Finally, it applies the Law of Sines to solve a real-world problem of finding the height of a telephone pole given the angle of elevation to its top, its shadow length, and the angle the pole was knocked from perpendicular.8-4 Sine, Cosine.ppt



8-4 Sine, Cosine.pptsmithj91
╠²
The document defines the trigonometric functions of sine, cosine, and tangent. It provides examples of calculating unknown side lengths and angles using trigonometric ratios and the definitions of sine, cosine, and tangent. Sample calculations include finding an unknown side length given two other sides and an angle measure, and finding an unknown angle measure given two side lengths.8-4 Tangent.ppt



8-4 Tangent.pptsmithj91
╠²
This document discusses using the tangent ratio to solve for unknown side lengths of right triangles. It provides examples of finding the opposite and adjacent legs for various angles in different triangles. The key steps are to identify the tangent ratio as opposite over adjacent, set up the ratio as an equation, and solve for the unknown side length. Readers are reminded to set calculators to degrees, show all work, express tangent ratios to 4 decimal places, and round answers to 1 decimal place.Recently uploaded (20)
Entity Framework Interview Questions PDF By ScholarHat



Entity Framework Interview Questions PDF By ScholarHatScholarhat
╠²
Entity Framework Interview Questions PDF By ScholarHatOne Click RFQ Cancellation in Odoo 18 - Odoo ║▌║▌▀Żs



One Click RFQ Cancellation in Odoo 18 - Odoo ║▌║▌▀ŻsCeline George
╠²
In this slide, weŌĆÖll discuss the one click RFQ Cancellation in odoo 18. One-Click RFQ Cancellation in Odoo 18 is a feature that allows users to quickly and easily cancel Request for Quotations (RFQs) with a single click.Unit 1 Computer Hardware for Educational Computing.pptx



Unit 1 Computer Hardware for Educational Computing.pptxRomaSmart1
╠²
Computers have revolutionized various sectors, including education, by enhancing learning experiences and making information more accessible. This presentation, "Computer Hardware for Educational Computing," introduces the fundamental aspects of computers, including their definition, characteristics, classification, and significance in the educational domain. Understanding these concepts helps educators and students leverage technology for more effective learning.Inventory Reporting in Odoo 17 - Odoo 17 Inventory App



Inventory Reporting in Odoo 17 - Odoo 17 Inventory AppCeline George
╠²
This slide will helps us to efficiently create detailed reports of different records defined in its modules, both analytical and quantitative, with Odoo 17 ERP.Intellectual Honesty & Research Integrity.pptx



Intellectual Honesty & Research Integrity.pptxNidhiSharma495177
╠²
Research Publication & Ethics contains a chapter on Intellectual Honesty and Research Integrity.
Different case studies of intellectual dishonesty and integrity were discussed.ASP.NET Interview Questions PDF By ScholarHat



ASP.NET Interview Questions PDF By ScholarHatScholarhat
╠²
ASP.NET Interview Questions PDF By ScholarHatMeeting the needs of modern students?, Selina McCoy



Meeting the needs of modern students?, Selina McCoyEconomic and Social Research Institute
╠²
NAPD Annual Symposium
ŌĆ£Equity in our Schools: Does the system deliver for all young people?ŌĆØOdoo 18 Accounting Access Rights - Odoo 18 ║▌║▌▀Żs



Odoo 18 Accounting Access Rights - Odoo 18 ║▌║▌▀ŻsCeline George
╠²
In this slide, weŌĆÖll discuss on accounting access rights in odoo 18. To ensure data security and maintain confidentiality, Odoo provides a robust access rights system that allows administrators to control who can access and modify accounting data. Azure Data Engineer Interview Questions By ScholarHat



Azure Data Engineer Interview Questions By ScholarHatScholarhat
╠²
Azure Data Engineer Interview Questions By ScholarHatHow to create security group category in Odoo 17



How to create security group category in Odoo 17Celine George
╠²
This slide will represent the creation of security group category in odoo 17. Security groups are essential for managing user access and permissions across different modules. Creating a security group category helps to organize related user groups and streamline permission settings within a specific module or functionality.Functional Muscle Testing of Facial Muscles.pdf



Functional Muscle Testing of Facial Muscles.pdfSamarHosni3
╠²
Functional Muscle Testing of Facial Muscles.pdfAI and Academic Writing, Short Term Course in Academic Writing and Publicatio...



AI and Academic Writing, Short Term Course in Academic Writing and Publicatio...Prof. (Dr.) Vinod Kumar Kanvaria
╠²
AI and Academic Writing, Short Term Course in Academic Writing and Publication, UGC-MMTTC, MANUU, 25/02/2025, Prof. (Dr.) Vinod Kumar Kanvaria, University of Delhi, vinodpr111@gmail.comHow to Configure Proforma Invoice in Odoo 18 Sales



How to Configure Proforma Invoice in Odoo 18 SalesCeline George
╠²
In this slide, weŌĆÖll discuss on how to configure proforma invoice in Odoo 18 Sales module. A proforma invoice is a preliminary invoice that serves as a commercial document issued by a seller to a buyer.Hannah Borhan and Pietro Gagliardi OECD present 'From classroom to community ...



Hannah Borhan and Pietro Gagliardi OECD present 'From classroom to community ...EduSkills OECD
╠²
Hannah Borhan, Research Assistant, OECD Education and Skills Directorate and Pietro Gagliardi, Policy Analyst, OECD Public Governance Directorate present at the OECD webinar 'From classroom to community engagement: Promoting active citizenship among young people" on 25 February 2025. You can find the recording of the webinar on the website https://oecdedutoday.com/webinars/
AI and Academic Writing, Short Term Course in Academic Writing and Publicatio...



AI and Academic Writing, Short Term Course in Academic Writing and Publicatio...Prof. (Dr.) Vinod Kumar Kanvaria
╠²
8-5 Trig Angles of Elev and Depress.ppt
- 1. ANGLES OF ELEVATION AND DEPRESSION Jim Smith JCHS Section 8-5 spi.3.1.B
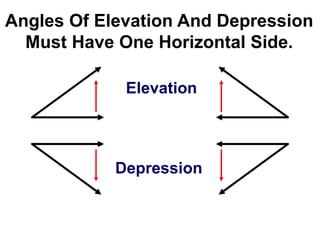
- 2. Angles Of Elevation And Depression Must Have One Horizontal Side. Elevation Depression
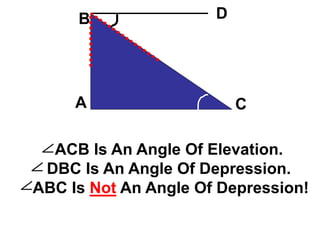
- 3. A B C ACB Is An Angle Of Elevation. DBC Is An Angle Of Depression. ABC Is Not An Angle Of Depression! D
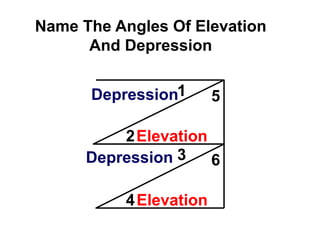
- 4. Name The Angles Of Elevation And Depression 1 2 3 4 5 6 Depression Elevation Elevation Depression
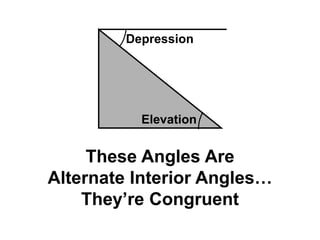
- 5. Depression Elevation These Angles Are Alternate Interior AnglesŌĆ” TheyŌĆÖre Congruent
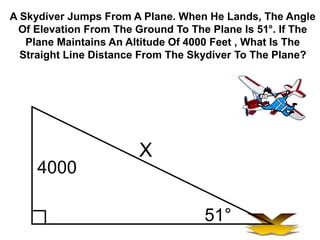
- 6. A Skydiver Jumps From A Plane. When He Lands, The Angle Of Elevation From The Ground To The Plane Is 51┬░. If The Plane Maintains An Altitude Of 4000 Feet , What Is The Straight Line Distance From The Skydiver To The Plane? 4000 51┬░ X
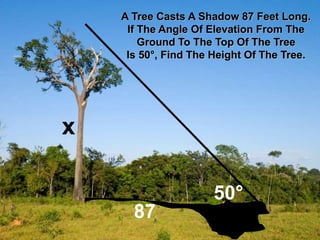
- 8. A Tree Casts A Shadow 87 Feet Long. If The Angle Of Elevation From The Ground To The Top Of The Tree Is 50┬░, Find The Height Of The Tree. 50┬░ 87 x
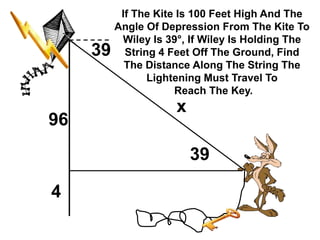
- 10. If The Kite Is 100 Feet High And The Angle Of Depression From The Kite To Wiley Is 39┬░, If Wiley Is Holding The String 4 Feet Off The Ground, Find The Distance Along The String The Lightening Must Travel To Reach The Key. 4 96 39 x 39
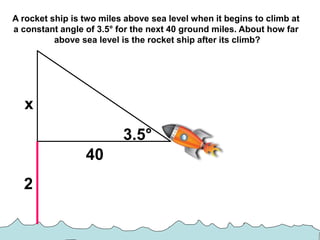
- 12. A rocket ship is two miles above sea level when it begins to climb at a constant angle of 3.5┬░ for the next 40 ground miles. About how far above sea level is the rocket ship after its climb? 3.5┬░ 40 x 2
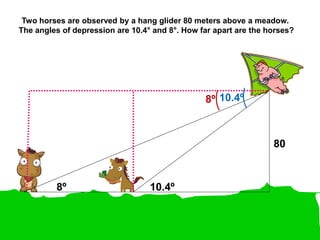
- 13. Two horses are observed by a hang glider 80 meters above a meadow. The angles of depression are 10.4┬░ and 8┬░. How far apart are the horses? 8┬║ 10.4┬║ 80 8┬║ 10.4┬║
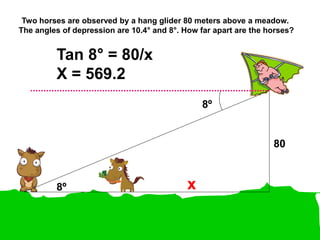
- 14. Two horses are observed by a hang glider 80 meters above a meadow. The angles of depression are 10.4┬░ and 8┬░. How far apart are the horses? 8┬║ 80 8┬║ Tan 8┬░ = 80/x X = 569.2 x
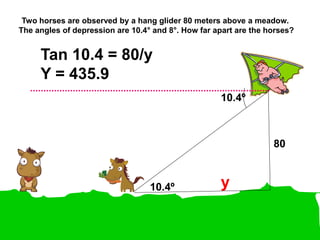
- 15. Two horses are observed by a hang glider 80 meters above a meadow. The angles of depression are 10.4┬░ and 8┬░. How far apart are the horses? 10.4┬║ 80 10.4┬║ y Tan 10.4 = 80/y Y = 435.9
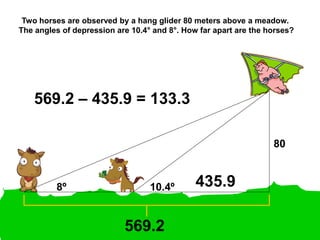
- 16. Two horses are observed by a hang glider 80 meters above a meadow. The angles of depression are 10.4┬░ and 8┬░. How far apart are the horses? 80 8┬║ 10.4┬║ 569.2 435.9 569.2 ŌĆō 435.9 = 133.3
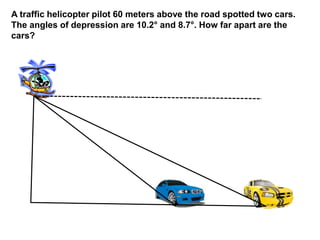
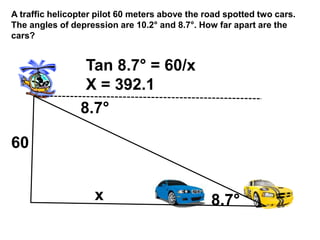
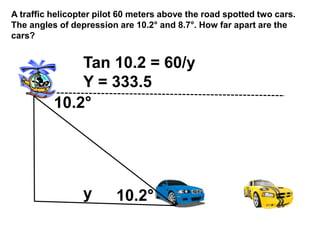
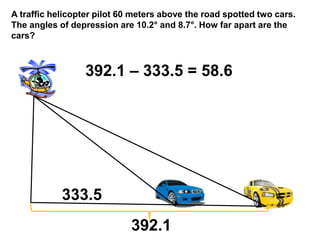
- 17. A traffic helicopter pilot 60 meters above the road spotted two cars. The angles of depression are 10.2┬░ and 8.7┬░. How far apart are the cars?
- 18. A traffic helicopter pilot 60 meters above the road spotted two cars. The angles of depression are 10.2┬░ and 8.7┬░. How far apart are the cars? 8.7┬░ 8.7┬░ 60 Tan 8.7┬░ = 60/x X = 392.1 x
- 19. A traffic helicopter pilot 60 meters above the road spotted two cars. The angles of depression are 10.2┬░ and 8.7┬░. How far apart are the cars? 10.2┬░ 10.2┬░ y Tan 10.2 = 60/y Y = 333.5
- 20. A traffic helicopter pilot 60 meters above the road spotted two cars. The angles of depression are 10.2┬░ and 8.7┬░. How far apart are the cars? 392.1 333.5 392.1 ŌĆō 333.5 = 58.6







