�ݺ�ߣ

Bell work 8-21-12
2330
342 xyyxyx ••
( )222
2 xyx
3
34
2
3
−
−
xy
yx
)323(4)432(3 22
−−−+− xyxxyx
)142(3)473( −−−−+ baba
Simplify
Simplify

Objectives
The student will be able to:
1. find the degree of a polynomial.
2. arrange the terms of a polynomial
in ascending or descending order.
SOL: none
Designed by Skip Tyler, Varina High School

What does each prefix mean?
mono
one
bi
two
tri
three

What about poly?
one or more
A polynomial is a monomial or a
sum/difference of monomials.
Important Note!!
An expression is not a polynomial if
there is a variable in the denominator.

State whether each expression is
a polynomial. If it is, identify it.
1) 7y - 3x + 4
trinomial
2) 10x3
yz2
monomial
3)
not a polynomial
2
5
7
2
y
y
+

The degree of a monomial is the sum
of the exponents of the variables.
Find the degree of each monomial.
1) 5x2
2
2) 4a4
b3
c
8
3) -3
0

To find the degree of a polynomial,
find the largest degree of the terms.
1) 8x2
- 2x + 7
Degrees: 2 1 0
Which is biggest? 2 is the degree!
2) y7
+ 6y4
+ 3x4
m4
Degrees: 7 4 8
8 is the degree!

Find the degree of x5
– x3
y2
+ 4
1. 0
2. 2
3. 3
4. 5
5. 10

A polynomial is normally put in
ascending or descending order.
What is ascending order?
Going from small to big exponents.
What is descending order?
Going from big to small exponents.

Put in descending order:
1) 8x - 3x2
+ x4
- 4
x4
- 3x2
+ 8x - 4
2) Put in descending order in terms of x:
12x2
y3
- 6x3
y2
+ 3y - 2x
-6x3
y2
+ 12x2
y3
- 2x + 3y

3) Put in ascending order in terms of y:
12x2
y3
- 6x3
y2
+ 3y - 2x
-2x + 3y - 6x3
y2
+ 12x2
y3
4) Put in ascending order:
5a3
- 3 + 2a - a2
-3 + 2a - a2
+ 5a3

Write in ascending order in terms of y:
x4
– x3
y2
+ 4xy –2x2
y3
1. x4
+ 4xy– x3
y2
–2x2
y3
2. –2x2
y3
– x3
y2
+ 4xy + x4
3. x4
– x3
y2
–2x2
y3
+ 4xy
4. 4xy –2x2
y3
– x3
y2
+ x4

Objectives
The student will be able to:
1. add and subtract polynomials.
SOL: A.11
Designed by Skip Tyler, Varina High School

1. Add the following polynomials:
(9y - 7x + 15a) + (-3y + 8x - 8a)
Group your like terms.
9y - 3y - 7x + 8x + 15a - 8a
6y + x + 7a

Combine your like terms.
3a2
+ 3ab + 4ab - b2
+ 6b2
3a2
+ 7ab + 5b2
2. Add the following polynomials:
(3a2
+ 3ab - b2
) + (4ab + 6b2
)

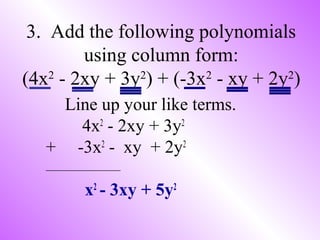
Line up your like terms.
4x2
- 2xy + 3y2
+ -3x2
- xy + 2y2
_________________________
x2
- 3xy + 5y2
3. Add the following polynomials
using column form:
(4x2
- 2xy + 3y2
) + (-3x2
- xy + 2y2
)

Rewrite subtraction as adding the
opposite.
(9y - 7x + 15a) + (+ 3y - 8x + 8a)
Group the like terms.
9y + 3y - 7x - 8x + 15a + 8a
12y - 15x + 23a
4. Subtract the following polynomials:
(9y - 7x + 15a) - (-3y + 8x - 8a)

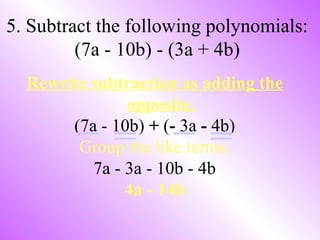
Rewrite subtraction as adding the
opposite.
(7a - 10b) + (- 3a - 4b)
Group the like terms.
7a - 3a - 10b - 4b
4a - 14b
5. Subtract the following polynomials:
(7a - 10b) - (3a + 4b)

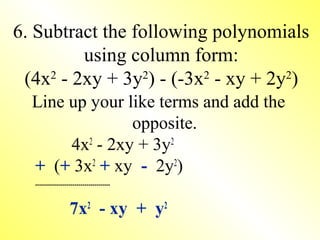
Line up your like terms and add the
opposite.
4x2
- 2xy + 3y2
+ (+ 3x2
+ xy - 2y2
)
--------------------------------------
7x2
- xy + y2
6. Subtract the following polynomials
using column form:
(4x2
- 2xy + 3y2
) - (-3x2
- xy + 2y2
)

Find the sum or difference.
(5a – 3b) + (2a + 6b)
1. 3a – 9b
2. 3a + 3b
3. 7a + 3b
4. 7a – 3b

Find the sum or difference.
(5a – 3b) – (2a + 6b)
1. 3a – 9b
2. 3a + 3b
3. 7a + 3b
4. 7a – 9b

yx 43 +
yx −5
The measures of two sides of a triangle are given. If P is the
perimeter, and , find the measure of the
third side.
yxP 510 +=

�ݺ�ߣ

Add sub polynomials

More Related Content

Add sub polynomials