Applications of Systmes
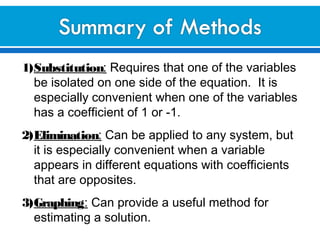
- 1. 1)Substitution: Requires that one of the variables be isolated on one side of the equation. It is especially convenient when one of the variables has a coefficient of 1 or -1. 2)Elimination: Can be applied to any system, but it is especially convenient when a variable appears in different equations with coefficients that are opposites. 3)Graphing: Can provide a useful method for estimating a solution.
- 2. ÔÇñÔÇó
- 3. 1. Define all variables. 2. Write the system of equations. 3. Solve using the best method & showing all steps. 4. State your solution in sentence form. 5. Check your solution.
- 4. 1. You are selling tickets for a high school basketball game. Student tickets cost $3 and general admission tickets cost $5. You sell 350 tickets and collect $1450. How many of each type of ticket did you sell?
- 5. Define variables:   System of equations: State your solution(s): Solve S = # of Student Tickets G = # of General Admin Tickets S + G = 350 3S + 5G = 1450 G = 200 S = 150I sold 200 general admission tickets and 150 student tickets.
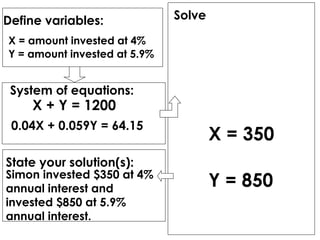
- 6. 2. Simon invests $1200 into two savings accounts. One account earns 4% annual interest and the other earns 5.9% annual interest. At the end of 1 year, Simon earned $64.15 in interest. How much did he invest at each rate?
- 7. Define variables:   System of equations: State your solution(s): Solve X = amount invested at 4% Y = amount invested at 5.9% X + Y = 1200 0.04X + 0.059Y = 64.15 X = 350 Y = 850Simon invested $350 at 4% annual interest and invested $850 at 5.9% annual interest.
- 8. 3. At an Italian bistro, the costs of 2 plates of spaghetti and 1 salad is $27.50. The cost for 4 plates of spaghetti and 3 salads is $59.50. Find the cost of a plate of spaghetti and a salad.
- 9. Define variables:   System of equations: State your solution(s): Solve P = cost plate of spaghetti S = cost salad 2P + S = 27.50 4P + 3S = 59.50 P = 11.50 S = 4.50A plate of spaghetti costs $11.50 and a salad costs $4.50.
- 10. 4. Peggy walks at a rate of 2 miles per hour and jogs at a rate of 4 miles per hour. She walked and jogged 3.4 miles in 1.2 hours. For how long did Peggy jog and for how long did she walk?
- 11. Define variables:   System of equations: State your solution(s): Solve W = hours walked J = hours jogged W + J = 1.2 2W + 4J = 3.4 W = .7 J = .5Peggy walked for 0.7 hours and jogged for 0.5 hours.