CE297-Ch3.pdf Rigid bodies internal and external forces
- 1. Chapter 3: Rigid Bodies; Equivalent Systems of forces Rigid Bodies: Bodies in which the relative position of any two points does not change. • In real life no body is perfectly rigid. We can approximate the behavior of most structures with rigid bodies because the deformations are usually small and negligible. Even in cases when the deformations are not negligible, we can still apply the principles of equilibrium and statics to the deformed or the current configuration of the body to find out what forces the body is carrying. Note: 3.1 -3.3 Introduction, Internal External Forces For example: force of gravity, applied external force on an object. External forces on a rigid body are due to causes that are external to the body. They can cause the body to move or remain at rest as a whole. For example: Internal TENSION or COMPRESSION in a bar, bending moment in a beam. Internal forces develop in any body (not just rigid bodies) that keep all the particles of a body together. External and Internal forces: • Similarly compression. Tension: Friday, September 04, 2009 11:26 AM CE297-FA09-Ch3 Page 1
- 2. Any force that is applied to a rigid body causes the body to translate or ROTATE or both. • The tendency to ROTATE is caused by MOMENTS generated by the force. • 3.4 -3.8 Moment of a force; Vector CROSS Product In general, a force F generates a moment about any point O which is offset by some distance from the line of action of F. • Moment of F about O. Moment is a VECTOR. Note: Wednesday, September 09, 2009 7:52 AM CE297-FA09-Ch3 Page 2
- 3. • Vector product of two vectors P and Q is defined as the vectorV which satisfiesthe following conditions: 1.Lineof action of V is perpendicularto plane containingP and Q. 2.Magnitude of V is 3.Directionof V is obtainedfrom the right-hand rule. θ sin Q P V = • Vector products: - are not commutative, - are distributive, - are not associative, ( ) Q P P Q × − = × ( ) 2 1 2 1 Q P Q P Q Q P × + × = + × ( ) ( ) S Q P S Q P × × ≠× × Vector Cross Product: • Vectorproductsof Cartesian unit vectors, 0 0 0 = × = × − = × − = × = × = × = × − = × = × k k i k j j k i i j k j j k j i j i k k i j i i • Vector productsin terms of rectangular coordinates ( ) ( ) k Q j Q i Q k P j P i P V z y x z y x + + × + + = ( ) ( ) ( ) y z z y z x x z x y y x P Q PQ i PQ P Q j P Q P Q k = − + − + − Thus Moment of F about O can be obtained as: Wednesday, September 09, 2009 8:59 AM CE297-FA09-Ch3 Page 3
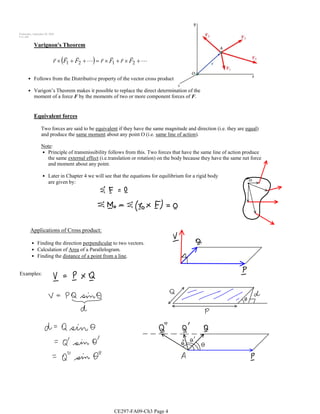
- 4. ( ) ⋯ ⋯ + × + × = + + × 2 1 2 1 F r F r F F r Varignon's Theorem Follows from the Distributive property of the vector cross product • Varigon’s Theorem makes it possible to replace the direct determination of the moment of a force F by the moments of two or more component forces of F. • Two forces are said to be equivalent if they have the same magnitude and direction (i.e. they are equal) and produce the same moment about any point O (i.e. same line of action). Principle of transmissibility follows from this. Two forces that have the same line of action produce the same external effect (i.e.translation or rotation) on the body because they have the same net force and moment about any point. • Later in Chapter 4 we will see that the equations for equilibrium for a rigid body • are given by: Note: Equivalent forces Applications of Cross product: Finding the direction perpendicular to two vectors. • Calculation of Area of a Parallelogram. • Finding the distance of a point from a line. • Examples: Wednesday, September 09, 2009 9:31 AM CE297-FA09-Ch3 Page 4
- 5. Read Examples 3.2, 3.3, 3.4. Example 3.1 A 100-lb vertical force is applied to the end of a lever which is attached to a shaft at O. Determine: moment about O, a) horizontal force at A which creates the same moment, b) smallest force at A which produces the same moment, c) location for a 240-lb vertical force to produce the same moment, d) whether any of the forces from b, c, and d is equivalent to the original force. e) Monday, September 14, 2009 6:07 PM CE297-FA09-Ch3 Page 5
- 6. Exercise 3.25 3.31 Given tension in each cable is 810N. Determine the moment at A due to the force at D. • Find the distance of point A from the line DE. • Recall Moment of F about A can be obtained as: Friday, September 11, 2009 9:37 AM CE297-FA09-Ch3 Page 6
- 7. 3.9 Scalar Product of two Vectors The scalar product or dot product between two vectors P and Q is defined as • ( ) result scalar cosθ PQ Q P = • are commutative, • are distributive, • are not associative, • Scalar products: • P Q Q P • = • ( ) 2 1 2 1 Q P Q P Q Q P • + • = + • ( ) undefined = • • S Q P Scalar products with Cartesian unit components, • 0 0 0 1 1 1 = • = • = • = • = • = • i k k j j i k k j j i i ( ) ( ) k Q j Q i Q k P j P i P Q P z y x z y x + + • + + = • 2 2 2 2 P P P P P P Q P Q P Q P Q P z y x z z y y x x = + + = • + + = • Applications of Dot Product W = F• • • •d = F d cosθ Work done by a force • Angle between two vectors: • PQ Q P Q P Q P Q P Q P Q P PQ Q P z z y y x x z z y y x x + + = + + = = • θ θ cos cos Projection of a vector on a given axis: • OL OL P P Q Q P PQ Q P OL P P P = = • = • = = θ θ θ cos cos along of projection cos Component in a given direction (unit vector): • z z y y x x OL P P P P P θ θ θ λ cos cos cos + + = • = Monday, September 14, 2009 11:13 AM CE297-FA09-Ch3 Page 7
- 8. 3.10 Mixed TRIPLE Product of 3 Vectors Mixed triple product of three vectors, • ( ) result scalar = × • Q P S The six mixed triple products formed from S, P, and Q have equal magnitudes but not the same sign, • Evaluating the mixed triple product, • ( ) ( ) ( ) ( ) ( ) ( ) S P Q Q S P P Q S P S Q S Q P Q P S × • − = × • − = × • − = × • = × • = × • ( ) ( ) ( ) ( ) z y x z y x z y x x y y x z z x x z y y z z y x Q Q Q P P P S S S Q P Q P S Q P Q P S Q P Q P S Q P S = − + − + − = × • 3.11 Moment of a force about an Axis Note: MOL is the projection of MO along OL. Finding the perpendicular distance between two non-intersecting lines in 3D space. Application: Read Example 3.5 in the book. A cube is acted on by a force P as shown. Determine the moment of P about A a) about the edge AB and b) about the diagonal AG of the cube. c) Determine the perpendicular distance between AG and FC. d) CE297-FA09-Ch3 Page 8
- 9. 3.12-3.13 Force Couples Two forces F and -F having the same magnitude, parallel lines of action, and opposite sense are said to form a couple. • ( ) ( ) Fd rF M F r F r r F r F r M B A B A = = × = × − = − × + × = θ sin Moment of the couple, • Note: Moment of a couple is always the same about any point. Equivalent Couples Two or more couples are equivalent iff they produce the same moment. 2 2 1 1 d F d F = • the two couples lie in parallel planes, and • the two couples have the same sense or the tendency to cause rotation in the same direction. • 3.14-3.15 Couples (and Moments) are Vectors Wednesday, September 16, 2009 9:48 AM CE297-FA09-Ch3 Page 9
- 10. Addition of Couples 2 2 2 1 1 1 plane in plane in P F r M P F r M × = × = ( ) 2 1 F F r R r M + × = × = 2 1 2 1 M M F r F r M + = × + × = Moment due to the individual couples: VECTOR sum of the two moments: Moment due to the resultant of the forces: 3.16 Equivalent Force and Moment Example 3.6 CE297-FA09-Ch3 Page 10
- 11. 3.17-3.18 Equivalent Systems of Forces Moments Any system of forces can be reduced to ONE resultant force and ONE resultant moment. • Once a resultant force moment has been found about O, a new resultant force moment about a different point 0' can be found as follows: • Two or more systems of forces Moments are said to be equivalent iff they have the same resultant force and the resultant moment about any (and all) points O. • Wednesday, September 16, 2009 4:21 PM CE297-FA09-Ch3 Page 11
- 12. 3.20 Special Case: Reduction to a SINGLE force In general, a system of forces and moments cannot be reduced to just a single force. However, if the resultant moment is perpendicular to the resultant force, one can reduce the system to just ONE force and NO moment. Concurrent forces: Forces acting at the same point. • Particular cases: Coplanar forces: Forces contained in the same plane (with non-concurrent lines of action) • Parallel forces in 3D space. • Thursday, September 17, 2009 10:08 PM CE297-FA09-Ch3 Page 12
- 13. Exercise 3.88 The shearing forces exerted on the cross section of a steel channel can be represented as 900N vertical and two 250 N horizontal forces. (C is called the shear center) Replace these forces with a SINGLE force at C. • Determine x. • Example 3.8 at A at B Reduce the forces to an equivalent force moment Reduce them to SINGLE force and find where it acts. Thursday, September 17, 2009 10:50 PM CE297-FA09-Ch3 Page 13