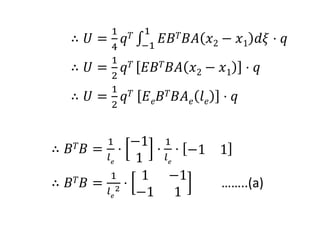Computer aided design
Download as pptx, pdf3 likes325 views
This document describes the derivation of the structural stiffness matrix used in structural analysis. It shows that the strain is related to displacement and geometry. The strain energy of an element is then related to the forces and displacements. Equating the partial derivative of the total potential energy to zero results in a system of equations that can be written in matrix form as KQ=F, where K is the structural stiffness matrix, Q are the nodal displacements, and F are the applied nodal forces. This generalized matrix equation is used for structural analysis.
![??
??
=
2
?2
??1
.(2)
? =
??
??
??
??
? =
?2
??1
?2
??1
? =
1
?2
??1
?1 1
?1
?2
LENGTH OF THE ELEMENT [B]
? = ? ? ?](https://image.slidesharecdn.com/cad-160919175915/85/Computer-aided-design-4-320.jpg)
Computer aided design
- 1. Computer Aided Design Topic : Derivation Of Structural Stiffness Matrix Prepared By : Abhirajsinh Mahida
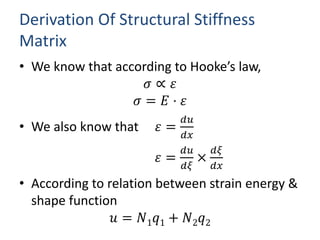
- 2. Derivation Of Structural Stiffness Matrix ? We know that according to Hookes law, ? ? ? = ? ? ? ? We also know that ? = ?? ?? ? = ?? ?? ?? ?? ? According to relation between strain energy & shape function ? = ?1 ?1 + ?2 ?2
- 3. ? = ?1 ?1 + ?2 ?2 ? = 1?? 2 ? ?1 + 1+? 2 ? ?2 ?? ?? = ? ?1 2 + ?2 2 (1) Where, ?1 & ?2 = displacements ? Then we also know the equation of ? in terms of x according to coordinate systems : ? = 2 ???1 ?2 ??1 ? 1
- 4. ?? ?? = 2 ?2 ??1 .(2) ? = ?? ?? ?? ?? ? = ?2 ??1 ?2 ??1 ? = 1 ?2 ??1 ?1 1 ?1 ?2 LENGTH OF THE ELEMENT [B] ? = ? ? ?
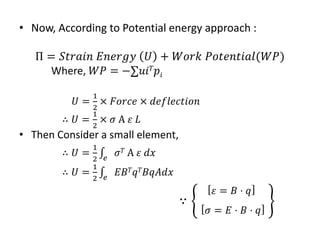
- 5. ? Now, According to Potential energy approach : = ?????? ?????? ? + ???? ?????????(??) Where, ?? = ??? ? ?? ? = 1 2 ????? ?????????? ? = 1 2 ? ? ? ? Then Consider a small element, ? = 1 2 ? ? ? ? ?? ? = 1 2 ? ?? ? ? ? ????? ? = ? ? ? ? = ? ? ? ? ?
- 6. ? = 1 4 ? ? ?1 1 ?? ? ?? ?2 ? ?1 ?? ? ? ? = 1 2 ? ? ?? ? ?? ?2 ? ?1 ? ? ? = 1 2 ? ? ? ? ? ? ?? ? ? ? ? ? ? ? ? = 1 ? ? ? ?1 1 ? 1 ? ? ? ?1 1 ? ? ? = 1 ? ? 2 ? 1 ?1 ?1 1 ..(a)
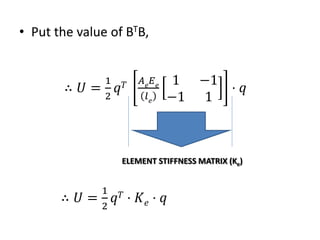
- 7. ? Put the value of BTB, ? = 1 2 ? ? ? ? ? ? ? ? 1 ?1 ?1 1 ? ? ELEMENT STIFFNESS MATRIX (Ke) ? = 1 2 ? ? ? ? ? ? ?
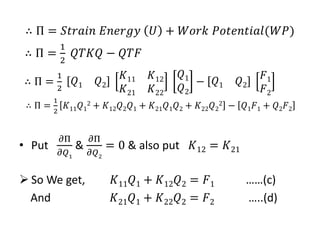
- 8. = ?????? ?????? ? + ???? ?????????(??) = 1 2 ???? ? ??? = 1 2 ?1 ?2 ?11 ?12 ?21 ?22 ?1 ?2 ? ?1 ?2 ?1 ?2 = 1 2 ?11 ?1 2 + ?12 ?2 ?1 + ?21 ?1 ?2 + ?22 ?2 2 ? ?1 ?1 + ?2 ?2 ? Put ? ??1 & ? ??2 = 0 & also put ?12 = ?21 ? So We get, ?11 ?1 + ?12 ?2 = ?1 (c) And ?21 ?1 + ?22 ?2 = ?2 ..(d)
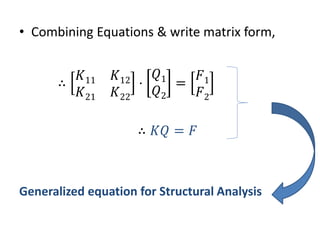
- 9. ? Combining Equations & write matrix form, ?11 ?12 ?21 ?22 ? ?1 ?2 = ?1 ?2 ?? = ? Generalized equation for Structural Analysis
- 10. THANK YOU