DC-circuit-theory.ppt
- 2. Learning outcomes • explain the behaviour of DC circuits using concepts of EMF, internal resistance of power sources and potential dividers • give a microscopic description of resistance in a wire • define and use concepts of resistivity and conductance • state Kirchhoff’s laws and use them to analyse DC circuits • define capacitance and solve DC circuit problems involving capacitors, including energy stored • carry out related practical work (using voltmeter, ammeter, multimeter, micrometer)
- 3. Teaching challenges It is always advisable to revisit concepts introduced at KS3 and GCSE level, to identify misconceptions about electricity and (try to) correct them. In pairs: Make a spidergram showing key concepts related to electric circuits, and relationships between them.
- 4. A-level: A battery maintains an electric field through the circuit. This enables it to do work on charges wherever there is a potential difference e.g. in a filament. Electromotive force is the energy supplied per unit charge. (work done on each coulomb of charge) Potential difference (p.d.) is the energy transferred per unit charge between 2 points in a circuit. (work done by each coulomb of charge) Unit (for both) is the volt = joule/coulomb EMF and potential difference
- 5. Resistors in series V = V1+ V2 [conservation of energy] IR = IR1 + IR2 R = R1 + R2 R is always larger than any of R1, R2 etc Resistors in parallel I = I1 + I2 [conservation of charge] V/R = V/R1 + V/R2 1/R = 1/R1 + 1/R2 R is always smaller than any of R1, R2 etc Resistor networks
- 6. Useful for constructing sensors In pairs, sketch • a dark sensor • a heat sensor • a cold sensor Potential dividers 2 1 2 1 2 1 R R IR IR V V  
- 7. Real power supplies Demonstrations: • 12V DC supply lighting more and more lamps in parallel • EHT with a 1.5V lamp What’s happening? E = Vinternal ('lost volts')+Vexternal E = I(r + R) IR = E - Ir terminal V = E - Ir
- 8. Graphical representation y = mx +c V = -rI + E
- 9. Resistance in a wire microscopic picture: free electrons drifting through a metal (polycrystalline, each crystal having an ionic lattice) • constant of proportionality is resistivity, unit  m • a material property Compare with rules for R networks. VPL simulation. A l R A R l R     1 l RA  
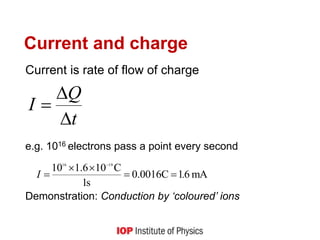
- 10. Current and charge Current is rate of flow of charge e.g. 1016 electrons pass a point every second Demonstration: Conduction by ‘coloured’ ions t Q I    mA 6 1 C 0016 . 0 s 1 C 10 6 . 1 10 19 16 . I      
- 11. Drift velocity where • n is the number of free electrons per unit volume • A is the cross sectional area • Δx is a small length along the wire • e is the charge of an electron e x nA Q Q ) ( particle per charge particles charged of number       I = DQ Dt = nADxe Dt vd = Dx Dt I = nAvde
- 12. Comparing copper with tungsten The difference in drift velocities explains why incandescent lamps glow white hot while their connecting wires stay safely at room temperature. metal electrons per m3 electron drift velocity in mm s-1 copper 8.5 √ó 1028 ~0.02 tungsten 3.4 √ó 1028 ~250
- 13. Conductivity Metal wires conduct extremely well. Conductance G = I / V , unit siemens (symbol S) • depends on the number of carriers available • ratio I / V is 'effect per unit of cause‘ Note: • conductance is the reciprocal of resistance • conductivity,  [unit S m-1] is the reciprocal of resistivity
- 14. Capacitance • a measure of how much charge a capacitor can separate at a given p.d. • unit of capacitance: farad (symbol F) demonstration super-capacitor Note: There are rules for adding capacitors in networks. V Q C   p.d. charge e capacitanc 2 2 1 2 1 stored, Energy CV QV W  
- 15. Lab practicals • internal resistance of a potato cell • resistivity of a wire (using micrometer) • charging and discharging a capacitor
- 16. Kirchhoff’s 1st law The total current entering a circuit junction equals the total current leaving it. [conservation of charge]
- 17. Kirchhoff’s 2nd law The sum of the emfs round a loop in any circuit = the sum of the p.d.s round the loop. [conservation of energy] E1 + E2 + E3 + … = I1R1 + I2R2 + I3R3 + … where I1, I2, I3 … represent currents through the resistances R1, R2, R3 … Physlets (simulations): ‘Second semester’< ‘DC Circuits’ • ‘Kirchhoff's Loop Rule’ • ‘Applying Kirchhoff's Rules’
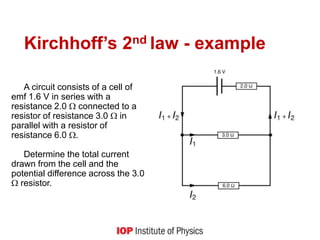
- 18. Kirchhoff’s 2nd law - example A circuit consists of a cell of emf 1.6 V in series with a resistance 2.0  connected to a resistor of resistance 3.0  in parallel with a resistor of resistance 6.0 . Determine the total current drawn from the cell and the potential difference across the 3.0  resistor.
- 19. Consider the circuit loop consisting of the cell and the 3.0  resistor: 1.6 V = 3 I1 + 2 (I1 + I2) Thus 1.6 V = 5 I1 + 2 I2 …(1) Consider the circuit loop consisting of the cell and the 6.0  resistor: 1.6 V = 6 I2 + 2 (I1 + I2) Thus 1.6 V = 2 I1 + 8  I2 …(2) Subtracting the second equation from the first gives: 0 V = 3 I1 - 6 I2 hence I1 = 2 I2 Substituting I1 = 2 I2 into the second equation gives: 1.6 V = 12 I2 Thus I2 = 0.13 A and I1 = 0.27 A Current through cell = I1 + I2 = 0.40 A pd across 3.0  resistor = I1 × 3.0 (= I2 × 6.0 ) = 0.8 V Solution
- 20. Endpoints Related topics • sensors of many types use the potential divider principle • factors affecting capacitance (plate spacing & area, dielectric material) • exponential nature of charging and discharging capacitors • how ammeters and voltmeters affect circuit behaviour • maximum power theorem • AC circuit theory





![Resistors in series
V = V1+ V2 [conservation of energy]
IR = IR1 + IR2
R = R1 + R2 R is always larger than any of R1, R2 etc
Resistors in parallel
I = I1 + I2 [conservation of charge]
V/R = V/R1 + V/R2
1/R = 1/R1 + 1/R2 R is always smaller than any of R1, R2 etc
Resistor networks](https://image.slidesharecdn.com/dc-circuit-theory-230217135655-efaea184/85/DC-circuit-theory-ppt-5-320.jpg)






![Conductivity
Metal wires conduct extremely well.
Conductance G = I / V , unit siemens (symbol S)
• depends on the number of carriers available
• ratio I / V is 'effect per unit of cause‘
Note:
• conductance is the reciprocal of resistance
• conductivity,  [unit S m-1] is the reciprocal of resistivity](https://image.slidesharecdn.com/dc-circuit-theory-230217135655-efaea184/85/DC-circuit-theory-ppt-13-320.jpg)


![Kirchhoff’s 1st law
The total current
entering a circuit
junction equals the
total current
leaving it.
[conservation of charge]](https://image.slidesharecdn.com/dc-circuit-theory-230217135655-efaea184/85/DC-circuit-theory-ppt-16-320.jpg)
![Kirchhoff’s 2nd law
The sum of the emfs round a loop in any circuit
= the sum of the p.d.s round the loop.
[conservation of energy]
E1 + E2 + E3 + … = I1R1 + I2R2 + I3R3 + …
where I1, I2, I3 … represent currents through the resistances R1, R2,
R3 …
Physlets (simulations): ‘Second semester’< ‘DC Circuits’
• ‘Kirchhoff's Loop Rule’
• ‘Applying Kirchhoff's Rules’](https://image.slidesharecdn.com/dc-circuit-theory-230217135655-efaea184/85/DC-circuit-theory-ppt-17-320.jpg)