discriminants.pptx
- 2. What is to be learned? What the discriminant is How we use the discriminant to find out how many solutions there are (if any)
- 3. Quadratic Formula x = -b + - b2 4ac 2a How many solutions? 1. x2 + 6x + 9 = 0 2. x2 + 8x + 9 = 0 3. x2 + 4x + 9 = 0 1 2 0
- 4. The Big Nasty Formula 2a x = -b + - b2 4ac How many solutions? 1. x2 + 6x + 9 = 0 2. x2 + 8x + 9 = 0 3. x2 + 4x + 9 = 0 1 2 0 The Discriminant tells you how many solutions you have zero positive negative
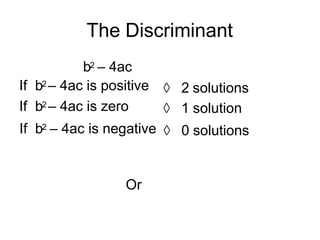
- 5. The Discriminant b2 4ac If b2 4ac is positive If b2 4ac is zero If b2 4ac is negative 0 solutions 2 solutions 1 solution
- 6. The Discriminant b2 4ac If b2 4ac is positive If b2 4ac is zero If b2 4ac is negative 0 solutions Or 2 solutions 1 solution
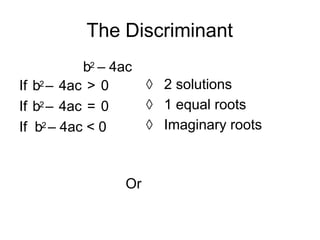
- 7. The Discriminant b2 4ac If b2 4ac > 0 If b2 4ac = 0 If b2 4ac < 0 2 solutions 1 equal roots Imaginary roots Or
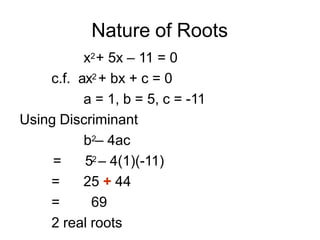
- 8. Nature of Roots x2+ 5x 11 = 0 c.f. ax2 + bx + c = 0 a = 1, b = 5, c = -11 Using Discriminant b2 4ac = 52 4(1)(-11) = 25 + 44 = 69 2 real roots
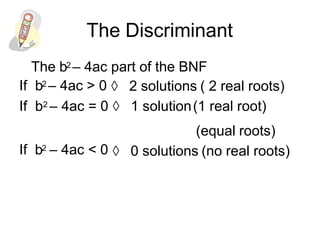
- 9. The Discriminant The b2 4ac part of the BNF If b2 4ac > 0 2 solutions ( 2 real roots) If b2 4ac = 0 1 solution(1 real root) (equal roots) If b2 4ac < 0 0 solutions (no real roots)
- 10. Nature of Roots x2+ 6x + 10 = 0 c.f. ax2 + bx + c = 0 a = 1, b = 6, c = 10 Using Discriminant b2 4ac = 62 4(1)(10) = - 4 no real roots Must be written like this
- 11. Key Question Find the nature of the roots of this equation 3m(m + 2) + 4m = 7 3m2 + 6m + 4m = 7 3m2 + 10m 7 = 0 c.f. am2 + bm + c = 0 a = 3, b = 10, c = -7 Using Discriminant b2 4ac = 102 4(3)(-7) = 100 + 84 = 184
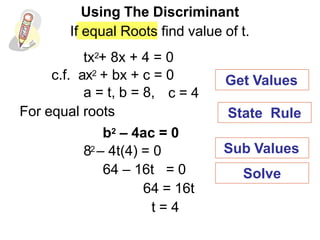
- 12. Using The Discriminant If equal Roots find value of t. tx2+ 8x + 4 = 0 c.f. ax2 + bx + c = 0 a = t, b = 8, For equal roots 64 16t = 0 64 = 16t t = 4 b2 4ac = 0 82 4t(4) = 0 c = 4 State Rule Get Values Sub Values Solve
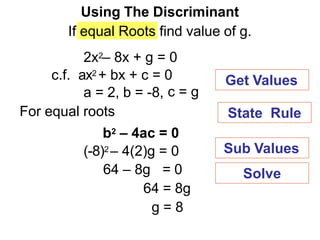
- 13. 64 8g = 0 64 = 8g g = 8 c.f. ax2 + bx + c = 0 a = 2, b = -8, c = g For equal roots b2 4ac = 0 (-8)2 4(2)g = 0 Using The Discriminant If equal Roots find value of g. 2x2 8x + g = 0 State Rule Get Values Sub Values Solve
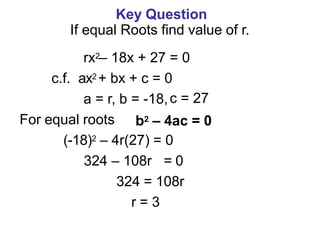
- 14. Key Question If equal Roots find value of r. rx2 18x + 27 = 0 c.f. ax2 + bx + c = 0 a = r, b = -18,c = 27 For equal roots (-18)2 4r(27) = 0 324 108r = 0 324 = 108r r = 3 b2 4ac = 0
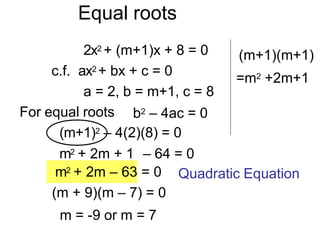
- 15. Equal roots 2x2 + (m+1)x + 8 = 0 c.f. ax2 + bx + c = 0 a = 2, b = m+1, c = 8 For equal roots m2 + 2m 63 = 0 (m + 9)(m 7) = 0 m = -9 or m = 7 b2 4ac = 0 (m+1)2 4(2)(8) = 0 m2 + 2m + 1 64 = 0 (m+1)(m+1) =m2 +2m+1 Quadratic Equation
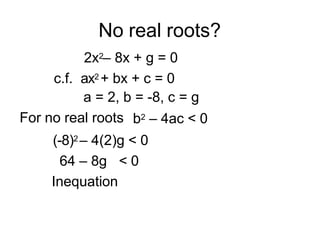
- 16. No real roots? 2x2 8x + g = 0 c.f. ax2 + bx + c = 0 a = 2, b = -8, c = g For no real roots b2 4ac < 0 (-8)2 4(2)g < 0 64 8g < 0 Inequation
- 17. Solving Equations - Reminder 4x = 12 4x > 12 x = 3 x > 3 (Solution) 4x 2 = 10 Solving Inequations 4x 2 > = 10 Main difference is the sign in the middle One other bigdifference
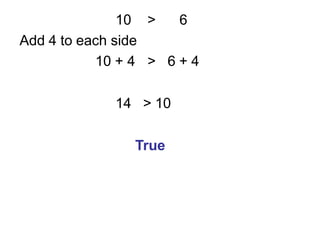
- 18. 10 > 6 Add 4 to each side 10 + 4 > 6 + 4 14 > 10 True
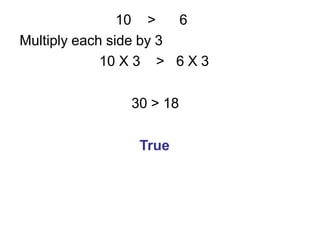
- 19. 10 > 6 Multiply each side by 3 10 X 3 > 6 X 3 30 > 18 True
- 20. 10 > 6 Divide each side by 2 10 歎 2 > 6 歎 2 5 > 3 True
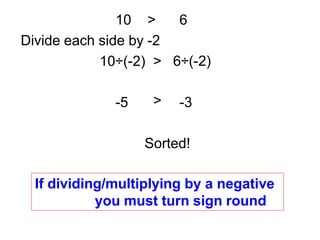
- 21. 10 > 6 Divide each side by -2 10歎(-2) > 6歎(-2) -5 -3 False!!!!!!! > If dividing/multiplying by a negative you must turn sign round Sorted!
- 22. Back to. 64 8g < 0 8g < -64 g > 8 No real roots if g > 8