�ݺ�ߣ

Copyright © ODL Jan 2005 Open University Malaysia
1
Subject Matter Expert/Author: Assoc. Prof. Dr Othman A. Karim (OUM)
Faculty of Engineering and
Technical Studies
FLUID MECHANICS FOR
CIVIL ENGINEERING TUTORIAL
1 –
UNIT 1: Elements of Fluid Mechanics
Chapter 1: Fluid Mechanics and
Fluid Properties
Assoc. Prof. Dr Othman A. Karim
EBVF4103 Fluid Mechanics for Civil Engineering
Jan 2005

2
Technical Studies
SEQUENCE OF CHAPTER 1
Introduction
Objectives
1.1 Definition of A Fluid
Shear stress in moving fluid
Differences between liquid and gases
Newtonian and Non-Newtonian Fluid
1.2 Engineering Units
1.3 Fluid Properties
Vapor Pressure
Engineering significance of vapor pressure
Surface Tension
Capillarity
Example 1.2
Example 1.3
Summary

3
Technical Studies
Introduction
• Fluid mechanics is a study of the behavior of fluids,
either at rest (fluid statics) or in motion (fluid
dynamics).
• The analysis is based on the fundamental laws of
mechanics, which relate continuity of mass and energy
with force and momentum.
• An understanding of the properties and behavior of
fluids at rest and in motion is of great importance in
engineering.

4
Technical Studies
1. Identify the units for the basic quantities of time,
length, force and mass.
2. Properly set up equations to ensure consistency of
units.
3. Define the basic fluid properties.
4. Identify the relationships between specific weight,
specific gravity and density, and solve problems using
their relationships.
Objectives

5
Technical Studies
1.1 Definition of Fluid
 A fluid is a substance, which deforms continuously, or
flows, when subjected to shearing force
 In fact if a shear stress is acting on a fluid it will flow
and if a fluid is at rest there is no shear stress acting on
it.
Fluid Flow Shear stress – Yes
Fluid Rest Shear stress – No

6
Technical Studies
Shear stress in moving fluid
• If fluid is in motion, shear stress are developed if the
particles of the fluid move relative to each other. Adjacent
particles have different velocities, causing the shape of the
fluid to become distorted
• On the other hand, the velocity of the fluid is the same at
every point, no shear stress will be produced, the fluid
particles are at rest relative to each other.
Moving plate Shear force
Fluid particles New particle position
Fixed surface

7
Technical Studies
Differences between liquid and gases
Liquid Gases
Difficult to compress and often
regarded as incompressible
Easily to compress – changes of volume
is large, cannot normally be neglected
and are related to temperature
Occupies a fixed volume and will
take the shape of the container
No fixed volume, it changes volume to
expand to fill the containing vessels
A free surface is formed if the
volume of container is greater
than the liquid.
Completely fill the vessel so that no free
surface is formed.

8
Technical Studies
Example:
Air
Water
Oil
Gasoline
Alcohol
Kerosene
Benzene
Glycerine
Fluid Newton’s law
of viscosity
Newtonian fluids
obey refer
Newton’s’ law of viscosity is given by;
dy
du


 (1.1)
• The viscosity  is a function only of the condition of the fluid, particularly its
temperature.
• The magnitude of the velocity gradient (du/dy) has no effect on the magnitude of .
 = shear stress
 = viscosity of fluid
du/dy = shear rate, rate of strain or velocity gradient

9
Technical Studies
Fluid Newton’s law
of viscosity
Non- Newtonian
fluids
Do not obey
•The viscosity of the non-Newtonian fluid is dependent on the
velocity gradient as well as the condition of the fluid.
Newtonian Fluids
 a linear relationship between shear stress and the velocity gradient (rate of
shear),
 the slope is constant
 the viscosity is constant
non-Newtonian fluids
 slope of the curves for non-Newtonian fluids varies

10
Technical Studies
Figure 1.1
Shear stress vs.
velocity gradient
Bingham plastic : resist a small shear stress but flow easily under large shear
stresses, e.g. sewage sludge, toothpaste, and jellies.
Pseudo plastic : most non-Newtonian fluids fall under this group. Viscosity
decreases with increasing velocity gradient, e.g. colloidal
substances like clay, milk, and cement.
Dilatants : viscosity decreases with increasing velocity gradient, e.g.
quicksand.

11
Technical Studies
Primary Units
In fluid mechanics we are generally only interested in the top four units from this
table.
1.2 Engineering Units
Quantity SI Unit
Length Metre, m
Mass Kilogram, kg
Time Seconds, s
Temperature Kelvin, K
Current Ampere, A
Luminosity Candela

12
Technical Studies
Derived Units
Quantity SI Unit
velocity m/s -
acceleration m/s2
-
force Newton (N) N = kg.m/s2
energy (or work) Joule (J) J = N.m = kg.m2
/s2
power Watt (W) W = N.m/s = kg.m2
/s3
pressure (or stress) Pascal (P) P = N/m2
= kg/m/s2
density kg/m3
-
specific weight N/m3
= kg/m2
/s2
N/m3
= kg/m2
/s2
relative density a ratio (no units) dimensionless
viscosity N.s/m2
N.s/m2
= kg/m/s
surface tension N/m N/m = kg/s2

13
Technical Studies
Unit Cancellation Procedure
1. Solve the equation algebraically for the desired terms.
2. Decide on the proper units of the result.
3. Substitute known values, including units.
4. Cancel units that appear in both the numerator and
denominator of any term.
5. Use correct conversion factors to eliminate unwanted units
and obtain the proper units as described in Step 2.
6. Perform the calculations.

14
Technical Studies
Example 1.1
Given m = 80 kg and a=10 m/s2
. Find the force
Solution
 F = ma
 F = 80 kg x 10 m/s2
= 800 kg.m/s2
 F= 800N

15
Technical Studies
1.3 Fluid Properties
Density
Density of a fluid, ,
Definition: mass per unit volume,
• slightly affected by changes in temperature and pressure.
 = mass/volume = m/ (1.2)
Units: kg/m3
Typical values:
Water = 1000 kg/m3
; Air = 1.23 kg/m3

16
Technical Studies
Fluid Properties (Continue)
Specific weight
Specific weight of a fluid, 
• Definition: weight of the fluid per unit volume
• Arising from the existence of a gravitational force
• The relationship  and g can be found using the following:
Since  = m/
therefore  = g (1.3)
Units: N/m3
Typical values:
Water = 9814 N/m3
; Air = 12.07 N/m3

17
Technical Studies
Specific gravity
The specific gravity (or relative density) can be defined in two ways:
Definition 1: A ratio of the density of a substance to the density
of water at standard temperature (4C) and
atmospheric pressure, or
Definition 2: A ratio of the specific weight of a substance to the
specific weight of water at standard
temperature (4C) and atmospheric pressure.
(1.4)
Unit: dimensionless.
C
w
s
C
w
s
SG

 





4
4 @
@

18
Technical Studies
Viscosity
• Viscosity, , is the property of a fluid, due to cohesion and
interaction between molecules, which offers resistance to shear
deformation.
• Different fluids deform at different rates under the same shear
stress. The ease with which a fluid pours is an indication of its
viscosity. Fluid with a high viscosity such as syrup deforms more
slowly than fluid with a low viscosity such as water. The viscosity is
also known as dynamic viscosity.
Units: N.s/m2
or kg/m/s
Typical values:
Water = 1.14x10-3 kg/m/s; Air = 1.78x10-5 kg/m/s

19
Technical Studies
Kinematic viscosity, 
Definition: is the ratio of the viscosity to the density;
• will be found to be important in cases in which significant
viscous and gravitational forces exist.
Units: m2
/s
Typical values:
Water = 1.14x10-6 m2/s; Air = 1.46x10-5 m2/s;
In general,
viscosity of liquids with temperature, whereas
viscosity of gases with in temperature.



 /

20
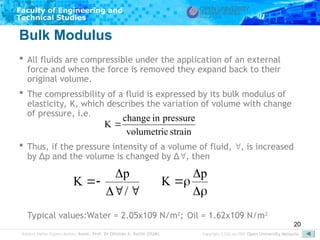
Technical Studies
Bulk Modulus
 All fluids are compressible under the application of an external
force and when the force is removed they expand back to their
original volume.
 The compressibility of a fluid is expressed by its bulk modulus of
elasticity, K, which describes the variation of volume with change
of pressure, i.e.
 Thus, if the pressure intensity of a volume of fluid, , is increased
by Δp and the volume is changed by Δ, then
Typical values:Water = 2.05x109 N/m2
; Oil = 1.62x109 N/m2
strain
volumetric
pressure
in
change
K 






/
p
K





p
K

21
Technical Studies
Vapor Pressure
 A liquid in a closed container is subjected to a partial
vapor pressure in the space above the liquid due to the
escaping molecules from the surface;
 It reaches a stage of equilibrium when this pressure
reaches saturated vapor pressure.
 Since this depends upon molecular activity, which is a
function of temperature, the vapor pressure of a fluid
also depends on its temperature and increases with it.
 If the pressure above a liquid reaches the vapor pressure
of the liquid, boiling occurs; for example if the pressure
is reduced sufficiently boiling may occur at room
temperature.

22
Technical Studies
Engineering significance of vapor pressure
 In a closed hydraulic system, Ex. in pipelines or pumps, water vaporizes
rapidly in regions where the pressure drops below the vapor pressure.
 There will be local boiling and a cloud of vapor bubbles will form.
 This phenomenon is known as cavitations, and can cause serious
problems, since the flow of fluid can sweep this cloud of bubbles on
into an area of higher pressure where the bubbles will collapse
suddenly.
 If this should occur in contact with a solid surface, very serious
damage can result due to the very large force with which the liquid hits
the surface.
 Cavitations can affect the performance of hydraulic machinery such as
pumps, turbines and propellers, and the impact of collapsing bubbles
can cause local erosion of metal surface.
 Cavitations in a closed hydraulic system can be avoided by maintaining
the pressure above the vapor pressure everywhere in the system.

23
Technical Studies
Surface Tension
 Liquids possess the properties of cohesion and adhesion due to molecular attraction.
 Due to the property of cohesion, liquids can resist small tensile forces at the
interface between the liquid and air, known as surface tension, .
 Surface tension is defined as force per unit length, and its unit is N/m.
 The reason for the existence of this force arises from intermolecular attraction. In
the body of the liquid (Fig. 1.2a), a molecule is surrounded by other molecules and
intermolecular forces are symmetrical and in equilibrium.
 At the surface of the liquid (Fig. 1.2b), a molecule has this force acting only through
180.
 This imbalance forces means that the molecules at the surface tend to be drawn
together, and they act rather like a very thin membrane under tension.
 This causes a slight deformation at the surface of the liquid (the meniscus effect).
Figure 1.2: Surface Tension

24
Technical Studies
 A steel needle floating on water, the spherical shape of
dewdrops, and the rise or fall of liquid in capillary tubes is
the results of the surface tension.
 Surface tension is usually very small compared with other
forces in fluid flows (e.g. surface tension for water at 20C is
0.0728 N/m).
 Surface tension,, increases the pressure within a droplet of
liquid. The internal pressure, P, balancing the surface
tensional force of a spherical droplet of radius r, is given by
r
2
P

 (1.7)

25
Technical Studies
Capillarity
• The surface tension leads to the phenomenon known as capillarity
• where a column of liquid in a tube is supported in the absence of
an externally applied pressure.
• Rise or fall of a liquid in a capillary tube is caused by surface
tension and depends on the relative magnitude of cohesion of the
liquid and the adhesion of the liquid to the walls of the containing
vessels.
• Liquid rise in tubes if they wet a surface (adhesion > cohesion),
such as water, and fall in tubes that do not wet (cohesion >
adhesion), such as mercury.
• Capillarity is important when using tubes smaller than 10 mm (3/8
in.).
• For tube larger than 12 mm (1/2 in.) capillarity effects are
negligible.

26
Technical Studies
Figure 1.3
Capillary actions
r
cos
2
h



 (1.8)
where h = height of capillary rise (or depression)
 = surface tension
 = wetting (contact) angle
 = specific weight of liquid
r = radius of tube

27
Technical Studies
A reservoir of oil has a mass of 825 kg. The reservoir has a
volume of 0.917 m3
. Compute the density, specific weight,
and specific gravity of the oil.
Solution:
3
/
900
917
.
0
825
m
kg
m
volume
mass
oil 





3
oil m
/
N
8829
81
.
9
x
900
g
mg
volume
weight








9
.
0
1000
900
SG
C
4
@
w
oil
oil 





Example 1.2

28
Technical Studies
Water has a surface tension of 0.4 N/m. In a 3-mm diameter
vertical tube, if the liquid rises 6 mm above the liquid outside the
tube, calculate the wetting angle.
Solution
Capillary rise due to surface tension is given by;
r
cos
2
h




 = 83.7
4
.
0
x
2
006
.
0
x
0015
.
0
x
9810
2
rh
cos 





Example 1.3

29
Technical Studies
This chapter has summarized on the aspect below:
 Understanding of a fluid
 The differences between the behaviours of liquid and gases
 Newtonian and non-Newtonian fluid were identified
 Engineering unit of SI unit were discussed
 Fluid properties of density, specific weight, specific
gravity, viscosity and bulk modulus were outlined and
taken up.
 Discussion on the vapor pressure of the liquid
 Surface tension
 Capillarity phenomena
Summary

30
Technical Studies
Learning Materials
http://hyperphysics.phy-astr.gsu.edu/hbase/surten.html
http://www.cartage.org.lb/en/themes/Sciences/Physics/Mechanics/
FluidMechanics/FluidMechanics/NatureFluids/NatureFluids.htm

31
Technical Studies
Thank You

�ݺ�ߣ

EBVF4103 (Chapter 1) Fluid Mechanics for Civil Engineering.ppt

More Related Content

EBVF4103 (Chapter 1) Fluid Mechanics for Civil Engineering.ppt