Facilitation in Population Dynamics
1 like490 views
This presentation is the result of a student group project, during the Southern-Summer School on Mathematical-Biology, hold in S達o Paulo, January 2012, http://www.ictp-saifr.org/mathbio
Facilitation in Population Dynamics
- 1. Facilitation Systems Maria Luisa Jorge Marina Cenamo Salles Raquel A. F. Neves Sabastian Krieger Tom叩s Gallo Aquino Victoria Romeo Aznar Southern Summer Mathematical Biology IFT UNESP S達o Paulo January 2012
- 2. Presentation Outline Facilitation: definition and conceptual models Facilitation vs. competition in a gradient of environmental stress The simplest model A real case our simple model Results of the model Other possible (and more complicated) scenarios
- 3. Facilitation Species that positively affects another species, directly or indirectly.
- 4. Bruno et al. TREE
- 5. Bruno et al., 2003
- 6. Bruno et al., 2003
- 7. S - - -- A B -
- 8. Salt Marshes Physical conditions - Waterlogged soils - High soil salinities
- 9. Juncus gerardi More tolerant to high salinities acts on amelioration of physical conditions: shades the soil limits surface evaporation and accumulation of soil salts.
- 10. Iva frutescens Relatively intolerant to high soil salinities and waterlogged soil conditions
- 11. S - - -- B Juncus girardi - Iva frutescens
- 12. Bertness & Hacker, 1994
- 13. - A - B Change of Exponential Saturation Competition Effect of species A term of or logistic effect of salinity on (facilitator) population term of species B species A abundance growth population (facilitated) (facilitator) over time growth on species A (facilitator)
- 14. - - B A Change of Exponential Saturation Competition Effect of species B term of or logistic effect of salinity on (facilitated population term of species A species B abundance growth for population (facilitator) (facilitated) over time species B growth for on species species B B (facilitated)
- 15. - A B Final Initial Effect of salinity salinity abundance of species A on salinity
- 16. Reducing the number of parameters. Nondimensionalization dA A A =r A A1 b AB Ba A S 0 e dt KA dA' A ' =A ' 1A 'c AB B ' F A e dt dB B A =r B B1 b BA Aa B S 0 e dt KB dB ' A' =rB ' 1B ' c BA A ' F B e dt
- 17. Looking for fixed points Condition : dA A f , B f =0 A=0B=1 A F A e A / c AB A f Don't dt Af are that 撃 A f e =0 have dB B=0 B=1c BA A F B e A dt A f , B f =0 analytical solution !! =1c AB , =1c AB c BA , = F A F B c AB A f Ok, we look ... 1' A f =' e ' 1 ' 0 one A f 0 else don ' t A f 0 We can have: No solution One solution
- 18. How do fixed points varie with the parameters? ' 1 ' 0 one A f 0 A 0' e f two A f 0 else don' t A f 0 We can have: No solution Two solutions
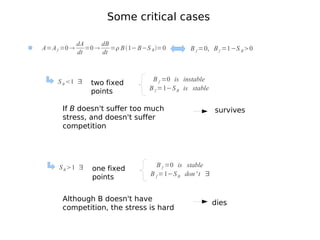
- 19. Some critical cases dA dB A=A f =0 =0 = B 1 BS B =0 B f =0, B f =1S B 0 dt dt S B 1 B f =0 is instable two fixed B f =1S B is stable points If B doesn't suffer too much survives stress, and doesn't suffer competition S B 1 B f =0 is stable one fixed B f =1S B don ' t points Although B doesn't have dies competition, the stress is hard
- 20. Some critical cases dB dA A B=B f =0 =0 = A1 AS A e =0 A f =0 and some A f 0 dt dt S A 1 A f =0 is instable A f 0,1 is stable If A doesn't suffer too much survives stress, and doesn't suffer competition A f =0 is stable S A 1 A f 0 don ' t if is small two A f 0,1 if is large. One stable , the other instable. dies With a high self-facilitation, A can survive if it has already large numbers survives
- 21. Species A Species B (with facilitation) Control for species B Salinity Temporal variation of both species and salinity when, at equilibrium, both co-exist.
- 22. Species A Species B (with facilitation) Control for species B Salinity Temporal variation of both species and salinity when, at equilibrium, both co-exist.
- 23. Species A Species B (with facilitation) Control for species B Salinity Temporal variation of both species and salinity when, at equilibrium, species B goes extinct.
- 24. Species A Species B (with facilitation) Control for species B Variation of abundance of both species with respect to salinity.
- 25. 多 Conditions of facilitation (treat - control > 0), competition (treat - control < 0) and no difference (treat - control = 0) with respect to a gradient of salinity and competition of B on A. Beta ab: 0.68
- 26. Conditions of facilitation (treat - control > 0), competition (treat - control < 0) and no difference (treat - control = 0) with respect to a gradient of salinity and competition of A on B. Beta ba: 1.2
- 27. Conditions of facilitation (treat - control < 0), competition (treat - control > 0) and no difference (treat - control = 0) with respect to a gradient of competition of A-B and B-A.
- 28. Real world example sustaining our model! Sp. A Stress level 0 Stress level 1 Stress level 2 Without B 900 300 300 With B 500 700 600 Sp. B Competition Facilitation Facilitation ( - -) (+ +) (+ +) Without A 750 200 150,2 Bertness & Hacker, 1994 With A 125 350 300
- 29. Other scenarios Auto- S facilitation of the facilitated - - Why does it -- - matter biologically? A B -
- 31. Forest Clearing
- 32. S - - --- A B -
- 33. This is what we had in the previous model: There are clear stable points
- 34. And this is what I ended up with: UNTRUE!
- 35. Another snapshot time: Population at time t = 4000
- 36. Population at time 400 Competitive exclusion
- 37. Plain competition: A is excluded
- 38. Population at time 400 Competitive exclusion Competition/ facilitation
- 39. B needs A, both are stable
- 40. Population at time 400 Competitive exclusion Successive Competition/ oscilations facilitation Ecological succession!
- 47. Looking for the bifurcation T = 400 T = 4000
- 48. Converges fast to stability Oscilating at very low frequency and high amplitude