Factors and multiples
- 1. Index Divisibility GCD and LCM Exercises Factors and Multiples Matem?ticas 2o E.S.O. a Alberto Pardo Milan?s e -
- 2. Index Divisibility GCD and LCM Exercises 1 Divisibility 2 GCD and LCM 3 Exercises Alberto Pardo Milan?s e Factors and Multiples
- 3. Index Divisibility GCD and LCM Exercises Divisibility Alberto Pardo Milan?s e Factors and Multiples
- 4. Index Divisibility GCD and LCM Exercises Divisibility Factors and multiples A factor of a number n, is a number d which divides n. Read ?? if and only if. d is a factor of n ?? d is a divisor of n ?? d divides n ?? n is divisible by d ?? n is a multiple of d. Examples: ?7 divides 14 ?? ?7 is a factor of 14 ?? 14 is divisible by ?7 ?? 14 is a multiple of ?7. Alberto Pardo Milan?s e Factors and Multiples
- 5. Index Divisibility GCD and LCM Exercises Divisibility Primes A prime number is a positive number that has only two positive factors 1 and the number itself (1 is not considered a prime number as it only has one positive factor). A number with more than two positive factors is called a composite number. Examples: 3 is a prime number because has only two positive fac- tors (1 and 3). 6 is a composite number because has four positive factors (1, 2, 3 and 6). Two numbers are relatively prime if they have no common positive divisors except 1. Example: 6 and 25 are relatively prime because the positive factors of 6 are 1, 2, 3, 6 and the positive factors of 25 are 1, 5, 25. Alberto Pardo Milan?s e Factors and Multiples
- 6. Index Divisibility GCD and LCM Exercises Divisibility Prime decomposition Prime decomposition is to ?nd the set of prime factors of an integer: To factorize a number you have to express the number as a product of its prime factors. To factorize negative numbers use also ?1. Examples: 45 3 15 3 ? 45 = 3 °§ 3 °§ 5 = 32 °§ 5. 5 5 1 25 5 5 5 ? ?25 = ?1 °§ 5 °§ 5 = ?1 °§ 52 . 1 Alberto Pardo Milan?s e Factors and Multiples
- 7. Index Divisibility GCD and LCM Exercises GCD and LCM Alberto Pardo Milan?s e Factors and Multiples
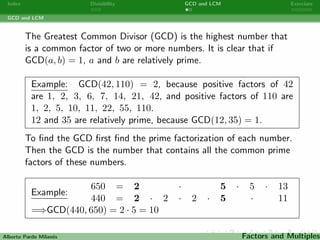
- 8. Index Divisibility GCD and LCM Exercises GCD and LCM The Greatest Common Divisor (GCD) is the highest number that is a common factor of two or more numbers. It is clear that if GCD(a, b) = 1, a and b are relatively prime. Example: GCD(42, 110) = 2, because positive factors of 42 are 1, 2, 3, 6, 7, 14, 21, 42, and positive factors of 110 are 1, 2, 5, 10, 11, 22, 55, 110. 12 and 35 are relatively prime, because GCD(12, 35) = 1. To ?nd the GCD ?rst ?nd the prime factorization of each number. Then the GCD is the number that contains all the common prime factors of these numbers. 650 = 2 °§ 5 °§ 5 °§ 13 Example: 440 = 2 °§ 2 °§ 2 °§ 5 °§ 11 =?GCD(440, 650) = 2 °§ 5 = 10 Alberto Pardo Milan?s e Factors and Multiples
- 9. Index Divisibility GCD and LCM Exercises GCD and LCM The Least Common Multiple (LCM) is the lowest positive number that is a common multiple of two or more numbers. Example: LCM(6, 9) = 18, because positive multiples of 6 are 6, 12, 18, 24, . . . and positive multiples of 9 are 9, 18, 27, . . . To ?nd the LCM ?rst ?nd the prime factorization of each number and write it in index form. Then the LCM will be the product of the each prime factors with the greatest power. 84 = 22 °§ 3 °§ 7 Example: 198 = 2 °§ 32 °§ 11 2772 = 22 °§ 32 °§ 7 °§ 11 =?LCM(84, 198) = 2772 Alberto Pardo Milan?s e Factors and Multiples
- 10. Index Divisibility GCD and LCM Exercises Exercises Alberto Pardo Milan?s e Factors and Multiples
- 11. Index Divisibility GCD and LCM Exercises Exercises Exercise 1 There are 28 students in our class and we want to divide them into groups with equal number of students. How many ways can the class be divided into groups? What are the results? Alberto Pardo Milan?s e Factors and Multiples
- 12. Index Divisibility GCD and LCM Exercises Exercises Exercise 2 Mary wants to serve hotdogs for 48 people. Sausages come in packages of 8 and hot dog buns come in packages of 12. She wants to have enough to serve everyone and have no leftovers. How many packages of sausages and hotdog buns should she purchase? Alberto Pardo Milan?s e Factors and Multiples
- 13. Index Divisibility GCD and LCM Exercises Exercises Exercise 3 Peter works in a ?orist shop. Today He has to make identical ?oral arrangements for a bridal party. He has 84 daisies, 66 lilies, and 30 orchids. He wants each arrangement to have the same number of each ?ower. What is the greatest number of arrangements that he can make if every ?ower is to be used? Alberto Pardo Milan?s e Factors and Multiples
- 14. Index Divisibility GCD and LCM Exercises Exercises Exercise 4 Samantha loves the sea. She has kayaking lessons every ?fth day and diving lessons every seventh day. If she had a kayaking lesson and a diving lesson on June the sixth, when will be the next date on which she has both kayaking and diving lessons? Alberto Pardo Milan?s e Factors and Multiples
- 15. Index Divisibility GCD and LCM Exercises Exercises Exercise 5 There are two ?ashing neon lights. One blinks every 4 seconds and the other blinks every 6 seconds. If they are turned on exactly at the same time, how many times will they blink at the same time in a minute? Alberto Pardo Milan?s e Factors and Multiples
- 16. Index Divisibility GCD and LCM Exercises Exercises Exercise 6 Peter sells books. He made 240e selling children°Øs books, 140e from cookbooks, and 280e from paperback books. He gets exactly the same bene?t from each book. What is the most that Peter could get for each book? How many books would Peter have sold then? Alberto Pardo Milan?s e Factors and Multiples