Final term 2012-2013 D1
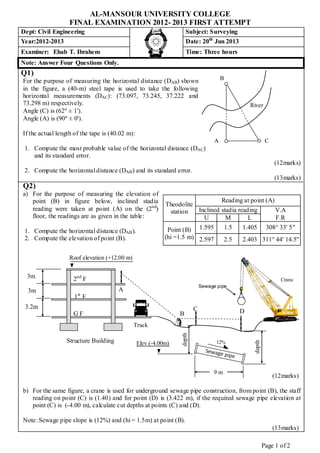
- 1. AL-MANSOUR UNIVERSITY COLLEGE FINAL EXAMINATION 2012- 2013 FIRST ATTEMPT Subject: SurveyingDept: Civil Engineering Date: 20th Jun 2013Year:2012-2013 Time: Three hoursExaminer: Ehab T. Ibrahem Note: Answer Four Questions Only. Q1) For the purpose of measuring the horizontal distance (DAB) shown in the figure, a (40-m) steel tape is used to take the following horizontal measurements (DAC): (73.097, 73.245, 37.222 and 73.298 m) respectively. Angle (C) is (62ĪŃ Ī└ 1'). Angle (A) is (90ĪŃ Ī└ 0'). If the actual length of the tape is (40.02 m): 1. Compute the most probable value of the horizontal distance (DAC) and its standard error. (12marks) 2. Compute the horizontal distance (DAB) and its standard error. (13marks) Q2) a) For the purpose of measuring the elevation of point (B) in figure below, inclined stadia reading were taken at point (A) on the (2nd ) floor, the readings are as given in the table: 1. Compute the horizontal distance (DAB). 2. Compute the elevation of point (B). (12marks) b) For the same figure, a crane is used for underground sewage pipe construction, from point (B), the staff reading on point (C) is (1.40) and for point (D) is (3.422 m), if the required sewage pipe elevation at point (C) is (-4.00 m), calculate cut depths at points (C) and (D). Note: Sewage pipe slope is (12%) and (hi = 1.5m) at point (B). (13marks) Theodolite station Reading at point (A) Inclined stadia reading V.A F.RU M L Point (B) (hi =1.5 m) 1.595 1.5 1.405 308ĪŃ 33' 5" 2.597 2.5 2.403 311ĪŃ 44' 14.5'' Page 1 of 2 A Structure Building Roof elevation (+12.00 m) B 3m 3m 3.2m Truck C DG F 1st F 2nd F Sewage pipe Crane 12%Elev (-4.00m) 9 m depth depth CA River B
- 2. Q3) a) For the purpose of measuring the area in the figure shown, a total station is used to measure the (UTM) system coordinates; the reading are as follows: Point E N Z 1 210.747 271.007 34.987 2 309.706 271.007 33.567 3 309.706 172.048 36.287 4 210.747 172.048 32.651 Calculate the area. (12marks) Q3 b) Calculate the volume of the earthwork between two cross-sections as shown in the table by prismoidal formula if the formation width of the road is (7 m): (13marks) Q4) For traverse (ABCDE) by using the angles to the right: 1. Tabulate and calculate the bearings and azimuths of all lines. (12mark) 2. If side (AE) is (50 m) long and point (A) coordinates are (200,200), find point (E) coordinates. (13mark) Q5) Two sides (AB) and (BC) are connected by a horizontal curve as shown in the figure, the degree of curvature of the curve is (8ĪŃ) calculated on a standard chord of (100m). If azimuth of (AB) is (50ĪŃ) and (BC) is (140?), length of (BC) is (950 m), station of (A) is (10+32.62) and of (B) is (25+32.62), the coordinate of point (A) is (2000, 2000), calculate: 1. Stations of (PC, PT, and point C). 2. The coordinates of (PC). (12marks) 3. If the station of point (G) is (19+00), find point (G) coordinates. Q4) (13 marks) Station Cross-section Area L C.L R Cut (m2 ) Fill (m2 ) 62+60 f 2.5 7.34 f 3.3 0.0 f 3.8 8.7 0.0 ? 62+90 f 0.7 6.05 f 1.4 0.0 f 1.4 6.3 0.0 ? Page 2 of 2 1 st point 2 nd point 3 rd point 4 th point Area?
- 4. 4 *9" ' 4<./-1' >:z!_l!! !il. trn, ./ _ / f t; g ,fu/ K : f.L"-rH/"c/,-?-k'._rI' D^. -- +l=.n .l-""JJ tiu {l ,it ;o", 71. Z+s X,= 13,t)1 r't t7' ztz ---J'l'+ etr'v lz> J) zt1 .^'t'ty^ 7l zyv 1et<te-t etr'v 7"t,,;ir ,'it'xr=13 7,,^ o, 40 4 0,02 11. a17 )<, F=. X 'r1^'-r)7 /- fa,r/.tvl / 13 (trof A *n. ^r.,^ 4@ 7 241 // F*N =r U- irJ '2- -- .l ) tt th4rtv't- tt -1- ,^ "1t. L)1 = A.o3?_ : O, OJ 2rL'*a,oz)+o5rtl d,oZtToS L J).{t : 7- .' (. I / 2 - )+.t,dlqT;l ptto, Fxi A. ( tr trn)'-= (t<n c)z(t Ac)' *( A( r",'c)' ( :-',5 I , .----. = (,n tz)'( d'oe)' *f+t'241 * t""(/.D.J' ( k " k ) fi = r.ot2a *-(1t.241r'+.s77)' ( y +a,s tn-D) =!tt,t,rr.u4 l 6,to4t1_--_ -JJ X t u,"dot 6) r]
- 5. (rlq)Q- (*) R*.- R.*, rl . {-.-.* ^l r'', S, r 7, ) ./. A,4 t4 S D Wl; Jt'D V.,=])1" ,:-ta 't4 14. s), 'Y (4t )or- D --tou (u-r1 c"L (v't .,, P'( i'5ts - r"4's) c's " , r L ,o'Lz'51-l'24attes a/ t2 5 7t) //-1, I (tt 2 ( D^ ^ = z 5- /.5 _-___------;-; AB- -- "'' -Lqn(4t 44 'f,)-t*(s8as 7 '7?/ p ."/./. t 3. 47L= 6.1)3 +t,5_ 1,281 el,"(D)=//4,'+^i- -c4 >lo -*f i ,or 1* -'----^--'-1)L.-i^- t ^ /a" I t( 5= r'"8 l L-- ""=- 0t (h) -+ 3n^+11 *'l: o'tt,s-r-r 5't'4 E[cv ltt' '= o4Jl ]el.v l'/- = a.-"r.. "/ n,p, ) ) ĆS, --h (r'tl ' / ,,, 4 -7''t 14 (i y'l (t) J..- c,t -{+ q)
- 6. (ztq) L -o <-", -k'- ' /-^ l-9 2V,,(<' TJ h. r" l^ "L(V lv r- e'. , -<- r- {Ć i,l '$t(, 1 0 q, , /' vr .r -s --t o , - , ,, ,^ NU L T s ^rTz .l
- 7. .+ <_i ; 1 tf ' "nn l/-x /, oDs o ot C'r' ; I lo t f! F"t Or ..1 otf?I f 'at!n If o 14 a Fr *rQ tfl t trr (r) ar) o fr) Or o t 5 l c s rY .S:n
- 8. /'lv'7sr 118 tsy q +17. tf ?4> ) Le(,0' 4 " 4tr Qt /1) 7 /4 l, c:" 4 z+1, o <: 1 t4z' '4t t-tz, - 4 8 L+t, 2o4 (")q % .-1 / 5+ttt, 7711 l-r1v 284 )o ll -[;ttz34']o ll l'ror /13.1i J I - 2- L;+1(. 4 L I L A..-.--' n9q 72, 41 r 9l t tz'4q + 9( zf 8'6.o +'>l zt 8.6" z 4 orTL' t5 t7 5 b5. +6 A 1lqz. tr8 ^" -- ----K's- /l' qrl I o- v^ l- a^ trJ ,SY 2- / 112, X til,14+ 3"1'7"4 7/o,74V Je7. |'( 7o1' A-6 21t->. ] 4 4 z1o ]44 t,/,_"
- 9. (h Yr) >1 1 6z -rlo {z-7) A,9').---.--.-4 Xrl 41(,r/ /l. /// - v-)/ lj'n') 4-4 t Lr,. r,, / h $/il Lqq- --..'..-...-.|-T'tnrl;2 /2Lr {94a" ,60 "b,4 l,L' I'lt1 /,:- I'of t# lt x 11 M4/ 6z+fo Ll t l,/l / 't, l t,A {-+4' '/,b') 12,12/ 35 [t t.t z, ,'/ ( 2.s1") (.t,5t") ,7 x x -+r+ (tt *--'un r -.i /- $.? )( -" , 712 + AL'c'l t+-421 -'/ f / ^t. I I G5.7 )^tt rtln=ll -2.5 LI 11 ) .-' A F-I (+.ta,-tt1 6i,+t1 ( 1,+ r-3'6) =- 74,q gt v < ?U,n 4 A^.r 4,) ".{l = *xs.attt4(tttaa) ttL'11)) = 11, Lt-+L' I 7 4 l * -7-5n-, b.T o, --.4,-r4 -4-5 q I
- 10. ,< ?.C ..-1 < 1"/ /2 .- {.{.1' -1. t t4 t nL/ b -2,4 _2 ,+5 *t.( 6 (( I /44 (7 s, A.() Btj 47 -t( 4.t (B) -t. t ,,- )') /,) , -/,b5 -t., (/,) ae )a p-,',f I I L t 4 ' I ) J f/ _2,zsa -/rz: /tr_2.> to=[ -'(1 -' i 7r/ (a, -z.zs) + /+-/z> :V zst -'i /' +10) L4 P';+t (6../ -/.4/ 1. I 3,5 /+r'411 t,ot-''a lJ / ^'a,n'1 l-t'os, -o'') , ^" / (ot */'/l) al -1.4 ^ -l "tl 1,A=Li -r,, r-'o) 1/, = L4'L4 /,= [2.]L y I t 7 4 I I / / --t,4I .- /.4 _o,4 / @Ći
- 11. dpr 5'1dra. AI /'.1. a 7,'h,uf/ A^ I r n O Azrhvtl AlJ - rty - +/$u ....> ' A*.BA |ot'----_--.'-i) 4rqtl< +('1o ? /. ) b" C/,) .5, at" A9 Bc cn ac ĆA lL5 1/ ,4 el '1 I fio 7-4 4 t, hon'i'5 oF s9s k sbq,e [l +1 Ć M'Jo w S L4'^ b tt+ AZ6 = 244 +lIo -7A * - 36u .--.-> A4.n e 61 --5i / t t2, t )./ ='-- -.-> A!fr * q of{-= A1 rQ lAt/ e,_t) - ,r Y < t?(D t38 -- 401* 3 6o ltt AL.c P 17 + tta llJ Ar ?L1' e. '-.,/r - -l , | ". - t/ r- 5f-r-LUb --> ^ rt r. hL.OE LV' /go 4b" --Y:- u+ r44 - ""t--al- ------:-11, +:Jt - a= I 4,$ /t,, (E-z)$0. S4a' <fB n"t4tl 'n,,..[ qllf t7tr + lA6)t .--:1p-;.'. < (d9, qJ t -- A7fr = AL'AE o bt = [4+/t' tz, "y v
- 12. rI'IP I i (ah).r (t4(b) /Fj : Ly F,uGd = tr't'/^'/o FrL(rte)' (A a't--#!^ G (r, t/ .-oY$..) E I a(4 ' X(o,r tne ( t-/ Lf. / /'2 /, z1 - r r- - 'uP'r '/ (wtW) 5i.^ A Z 46) / r t)'- t--4 4,177 lvl Jrt t b'r/^ ,./. r A . / (Ll = 'L&'t )", -/// x (-)( 64) :,LI, l' 1 l{ f t ,- E ( 244 11 ,'z'1' q'6 o a
- 13. ( t ,-/l z r.rlt." = ' L"- 40".- 7o'= 1o' fA) - s^( '( = 1 I L/ - z- '/ z, ' T= <*^H =at/>. /71u L. Ć. Qr^r( - lItT;TtW =-4r/ r,t' (i;,ff)=r,t4,1q1 a A d j tl21 n -rrV ^ r- .<- ,0" ={<7Ex>u) {?t1lt6'lq+'m 3,912 -= R Q, , ^1 rf ..? $j, 54A =1,1;t"'+ !!', r ia=) . 0,= I 4l /o,l d''1, ' t:4 4 trt rt' C1= zR si't O' ,' a .:') C,- z /1tt l1+ t,', ( L 4r /t t ' = / 1,{.'1X4 .},1 :-'oo.J.-'.r^:1 X PC = looo af l8t6'127^ t0''u) Y PC. Zuu' + I- A{7'8"t }| * il ffiQ,tz-r5d")n'-l 2' (,00,+nt l 6b'-1114 ;' t ( sl' 2". . J-r z,.t t/.1 J1y' /'- / r,' ',.1. '1 G = zto),tlJ + /6t 111 L ")L )) ta C z+34,2;4, Llo/,36 1j (e ,' (fo > ( 5o y,o i)' b,,1')= 7734,2rI a zLa|.)g rn zs+12,1,4 1$+ rL'4t) PC - FI --f= z5 j),62 -1t6.ttl ftq' = /gtL,4z7.n =51'o11[,4[t)t___--..,,r "i' I il'r25'uu- p;irq7v-77=l t'l 4, t'l q ?c :- 5l c Gst"- )lttrt - /tr// 42 t) {- .1 <,t t < zt.A) -1-1Ju = sl 62',-l)v='-")-'" | --i---- ?4 92.6L.----.''-| " .,1 a1 At- | 5 4 1- D.-' " " I I l1g,+ ft'4tt l_:_------6 "). 2b01,427 )-' ')= 25t),'611 ^ 't 'l4 +$L'bL l0i It -2ltu, 41'A,B el'w 00atL0 0c



![4
*9" '
4<./-1' >:z!_l!! !il. trn, ./ _ / f t; g ,fu/ K : f.L"-rH/"c/,-?-k'._rI'
D^. -- +l=.n .l-""JJ tiu {l ,it ;o",
71. Z+s X,= 13,t)1 r't
t7' ztz ---J'l'+ etr'v lz> J) zt1 .^'t'ty^
7l zyv
1et<te-t etr'v
7"t,,;ir ,'it'xr=13
7,,^ o,
40
4 0,02
11. a17
)<,
F=. X 'r1^'-r)7
/- fa,r/.tvl /
13
(trof A *n. ^r.,^
4@
7
241
//
F*N =r U-
irJ '2- --
.l
)
tt th4rtv't- tt -1-
,^ "1t. L)1
= A.o3?_
: O, OJ
2rL'*a,oz)+o5rtl
d,oZtToS
L
J).{t
:
7-
.' (. I /
2 - )+.t,dlqT;l ptto,
Fxi
A.
( tr trn)'-= (t<n c)z(t Ac)' *( A( r",'c)' ( :-',5 I , .----.
= (,n tz)'( d'oe)' *f+t'241 * t""(/.D.J' (
k " k ) fi
= r.ot2a *-(1t.241r'+.s77)' ( y +a,s tn-D)
=!tt,t,rr.u4 l
6,to4t1_--_
-JJ
X
t u,"dot
6)
r]](https://image.slidesharecdn.com/90331bff-544d-4e40-af32-6dcc061c0663-141216115837-conversion-gate01/85/Final-term-2012-2013-D1-4-320.jpg)
![(rlq)Q- (*)
R*.- R.*,
rl .
{-.-.* ^l r'', S, r
7, ) ./.
A,4 t4 S
D
Wl; Jt'D
V.,=])1"
,:-ta
't4 14. s),
'Y
(4t
)or-
D --tou (u-r1 c"L (v't .,,
P'( i'5ts - r"4's) c's "
, r L
,o'Lz'51-l'24attes
a/
t2
5
7t)
//-1,
I (tt
2
( D^
^ = z 5- /.5 _-___------;-; AB- --
"'' -Lqn(4t 44
'f,)-t*(s8as
7 '7?/ p
."/./. t 3. 47L= 6.1)3 +t,5_ 1,281
el,"(D)=//4,'+^i- -c4
>lo -*f
i ,or
1*
-'----^--'-1)L.-i^- t
^ /a" I
t( 5= r'"8 l
L-- ""=-
0t (h) -+
3n^+11 *'l: o'tt,s-r-r 5't'4
E[cv ltt'
'= o4Jl ]el.v l'/- = a.-"r..
"/ n,p, )
) ĆS, --h (r'tl ' / ,,,
4 -7''t 14 (i y'l (t)
J..-
c,t -{+ q)](https://image.slidesharecdn.com/90331bff-544d-4e40-af32-6dcc061c0663-141216115837-conversion-gate01/85/Final-term-2012-2013-D1-5-320.jpg)


![/'lv'7sr 118 tsy
q +17. tf ?4>
)
Le(,0' 4
" 4tr
Qt /1)
7
/4 l, c:" 4
z+1, o <: 1
t4z' '4t
t-tz, - 4 8
L+t, 2o4
(")q
%
.-1
/ 5+ttt, 7711
l-r1v 284 )o ll
-[;ttz34']o ll
l'ror
/13.1i J I
- 2- L;+1(. 4 L
I
L A..-.--'
n9q 72, 41
r 9l t tz'4q
+ 9( zf 8'6.o
+'>l zt 8.6"
z 4 orTL' t5
t7 5 b5. +6
A 1lqz. tr8
^"
-- ----K's- /l'
qrl
I o- v^
l- a^
trJ
,SY
2-
/ 112,
X
til,14+ 3"1'7"4
7/o,74V
Je7. |'(
7o1' A-6
21t->. ] 4 4
z1o ]44
t,/,_"](https://image.slidesharecdn.com/90331bff-544d-4e40-af32-6dcc061c0663-141216115837-conversion-gate01/85/Final-term-2012-2013-D1-8-320.jpg)

![,<
?.C
..-1 <
1"/
/2
.- {.{.1'
-1.
t
t4
t
nL/
b
-2,4
_2 ,+5
*t.(
6
((
I /44
(7 s, A.()
Btj
47 -t( 4.t
(B)
-t. t
,,-
)')
/,)
,
-/,b5
-t.,
(/,)
ae )a
p-,',f
I
I
L
t
4
' I
)
J
f/ _2,zsa -/rz: /tr_2.>
to=[ -'(1 -'
i
7r/
(a, -z.zs)
+ /+-/z> :V zst
-'i /' +10)
L4
P';+t
(6../ -/.4/
1. I
3,5 /+r'411
t,ot-''a lJ
/
^'a,n'1
l-t'os, -o'') , ^"
/ (ot */'/l)
al -1.4
^
-l "tl
1,A=Li -r,, r-'o)
1/, = L4'L4
/,= [2.]L y
I
t
7
4
I
I
/
/
--t,4I
.- /.4
_o,4
/
@Ći](https://image.slidesharecdn.com/90331bff-544d-4e40-af32-6dcc061c0663-141216115837-conversion-gate01/85/Final-term-2012-2013-D1-10-320.jpg)


