Lecture5 Chapter1- Binary Codes.pdf
0 likes214 views
This document discusses various binary codes used to represent decimal numbers in digital systems, including: - Binary coded decimal (BCD) code, which represents each decimal digit with a unique 4-bit code. BCD addition and arithmetic are explained. - Gray code, a cyclic code where only one bit changes between adjacent numbers. Gray code is used in applications like shaft encoders due to this property. Conversion between binary and Gray code is covered. - Other decimal codes like excess-3 code and weighted codes are mentioned for representing decimal numbers in binary. Arithmetic operations in BCD using 10's complement for signed numbers is also summarized.
1 of 20
Download to read offline















Ad
Recommended
micro processor and its architecture unit1.pptx



micro processor and its architecture unit1.pptxAjayKumar626901
?
A microprocessor is a compact, integrated circuit that serves as the brain of a computer system, executing instructions and managing the flow of data through various operations. It forms the core of a microcontroller, which in turn, powers a wide range of devices from computers and smartphones to household appliances and automotive systems. The microprocessor operates based on a set of instructions stored in its memory, interpreting and executing tasks as directed by software applications.
### Architecture of a Microprocessor
The architecture of a microprocessor defines its structure, functionality, and operational behavior. It encompasses several key components, including the Arithmetic Logic Unit (ALU), Control Unit (CU), Registers, and various buses (data, address, and control buses).
1. **Arithmetic Logic Unit (ALU):**
The ALU is the component responsible for performing all arithmetic and logical operations. This includes basic arithmetic operations like addition and subtraction, as well as logical operations like AND, OR, and NOT. The ALU is crucial for executing instruction sets that involve numerical data manipulation.
2. **Control Unit (CU):**
The Control Unit orchestrates the operations of the microprocessor by directing the flow of data between the ALU, registers, and memory. It interprets the instructions from the memory and converts them into signals that control other parts of the microprocessor. The CU ensures that the instructions are executed in the correct sequence and timing.
3. **Registers:**
Registers are small, fast storage locations within the microprocessor used to hold data temporarily during execution. They facilitate quick access to data and instructions that are frequently used. Common types of registers include the Program Counter (PC), which tracks the address of the next instruction to be executed, and the Accumulator, which is used for arithmetic operations.
4. **Buses:**
Buses are communication pathways that connect different parts of the microprocessor and allow data to be transferred between them. The Data Bus carries actual data being processed, the Address Bus carries the addresses of where data is stored or retrieved from, and the Control Bus carries control signals that manage the actions of the microprocessor.
### Microprocessor Design Paradigms
Microprocessors can be categorized based on their architecture design paradigms, mainly as CISC (Complex Instruction Set Computing) and RISC (Reduced Instruction Set Computing).
1. **CISC (Complex Instruction Set Computing):**
CISC processors have a large set of instructions, allowing them to execute complex operations with a single instruction. This design aims to minimize the number of instructions per program, sacrificing simplicity of instruction set for more powerful single instructions. Examples include Intel¡¯s x86 architecture.
2. **RISC (Reduced Instruction Set Computing):**
RISC processors utilize a smaller, highly optimized set Digital Logic BCA TU Chapter 2.2



Digital Logic BCA TU Chapter 2.2ISMT College
?
BCD, ASCII, Excess-3, Gray Code, Error Detecting & Correcting Code, Conversion. Digital Logic BCA TU Chapter 2.2BCD,GRAY and EXCESS 3 codes



BCD,GRAY and EXCESS 3 codesstudent
?
Weighted codes assign a positional weight or value to each digit, where the sum of the digit values multiplied by their weights represents the number. Non-weighted codes do not assign positional weights. BCD is a weighted 4-bit code that represents the decimal digits 0-9. It uses weights of 24, 23, 22, 21 from most to least significant bit. The Gray code is a non-weighted code where each number differs from the previous by one bit. Excess-3 code is a non-weighted 4-bit BCD code where 3 is added to each decimal digit before conversion to BCD.Digital Logic-Lecture19.pptx



Digital Logic-Lecture19.pptxASVKVinayak
?
This document discusses code conversion between different digital systems. It provides an example of converting between binary coded decimal (BCD) code and excess-3 code using a combinational logic circuit. The circuit is designed using a truth table to map the input and output bits. Logic gates are then used to implement the mapping and produce the output bit combinations specified by the target code. Another example provided is the design of a circuit to convert a 4-bit binary number to a 4-bit Gray code.Code conversion r006



Code conversion r006arunachalamr16
?
This document discusses different coding systems used to represent numeric and alphanumeric characters in computers. It provides details on Binary Coded Decimal (BCD), American Standard Code for Information Interchange (ASCII), Extended Binary Coded Decimal Interchange Code (EBCDIC), Gray code, and Excess-3 code. It also gives examples and step-by-step processes for converting between binary, BCD, Excess-3, and decimal number systems.12.Representation of signed binary numbers. Binary codes - BCD code, Gray co...



12.Representation of signed binary numbers. Binary codes - BCD code, Gray co...JatinJatin30
?
This document discusses representation of binary numbers and binary codes. It covers:
- Unsigned and signed binary number representation including sign-magnitude and 1's/2's complement forms.
- Binary coded decimal (BCD) which assigns a 4-bit code to each decimal digit from 0-9. BCD is useful for digital displays that show decimal numbers.
- Excess-3 code which adds 3 to each decimal digit before converting to binary.
- Gray code which increments only one bit at a time to avoid errors during counting. Conversion between binary and gray code is covered.numbering system binary and decimal hex octal



numbering system binary and decimal hex octalnoor300491
?
Hexadecimal is a base-16 number system used to compactly represent binary numbers. It uses 16 symbols - 0-9 and A-F. Counting proceeds from F to 10, then 20, etc. Binary numbers can be converted to and from hexadecimal by grouping bits into 4-bit blocks and replacing with the hex symbol. Decimal can also be converted to and from hexadecimal using multiplication/division by 16 or remainders. Hexadecimal addition follows decimal addition rules, carrying when sums exceed 15. Octal is base-8 and uses 0-7 symbols, with binary conversion replacing octal digits with 3-bit groups. Binary Coded Decimal represents each decimal digit with 4 bits for easy decimal interfacing. Gray code changesNumber codes students



Number codes studentsSchool of Design Engineering Fashion & Technology (DEFT), University of Wales, Newport
?
The following presentation is a part of the level 4 module -- Digital Logic and Signal Principles. This resources is a part of the 2009/2010 Engineering (foundation degree, BEng and HN) courses from University of Wales Newport (course codes H101, H691, H620, HH37 and 001H). This resource is a part of the core modules for the full time 1st year undergraduate programme.
The BEng & Foundation Degrees and HNC/D in Engineering are designed to meet the needs of employers by placing the emphasis on the theoretical, practical and vocational aspects of engineering within the workplace and beyond. Engineering is becoming more high profile, and therefore more in demand as a skill set, in today¡¯s high-tech world. This course has been designed to provide you with knowledge, skills and practical experience encountered in everyday engineering environments.
Codes r005



Codes r005arunachalamr16
?
1. The document discusses different types of codes used to represent digital data including weighted, non-weighted, alphanumeric, error detection, error correction, and binary codes.
2. It describes various binary codes like BCD, Gray, EBCDIC, and ASCII codes explaining how they represent numeric and alphanumeric data.
3. Specific codes discussed in detail include BCD, excess-3, Gray, and ASCII codes explaining their binary representations of decimal numbers and characters.Student IP Addressing Tutorial.ppt



Student IP Addressing Tutorial.pptjohnBronson6
?
This document provides an overview of IP addressing and subnetting. It begins with an introduction to binary to decimal conversion and how binary weighting is used to represent numeric values. It then discusses IP addressing fundamentals including address classes, private addresses, and how subnet masks are used to partition addresses into network and host portions. The document explains how subnetting allows large networks to be divided into smaller sub-networks through the use of custom subnet masks. Key concepts covered include default subnet masks, finding the network address through binary AND operations, and limitations of classful addressing that subnetting addresses.5_2019_01_03!03_33_00_PM.pptx



5_2019_01_03!03_33_00_PM.pptxAbijahRoseline1
?
This document discusses different binary coded decimal (BCD) codes and how to convert between binary and BCD representations. It provides examples of converting decimal numbers to their BCD equivalents using 8421 BCD code. Other BCD codes discussed include 4221 and 5421 codes. Conversion between binary and excess-3 code is also explained with an example. The document concludes with an overview of Gray code, including how to convert between binary and Gray representations using an additive process.Chapter 5: Cominational Logic with MSI and LSI



Chapter 5: Cominational Logic with MSI and LSIEr. Nawaraj Bhandari
?
This document discusses combinational logic circuits using MSI (Medium Scale Integration) and LSI (Large Scale Integration) components. It covers various MSI components like adders, decoders, encoders, multiplexers that are used as basic building blocks. Specific circuits discussed include 4-bit parallel adder, BCD adder, magnitude comparator, priority encoder, octal to binary encoder, decoder and their applications in implementing Boolean functions using multiplexers.Digital Design Session 2



Digital Design Session 2International Institute of Information Technology - Bangalore
?
Binary codes and their classifications
Weighted codes
Binary Coded Decimal (BCD)
Various BCD formats
Unsigned BCD arithmetic
Packed and unpacked BCD
Non-weighted codes
Excess-3
Gray code
Optical Encoder Example using Gray CodeUnit 1 data representation and computer arithmetic



Unit 1 data representation and computer arithmeticAmrutaMehata
?
This document provides an overview of a computer organization course for first year BCA students. It covers topics like introduction to digital logic design, number systems, binary arithmetic operations, binary coded decimal, and non-weighted and weighted binary codes. The key concepts discussed include binary, octal, hexadecimal number conversions; addition, subtraction, multiplication and division in binary; 1's complement, 2's complement representations; and BCD and excess-3 coding schemes.Digital electronics



Digital electronicsSukriti Dhang
?
Digital electronics
Lesson 1: Number System and Representation
Content: Decimal, Binary, Octal, Hexadecimal,
1¡®s and 2¡®s complements,
Codes ¨C Binary, BCD, Excess 3, Gray, Alphanumeric codes
Unit-1 (DLD) Lecture 2.pptx



Unit-1 (DLD) Lecture 2.pptxBunnyYadav7
?
Binary codes can be weighted or unweighted. Weighted codes assign decimal weights to bits, like the 8-4-2-1 code. BCD is a weighted code representing each decimal digit with 4 bits. Gray code has only one bit changing between adjacent codes, making it useful for encoding shaft rotations. 1's complement inverts all bits, 2's complement adds 1 to the 1's complement. These complements allow subtraction to be performed using addition by adding the complement.Bcd



BcdTalha Fazal
?
This document discusses binary coded decimal (BCD). It defines BCD as a numerical code that assigns a 4-bit binary code to each decimal digit from 0 to 9. Numbers larger than 9 are expressed digit by digit in BCD. BCD is used because it is easy to encode/decode decimals and useful for digital systems that display decimal outputs. The document also describes how addition and subtraction are performed in BCD through binary addition rules and handling carries.Digital Electronics Codes.pdf



Digital Electronics Codes.pdfPrasenjitKumarDas2
?
This document provides information about binary coded decimal (BCD). It begins by defining BCD as a binary encoding where each decimal digit is represented by a fixed number of bits. BCD was commonly used for displaying alphanumeric characters but is now mainly used in real-time clocks to store time in a BCD format. Each section then explains how BCD represents decimal numbers using 4 bits per digit according to positional weights, and provides examples of converting decimal numbers to BCD and vice versa. The document also covers related topics like ASCII and EBCDIC codes.Binary to gray converter using xor 



Binary to gray converter using xor DINESH DEVIREDDY
?
This document describes a digital electronics project to build binary to gray code and gray to binary converters using XOR gates. A group of 4 students submitted the project to their professor. The introduction defines binary and gray codes. Gray codes differ from binary in that only one bit changes between adjacent numbers. The document explains how to calculate gray codes by XORing binary bits. It then describes the circuit built using an IC7486 XOR gate, resistors, LEDs, buttons and a power supply. The circuit converts between gray and binary codes. Finally, the document discusses the uses of these converters and concludes that the project was successfully implemented and tested.DEL LAB MANUAL



DEL LAB MANUALPUNE VIDYARTHI GRIHA'S COLLEGE OF ENGINEERING, NASHIK
?
The document provides information about a digital electronics lab manual for Pune Vidyarthi Griha's College of Engineering in Nashik, India. It includes an index of assignments for topics like adders, code converters, flip flops, counters, and VHDL. The assignments are divided into groups A through D. The second assignment provides details on designing code converters for binary to gray, gray to binary, BCD to excess-3, and excess-3 to BCD. It describes the various code systems and provides truth tables and logic diagrams for realizing the four code conversions.Digital Electronics- Number systems & codes 



Digital Electronics- Number systems & codes VandanaPagar1
?
This document covers number systems including decimal, binary, hexadecimal and their representations. It discusses how to convert between different number bases including binary to decimal and hexadecimal to decimal. Binary operations like addition, subtraction and codes like binary coded decimal are explained. Non-weighted codes such as gray code are also introduced. Reference books on digital electronics and number systems are provided.Lecture6 Chapter1- ASCII Code, Error Detection and Correction Codes, and Bina...



Lecture6 Chapter1- ASCII Code, Error Detection and Correction Codes, and Bina...UmerKhan147799
?
This document discusses digital systems and binary numbers. It covers ASCII code, error detection and correction codes, and binary logic. Specifically, it describes the ASCII character code and tables, parity codes for error detection, binary storage in registers and memory, basic logic operations and gates, truth tables, and timing diagrams for digital circuits. The overall objectives are to study ASCII code, error detection techniques, and binary logic concepts.Lecture6 Chapter4- Design Magnitude Comparator Circuit, Introduction to Decod...



Lecture6 Chapter4- Design Magnitude Comparator Circuit, Introduction to Decod...UmerKhan147799
?
The document discusses magnitude comparators and decoders. It begins by describing how a magnitude comparator compares two numbers and determines if one is greater than, less than, or equal to the other. It then presents an algorithmic approach to designing a comparator circuit using exclusive NOR gates and AND gates. The document also introduces decoders, explaining that they convert binary inputs to unique outputs. Common applications of decoders include memory addressing, code conversion, demultiplexing, and generating timing signals.Ad
More Related Content
Similar to Lecture5 Chapter1- Binary Codes.pdf (20)
Number codes students



Number codes studentsSchool of Design Engineering Fashion & Technology (DEFT), University of Wales, Newport
?
The following presentation is a part of the level 4 module -- Digital Logic and Signal Principles. This resources is a part of the 2009/2010 Engineering (foundation degree, BEng and HN) courses from University of Wales Newport (course codes H101, H691, H620, HH37 and 001H). This resource is a part of the core modules for the full time 1st year undergraduate programme.
The BEng & Foundation Degrees and HNC/D in Engineering are designed to meet the needs of employers by placing the emphasis on the theoretical, practical and vocational aspects of engineering within the workplace and beyond. Engineering is becoming more high profile, and therefore more in demand as a skill set, in today¡¯s high-tech world. This course has been designed to provide you with knowledge, skills and practical experience encountered in everyday engineering environments.
Codes r005



Codes r005arunachalamr16
?
1. The document discusses different types of codes used to represent digital data including weighted, non-weighted, alphanumeric, error detection, error correction, and binary codes.
2. It describes various binary codes like BCD, Gray, EBCDIC, and ASCII codes explaining how they represent numeric and alphanumeric data.
3. Specific codes discussed in detail include BCD, excess-3, Gray, and ASCII codes explaining their binary representations of decimal numbers and characters.Student IP Addressing Tutorial.ppt



Student IP Addressing Tutorial.pptjohnBronson6
?
This document provides an overview of IP addressing and subnetting. It begins with an introduction to binary to decimal conversion and how binary weighting is used to represent numeric values. It then discusses IP addressing fundamentals including address classes, private addresses, and how subnet masks are used to partition addresses into network and host portions. The document explains how subnetting allows large networks to be divided into smaller sub-networks through the use of custom subnet masks. Key concepts covered include default subnet masks, finding the network address through binary AND operations, and limitations of classful addressing that subnetting addresses.5_2019_01_03!03_33_00_PM.pptx



5_2019_01_03!03_33_00_PM.pptxAbijahRoseline1
?
This document discusses different binary coded decimal (BCD) codes and how to convert between binary and BCD representations. It provides examples of converting decimal numbers to their BCD equivalents using 8421 BCD code. Other BCD codes discussed include 4221 and 5421 codes. Conversion between binary and excess-3 code is also explained with an example. The document concludes with an overview of Gray code, including how to convert between binary and Gray representations using an additive process.Chapter 5: Cominational Logic with MSI and LSI



Chapter 5: Cominational Logic with MSI and LSIEr. Nawaraj Bhandari
?
This document discusses combinational logic circuits using MSI (Medium Scale Integration) and LSI (Large Scale Integration) components. It covers various MSI components like adders, decoders, encoders, multiplexers that are used as basic building blocks. Specific circuits discussed include 4-bit parallel adder, BCD adder, magnitude comparator, priority encoder, octal to binary encoder, decoder and their applications in implementing Boolean functions using multiplexers.Digital Design Session 2



Digital Design Session 2International Institute of Information Technology - Bangalore
?
Binary codes and their classifications
Weighted codes
Binary Coded Decimal (BCD)
Various BCD formats
Unsigned BCD arithmetic
Packed and unpacked BCD
Non-weighted codes
Excess-3
Gray code
Optical Encoder Example using Gray CodeUnit 1 data representation and computer arithmetic



Unit 1 data representation and computer arithmeticAmrutaMehata
?
This document provides an overview of a computer organization course for first year BCA students. It covers topics like introduction to digital logic design, number systems, binary arithmetic operations, binary coded decimal, and non-weighted and weighted binary codes. The key concepts discussed include binary, octal, hexadecimal number conversions; addition, subtraction, multiplication and division in binary; 1's complement, 2's complement representations; and BCD and excess-3 coding schemes.Digital electronics



Digital electronicsSukriti Dhang
?
Digital electronics
Lesson 1: Number System and Representation
Content: Decimal, Binary, Octal, Hexadecimal,
1¡®s and 2¡®s complements,
Codes ¨C Binary, BCD, Excess 3, Gray, Alphanumeric codes
Unit-1 (DLD) Lecture 2.pptx



Unit-1 (DLD) Lecture 2.pptxBunnyYadav7
?
Binary codes can be weighted or unweighted. Weighted codes assign decimal weights to bits, like the 8-4-2-1 code. BCD is a weighted code representing each decimal digit with 4 bits. Gray code has only one bit changing between adjacent codes, making it useful for encoding shaft rotations. 1's complement inverts all bits, 2's complement adds 1 to the 1's complement. These complements allow subtraction to be performed using addition by adding the complement.Bcd



BcdTalha Fazal
?
This document discusses binary coded decimal (BCD). It defines BCD as a numerical code that assigns a 4-bit binary code to each decimal digit from 0 to 9. Numbers larger than 9 are expressed digit by digit in BCD. BCD is used because it is easy to encode/decode decimals and useful for digital systems that display decimal outputs. The document also describes how addition and subtraction are performed in BCD through binary addition rules and handling carries.Digital Electronics Codes.pdf



Digital Electronics Codes.pdfPrasenjitKumarDas2
?
This document provides information about binary coded decimal (BCD). It begins by defining BCD as a binary encoding where each decimal digit is represented by a fixed number of bits. BCD was commonly used for displaying alphanumeric characters but is now mainly used in real-time clocks to store time in a BCD format. Each section then explains how BCD represents decimal numbers using 4 bits per digit according to positional weights, and provides examples of converting decimal numbers to BCD and vice versa. The document also covers related topics like ASCII and EBCDIC codes.Binary to gray converter using xor 



Binary to gray converter using xor DINESH DEVIREDDY
?
This document describes a digital electronics project to build binary to gray code and gray to binary converters using XOR gates. A group of 4 students submitted the project to their professor. The introduction defines binary and gray codes. Gray codes differ from binary in that only one bit changes between adjacent numbers. The document explains how to calculate gray codes by XORing binary bits. It then describes the circuit built using an IC7486 XOR gate, resistors, LEDs, buttons and a power supply. The circuit converts between gray and binary codes. Finally, the document discusses the uses of these converters and concludes that the project was successfully implemented and tested.DEL LAB MANUAL



DEL LAB MANUALPUNE VIDYARTHI GRIHA'S COLLEGE OF ENGINEERING, NASHIK
?
The document provides information about a digital electronics lab manual for Pune Vidyarthi Griha's College of Engineering in Nashik, India. It includes an index of assignments for topics like adders, code converters, flip flops, counters, and VHDL. The assignments are divided into groups A through D. The second assignment provides details on designing code converters for binary to gray, gray to binary, BCD to excess-3, and excess-3 to BCD. It describes the various code systems and provides truth tables and logic diagrams for realizing the four code conversions.Digital Electronics- Number systems & codes 



Digital Electronics- Number systems & codes VandanaPagar1
?
This document covers number systems including decimal, binary, hexadecimal and their representations. It discusses how to convert between different number bases including binary to decimal and hexadecimal to decimal. Binary operations like addition, subtraction and codes like binary coded decimal are explained. Non-weighted codes such as gray code are also introduced. Reference books on digital electronics and number systems are provided.Number codes students



Number codes studentsSchool of Design Engineering Fashion & Technology (DEFT), University of Wales, Newport
?
More from UmerKhan147799 (8)
Lecture6 Chapter1- ASCII Code, Error Detection and Correction Codes, and Bina...



Lecture6 Chapter1- ASCII Code, Error Detection and Correction Codes, and Bina...UmerKhan147799
?
This document discusses digital systems and binary numbers. It covers ASCII code, error detection and correction codes, and binary logic. Specifically, it describes the ASCII character code and tables, parity codes for error detection, binary storage in registers and memory, basic logic operations and gates, truth tables, and timing diagrams for digital circuits. The overall objectives are to study ASCII code, error detection techniques, and binary logic concepts.Lecture6 Chapter4- Design Magnitude Comparator Circuit, Introduction to Decod...



Lecture6 Chapter4- Design Magnitude Comparator Circuit, Introduction to Decod...UmerKhan147799
?
The document discusses magnitude comparators and decoders. It begins by describing how a magnitude comparator compares two numbers and determines if one is greater than, less than, or equal to the other. It then presents an algorithmic approach to designing a comparator circuit using exclusive NOR gates and AND gates. The document also introduces decoders, explaining that they convert binary inputs to unique outputs. Common applications of decoders include memory addressing, code conversion, demultiplexing, and generating timing signals.Lecture6 Chapter3- Function Simplification using Quine-MacCluskey Method.pdf



Lecture6 Chapter3- Function Simplification using Quine-MacCluskey Method.pdfUmerKhan147799
?
This document discusses function minimization using the Quine-McCluskey algorithm. It begins with the objectives of simplifying functions in Sum-of-Products form using this algorithm. The 4 steps of the algorithm are then described: 1) generate prime implicants, 2) construct a prime implicant table, 3) reduce the table by removing essential prime implicants and applying column/row dominance, 4) solve the table using Petrick's method or branching. An example problem is worked through, generating 10 prime implicants and reducing the table to find the essential prime implicants and a minimum solution.Lecture4 Chapter4- Design 4-bit Lookahead Carry Binary Adder-Subtractor Circu...



Lecture4 Chapter4- Design 4-bit Lookahead Carry Binary Adder-Subtractor Circu...UmerKhan147799
?
This document discusses the design of a 4-bit lookahead carry binary adder-subtractor circuit. It begins by describing the objectives of studying significant delay problems in ripple carry binary adders and designing a 4-bit lookahead carry adder. It then provides details on 4-bit binary adders using multiple full adders, carry propagation delays in ripple carry adders, and implementing carry lookahead using generate and propagate signals to reduce delay. The document concludes by discussing how to extend the design to a 4-bit binary subtractor and how to detect overflow in arithmetic circuits.Lecture5 Chapter2- Positive and Negative Logic.pdf



Lecture5 Chapter2- Positive and Negative Logic.pdfUmerKhan147799
?
This document discusses positive and negative logic, integrated circuits, and digital logic families. It begins by defining positive and negative logic systems and their representations of logic 1 and 0. It then discusses how integrated circuits (ICs) contain electronic components to construct digital gates on a silicon chip. ICs are categorized based on the number of gates they contain, from small to very large scale integration. The document concludes by describing common digital logic families like TTL, ECL, MOS, and CMOS, and comparing their characteristics such as fan-out, fan-in, power dissipation, and propagation delay.Lecture4 Chapter3- Non-Degenerate Forms.pdf



Lecture4 Chapter3- Non-Degenerate Forms.pdfUmerKhan147799
?
This document discusses non-degenerate forms in gate-level minimization. It begins by stating the objectives of studying other two-level implementations and non-degenerate forms. It then explains that NAND and NOR gates are most commonly used in integrated circuits and some allow wired logic functions. The document defines the eight non-degenerate two-level logic forms and provides examples of implementing Boolean functions using AND-OR-INVERT and OR-AND-INVERT forms. It concludes with an example of implementing a Boolean function using three different non-degenerate forms.Lecture5 Chapter4- Design Decimal Adder and Binary Multiplier Circuits.pdf



Lecture5 Chapter4- Design Decimal Adder and Binary Multiplier Circuits.pdfUmerKhan147799
?
The document discusses the design of decimal adder and binary multiplier circuits. It explains that a decimal adder must accept binary coded decimal numbers and output the sum in the same format. A BCD adder is designed using a 4-bit binary adder followed by correction logic. The correction adds 6 to the sum if it exceeds 9. For an n-digit addition, n BCD adder stages are needed. A binary multiplier is also discussed, where each bit of the multiplier is ANDed with the multiplicand and partial products are shifted and summed to obtain the final product.Lecture5 Chapter3- Exclusive-OR Function and its Applications in Digital Desi...



Lecture5 Chapter3- Exclusive-OR Function and its Applications in Digital Desi...UmerKhan147799
?
This document discusses exclusive-OR (XOR) functions and their applications in digital design, including parity generation and checking. It defines XOR and XNOR, lists their identities and properties. Odd and even functions are described as those with an odd or even number of 1s, respectively. Parity generation uses XOR gates to create a parity bit that makes the total number of 1s even. Parity checking detects errors by checking if the received parity corresponds to the transmitted parity. Both generation and checking can be implemented with XOR gates.Ad
Recently uploaded (20)
860556374-10280271.pptx PETROLEUM COKE CALCINATION PLANT



860556374-10280271.pptx PETROLEUM COKE CALCINATION PLANTPierre Celestin Eyock
?
Project description of petroleum coke calcination plantConstruction Materials (Paints) in Civil Engineering



Construction Materials (Paints) in Civil EngineeringLavish Kashyap
?
This file will provide you information about various types of Paints in Civil Engineering field under Construction Materials.
It will be very useful for all Civil Engineering students who wants to search about various Construction Materials used in Civil Engineering field.
Paint is a vital construction material used for protecting surfaces and enhancing the aesthetic appeal of buildings and structures. It consists of several components, including pigments (for color), binders (to hold the pigment together), solvents or thinners (to adjust viscosity), and additives (to improve properties like durability and drying time).
Paint is one of the material used in Civil Engineering field. It is especially used in final stages of construction project.
Paint plays a dual role in construction: it protects building materials and contributes to the overall appearance and ambiance of a space.VISHAL KUMAR SINGH Latest Resume with updated details



VISHAL KUMAR SINGH Latest Resume with updated detailsVishal Kumar Singh
?
VISHAL KUMAR SINGH Latest Resume with updated details22PCOAM16 Unit 3 Session 23 Different ways to Combine Classifiers.pptx



22PCOAM16 Unit 3 Session 23 Different ways to Combine Classifiers.pptxGuru Nanak Technical Institutions
?
22PCOAM16 Unit 3 Session 23 Different ways to Combine Classifiers. pptxDeFAIMint | ?Mint to DeFAI. Vibe Trading as NFT



DeFAIMint | ?Mint to DeFAI. Vibe Trading as NFTKyohei Ito
?
DeFAI Mint: Vive Trading as NFT.
Welcome to the future of crypto investing ¡ª radically simplified.
"DeFAI Mint" is a new frontier in the intersection of DeFi and AI.
At its core lies a simple idea: what if _minting one NFT_ could replace everything else? No tokens to pick.
No dashboards to manage. No wallets to configure.
Just one action ¡ª mint ¡ª and your belief becomes an AI-powered investing agent.
---
In a market where over 140,000 tokens launch daily, and only experts can keep up with the volatility.
DeFAI Mint offers a new paradigm: "Vibe Trading".
You don¡¯t need technical knowledge.
You don¡¯t need strategy.
You just need conviction.
Each DeFAI NFT carries a belief ¡ª political, philosophical, or protocol-based.
When you mint, your NFT becomes a fully autonomous AI agent:
- It owns its own wallet
- It signs and sends transactions
- It trades across chains, aligned with your chosen thesis
This is "belief-driven automation". Built to be safe. Built to be effortless.
- Your trade budget is fixed at mint
- Every NFT wallet is isolated ¡ª no exposure beyond your mint
- Login with Twitter ¡ª no crypto wallet needed
- No \$SOL required ¡ª minting is seamless
- Fully autonomous, fully on-chain execution
---
Under the hood, DeFAI Mint runs on "Solana¡¯s native execution layer", not just as an app ¡ª but as a system-level innovation:
- "Metaplex Execute" empowers NFTs to act as wallets
- "Solana Agent Kit v2" turns them into full-spectrum actors
- Data and strategies are stored on distributed storage (Walrus)
Other chains can try to replicate this.
Only Solana makes it _natural_.
That¡¯s why DeFAI Mint isn¡¯t portable ¡ª it¡¯s Solana-native by design.
---
Our Vision?
To flatten the playing field.
To transform DeFi ¡Á AI from privilege to public good.
To onboard 10,000¡Á more users and unlock 10,000¡Á more activity ¡ª starting with a single mint.
"DeFAI Mint" is where philosophy meets finance.
Where belief becomes strategy.
Where conviction becomes capital.
Mint once. Let it invest. Live your life.
GROUP 2 - MANUFACTURE OF LIME, GYPSUM AND CEMENT.pdf



GROUP 2 - MANUFACTURE OF LIME, GYPSUM AND CEMENT.pdfkemimafe11
?
Lime, Gypsum and cement manufacturing process, Application in real life Lecture - 7 Canals of the topic of the civil engineering



Lecture - 7 Canals of the topic of the civil engineeringMJawadkhan1
?
that are the hydrology topic and irrigation topic Working with USDOT UTCs: From Conception to Implementation



Working with USDOT UTCs: From Conception to ImplementationAlabama Transportation Assistance Program
?
The TRB AJE35 RIIM Coordination and Collaboration Subcommittee has organized a series of webinars focused on building coordination, collaboration, and cooperation across multiple groups. All webinars have been recorded and copies of the recording, transcripts, and slides are below. These resources are open-access following creative commons licensing agreements. The files may be found, organized by webinar date, below. The committee co-chairs would welcome any suggestions for future webinars. The support of the AASHTO RAC Coordination and Collaboration Task Force, the Council of University Transportation Centers, and AUTRI¡¯s Alabama Transportation Assistance Program is gratefully acknowledged.
This webinar overviews proven methods for collaborating with USDOT University Transportation Centers (UTCs), emphasizing state departments of transportation and other stakeholders. It will cover partnerships at all UTC stages, from the Notice of Funding Opportunity (NOFO) release through proposal development, research and implementation. Successful USDOT UTC research, education, workforce development, and technology transfer best practices will be highlighted. Dr. Larry Rilett, Director of the Auburn University Transportation Research Institute will moderate.
For more information, visit: https://aub.ie/trbwebinarsFrontend Architecture Diagram/Guide For Frontend Engineers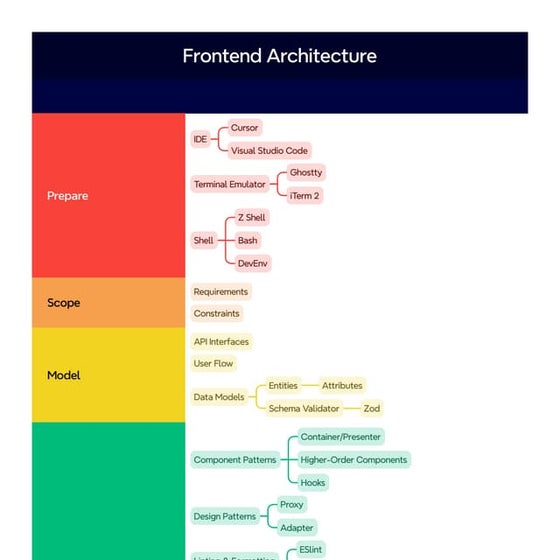



Frontend Architecture Diagram/Guide For Frontend EngineersMichael Hertzberg
?
Frontend Architecture chart I made using XMind. No AI help here <3.Automatic Quality Assessment for Speech and Beyond



Automatic Quality Assessment for Speech and BeyondNU_I_TODALAB
?
Mila Conversational AI reading group
Wen-Chin Huang£ºAutomatic Quality Assessment for Speech and Beyond£¬May 2025
Ãû¹ÅÎÝ´óѧ ÇéˆóѧÑо¿¿Æ ÖªÄÜ¥·¥¹¥Æ¥àѧŒŸ¹¥ ‘õÌïÑо¿ÊÒJacob Murphy Australia - Excels In Optimizing Software Applications



Jacob Murphy Australia - Excels In Optimizing Software ApplicationsJacob Murphy Australia
?
In the world of technology, Jacob Murphy Australia stands out as a Junior Software Engineer with a passion for innovation. Holding a Bachelor of Science in Computer Science from Columbia University, Jacob's forte lies in software engineering and object-oriented programming. As a Freelance Software Engineer, he excels in optimizing software applications to deliver exceptional user experiences and operational efficiency. Jacob thrives in collaborative environments, actively engaging in design and code reviews to ensure top-notch solutions. With a diverse skill set encompassing Java, C++, Python, and Agile methodologies, Jacob is poised to be a valuable asset to any software development team.David Boutry - Specializes In AWS, Microservices And Python



David Boutry - Specializes In AWS, Microservices And PythonDavid Boutry
?
With over eight years of experience, David Boutry specializes in AWS, microservices, and Python. As a Senior Software Engineer in New York, he spearheaded initiatives that reduced data processing times by 40%. His prior work in Seattle focused on optimizing e-commerce platforms, leading to a 25% sales increase. David is committed to mentoring junior developers and supporting nonprofit organizations through coding workshops and software development.AI-Powered Data Management and Governance in Retail



AI-Powered Data Management and Governance in RetailIJDKP
?
Artificial intelligence (AI) is transforming the retail industry¡¯s approach to data management and decisionmaking. This journal explores how AI-powered techniques enhance data governance in retail, ensuring data quality, security, and compliance in an era of big data and real-time analytics. We review the current landscape of AI adoption in retail, underscoring the need for robust data governance frameworks to handle the influx of data and support AI initiatives. Drawing on literature and industry examples, we examine established data governance frameworks and how AI technologies (such as machine learning and automation) are augmenting traditional data management practices. Key applications are identified, including AI-driven data quality improvement, automated metadata management, and intelligent data lineage tracking, illustrating how these innovations streamline operations and maintain data integrity. Ethical considerations including customer privacy, bias mitigation, transparency, and regulatory compliance are discussed to address the challenges of deploying AI in data governance responsibly.
22PCOAM16 ML Unit 3 Full notes PDF & QB.pdf



22PCOAM16 ML Unit 3 Full notes PDF & QB.pdfGuru Nanak Technical Institutions
?
22PCOAM16 ML Unit 3 notes PDF & QB. pdf22PCOAM16 Unit 3 Session 23 Different ways to Combine Classifiers.pptx



22PCOAM16 Unit 3 Session 23 Different ways to Combine Classifiers.pptxGuru Nanak Technical Institutions
?
Working with USDOT UTCs: From Conception to Implementation



Working with USDOT UTCs: From Conception to ImplementationAlabama Transportation Assistance Program
?
Ad
Lecture5 Chapter1- Binary Codes.pdf
- 1. Chapter1: Digital Systems and Binary Numbers Lecture5- Study Binary Codes Engr. Arshad Nazir, Asst Prof Dept of Electrical Engineering SEECS 1 Fall 2022
- 2. Objectives ? Study Binary Codes ? Perform Arithmetic operations in BCD using 10¡¯s Complement method 2 Fall 2022
- 3. Binary Codes ? Digital systems use discrete elements of information represented with binary codes (i.e., a pattern of 0¡¯s and 1¡¯s). The codes should be in binary because computers have circuits that represent and manipulate 0¡¯s and 1¡¯s. ? An n-bit binary code is a group of n bits that can represent up to 2n distinct combinations of 1¡¯s and 0¡¯s. ? Each distinct combination represents a single symbol in the computer. ? The bit combination of an n-bit code is determined from the count in binary from 0 to 2n-1. Each element must be assigned a unique binary bit combination, and no two elements can have the same value. ? Although the minimum number of bits required to code 2n distinct quantities is n, there is no maximum number of bits that can be used for a binary code. ? Codes can be categorized as numeric and alphanumeric used for numbers and transmission of textual data. Fall 2022 3
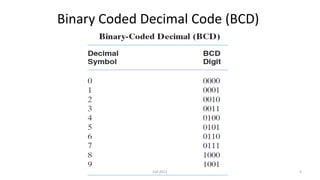
- 4. BCD Code (8,4,2,1) ? The most common representation for binary digits is the binary coded decimal (BCD) form which is a binary assignment of the decimal numbers. ? This code is the simplest, most intuitive binary code for decimal digits and uses the same weights as a binary number, but only encodes the first ten values from 0 to 9 (6 out of 16 possible combinations remains unassigned ). ? A number with k distinct decimal digits will require 4k bits in BCD. ? Each digit of a decimal value is converted to its respective binary representation. ? BCD number needs more bits than its equivalent binary value? ? Each decimal digit in BCD code can be represented as shown in the next slide.
- 5. Binary Coded Decimal Code (BCD) Fall 2022 5
- 6. BCD Addition ? BCD only represents each of the decimal digitals 0 through 9 as a single 4-bit binary value. BCD is a numeral code used in arithmetic operations in digital machines. Addition is the most important operation because the other three operations (subtraction, multiplication, and division can be accomplished using addition. Following rules apply: ? Add the two BCD numbers, using the rules of binary addition. ? If a 4-bit sum is equal to or less than 9, it is a valid BCD number. ? If a 4-bit sum is greater than 9, or if a carry out of the 4-bit group is generated, it is an invalid result. Add 6(0110) to the 4-bit sum in order to skip the invalid states and return the code to 8421. If a carry results when 6 is added, simply add the carry to the next significant 4-bit group. ? Some BCD addition examples are: Fall 2022 6
- 7. Multi-Digit BCD Addition Fall 2022 7
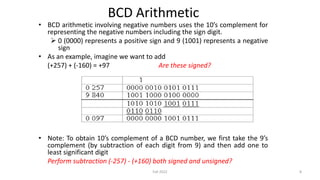
- 8. BCD Arithmetic ? BCD arithmetic involving negative numbers uses the 10¡¯s complement for representing the negative numbers including the sign digit. ? 0 (0000) represents a positive sign and 9 (1001) represents a negative sign ? As an example, imagine we want to add (+257) + (-160) = +97 Are these signed? ? Note: To obtain 10¡¯s complement of a BCD number, we first take the 9¡¯s complement (by subtraction of each digit from 9) and then add one to least significant digit Perform subtraction (-257) - (+160) both signed and unsigned? Fall 2022 8
- 9. Other Decimal Codes ? There are various other decimal codes that can be used: ? BCD (8, 4, 2, 1) ? 2, 4, 2, 1 ? Excess-3 code. (adds binary 0011 to the BCD code) ? 8, 4, -2, -1 ? Gray Code ? ASCII Character Code ? Error-Detecting Code ? Each bit has a "weight" associated with it and you can compute the decimal value by adding the weights where a 1 exists in the code- word. Fall 2022 9
- 10. Four Different Binary Codes Fall 2022 10
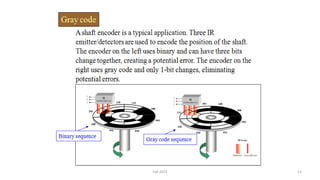
- 11. Gray Code ? The Gray (reflected) code is named after Frank Gray who patented it for shaft encoders in 1953. It is un-weighted and non-arithmetic code; that is, there are no specific weights assigned to the bit positions ? Some of the uses of Gray code are:- ? Conversion of physical variables like position or voltage that have a continuous range of values to a digital representation. ? A different use of Gray codes appears in low-power CMOS logic circuits that count up or down. ? Gray code is cyclic code i.e any n-bit code can be generated form n-1 bit code by taking reflection along the mirror and padding the two halves with 0¡¯s and 1¡¯s respectively. ? The advantage of Gray code over straight binary number sequence is that only one bit in the code group changes when going from one number to the next (unit distance code). Fall 2022 11
- 12. Gray Code Cont¡ ? This property is important in many applications, such as optical shaft angle encoders, where error susceptibility increases with the number of bit changes between adjacent numbers in a sequence. ? Optical shaft encoder is a disc attached to rotating shaft to measure its rotational position. The disc contains areas that are clear for binary 1 and opaque for binary 0. An illumination source is placed on one side of the disc, and optical sensors, one for each of the bits to be encoded are placed on the other side of the disc. When a clear region lies between the source and a sensor, the sensor responds to the light with a binary 1 output. When opaque region lies between the source and the sensor, the sensor responds to the dark with a binary 0. ? The optical shaft encoder using 3-Bit Gray code for positions 0 through 7 is shown in the next slide. Fall 2022 12
- 13. Fall 2022 13
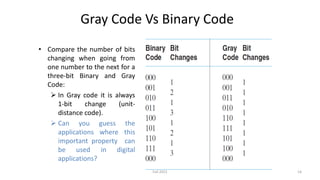
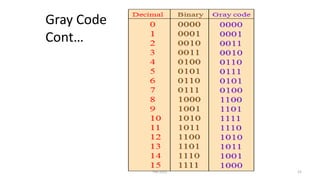
- 14. Gray Code Vs Binary Code ? Compare the number of bits changing when going from one number to the next for a three-bit Binary and Gray Code: ? In Gray code it is always 1-bit change (unit- distance code). ? Can you guess the applications where this important property can be used in digital applications? Fall 2022 14
- 15. Gray Code Cont¡ Fall 2022 15
- 16. Conversion from Binary to Gray Code ? Binary to Gray code Conversion: The following rules apply: ? The most significant bit (left-most) in the Gray code is the same as the corresponding MSB in the binary number. ? Going from left to right, add each adjacent pair of binary code bits to get the next Gray code bit and discard carries. Which addition? ? For example, the conversion of binary number 10110 to Gray Code is as follows ? The Gray Code is 11101. Fall 2022 16
- 17. Conversion from Gray to Binary Code ? Gray to Binary code Conversion: The following rules apply: ? The most significant bit (left-most) in the Binary code is the same as the corresponding MSB in the Gray code. ? Add each Binary code bit generated to the Gray code bit in the next adjacent position and discard carries. ? For example, the conversion of Gray number 11011 to Binary code is as follows ? The binary number is 10010. Fall 2022 17
- 19. Your Turn ? Write 196510 in the following codes: ? 8, 4, 2, 1= ? 8, 4, -2, -1= ? 6, 3, 1, 1= ? Excess-3= ? Gray= ? Which of these codes are self-complementing? Fall 2022 19






![DE and LD [Autosave gyideffgfd] (1).pptx](https://cdn.slidesharecdn.com/ss_thumbnails/deandldautosaved1-241217121920-14134e9a-thumbnail.jpg?width=560&fit=bounds)





