Lesson9
1 like1,372 views
This document provides an overview of modeling approaches for seismic design and assessment of masonry structures, including: - Vertical structures can be modeled as cantilever walls, equivalent frames with varying degrees of coupling between floors/piers. - Equivalent frame models are more realistic and require defining floor/spandrel stiffness. Rigid offsets can limit horizontal deformation. - Refined 2D/3D finite element models may be needed for complex geometries or nonlinear analysis, but are not usually practical. - Linear static analysis uses equivalent static loads distributed by storey based on vibration mode. Nonlinear static pushover analyzes failure by increasing loads until a mechanism forms.
![Storey mechanism idealization (3-d, assuming rigid floor)
The method can be implemented by progressively increasing the displacement of the
center of the seismic force C, and applying the equations developed for the elastic case,
considering a modified stifness for each pier as follows:
u ix = u Rx 慮 ( y i y R ) ; u iy = u Ry + 慮 ( xi x R ) ; 慮i =慮
Stiffness of wall i :
Vix = K xi uix ; K xi = K xi ,elastic if uix uix ,e ;
Vix ,u
Vix = Vix ,u ; K xi = if uix ,e < uix uix ,u ;
uix
Vix = 0; K xi = 0 if uix > uix ,u
Center of rigidity:
K yi xi K xi yi
xR = i ; yR = i
K yi K xi
i i Iterations must be carried out until equilibrium is satisfied
etc. at each displacement increment
Masonry Structures, lesson 8 slide 25
Example of nonlinear storey envelope:
When a relatively large number 1200
Forza alla base-Spostamento
T ET T O
of walls is present, as in most 1属 PIANO
buildings, the storey envelope 1000
has a smooth transition from 800
Interstorey shear (kN)
elastic to ultimate.
Forza [KN]
600
In general, internal forces
distribution at ultimate is 400
governed by strength of walls, 200
not by elastic stiffness, even
when a limited inelastic 0
0 0.01 0.02
deformation capacity of piers is Spostamento [m]
Interstorey displacement at centre of mass (m)
assumed.
Masonry Structures, lesson 8 slide 26](https://image.slidesharecdn.com/lesson9-1211797575288193-8/85/Lesson9-13-320.jpg)
Lesson9
- 1. Seismic design and assessment of Seismic design and assessment of Masonry Structures Masonry Structures Lesson 9 October 2004 Masonry Structures, lesson 8 slide 1 Vertical structures: degree of coupling Role of coupling provided by floors and/or spandrel beams deflected shape and shears and moments deflected shape shears and moments crack pattern and crack pattern (b) (a) deflected shape and shears and moments crack pattern (c) Masonry Structures, lesson 8 slide 2
- 2. Vertical structures: degree of coupling Role of coupling provided by floors and/or spandrel beams Cantilever walls with floor slabs: e.g. reinforced masonry walls heavily reinforced, where out-of-plane stiffness/strength of floor slabs/ring beams is negligible compared to cantilever walls. deflected shape and shears and moments crack pattern Masonry Structures, lesson 8 slide 3 Vertical structures: degree of coupling Role of coupling provided by floors and/or spandrel beams Piers weaker than spandrels: e.g. unreinforced masonry walls w. r.c. slabs and masonry spandrels/deep beams. deflected shape and crack shears and moments pattern Masonry Structures, lesson 8 slide 4
- 3. Vertical structures: degree of coupling Role of coupling provided by floors and/or spandrel beams Spandrels weaker than piers: e.g. reinforced masonry walls w. r.c. slabs and masonry spandrels/deep beams (similar to coupled r.c. walls) deflected shape and crack shears and moments pattern Masonry Structures, lesson 8 slide 5 Vertical structures: modeling Some possible modelling approaches for multistorey masonry walls a) cantilever model b) equivalent frame c) equivalent frame d) 2-D or 3-D finite with rigid offsets element modelling Masonry Structures, lesson 8 slide 6
- 4. Vertical structures: modeling Cantilever model: Is the most conservative type of modeling. Traditionally used for analysis under wind loads. For seismic loading and elastic analysis in the great majority of the cases will give very penalizing results for the designer, especially for unreinforced masonry (sketch on board) Masonry Structures, lesson 8 slide 7 Vertical structures: modeling Equivalent frame, with or without rigid offsets: Can be applied both 2-D and 3-D modeling. Tends to give a more realistic picture of the response. It is more complex because it requires the definition of the stiffness/strength characteristics of horizontal coupling elements (ring beam, spandrels). The use of rigid offsets can be appropriate to limit the deformability of horizontal elements. Horizontal elements are structural, their strength should be verified . Masonry Structures, lesson 8 slide 8
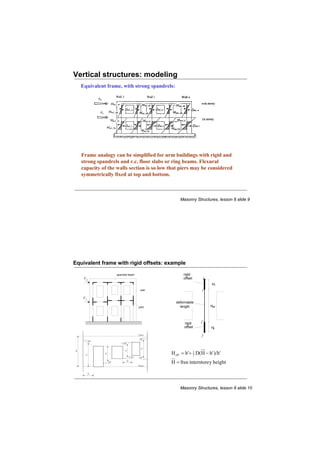
- 5. Vertical structures: modeling Equivalent frame, with strong spandrels: Frame analogy can be simplified for urm buildings with rigid and strong spandrels and r.c. floor slabs or ring beams. Flexural capacity of the walls section is so low that piers may be considered symmetrically fixed at top and bottom. Masonry Structures, lesson 8 slide 9 Equivalent frame with rigid offsets: example spandrel beam rigid F2 offset i H1 pier i' F1 deformable joint length Heff rigid j' offset H2 j H eff = h'+ 1 D( H h' )/h' 3 H = free interstore y height Masonry Structures, lesson 8 slide 10
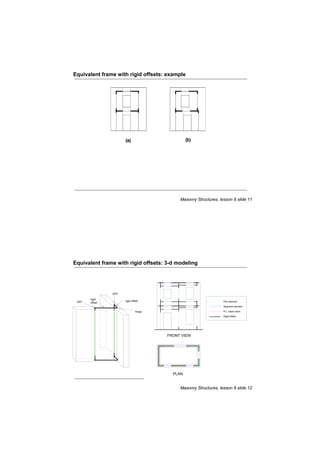
- 6. Equivalent frame with rigid offsets: example Masonry Structures, lesson 8 slide 11 Equivalent frame with rigid offsets: 3-d modeling nodo joint braccio rigid rigido braccio rigido joint nodo rigid offset Pier element offset Spandrel element cerniera hinge R.c. beam elem. Rigid offset FRONT VIEW PLAN Masonry Structures, lesson 8 slide 12
- 7. Equivalent frame with rigid offsets: 3-d modeling Four-storey urm existing building piano rialzato plan 0,6 0,75 letto 0,3 letto bagno 14,3 pranzo cucina 17,7 Masonry Structures, lesson 8 slide 13 Example of linear elastic frame model with commercial software Structural model - Plan Masonry Structures, lesson 8 slide 14
- 8. Example of linear elastic frame model with commercial software Structural model 3D view Masonry Structures, lesson 8 slide 15 Refined 2-d or 3-d finite element modeling Refined finite element modeling could be needed: -In linear elastic analysis, when geometry is rather complicated and no equivalent frame idealization is possible; its use in terms of stress evaluation is questionable, since local elastic stresses are not necessarily related to safety w. respect to collapse of the structure. -In practice in linear elastic models the integration of the stresses to obtain forces and moments is often needed to perform safety checks according to design codes. - In nonlinear analysis for important structures (e.g. monuments) provided suitable constitutive models are used Full nonlinear 3-d f.e.m. modeling of whole buildings is still far from being a usable tool in real practice Masonry Structures, lesson 8 slide 16
- 9. Seismic resistance verification of masonry buildings As will be seen in next lessons, seismic resistance verification of masonry buildings can in principle be carried out using different methods of analysis: - linear static - linear dynamic (modal analysis) - nonlinear static - nonlinear dynamic Masonry Structures, lesson 8 slide 17 Seismic resistance verification of masonry buildings In most cases, for masonry structures there is no need for sophisticated dynamic analyses for seismic resistance verification. An equivalent static analysis (linear on non linear) can often be be adequate. In this lesson, attention will be focused on static analysis. The calculation procedure depends on whether linear or non linear methods are used for assessing the seismic action effects. The typical procedure for linear analysis and seismic resistance verification consists of a series of calculation and steps that are in general common to all design/assessment codes. i. The weight of the building, concentrated at floor levels, is determined by taking into account the suitable combination of gravity loads. Masonry Structures, lesson 8 slide 18
- 10. Linear elastic analysis, equivalent static procedure ii. Using appropriate mathematical models, the stiffness of individual walls in each storey is calculated. The stiffness matrix of the entire structure is evaluated. iii. The period of vibration T is calculated when necessary and the ordinate of the design response spectrum Sd(T) is determined. iv. Assuming that Sd(T) is normalized w. respect to gravity acceleration, the design base shear is determined as Fb,d = Sd(T)W where W is the weight of the seismic masses. v. The base shear is distributed along the height of the building according to a specified rule, derived from a predominant first- mode response, e.g. Wi si Fi = Fb ,d j W j s j Masonry Structures, lesson 8 slide 19 Linear elastic analysis, equivalent static procedure vi. The storey shear is distributed among the walls according to the structural model adopted and the design values of action effects are calculated combining seismic loading and other actions (dead load, variable loads.) vii. Finally the design resistance of wall sections is calculated and compared to the design action effects. Masonry Structures, lesson 8 slide 20
- 11. Nonlinear analysis, equivalent static procedure (a.k.a. pushover) Masonry buildings were among the first structures in which the need for nonlinear analysis methods was felt in practical design/assessment procedures. Simplified nonlinear static procedures were developed and adopted in some national codes in Europe as early as the late Seventies, after the Friuli 1976 earthquake. These procedures were based on the concept of storey mechanism, in which it is assumed that collapse or ultimate limit state of the structure is due by a shear-type failure of a critical storey. The bases of this method are also useful to introduce further developments in nonlinear modeling and nonlinear static procedures as defined in most recent codes. Masonry Structures, lesson 8 slide 21 Nonlinear behaviour of a masonry wall (pier) Possible bi-linear idealization V cyclic envelope Vmax Vu 0,75Vu 0,8V u K el 隆e 隆u 隆 Masonry Structures, lesson 8 slide 22
- 12. Nonlinear behaviour of a masonry wall (pier) Ultimate deflection capacity for masonry piers Earlier proposals based on ductility V cyclic envelope Vmax (隆u = 袖u 隆u ) without reference to failure mode. Vu 0,75Vu e.g. : 袖u= 2.0-3.0 for urm 0,8V u K el 袖u= 3.0-4.0 for confined masonry 隆e 隆u 隆 袖u= 4.0-5.0 for reinforced masonry More recent proposals based on drift (慮= 隆/h) limits: e.g. : 慮u = 0.4-0.5 % for urm failing in shear h 慮u = 0.8-1.2 % for urm failing in flexure/rocking Masonry Structures, lesson 8 slide 23 Storey mechanism idealization Masonry Structures, lesson 8 slide 24
- 13. Storey mechanism idealization (3-d, assuming rigid floor) The method can be implemented by progressively increasing the displacement of the center of the seismic force C, and applying the equations developed for the elastic case, considering a modified stifness for each pier as follows: u ix = u Rx 慮 ( y i y R ) ; u iy = u Ry + 慮 ( xi x R ) ; 慮i =慮 Stiffness of wall i : Vix = K xi uix ; K xi = K xi ,elastic if uix uix ,e ; Vix ,u Vix = Vix ,u ; K xi = if uix ,e < uix uix ,u ; uix Vix = 0; K xi = 0 if uix > uix ,u Center of rigidity: K yi xi K xi yi xR = i ; yR = i K yi K xi i i Iterations must be carried out until equilibrium is satisfied etc. at each displacement increment Masonry Structures, lesson 8 slide 25 Example of nonlinear storey envelope: When a relatively large number 1200 Forza alla base-Spostamento T ET T O of walls is present, as in most 1属 PIANO buildings, the storey envelope 1000 has a smooth transition from 800 Interstorey shear (kN) elastic to ultimate. Forza [KN] 600 In general, internal forces distribution at ultimate is 400 governed by strength of walls, 200 not by elastic stiffness, even when a limited inelastic 0 0 0.01 0.02 deformation capacity of piers is Spostamento [m] Interstorey displacement at centre of mass (m) assumed. Masonry Structures, lesson 8 slide 26