Manisha ratio
- 1. Fractions 1 /8 55 / 60 /12 11 1 2/10 1¬Ω 1 /12
- 2. What is a fraction? Loosely speaking, a fraction is a quantity that cannot be represented by a whole number. Why do we need fractions? Consider the following scenario. Can you finish the whole cake? If not, how many cakes did you eat? 1 is not the answer, neither is 0. This suggest that we need a new kind of number.
- 3. Definition: A fraction is an ordered pair of whole numbers, the 1st one is usually written on top of the other, such as ½ or ¾ . a numerator b denominator The denominator tells us how many congruent pieces the whole is divided into, thus this number cannot be 0. The numerator tells us how many such pieces are being considered.
- 4. Examples: How much of a pizza do we have below? • we first need to know the size of the original pizza. The blue circle is our whole. - if we divide the whole into 8 congruent pieces, - the denominator would be 8. We can see that we have 7 of these pieces. Therefore the numerator is 7, and we have 7 of a pizza. 8
- 5. DO NOW (not later): ÔÅÆ Compare the number of students sitting on left side and on the right side of the class.
- 6. ÔÅÆ The number of students sitting at left side= ÔÅÆ The number of students sitting at right side = ÔÅÆ If we compare students sitting at left side to students sitting at right side we get ___ students sitting at left side to _____ students sitting at right side.
- 7. What do we call a comparison between two or more quantities? We just found the RATIO RATIO of students sitting at left side to right side. Is the ratio of students sitting at left side to right side the same ? No, when writing a ratio, ORDER matters.
- 8. AIM: What is a ratio?
- 9. How many basketballs to footballs are there? ÔÅÆ For every 4 basketballs there are 6 footballs. ÔÅÆ The ratio is 4 to 6.
- 10. What are some other ways we can write the ratio of basketball to footballs? Every ratio can be written in 3 ways: ÔÅÆ 4 to 6 First quantity to Second quantity Careful!! Order matters in a ratio. ÔÅÆ 4:6 First quantity : Second quantity 4 to 6 ÔÅÆ 4 First quantity divided by the second Is NOT the same as 6 quantity (as a fraction). 6 to 4
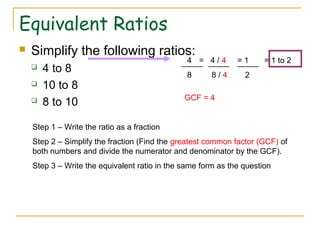
- 11. Equivalent Ratios  Simplify the following ratios: 4 = 4/4 =1 = 1 to 2  4 to 8 8 8/4 2  10 to 8 GCF = 4  8 to 10 Step 1 – Write the ratio as a fraction Step 2 – Simplify the fraction (Find the greatest common factor (GCF) of both numbers and divide the numerator and denominator by the GCF). Step 3 – Write the equivalent ratio in the same form as the question
- 12. Equivalent Ratios can be formed by multiplying the ratio by any number.  For example, the ratio 2 : 3 can also be written as  4 : 6 (multiply original ratio by by 2)  6 : 9 (multiply original ratio by by 3)  8 : 12 (multiply original ratio by by 4) The ratio 2 : 3 can be expressed as 2x to 3x (multiply the original ratio by any number x)
- 13. Compound Ratios  A ratio that compares more than 2 quantities is called a compound ratio.  Example:  A cake recipe says the ratio of cups of milk, sugar, and butter are 1:2:4.  This means that there is one cup of milk for every two cups of sugar and four cups of butter.
- 14. Home assignment: 1) You go to a party where the ratio of boys to girls is 28 to 56. Express the ratio of boys to girls in simplest form. 2) Explain what this ratio tells us.