material science 2 .pptx
- 1. MATERIAL SCIENCE ENG. KAREEM. H. MOKHTAR
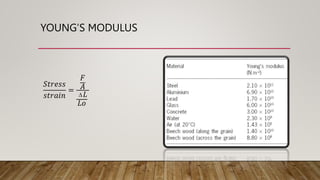
- 2. STRESS AND STRAIN ‚Ä¢ Stress = ‚Ä¢ Strain = ‚Ä¢ ùëÜùë°ùëüùëíùë†ùë† ùë†ùë°ùëüùëéùëñùëõ = Y (young‚Äôs modulus) F A eL = DL L o Big Small Weak material Strong material
- 3. STRESS
- 4. STRESS • 1- Compressive stress = F A o 2 m N
- 5. STRESS original area before loading Area, A Ft Ft s = Ft A o 2 f 2 m N or in lb = 2- Tensile stress (s)
- 6. STRESS • 3- Shear stress (t) Area, A Ft Ft Fs F F Fs t = Fs Ao
- 7. STRAIN e = d Lo d/2 dL/2 Lo wo • Lateral strain: eL = L wo d • Tensile strain:
- 8. STRAIN • Shear strain g = Dx/H H
- 10. EXAMPLE 1 Givens Weight = 1000N Area of the beam is 2cm x 2cm Lo = 1.75 m Find delta L Material Fe ÔɆ youngs modulus Yùëúùë¢ùëõùëîùë† ùëöùëúùëëùë¢ùëôùë¢ùë† = ùëÜùë°ùëüùëíùë†ùë† ùë†ùë°ùëüùëéùëñùëõ = ùêπ ùê¥ ùêø ùêøùëú = 1000 2 ùë• 2 ùë• 10‚àí4 ? 1.75 D
- 11. EXAMPLE 2 F Givens Weight= 50 Kg Radius of the heel= 0.5 cm 30% of the woman's weight acts on the heel Solution ‚Ä¢ stress = F A o = 50 ‚àó 0.3 ‚àó 9.8 ùëùùëñ 4 ‚àó 0.012 = 1.87 ‚àó 106 ùëÅ/ùëö2
- 12. EXAMPLE 4 Givens D= 1mm Lo= 4m m= 500 kg Find the elongation young‚Äôs modulus (AL) = 6.9* 1010 N/m2 Solution young‚Äôs modulus= ùëÜùë°ùëüùëíùë†ùë† ùë†ùë°ùëüùëéùëñùëõ = ùêπ ùê¥ ùêø ùêøùëú = 500‚àó9.8 ùëùùëñ 4 ‚àó 10‚àí6 ? 4 = 6.9* 1010
- 13. MAX STRESS (BREAKING STRESS) • It is the max stress a material can handle before it breaks
- 14. EXAMPLE 6 • Would the wire breaks or not? • F/ A = 500 * 9.8 / pi*0.00052 • If the stress is > max stress  the wire will break • If the stress is < max stress  it will not break • What is the maximum weight this wire could handle • StressMAX = mmax* g / A Mmax= 6 kg
- 15. STRESS AND STRAIN IN DIFFERENT FORCE DIRECTIONS Tension Compression Shear Stress= F/A Stress= F/A Stress= F/A Strain= delta (L)/Lo Strain= delta (L)/Lo Strain= delta (x)/H
- 16. EXAMPLE 3 • F = ? • Strain = 1% • L= 1cm • Steel youngs modulus = 21x1010 N/m2 • Solution • 21 MPa
- 17. EXAMPLE 8 • Lo = 40m • Dia= 1cm • Delta(L) = 2m • Weight = 900N climber • Y = ? • Solution • Y=229,183,118 N/m2
- 18. SHEAR MODULUS (S) ‚Ä¢ ù놂Ñéùëíùëéùëü ùë†ùë°ùëüùëíùë†ùë† ù놂Ñéùëíùëéùëü ùë†ùë°ùëüùëéùëñùëõ = ùêπ ùê¥ ùëëùëíùëôùë°ùëé (ùë•) ùêª H
- 19. SHEAR STRESS • It is used in cutting materials • The area used is the area that is going to be cut
- 20. EXAMPLE 9 ‚Ä¢ H = 5 cm ‚Ä¢ W= 20 cm ‚Ä¢ L= 2cm ‚Ä¢ F= 1000N ‚Ä¢ Carbon steel shear modulus= 7.7 * 1010 W H ù놂Ñéùëíùëéùëü ùë†ùë°ùëüùëíùë†ùë† ù놂Ñéùëíùëéùëü ùë†ùë°ùëüùëéùëñùëõ = ùêπ ùê¥ ùëëùëíùëôùë°ùëé (ùë•) ùêª ùëëùëíùëôùë°ùëé (ùë•) = 1.67 * 10-7 m
- 21. EXAMPLE 10 5 m 2.4 m Max stress = ùêπ ùê¥ 4 x 108 = ùêπ 2.4 ‚àó0.002 F must be greater than 1920000 N
- 22. EXAMPLE 12 Givens The diameter of the drilling bit= 4.2 cm Thickness of the sheet = 5mm Length of the sheet = 5 m Width of the sheet = 3 m Shear stress of steel = 4 x 10^8 Solution Shear stress= F/A F= 4 *10^8 * pi * 0.042*0.005 = 263893.8 N
- 23. BULK MODULUS ùêµùë¢ùëôùëò ùë†ùë°ùëüùëíùë†ùë† ùêµùë¢ùëôùëò ùë†ùë°ùëüùëéùëñùëõ = ùêπ ùê¥ ‚àí ùëëùëíùëôùë°ùëé (ùë£) ùëâùëú Compressibility = 1 ùêµùë¢ùëôùëò ùëöùëúùëëùë¢ùëôùë¢ùë† The higher the bulk modulus the more force you need to Change the volume of the material
- 24. EXAMPLE 13 ‚Ä¢ A Bowling ball made of steel sunk in an ocean, find the change in it‚Äôs volume if you know that the ocean is 10000 m in depth and the original volume of the ball is 1m3 ùêµùë¢ùëôùëò ùë†ùë°ùëüùëíùë†ùë† ùêµùë¢ùëôùëò ùë†ùë°ùëüùëéùëñùëõ = ùêπ ùê¥ ùëëùëíùëôùë°ùëé (ùë£) ùëâùëú = Bulk modulus
- 25. EXAMPLE 14 ‚Ä¢ Compressibility = ‚àí ùëëùëíùëôùë°ùëé (ùë£) ùëâùëú ùêπ ùê¥ ‚Ä¢ Substitute ‚Ä¢ Compressibility = 3 x 10-10 m3/ N
- 26. EXAMPLE 15 Shear modulus (S) = ù놂Ñéùëíùëéùëü ùë†ùë°ùëüùëíùë†ùë† ù놂Ñéùëíùëéùëü ùë†ùë°ùëüùëéùëñùëõ = ùêπ ùê¥ ùëëùëíùëôùë°ùëé (ùë•) ùêª
- 27. SOLVE THIS QUESTION • A carbon steel cylinder that has a young’s modulus of 200 GPa, bulk modulus of 140 GPA, And max shear stress of 256000 N/m2 • The radius of the upper and lower faces are 5 cm and the height is 20 cm • A) If the specimen is subjected to a force of 100 KN. How long will it be stretched? • B) Will it be cut using a force of 20000. N • C) The strain at which this specimen starts its plastic region is at 6 x 10-5 , after releasing the applied force will it return back or not? And why (by calculations)
- 28. SOLUTION : A) IF THE SPECIMEN IS SUBJECTED TO A FORCE OF 100 KN. HOW LONG WILL IT BE STRETCHED? • Y = stress/strain • Stress = F/A = 100,000/pi * 0.052 = 12732395.4 N/m2 • Strain = delta (l) / 0.2 • Using Y= 200 Gpa • Delta (l) = 0.000013 m
- 29. SOLUTION: B) WILL IT BE CUT USING A FORCE OF 20000 N • Shear stress = F/A = 20000/pi*0.052 • And given that max shear stress is 256000 N/m2 • As the shear stress is
- 30. ANSWER: C) THE STRAIN AT WHICH THIS SPECIMEN STARTS ITS PLASTIC REGION IS AT 6 X 10-5 , AFTER RELEASING THE APPLIED FORCE WILL IT RETURN BACK OR NOT? AND WHY (BY CALCULATIONS) • From a) the change in length is found to be 0.000013 m • The strain = delta (l)/Lo = 0.000065 = 6.5*10-5 • As the strain is higher than the strain at which the specimen enters the plastic region, it will not return back to its original shape.