MATHEMATICS XI TEST PAPER SOL.pdf
0 likes7 views
The document contains 12 math problems involving expansions of binomial expressions, arithmetic progressions, and other algebra topics. The problems are multi-step and require setting up and solving equations. No final answers are provided.
1 of 9
Download to read offline




![Ans :
4
10.
Ans :
4
11.
Ans :
4
12.
Sum = (33 + 53 + 73 + ...) – (23 + 43 + 63 + ...)
For 33 + 53 + 73 + ...
an = (2n + 1)3 = 8n3 + 12n2 + 6n + 1
∴ S1 = 8 Σn3 + 12 Σn2 + 6 Σn + n ... (i)
For 23 + 43 + 63 + ...
an = (2n)3 = 8n3
S2 = 8Σn3 ... (ii)
∴ Sum = S1 – S2 = 12 Σn2 + 6 Σn + n [From (i), (ii)]
Sum to 10 terms =
= 4620 + 330 + 10 = 4960
If a, b, c be the first, third and nth term respectively of an A.P. Prove that the sum to n terms is
Given first term = a, a3 = b and an = c
Sn = [2a + (n – 1)d] ... (i)
We have first term = a
b = a + 2d and a + (n – 1) d = c
Sn = [a + c] [From (i)] ... (ii)
Also a + (n – 1) = c
⇒ n – 1 =
Substituting in (ii), we get
Sn =
If a, b, c are in G.P and . Prove that x, y, z are in A.P.
⇒ a = kx, b = ky, c = kz
Given b2 = ac ⇒ (ky)2 = kx. kz ⇒ k2y = kx + z
⇒ 2y = x + z ⇒ x, y, z in A.P.
If a1, a2, a3, ... an are in A.P., where ai > 0 for all i, show that
=](https://image.slidesharecdn.com/mathematicsxitestpapersol-221017171711-310f27e7/85/MATHEMATICS-XI-TEST-PAPER-SOL-pdf-5-320.jpg)



![Ans :
6
17.
Ans :
mn (mn + 1)
S1 = Σ(1, 2, 3..............) = [2.1 + (n – 1).1]
S2 = Σ(2, 5, 8..............) = [2.2 + (n – 1).3]
S3 = Σ(3, 8, 13............) = [2.3 + (n – 1).5]
Sm = [2.m + (n – 1) (2m – 1)]
S1 + S2 + ...... + Sm = [2 (1 + 2 + 3.......m) + (n – 1) (1 + 3 + 5........+ 2m – 1)]
=
=
If A and G be A.M. and G.M., respectively between two positive numbers, prove that the
numbers are A ± .
Let the numbers be a and b.
= A ⇒ a + b = 2A ...(i)
= G ⇒ ab = G2
(a – b)2 = (a + b)2 – 4ab = 4A2 – 4G2
⇒ 4(A2 – G2)
a – b = ...(ii)
From (i) and (ii), we get
a = A + and b = A –
Hence, the numbers are A ±](https://image.slidesharecdn.com/mathematicsxitestpapersol-221017171711-310f27e7/85/MATHEMATICS-XI-TEST-PAPER-SOL-pdf-9-320.jpg)
Recommended
MATHEMATICS XI TEST PAPER.pdf



MATHEMATICS XI TEST PAPER.pdfAshwani Jha
Ìı
1. The document provides a list of 16 math problems involving topics like binomial expansions, arithmetic progressions, common differences, ratios of means, and equations with common roots.
2. Problem 4 asks to prove that if the coefficients of the 2nd, 3rd, and 4th terms in the expansion of (1 + x)2n are in arithmetic progression, then 2n2 – 9n + 7 = 0.
3. Problem 15 asks to find the sum of the terms of n arithmetic progressions up to n terms, where the first terms are consecutive integers and the common differences increase consecutively by 2.ArithmeticProgression 



ArithmeticProgression Prashant Jain
Ìı
1. The document contains examples of arithmetic progressions and their properties. It includes problems involving finding terms, common differences, and sums of APs.
2. One problem involves two APs where the ratio of the sum of the first n terms is 7n+1:4n+27. It is shown that the ratio of the 11th terms is 4:3.
3. Another problem proves that if an AP has (2n+1) terms, the ratio of the sum of odd terms to the sum of even terms is (n+1):n.mathematics part-2.docx



mathematics part-2.docxLakeshkumarpadhy
Ìı
The document contains solutions to several math problems involving arithmetic progressions (APs) and geometric progressions (GPs). It summarizes key information about various AP and GP sequences, including their first terms, common differences/ratios, number of terms, and calculated sums. It also shows sample calculations for finding terms, differences, and sums of numeric AP and GP sequences.3.-SEQUENCES-AND-SERIES-THEORY.hhsssspdf



3.-SEQUENCES-AND-SERIES-THEORY.hhsssspdfnassorokayanda9412
Ìı
JshshsusjwusbzhzhssjzisjsjshsbzjjznsnsjzhzjswnsnjszjzhsnjskzzjbzjsskzjhsnsnsnsjsnsnsjsnnzzjjzjzzjzjzjjzjzjzjjzjzjznsbsbszbzbzjzjzjzjjssjjzjzsjzjzjzjzjsjsjjzjzzjjzzjjzzjjzzjzjAlgebra



Algebrashahzadebaujiti
Ìı
The document discusses several topics in algebra including:
1. Indices laws including am x an = am + n, am ÷ an = am - n, and (am)n = amn. Negative and fractional indices are also discussed.
2. Logarithms including the definition that logarithm of 'x' to base 'a' is the power to which 'a' must be raised to give 'x'. Change of base formula is also provided.
3. Series including the definition of finite and infinite series. Notation of sigma notation ∑ is introduced to represent the sum of terms.2018 mtap for g10 with answers



2018 mtap for g10 with answersJashey Dee
Ìı
The document contains 18 math word problems with their step-by-step solutions. The problems cover a range of topics including arithmetic sequences, geometric sequences, percentages, factorials, trigonometry, and more. The final problem asks to find the 12th term of a sequence where the first two terms are 3 and 2, and subsequent terms are the sum of all preceding terms. The solution shows this forms a geometric sequence and calculates the 12th term as 2,560.Sequence and Series



Sequence and SeriesTCYonline No.1 testing platform(Top Careers and You)
Ìı
FREE MCA NATIONAL LEVEL MOCK TEST
Click on link given below:
www.tcyonline.com/activatefree.php?id=14
TCYonline.com
No.1 Testing PlatformOn Triplet of Positive Integers Such That the Sum of Any Two of Them is a Per...



On Triplet of Positive Integers Such That the Sum of Any Two of Them is a Per...inventionjournals
Ìı
In this article we discussed determination of distinct positive integers a, b, c such that a + b, a + c, b + c are perfect squares. We can determine infinitely many such triplets. There are such four tuples and from them eliminating any one number we obtain triplets with the specific property. We can also obtain infinitely many such triplets from a single triplet. ch3.ppt



ch3.pptHaiderAli252366
Ìı
The simplest and most common form of mathematical induction infers that a statement involving a natural number n (that is, an integer n ≥ 0 or 1) holds for all values of n. The proof consists of two steps:
The base case (or initial case): prove that the statement holds for 0, or 1.
The induction step (or inductive step, or step case): prove that for every n, if the statement holds for n, then it holds for n + 1. In other words, assume that the statement holds for some arbitrary natural number n, and prove that the statement holds for n + 1Sequence function



Sequence functionjennytuazon01630
Ìı
The document defines key concepts related to sequences and series. It explains that a sequence is an ordered list of numbers with a specific pattern or rule. A sequence function is a function whose domain is the set of natural numbers. Terms are the individual numbers in a sequence. Finite sequences have a set number of terms while infinite sequences continue without end. Partial sums refer to adding a specific number of terms. Sigma notation compactly represents the sum of terms in a sequence. The document also introduces the principle of mathematical induction as a method to prove that statements are true for all natural numbers.Introduction to Mathematical Induction!!



Introduction to Mathematical Induction!!ssuser29ba22
Ìı
A brief introduction to MI and some simple examples New Pythagorean Triples copy



New Pythagorean Triples copyAndrew Erskine
Ìı
Pythagorean triples are whole number sets that satisfy the Pythagorean theorem, where a2 + b2 = c2. The document discusses properties of Pythagorean triples and how they relate to rational points on the unit circle. It presents theorems showing that every basic Pythagorean triple corresponds to a rational point on the unit circle, and vice versa. Formulas are derived for generating Pythagorean triples from a given rational slope of a line passing through (-1,0) and a point on the unit circle. The document also briefly discusses 60-degree triangles, whose sides satisfy the equation c2 = a2 + b2 - ab, relating to an ellipse rather than a circle.(678215997) neethutext



(678215997) neethutextneethumaths
Ìı
This document provides an introduction to sequences and series. It begins with definitions of sequences, finite and infinite sequences, and series. It then covers topics like arithmetic progressions, geometric progressions, and harmonic progressions. It provides formulas for the nth term and sum of terms for arithmetic and geometric progressions. It also defines arithmetic mean and geometric mean between terms in progressions. The document aims to help secondary students understand key concepts related to sequences and series.Presntation11



Presntation11Bindiya syed
Ìı
This document contains information from a class presentation on computing elements of one-dimensional arrays and recursively defined sequences. It discusses how to count the elements of an array that is cut in the middle, the probability of an element having an even or odd subscript, computing terms of a recursively defined sequence, and properties of relations. The presentation was given by 5 students to their instructor for their BS in Computer Science class from 2013-2017.Sequence and series



Sequence and seriesKAZEMBETVOnline
Ìı
SEQUENCE AND SERIES
SEQUENCE
Is a set of numbers written in a definite order such that there is a rule by which the terms are obtained. Or
Is a set of number with a simple pattern.
Example
1. A Ìıset of even numbers
ÌıÌıÌıÌı • 2, 4, 6, 8, 10 ……
2. A set of odd numbers
ÌıÌıÌıÌı • 1, 3, 5, 7, 9, 11….
Knowing the pattern the next number from the previous can be obtained.
ÌıExample
Ìı1. Find the next term from the sequence
ÌıÌı • 2, 7, 12, 17, 22, 27, 32
The next term is 37.
2. Given the sequence
ÌıÌı • 2, 4, 6, 8, 10, 12………Chapter1.integer s.y.b.c.s



Chapter1.integer s.y.b.c.svidyabhoge1
Ìı
This document discusses the key concepts from several units in mathematics including integers, groups, finite groups, subgroups, and groups in coding theory. It then provides details on specific topics within these units, including equivalence relations, congruence relations, equivalence class partitions, the division algorithm, greatest common divisors (GCD) using division, and Euclid's lemma. The document aims to provide students with fundamental mathematical principles, methods, and tools to model, solve, and interpret a variety of problems. It also discusses enhancing students' development, problem solving skills, communication, and attitude towards mathematics.Arithmetic progressions



Arithmetic progressionsPrakashChinchali
Ìı
The document defines arithmetic progressions and provides examples. Some key points:
- An arithmetic progression is a list of numbers where each term is obtained by adding a fixed number (called the common difference) to the preceding term.
- The general formula for the nth term of an AP is an = a + (n-1)d, where a is the first term and d is the common difference.
- Examples show how to determine if a list of numbers forms an AP, write the next terms, and find a specific term by using the general formula.
- There are finite APs, which have a last term, and infinite APs, which go on forever without a last term.(677528443) neethu text (2)



(677528443) neethu text (2)neethumaths
Ìı
This document provides an introduction to sequences and series. It begins with definitions of sequences, series, arithmetic progressions, geometric progressions and their key terms. It then presents several examples of finding terms in arithmetic and geometric progressions. The document also defines arithmetic mean and geometric mean, and discusses how to find the sum of terms in an arithmetic progression using formulas. Overall, the document serves as an introductory guide to common concepts involving sequences and progressions.Maths05



Maths05sansharmajs
Ìı
The document provides solutions to questions from an IIT-JEE mathematics exam. It includes 8 questions worth 2 marks each, 8 questions worth 4 marks each, and 2 questions worth 6 marks each. The solutions solve problems related to probability, trigonometry, geometry, calculus, and loci. The summary focuses on the high-level structure and content of the document.Binomial



BinomialDEDESUDJADI
Ìı
The document provides an introduction to the binomial theorem. It begins by discussing binomial coefficients through the Pascal's triangle. It then derives an explicit formula for binomial coefficients using factorials. Finally, it states the binomial theorem and provides examples of using it to expand algebraic expressions and estimate numerical values.Imc2017 day1-solutions



Imc2017 day1-solutionsChristos Loizos
Ìı
This document contains solutions to 5 problems posed at the IMC 2017 conference. The solutions are summarized as follows:
1) The possible eigenvalues of the matrix A described in Problem 1 are 0, 1, and -1±√3i/2.
2) Problem 2 proves that for any differentiable function f satisfying the Lipschitz condition, f(x)2 < 2Lf(x) for all x.
3) Problem 3 shows that for any set S subset of {1,2,...,2017}, there exists an integer n such that the sequence ak(n) defined in the problem satisfies the property that ak(n) is a perfect square if and only if k isExtension 1 miscellaneous worksheet



Extension 1 miscellaneous worksheetAndrew Rybkow
Ìı
This document contains 10 math problems involving trigonometric functions, coordinate geometry, and calculus. The problems cover topics like expressing trig functions in alternate forms, proving trigonometric identities, finding equations of lines tangent to parabolas and cubic functions, solving triangles given side lengths and angles, and dividing line segments in a given ratio.Ijetr011923



Ijetr011923ER Publication.org
Ìı
ER Publication,
IJETR, IJMCTR,
Journals,
International Journals,
High Impact Journals,
Monthly Journal,
Good quality Journals,
Research,
Research Papers,
Research Article,
Free Journals, Open access Journals,
erpublication.org,
Engineering Journal,
Science Journals,
Binomial theorem for any index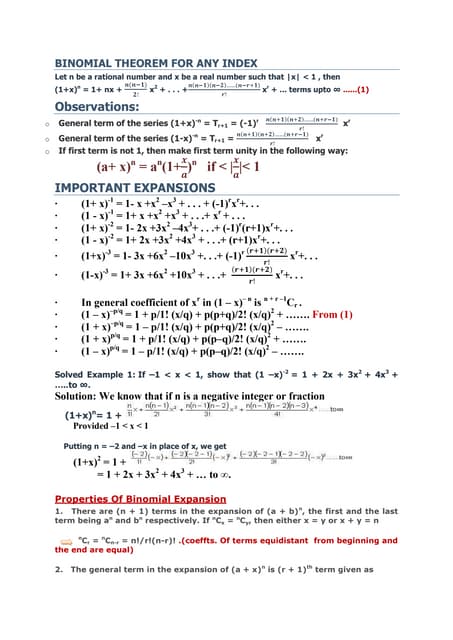



Binomial theorem for any indexindu psthakur
Ìı
The document summarizes the binomial theorem and properties of binomial coefficients. It provides:
1) The binomial theorem expresses the expansion of (a + b)n as a sum of terms involving binomial coefficients for any positive integer n.
2) Important properties of binomial coefficients are discussed, such as their relationship to factorials and the symmetry of coefficients.
3) Examples are given of using the binomial theorem to find coefficients and solve problems involving divisibility and series of binomial coefficients.PHY XII TEST PAPER.pdf



PHY XII TEST PAPER.pdfAshwani Jha
Ìı
This document contains the answers to multiple choice questions related to concepts in electrostatics. The answers reference concepts like Gauss's law, electric field, capacitance, and electric potential. For each question, the response provides a brief 1-2 sentence explanation for the reasoning behind the selected multiple choice answer.PHY XII TEST PAPERQ.pdf



PHY XII TEST PAPERQ.pdfAshwani Jha
Ìı
1. The document contains 14 multiple choice questions about concepts in electrostatics such as electric fields, electric flux, Gauss's law, capacitors, and electric potential.
2. The questions cover topics like determining the direction of an electric field, calculating electric flux through surfaces, comparing stored electrostatic energy in capacitors, and analyzing diagrams of electric field configurations.
3. Many questions ask the examinee to apply concepts like Gauss's law to solve for electric fields or fluxes in situations involving conducting and non-conducting charged objects.Advanced Support Vector Machine for classification in Neural Network



Advanced Support Vector Machine for classification in Neural NetworkAshwani Jha
Ìı
This paper proposes a method to classify EEG signals using a support vector machine (SVM) classifier combined with a quicksort sorting algorithm (SVM-Q). The method involves extracting phase locking value (PLV) features from EEG data, sorting the feature vectors using quicksort, and then applying SVM classification. When tested on two BCI competition datasets, SVM-Q achieved classification accuracies of 86% and 77%, outperforming plain SVM which achieved 62% and 54% respectively. The paper concludes that adding a sorting algorithm to SVM can improve its classification performance for brain-computer interface applications.FEM problem of elasticity



FEM problem of elasticityAshwani Jha
Ìı
(1) The document solves for the approximate deflection of a simply supported beam carrying a symmetrical triangular load P using the Rayleigh Ritz method.
(2) It models the deflection w(x) and derives equations for the total potential energy Î and moment of inertia I2 in terms of w(x).
(3) By taking the derivative of Î with respect to the constants C1 and C2 and setting them to zero, it arrives at the solution for the deflection w(x) = (Pl^3/48EI)(4x^3/l^3 - 3x^2/l^2) which matches the exact solution.K nn



K nnAshwani Jha
Ìı
K-Nearest Neighbor (KNN) is an instance-based learning algorithm where classification of new data points is based on the majority class of its k nearest neighbors. It works by storing all training examples and classifying new examples based on the majority class of its nearest neighbors, where distance between examples is measured using a metric like Euclidean distance. KNN can perform both classification and regression tasks, with classification being the majority class for discrete targets and regression being the average of the k nearest neighbors' values for continuous targets.More Related Content
Similar to MATHEMATICS XI TEST PAPER SOL.pdf (17)
ch3.ppt



ch3.pptHaiderAli252366
Ìı
The simplest and most common form of mathematical induction infers that a statement involving a natural number n (that is, an integer n ≥ 0 or 1) holds for all values of n. The proof consists of two steps:
The base case (or initial case): prove that the statement holds for 0, or 1.
The induction step (or inductive step, or step case): prove that for every n, if the statement holds for n, then it holds for n + 1. In other words, assume that the statement holds for some arbitrary natural number n, and prove that the statement holds for n + 1Sequence function



Sequence functionjennytuazon01630
Ìı
The document defines key concepts related to sequences and series. It explains that a sequence is an ordered list of numbers with a specific pattern or rule. A sequence function is a function whose domain is the set of natural numbers. Terms are the individual numbers in a sequence. Finite sequences have a set number of terms while infinite sequences continue without end. Partial sums refer to adding a specific number of terms. Sigma notation compactly represents the sum of terms in a sequence. The document also introduces the principle of mathematical induction as a method to prove that statements are true for all natural numbers.Introduction to Mathematical Induction!!



Introduction to Mathematical Induction!!ssuser29ba22
Ìı
A brief introduction to MI and some simple examples New Pythagorean Triples copy



New Pythagorean Triples copyAndrew Erskine
Ìı
Pythagorean triples are whole number sets that satisfy the Pythagorean theorem, where a2 + b2 = c2. The document discusses properties of Pythagorean triples and how they relate to rational points on the unit circle. It presents theorems showing that every basic Pythagorean triple corresponds to a rational point on the unit circle, and vice versa. Formulas are derived for generating Pythagorean triples from a given rational slope of a line passing through (-1,0) and a point on the unit circle. The document also briefly discusses 60-degree triangles, whose sides satisfy the equation c2 = a2 + b2 - ab, relating to an ellipse rather than a circle.(678215997) neethutext



(678215997) neethutextneethumaths
Ìı
This document provides an introduction to sequences and series. It begins with definitions of sequences, finite and infinite sequences, and series. It then covers topics like arithmetic progressions, geometric progressions, and harmonic progressions. It provides formulas for the nth term and sum of terms for arithmetic and geometric progressions. It also defines arithmetic mean and geometric mean between terms in progressions. The document aims to help secondary students understand key concepts related to sequences and series.Presntation11



Presntation11Bindiya syed
Ìı
This document contains information from a class presentation on computing elements of one-dimensional arrays and recursively defined sequences. It discusses how to count the elements of an array that is cut in the middle, the probability of an element having an even or odd subscript, computing terms of a recursively defined sequence, and properties of relations. The presentation was given by 5 students to their instructor for their BS in Computer Science class from 2013-2017.Sequence and series



Sequence and seriesKAZEMBETVOnline
Ìı
SEQUENCE AND SERIES
SEQUENCE
Is a set of numbers written in a definite order such that there is a rule by which the terms are obtained. Or
Is a set of number with a simple pattern.
Example
1. A Ìıset of even numbers
ÌıÌıÌıÌı • 2, 4, 6, 8, 10 ……
2. A set of odd numbers
ÌıÌıÌıÌı • 1, 3, 5, 7, 9, 11….
Knowing the pattern the next number from the previous can be obtained.
ÌıExample
Ìı1. Find the next term from the sequence
ÌıÌı • 2, 7, 12, 17, 22, 27, 32
The next term is 37.
2. Given the sequence
ÌıÌı • 2, 4, 6, 8, 10, 12………Chapter1.integer s.y.b.c.s



Chapter1.integer s.y.b.c.svidyabhoge1
Ìı
This document discusses the key concepts from several units in mathematics including integers, groups, finite groups, subgroups, and groups in coding theory. It then provides details on specific topics within these units, including equivalence relations, congruence relations, equivalence class partitions, the division algorithm, greatest common divisors (GCD) using division, and Euclid's lemma. The document aims to provide students with fundamental mathematical principles, methods, and tools to model, solve, and interpret a variety of problems. It also discusses enhancing students' development, problem solving skills, communication, and attitude towards mathematics.Arithmetic progressions



Arithmetic progressionsPrakashChinchali
Ìı
The document defines arithmetic progressions and provides examples. Some key points:
- An arithmetic progression is a list of numbers where each term is obtained by adding a fixed number (called the common difference) to the preceding term.
- The general formula for the nth term of an AP is an = a + (n-1)d, where a is the first term and d is the common difference.
- Examples show how to determine if a list of numbers forms an AP, write the next terms, and find a specific term by using the general formula.
- There are finite APs, which have a last term, and infinite APs, which go on forever without a last term.(677528443) neethu text (2)



(677528443) neethu text (2)neethumaths
Ìı
This document provides an introduction to sequences and series. It begins with definitions of sequences, series, arithmetic progressions, geometric progressions and their key terms. It then presents several examples of finding terms in arithmetic and geometric progressions. The document also defines arithmetic mean and geometric mean, and discusses how to find the sum of terms in an arithmetic progression using formulas. Overall, the document serves as an introductory guide to common concepts involving sequences and progressions.Maths05



Maths05sansharmajs
Ìı
The document provides solutions to questions from an IIT-JEE mathematics exam. It includes 8 questions worth 2 marks each, 8 questions worth 4 marks each, and 2 questions worth 6 marks each. The solutions solve problems related to probability, trigonometry, geometry, calculus, and loci. The summary focuses on the high-level structure and content of the document.Binomial



BinomialDEDESUDJADI
Ìı
The document provides an introduction to the binomial theorem. It begins by discussing binomial coefficients through the Pascal's triangle. It then derives an explicit formula for binomial coefficients using factorials. Finally, it states the binomial theorem and provides examples of using it to expand algebraic expressions and estimate numerical values.Imc2017 day1-solutions



Imc2017 day1-solutionsChristos Loizos
Ìı
This document contains solutions to 5 problems posed at the IMC 2017 conference. The solutions are summarized as follows:
1) The possible eigenvalues of the matrix A described in Problem 1 are 0, 1, and -1±√3i/2.
2) Problem 2 proves that for any differentiable function f satisfying the Lipschitz condition, f(x)2 < 2Lf(x) for all x.
3) Problem 3 shows that for any set S subset of {1,2,...,2017}, there exists an integer n such that the sequence ak(n) defined in the problem satisfies the property that ak(n) is a perfect square if and only if k isExtension 1 miscellaneous worksheet



Extension 1 miscellaneous worksheetAndrew Rybkow
Ìı
This document contains 10 math problems involving trigonometric functions, coordinate geometry, and calculus. The problems cover topics like expressing trig functions in alternate forms, proving trigonometric identities, finding equations of lines tangent to parabolas and cubic functions, solving triangles given side lengths and angles, and dividing line segments in a given ratio.Ijetr011923



Ijetr011923ER Publication.org
Ìı
ER Publication,
IJETR, IJMCTR,
Journals,
International Journals,
High Impact Journals,
Monthly Journal,
Good quality Journals,
Research,
Research Papers,
Research Article,
Free Journals, Open access Journals,
erpublication.org,
Engineering Journal,
Science Journals,
Binomial theorem for any index



Binomial theorem for any indexindu psthakur
Ìı
The document summarizes the binomial theorem and properties of binomial coefficients. It provides:
1) The binomial theorem expresses the expansion of (a + b)n as a sum of terms involving binomial coefficients for any positive integer n.
2) Important properties of binomial coefficients are discussed, such as their relationship to factorials and the symmetry of coefficients.
3) Examples are given of using the binomial theorem to find coefficients and solve problems involving divisibility and series of binomial coefficients.More from Ashwani Jha (6)
PHY XII TEST PAPER.pdf



PHY XII TEST PAPER.pdfAshwani Jha
Ìı
This document contains the answers to multiple choice questions related to concepts in electrostatics. The answers reference concepts like Gauss's law, electric field, capacitance, and electric potential. For each question, the response provides a brief 1-2 sentence explanation for the reasoning behind the selected multiple choice answer.PHY XII TEST PAPERQ.pdf



PHY XII TEST PAPERQ.pdfAshwani Jha
Ìı
1. The document contains 14 multiple choice questions about concepts in electrostatics such as electric fields, electric flux, Gauss's law, capacitors, and electric potential.
2. The questions cover topics like determining the direction of an electric field, calculating electric flux through surfaces, comparing stored electrostatic energy in capacitors, and analyzing diagrams of electric field configurations.
3. Many questions ask the examinee to apply concepts like Gauss's law to solve for electric fields or fluxes in situations involving conducting and non-conducting charged objects.Advanced Support Vector Machine for classification in Neural Network



Advanced Support Vector Machine for classification in Neural NetworkAshwani Jha
Ìı
This paper proposes a method to classify EEG signals using a support vector machine (SVM) classifier combined with a quicksort sorting algorithm (SVM-Q). The method involves extracting phase locking value (PLV) features from EEG data, sorting the feature vectors using quicksort, and then applying SVM classification. When tested on two BCI competition datasets, SVM-Q achieved classification accuracies of 86% and 77%, outperforming plain SVM which achieved 62% and 54% respectively. The paper concludes that adding a sorting algorithm to SVM can improve its classification performance for brain-computer interface applications.FEM problem of elasticity



FEM problem of elasticityAshwani Jha
Ìı
(1) The document solves for the approximate deflection of a simply supported beam carrying a symmetrical triangular load P using the Rayleigh Ritz method.
(2) It models the deflection w(x) and derives equations for the total potential energy Î and moment of inertia I2 in terms of w(x).
(3) By taking the derivative of Î with respect to the constants C1 and C2 and setting them to zero, it arrives at the solution for the deflection w(x) = (Pl^3/48EI)(4x^3/l^3 - 3x^2/l^2) which matches the exact solution.K nn



K nnAshwani Jha
Ìı
K-Nearest Neighbor (KNN) is an instance-based learning algorithm where classification of new data points is based on the majority class of its k nearest neighbors. It works by storing all training examples and classifying new examples based on the majority class of its nearest neighbors, where distance between examples is measured using a metric like Euclidean distance. KNN can perform both classification and regression tasks, with classification being the majority class for discrete targets and regression being the average of the k nearest neighbors' values for continuous targets.Dataset ii ia



Dataset ii iaAshwani Jha
Ìı
This document summarizes an EEG dataset containing motor imagery trials from 4 classes: left hand, right hand, foot, and tongue. The dataset contains recordings from 3 subjects and includes 60 channels of EEG data across 60 trials per class. The data is provided in MATLAB format in two tables: S, containing the EEG signal data, and HDR, containing metadata about the data in S including trial onset times and class labels. The document describes how to segment the continuous EEG data in S into separate trials based on the information in HDR to create a segregated 4-class dataset for analysis.Recently uploaded (20)
Effective Product Variant Management in Odoo 18



Effective Product Variant Management in Odoo 18Celine George
Ìı
In this slide we’ll discuss on the effective product variant management in Odoo 18. Odoo concentrates on managing product variations and offers a distinct area for doing so. Product variants provide unique characteristics like size and color to single products, which can be managed at the product template level for all attributes and variants or at the variant level for individual variants.How to create security group category in Odoo 17



How to create security group category in Odoo 17Celine George
Ìı
This slide will represent the creation of security group category in odoo 17. Security groups are essential for managing user access and permissions across different modules. Creating a security group category helps to organize related user groups and streamline permission settings within a specific module or functionality.How to Configure Deliver Content by Email in Odoo 18 Sales



How to Configure Deliver Content by Email in Odoo 18 SalesCeline George
Ìı
In this slide, we’ll discuss on how to configure proforma invoice in Odoo 18 Sales module. A proforma invoice is a preliminary invoice that serves as a commercial document issued by a seller to a buyer.Full-Stack .NET Developer Interview Questions PDF By ScholarHat



Full-Stack .NET Developer Interview Questions PDF By ScholarHatScholarhat
Ìı
Full-Stack .NET Developer Interview Questions PDF By ScholarHatBISNIS BERKAH BERANGKAT KE MEKKAH ISTIKMAL SYARIAH



BISNIS BERKAH BERANGKAT KE MEKKAH ISTIKMAL SYARIAHcoacharyasetiyaki
Ìı
BISNIS BERKAH BERANGKAT KE MEKKAH ISTIKMAL SYARIAHAzure Data Engineer Interview Questions By ScholarHat



Azure Data Engineer Interview Questions By ScholarHatScholarhat
Ìı
Azure Data Engineer Interview Questions By ScholarHatHannah Borhan and Pietro Gagliardi OECD present 'From classroom to community ...



Hannah Borhan and Pietro Gagliardi OECD present 'From classroom to community ...EduSkills OECD
Ìı
Hannah Borhan, Research Assistant, OECD Education and Skills Directorate and Pietro Gagliardi, Policy Analyst, OECD Public Governance Directorate present at the OECD webinar 'From classroom to community engagement: Promoting active citizenship among young people" on 25 February 2025. You can find the recording of the webinar on the website https://oecdedutoday.com/webinars/
Functional Muscle Testing of Facial Muscles.pdf



Functional Muscle Testing of Facial Muscles.pdfSamarHosni3
Ìı
Functional Muscle Testing of Facial Muscles.pdfAdministrative bodies( D and C Act, 1940



Administrative bodies( D and C Act, 1940P.N.DESHMUKH
Ìı
These presentation include information about administrative bodies such as D.T.A.B
CDL AND DCC, etc.Interim Guidelines for PMES-DM-17-2025-PPT.pptx



Interim Guidelines for PMES-DM-17-2025-PPT.pptxsirjeromemanansala
Ìı
This is the latest issuance on PMES as replacement of RPMS. Kindly message me to gain full access of the presentation. AI and Academic Writing, Short Term Course in Academic Writing and Publicatio...



AI and Academic Writing, Short Term Course in Academic Writing and Publicatio...Prof. (Dr.) Vinod Kumar Kanvaria
Ìı
AI and Academic Writing, Short Term Course in Academic Writing and Publication, UGC-MMTTC, MANUU, 25/02/2025, Prof. (Dr.) Vinod Kumar Kanvaria, University of Delhi, vinodpr111@gmail.comYear 10 The Senior Phase Session 3 Term 1.pptx



Year 10 The Senior Phase Session 3 Term 1.pptxmansk2
Ìı
Year 10 The Senior Phase Session 3 Term 1.pptxBlind spots in AI and Formulation Science, IFPAC 2025.pdf



Blind spots in AI and Formulation Science, IFPAC 2025.pdfAjaz Hussain
Ìı
The intersection of AI and pharmaceutical formulation science highlights significant blind spots—systemic gaps in pharmaceutical development, regulatory oversight, quality assurance, and the ethical use of AI—that could jeopardize patient safety and undermine public trust. To move forward effectively, we must address these normalized blind spots, which may arise from outdated assumptions, errors, gaps in previous knowledge, and biases in language or regulatory inertia. This is essential to ensure that AI and formulation science are developed as tools for patient-centered and ethical healthcare.Dr. Ansari Khurshid Ahmed- Factors affecting Validity of a Test.pptx



Dr. Ansari Khurshid Ahmed- Factors affecting Validity of a Test.pptxKhurshid Ahmed Ansari
Ìı
Validity is an important characteristic of a test. A test having low validity is of little use. Validity is the accuracy with which a test measures whatever it is supposed to measure. Validity can be low, moderate or high. There are many factors which affect the validity of a test. If these factors are controlled, then the validity of the test can be maintained to a high level. In the power point presentation, factors affecting validity are discussed with the help of concrete examples.ASP.NET Web API Interview Questions By Scholarhat



ASP.NET Web API Interview Questions By ScholarhatScholarhat
Ìı
ASP.NET Web API Interview Questions By ScholarhatOral exam Kenneth Bech - What is the meaning of strategic fit?



Oral exam Kenneth Bech - What is the meaning of strategic fit?MIPLM
Ìı
Presentation of the CEIPI DU IPBA oral exam of Kenneth Bech - What is the meaning of strategic fit? Helping Autistic Girls Shine Webinar ºİºİߣs



Helping Autistic Girls Shine Webinar ºİºİߣsPooky Knightsmith
Ìı
For more information about my speaking and training work, visit: https://www.pookyknightsmith.com/speaking/AI and Academic Writing, Short Term Course in Academic Writing and Publicatio...



AI and Academic Writing, Short Term Course in Academic Writing and Publicatio...Prof. (Dr.) Vinod Kumar Kanvaria
Ìı
MATHEMATICS XI TEST PAPER SOL.pdf
- 1. Roll No. : Date : Time - MM - 70 2 Ans : 4 2. Ans : 4 3. Ans : 4 4. Ans : Find the number of terms in the expansion of (a + 2b – 3c)n. Consider (a + 2b – 3c)n = {a + (2b – 3c)}n = nC0 an + nC1 an–1(2b – 3c) + nC2 an–2(2b – 3c)2 + ... + nCn(2b – 3c)n. We notice first term gives one term, second term gives two terms, third term gives three terms and so on. ∴ Total terms 1 + 2 + ... + n + (n + 1) = Find the middle term(s) in the expansion of : (1 + x)2n Since, 2n would always be even so (n + 1)th term would be the middle term ∴ The coefficients of 2nd, 3rd and 4th terms in the expansion of (1 + x)2n are in A.P., prove that 2n2 – 9n + 7 = 0 2nC1, 2nC2, 2nC3 are in A.P. ⇒ 2. 2nC2 = 2nC1 + 2nC3 ⇒ ⇒ 2n2 – 9n + 7 = 0 If Tr is rth term in the expansion of (1 + x)n in the ascending powers of x, prove that r (r + 1) Tr+2 = (n – r + 1) (n – r)x2 Tr. LHS = r (r + 1) Tr+2 = r (r + 1). nCr+1 xr+1 = r (r + 1) = RHS = (n – r + 1) (n – r) x2 Tr = (n – r + 1) (n – r)x2. nCr–1 xr–1 = (n – r + 1) (n – r). x2 . = LHS = RHS
- 2. 4 5. Ans : 4 6. If the 3rd, 4th, 5th and 6th terms in the expansion of (x + y)n be a, b, c and d respectively, prove that If a, b, c be the three consecutive coefficients in the expansion of (1 + x)n, prove that n =
- 3. Ans : 4 7. Ans : 4 8. Using binomial theorem, prove that 6n – 5n always leaves remainder 1 when divided by 25. For two numbers a and b if we can find numbers q and r such that a = bq + r, then we say that b divides a with q as quotient and r as remainder. Thus, in order to show that 6n – 5n leaves remainder 1 when divided by 25, we prove that 6n – 5n = 25k + 1, where k is some natural number. We have (1 + a)n = nC0 + nC1a + nC2a2 + ... nCnan For a = 5, we get (1 + 5)n = nC0 + nC15 + nC252 + ... nCn5n i.e. (6)n = 1 + 5n + 52 nC2 + 53 nC3 + ... + 5n i.e. 6n – 5n = 1 + 52 (nC2 + nC3 5 + ... + 5n–2) or 6n – 5n = 1 + 25 (nC2 + 5. nC3 + ... + 5n–2) or 6n – 5n = 25k + 1 where k = nC2 + 5. nC3 + ... + 5n–2 This shows that when divided by 25, 6n – 5n leaves remainder 1. If a1, a2, a3 and a4 are the coefficient of any four consecutive terms in the expansion of (1 + x)n, prove that
- 4. Ans : 4 9. Let a1, a2, a3 and a4 be the coefficient of four consecutive terms Tr+1, Tr+2, Tr+3 and Tr+4 respectively. Then a1 = coefficient of Tr+1 = nCr a2 = coefficient of Tr+2 = nCr+1 a3 = coefficient of Tr+3 = nCr+2 and a4 = coefficient of Tr+4 = nCr+3 Find the sum (33 – 23) + (53 – 43) + (73 – 63) + ... 10 terms.
- 5. Ans : 4 10. Ans : 4 11. Ans : 4 12. Sum = (33 + 53 + 73 + ...) – (23 + 43 + 63 + ...) For 33 + 53 + 73 + ... an = (2n + 1)3 = 8n3 + 12n2 + 6n + 1 ∴ S1 = 8 Σn3 + 12 Σn2 + 6 Σn + n ... (i) For 23 + 43 + 63 + ... an = (2n)3 = 8n3 S2 = 8Σn3 ... (ii) ∴ Sum = S1 – S2 = 12 Σn2 + 6 Σn + n [From (i), (ii)] Sum to 10 terms = = 4620 + 330 + 10 = 4960 If a, b, c be the first, third and nth term respectively of an A.P. Prove that the sum to n terms is Given first term = a, a3 = b and an = c Sn = [2a + (n – 1)d] ... (i) We have first term = a b = a + 2d and a + (n – 1) d = c Sn = [a + c] [From (i)] ... (ii) Also a + (n – 1) = c ⇒ n – 1 = Substituting in (ii), we get Sn = If a, b, c are in G.P and . Prove that x, y, z are in A.P. ⇒ a = kx, b = ky, c = kz Given b2 = ac ⇒ (ky)2 = kx. kz ⇒ k2y = kx + z ⇒ 2y = x + z ⇒ x, y, z in A.P. If a1, a2, a3, ... an are in A.P., where ai > 0 for all i, show that =
- 6. Ans : 4 13. The ratio of the A.M. and G.M. of two positive numbers a and b is m : n, show that:
- 7. Ans : 4 14. Given A.M. : G.M. = m : n If p, q, r are in G.P. and the equations px2 + 2qx + r = 0 and dx2 + 2ex + f = 0 have a common root then show that are in A.P.
- 8. Ans : 6 15. Ans : 4 16. Given p, q, r are in G.P. ⇒ q2 = pr …(i) Equations px2 + 2qx + r = 0 and dx2 + 2ex + f = 0 have common root …(ii) Substituting for q, from (i) in px2 + 2qx + r = 0 Find the sum of, + .......................... up to n terms. If S1, S2, S3, ......, Sm are the sums of n terms of m A.P.'s whose first terms are 1, 2, 3, ......, m and common differences are 1, 3, 5, ......, 2m – 1 respectively, show that S1 + S2 + S3 + ...... + Sm =
- 9. Ans : 6 17. Ans : mn (mn + 1) S1 = Σ(1, 2, 3..............) = [2.1 + (n – 1).1] S2 = Σ(2, 5, 8..............) = [2.2 + (n – 1).3] S3 = Σ(3, 8, 13............) = [2.3 + (n – 1).5] Sm = [2.m + (n – 1) (2m – 1)] S1 + S2 + ...... + Sm = [2 (1 + 2 + 3.......m) + (n – 1) (1 + 3 + 5........+ 2m – 1)] = = If A and G be A.M. and G.M., respectively between two positive numbers, prove that the numbers are A ± . Let the numbers be a and b. = A ⇒ a + b = 2A ...(i) = G ⇒ ab = G2 (a – b)2 = (a + b)2 – 4ab = 4A2 – 4G2 ⇒ 4(A2 – G2) a – b = ...(ii) From (i) and (ii), we get a = A + and b = A – Hence, the numbers are A ±




