Measures of Variability
Download as PPTX, PDF
1 like816 views
The document provides formulas for calculating measures of variation such as variance, standard deviation, and quartile deviation. It includes an example using data on class intervals and frequencies to calculate the mean, median, mode, variance, standard deviation, first quartile, third quartile, and quartile deviation.
Measures of Variability
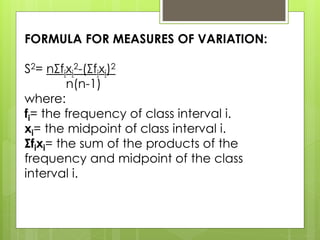
- 1. FORMULA FOR MEASURES OF VARIATION: S2= n裡fixi 2-(裡fixi)2 n(n-1) where: fi= the frequency of class interval i. xi= the midpoint of class interval i. 裡fixi= the sum of the products of the frequency and midpoint of the class interval i.
- 2. THE QUARTILE DEVIATION: The quartile deviation is used when the median is used as an average; when the data depart noticeably from the normal. It is used for ordinal data. The quartile deviation, Q, is frequently called the semi-interquartile range. It is half of the distance between two quartile points, Q1, and Q3.
- 3. FORMULA FOR QUARTILE DEVIATION: Q= Q3-Q1 2 Q1= L1+ (n/4 cf)c f Q3= L1+(3n/4 cf)c f
- 4. EXAMPLE: Given the following data, Class interval f 36- 40 2 31- 35 8 26- 30 12 21- 25 18 16- 20 10
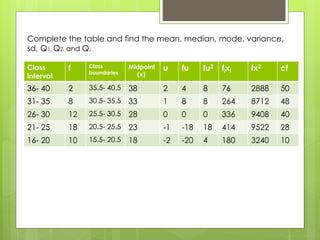
- 5. Complete the table and find the mean, median, mode, variance, sd, Q1, Q2, and Q. Class interval f Class boundaries Midpoint (x) u fu fu2 fixi fx2 cf 36- 40 2 35.5- 40.5 38 2 4 8 76 2888 50 31- 35 8 30.5- 35.5 33 1 8 8 264 8712 48 26- 30 12 25.5- 30.5 28 0 0 0 336 9408 40 21- 25 18 20.5- 25.5 23 -1 -18 18 414 9522 28 16- 20 10 15.5- 20.5 18 -2 -20 4 180 3240 10
- 6. MEAN= 裡fi Xi = 1270 = 25.4 n 50 MEDIAN= L1+ (n/2-cf)c = 20.5+ 50/2 -10 (5) = 24.67 f 18 MODE= L1+ (d1)c = 20.5+ _8_ (5)= 23.36 d1+d2 8+6 2-(裡fixi)2 = 50(33770)-(1270) 2 n(n-1) 50(49) VARIANCE= n裡fixi = 1688500-1612900 2450 = 75600 = 30.86 2450
- 7. S= . QUARTILE DEVIATION Q1= L1+ (n/4 cf)c = 20.5 + (50/4 10)5 f 18 = 20.5 + 0.69= 21.19 Q3= L1+(3n/4 cf)c = 25.5 + (3/4 (50) 28) 5 f 12 = 25.5 + 3. 96 = 29. 46 Q= Q3-Q1 = 29. 46- 21. 19 = 4. 135 2 2