Measuring Segments and Coordinate Plane
Download as PPTX, PDF
5 likes8,955 views
This slideshow was used to introduce application of Segment Addition Postulate along with Coordinate Plane in Geometry. There is a review of several concepts at the end of the two lessons.
Measuring Segments and Coordinate Plane
- 1. 1.4: Measuring Segments and AnglesPrentice Hall Geometry
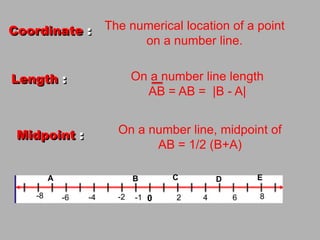
- 2. CEABD0-88-2-4-1246-6The numerical location of a point on a number line.Coordinate :On a number line length AB = AB = |B - A|Length :On a number line, midpoint of AB = 1/2 (B+A) Midpoint :
- 3. Find which two of the segments XY, ZY, and ZW are congruent. Because XY = ZW, XYZW.Measuring Segments and AnglesGEOMETRY LESSON 1-4Find the length of each segment.XY = | 5 (1)| = | 4| = 4ZY = | 2 (1)| = |3| = 3ZW = | 2 6| = |4| = 4
- 4. The Segment Addition PostulateIf three points A, B, and C are collinear and B is between A and C, then AB + BC = AC.ABC
- 5. AN = 2x 6 = 2(8) 6 = 10NB = x + 7 = (8) + 7 = 15Substitute 8 for x.If AB = 25, find the value of x. Then find AN and NB.Use the Segment Addition Postulate to write an equation.AN + NB = ABSegment Addition Postulate(2x 6) + (x + 7) = 25 Substitute.3x + 1 = 25 Simplify the left side. 3x = 24 Subtract 1 from each side.x = 8 Divide each side by 3.AN = 10 and NB = 15, which checks because the sum of the segment lengths equals 25.
- 6. RM = 5x + 9 = 5(15) + 9 = 84 MT = 8x 36 = 8(15) 36 = 84Substitute 15 for x.RM and MT are each 84, which is half of 168, the length of RT.Mis the midpoint of RT. Find RM, MT, and RT.Use the definition of midpoint to write an equation.RM = MTDefinition of midpoint5x + 9 = 8x 36Substitute.5x + 45 = 8xAdd 36 to each side. 45 = 3xSubtract 5x from each side. 15 = xDivide each side by 3.RT = RM + MT= 168
- 7. Quiz1. T is in between of XZ. If XT = 12 and XZ = 21, then TZ = ?2. T is the midpoint of XZ. If XT = 2x +11 and XZ = 5x + 8, find the value of x.
- 9. Parts of Coordinate Planey-axisQuadrant IIQuadrant I( - , + )( +, + )originx-axisQuadrant IIIQuadrant IV( - , - )( + , - )
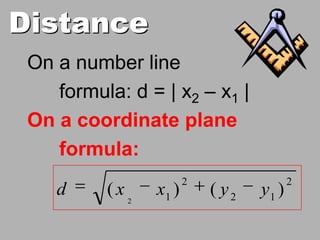
- 10. DistanceOn a number line formula: d = | x2 x1 |On a coordinate plane formula:
- 11. Find the distance between T(5, 2) and R( -4. -1) to the nearest tenth.
- 12. MidpointOn a number line formula: On a coordinate plane formula:
- 13. The midpoint of AB is M(3, 4). One endpoint is A(-3, -2). Find the coordinates of the other endpoint B.
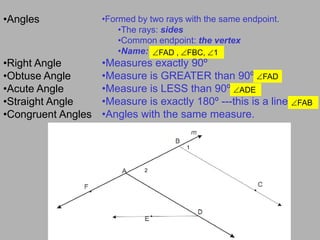
- 14. Angles
- 15. Formed by two rays with the same endpoint.
- 16. The rays: sides
- 17. Common endpoint: the vertex
- 18. Name:
- 20. Measure is GREATER than 90尊
- 21. Measure is LESS than 90尊
- 22. Measure is exactly 180尊 ---this is a line
- 23. Angles with the same measure.
- 24. Right Angle
- 25. Obtuse Angle
- 26. Acute Angle
- 27. Straight Angle
- 28. Congruent AnglesFAD , FBC, 1 FADADE FAB 12
- 29. Name the angle below in four ways.The name can be the number between the sides of the angle: The name can be the vertex of the angle: G.Finally, the name can be a point on one side, the vertex, and a point on the other side of the angle: AGC,CGA.3
- 30. Suppose that m 1 = 42 and m ABC = 88. Find m 2.Use the Angle Addition Postulate to solve.m 1 + m 2 = m ABCAngle Addition Postulate.42 + m 2 = 88Substitute 42 for m 1 and 88 for m ABC.m 2 = 46 Subtract 42 from each side.
- 31. Use the figure below for Exercises 1-3.1. If XT = 12 and XZ = 21, then TZ = 7.2. If XZ = 3x, XT = x + 3, and TZ = 13, find XZ.3. Suppose that T is the midpoint of XZ. If XT = 2x + 11 and XZ = 5x + 8, find the value of x. Use the figure below for Exercises 46.4. Name 2 two different ways. 5. Measure and classify 1, 2, and BAC.6. Which postulate relates the measures of 1, 2, and BAC?924DAB and BAD90属, right; 30属, acute; 120属, obtuse14Angle Addition Postulate
- 32. HomeworkPage 56 # 2, 4, 18, 20, 24, 26
- 33. REVIEW!Page 71 # 1- 16Page 72 # 19- 31Page 73 # 34- 38