Metric System Scientific Notation
- 1. Metric System & Scientific Notation Chemistry August 20 th /21 st , 2009
- 2. Metric System The metric system is based on a base unit that corresponds to a certain kind of measurement Length = meter Volume = liter Weight (Mass) = gram Prefixes plus base units make up the metric system Example: Centi + meter = Centimeter Kilo + liter = Kiloliter
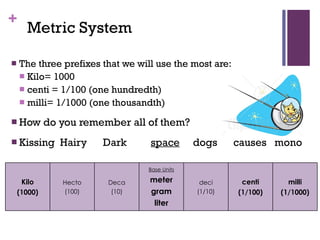
- 3. Metric System The three prefixes that we will use the most are: Kilo= 1000 centi = 1/100 (one hundredth) milli= 1/1000 (one thousandth) How do you remember all of them? Kissing Hairy Dark space dogs causes mono Kilo (1000) Hecto (100) Deca (10) Base Units meter gram liter deci (1/10) centi (1/100) milli (1/1000)
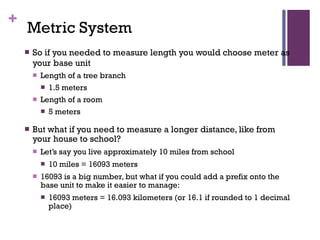
- 4. Metric System So if you needed to measure length you would choose meter as your base unit Length of a tree branch 1.5 meters Length of a room 5 meters But what if you need to measure a longer distance, like from your house to school? LetŌĆÖs say you live approximately 10 miles from school 10 miles = 16093 meters 16093 is a big number, but what if you could add a prefix onto the base unit to make it easier to manage: 16093 meters = 16.093 kilometers (or 16.1 if rounded to 1 decimal place)
- 5. Typical Metric Units What metric unit would you use to measure the length of the room? What metric unit would you use to measure the distance between the mall and school? What metric unit would you use to measure your weight? What metric unit would you use to measure the amount of liquid in a soda bottle? What unit would you use to measure the amount of liquid in an eye dropper?
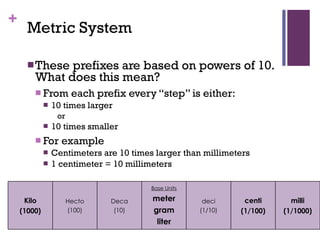
- 6. Metric System These prefixes are based on powers of 10. What does this mean? From each prefix every ŌĆ£stepŌĆØ is either: 10 times larger or 10 times smaller For example Centimeters are 10 times larger than millimeters 1 centimeter = 10 millimeters Kilo (1000) Hecto (100) Deca (10) Base Units meter gram liter deci (1/10) centi (1/100) milli (1/1000)
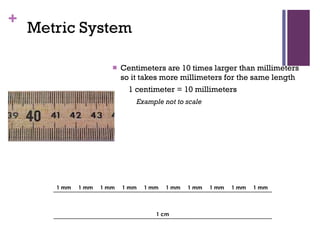
- 7. Metric System Centimeters are 10 times larger than millimeters so it takes more millimeters for the same length 1 centimeter = 10 millimeters Example not to scale 1 cm 40 41 41 40 1 mm 1 mm 1 mm 1 mm 1 mm 1 mm 1 mm 1 mm 1 mm 1 mm
- 8. Metric System For each ŌĆ£stepŌĆØ to right, you are multiplying by 10 For example, letŌĆÖs go from a base unit to centi 1 liter = 10 deciliters = 100 centiliters 2 grams = 20 decigrams = 200 centigrams ( 1 x 10 = 10) = (10 x 10 = 100) (2 x 10 = 20) = (20 x 10 = 200) Kilo (1000) Hecto (100) Deca (10) Base Units meter gram liter deci (1/10) centi (1/100) milli (1/1000)
- 9. Metric System An easy way to move within the metric system is by moving the decimal point one place for each ŌĆ£stepŌĆØ desired Example: change meters to centimeters 1 meter = 10 decimeters = 100 centimeters or 1.00 meter = 10.0 decimeters = 100. centimeters Kilo (1000) Hecto (100) Deca (10) Base Units meter gram liter deci (1/10) centi (1/100) milli (1/1000)
- 10. Metric System Now letŌĆÖs try our previous example from meters to kilometers: 16093 meters = 1609.3 decameters = 160.93 hectometers = 16.093 kilometers So for every ŌĆ£stepŌĆØ from the base unit to kilo, we moved the decimal 1 place to the left (the same direction as in the diagram below) Kilo (1000) Hecto (100) Deca (10) Base Units meter gram liter deci (1/10) centi (1/100) milli (1/1000)
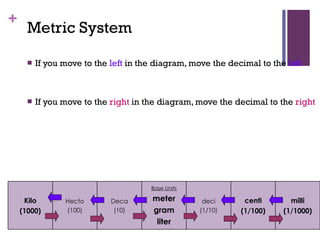
- 11. Metric System If you move to the left in the diagram, move the decimal to the left If you move to the right in the diagram, move the decimal to the right Kilo (1000) Hecto (100) Deca (10) Base Units meter gram liter deci (1/10) centi (1/100) milli (1/1000)
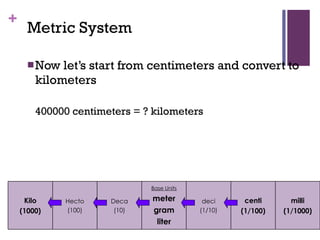
- 12. Metric System Now letŌĆÖs start from centimeters and convert to kilometers 400000 centimeters = ? kilometers Kilo (1000) Hecto (100) Deca (10) Base Units meter gram liter deci (1/10) centi (1/100) milli (1/1000)
- 13. Metric System Now letŌĆÖs start from meters and convert to centimeters 5 meters = ? centimeters Now letŌĆÖs start from kilometers and convert to meters .3 kilometers = ? meters Kilo (1000) Hecto (100) Deca (10) Base Units meter gram liter deci (1/10) centi (1/100) milli (1/1000) Kilo (1000) Hecto (100) Deca (10) Base Units meter gram liter deci (1/10) centi (1/100) milli (1/1000)
- 14. Metric System Summary Base units in the metric system are meter, liter, gram Metric system is based on powers of 10 For conversions within the metric system, each ŌĆ£stepŌĆØ is 1 decimal place to the right or left Using the diagram below, converting to the right, moves the decimal to the right and vice versa Kilo (1000) Hecto (100) Deca (10) Base Units meter gram liter deci (1/10) centi (1/100) milli (1/1000)
- 15. Scientific Notation Scientific notation is a way of expressing really big numbers or really small numbers. For very large and very small numbers, scientific notation is more concise. Scientific Notation always has two parts: A number between 1 and 9.9999ŌĆ” A power of 10 N x 10 x
- 16. Writing Scientific Notation Place the decimal point so that there is one non-zero digit to the left of the decimal point. Count the number of decimal places the decimal point has ŌĆ£movedŌĆØ from the original number. This will be the exponent on the 10. If the original number was less than 1, then the exponent is negative. If the original number was greater than 1, then the exponent is positive. Example: Given: 289,800,000 Start with: 2.898 Decimal needs to move 8 places to the right Answer: 2.898 x 10 8
- 17. Try this: Given: 0.000567 Start with: Decimal needs to move: Answer:
- 18. Change Scientific Notation back to Standard Form Simply move the decimal point to the right for positive exponent 10. Move the decimal point to the left for negative exponent 10. (Use zeros to fill in places.) Example: Given: 5.093 x 10 6 Move: 6 places to the right (positive) Answer: 5,093,000
- 19. Try This: Given: 1.976 x 10 -4 Move: Answer: