Some facts about oxygen diffusion in porous systems
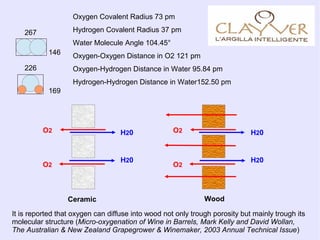
- 1. Oxygen Covalent Radius 73 pm Hydrogen Covalent Radius 37 pm Water Molecule Angle 104.45° Oxygen-Oxygen Distance in O2 121 pm Oxygen-Hydrogen Distance in Water 95.84 pm Hydrogen-Hydrogen Distance in Water152.50 pm 169 146 267 226 H20 H20 O2 O2 H20 H20 O2 O2 WoodCeramic It is reported that oxygen can diffuse into wood not only trough porosity but mainly trough its molecular structure (Micro-oxygenation of Wine in Barrels, Mark Kelly and David Wollan, The Australian & New Zealand Grapegrower & Winemaker, 2003 Annual Technical Issue)
- 2. R H y V=225 l Let us use the Darcy formula to calculate the flow rate of a fluid trough a porous cylinder simulating a barrique Q( y)= kA μ Δ P( y) L k=permeability (m2 ) (*) ? liquid viscosity and P hydraulic pressure L dQ (y)= k2π Rdy μ ρgy L Q( y)= 2πRk ρg μ L ∫0 H ydy if K = k ρ g μ Q= K π RH 2 L (side)+ K πR 2 H L (bottom) Kmax(cemento)=7.7°10 ?13 m/sec Kmax(claybrick)=3.8°10 ?9 m/ sec If R= 0.267m H=1 m L=2.7 cm (values typical of a barrique) Qconcrete=10 ?4 l /h Qclay brick=0.54l /h (*) For packed particles porous systems it is found that k=Cd2 where d is the particle radius and C a geometrical constant. In these systems d is proportional to pore dimensions. (**) K takes into account the characteristics of both the porous media and the fluid flowing through. (***) The University of Edinburgh, Division of Engineering, Session 2001-2002, Materials Science and Engineering K=Hydraulic Conductivity (m/sec) (**) (***) For the Barrique side In a clayware system the fluid loss can be very high
- 3. Let us try to calculate oxygen flow through a porous clayware barrique using Fick diffusion equation ΦO2=Deff ΔCO2 Δ X Deff =DH20 O2 εδ τ 2 1lO2 =1.43g ? = Pores constrictivity ~ 0.7 ??= Pores tortuosity ~ 1.4 ? = Porosity ~ 0.2 They are typical values for a clayware brick (*) DO2 H20 = 2*10-9 m2 /sec Deff ~ 7*10-11 m2 /sec CO2 = 0 Inside wine CO2 = 7.2 mg/l On the external saturated surface Barrique thickness = 2.7 cm Barrique surface ~ 2 m2 Barrique volume = 225 l ?O2 ~0.6 mlO2 /litre/month The usual value for a oak wood barrel is 2.2 mlO2 /litre/month (**) Therefore is possible that a porous system let a small oxygen go in but a lot of fluid go out!! (*) M.Raimondo, M.Dondi, D.Gardini, G.Guarini, F.Mazzanti, Predicting the initial rate of water absorption in clay bricks, Construction and Building Materials, 23 (2009) 2623-2630. (**) Micro-oxygenation of Wine in Barrels, Mark Kelly and David Wollan, The Australian & New Zealand Grapegrower & Winemaker, 2003 Annual Technical Issue δ= √DMAX DMIN DAVERAGE = ?DGeometric ?DAritmetic ε= Vol.Holes Vol.Total τ= Covered ( A?B)distance (A?B)Gap A B DMAX DMIN
- 4. To calculate k and K we correlate Darcy and Poiseuille relations (open porosity systems) A L' 2r Q=∑ Qi Qi= π r 4 8μ Δ P L y Qi= π r 4 8μ ρ gy L If ? is the porosity, it comes out that the number of pores in a section A is n(pori) =?A/?r2 Q( y)= ε Ar 2 ρ gy 4μ L For a cylindrical container A=2?Rdy Q= εr 2 ρg π R 2μ L ∫0 H ydy But L=??L' and ???is the tortuosity Q= ρg επr 2 RH 2 4μ τ L' (side)+ ρgεπ r 2 R 2 H 4μ τ L' (bottom) But according to Darcy Because L'=L we finally have k= εr 2 4 τ with r average pore radius Q= K π RH 2 L (side)+ K πR 2 H L (bottom) After mercury porosimeter measurements we obtain for our Clayver
- 5. Dati tecnici caratteristici di Clayver Water absorption 2.1% Dry weight of 250 l Clayver 95 Kg Open porosity 5% Volume 250 l Bulk Density 2.34 g/cm3 Average pore diameter 0.04 ?m Constrictivity 0.76 Permeability 4.60?10-18 m2 Hydraulic conductivity 2.50?10-11 m/sec O2 flow rate 0.2 mlO2 /litre/month (Barrique ratio) ~0.1 Hydraulic loss 0.0035 l/h (initial stage)