Mom lab revised
Download as DOCX, PDF1 like428 views
The document describes a tensile test experiment conducted to determine the mechanical properties of mild steel. The experiment involved applying a tensile load to a mild steel specimen and measuring its elongation. Key results were: 1) The specimen necked at a load of just over 8kN, exceeding its elastic limit. 2) The maximum load of 12kN caused necking in the specimen. 3) The specimen fractured at a load of 8.9kN after continued elongation beyond the maximum load. 4) Results from the experiment matched the expected mechanical behavior of mild steel under tension, validating the initial hypothesis.
1 of 8
Download to read offline
![Polytechnic of Namibia
Fluid Mechanics 610S1
Bernoulliâs Experiment
[Type here]
9/11/2013
Group members
1. E.J.N Conceicao 212086561
2.
3.
4.
5.
6.
7.
8.
9.
10.
Lecturer: Mr Okorie Maduako E. B.Eng. (Mech.), M.
Eng.(Mech.)
Lab. Technician: Isabel Bishi (Mrs), B Tech, Mech. Eng.](https://image.slidesharecdn.com/momlabrevised-141031050637-conversion-gate01/85/Mom-lab-revised-1-320.jpg)




Recommended
Tensile test newyh



Tensile test newyhYanie Hadzir
Ėý
The experiment involves tensile testing of materials using an Instron load frame and BlueHill data acquisition software. Four materials - 6061-T6 aluminum alloy, A-36 hot rolled steel, PMMA, and polycarbonate - were tested with cylindrical specimens containing a reduced gage section. Testing was conducted according to ASTM standards. The data gathered was used to calculate properties like elastic modulus, yield strength, and ultimate tensile strength, which were plotted on stress-strain curves. The purpose was to determine key mechanical properties of each material and familiarize students with tensile testing procedures.Group presentation for tensile testing a4



Group presentation for tensile testing a4Prajwal Vc
Ėý
The document describes the methodology, instrumentation, and results of tensile testing experiments on various metal samples. The methodology involved determining sample dimensions, marking intervals on the samples, setting up the tensile testing equipment which includes an extensometer and Hounsfield testing machine, applying and increasing load while recording extension and results. Graphs of stress-strain responses were produced for aluminum, brass, and mild steel samples, showing their different mechanical behaviors under tensile loading. Key properties of each metal were also discussed.Testing Machine- universal tester



Testing Machine- universal testerAshita Agrawal
Ėý
AĖýuniversal testing machine, also known as aĖýuniversal tester, materials testing machineĖýorĖýmaterials test frame, is used toĖýtestĖýtheĖýtensile stressĖýandĖýcompressive strengthĖýofĖýmaterials.TENSILE TEST REPORT



TENSILE TEST REPORTmusadoto
Ėý
In the material testing laboratory, Tensile test was done on a mild steel specimen as figure 4 to identify the youngâs modulus, ultimate tensile strength, yield strength and percentage elongation. The results were as table 1Universal testing machines



Universal testing machinesShivam Tiwari
Ėý
The document discusses tensile testing procedures and principles. It outlines the components of a universal testing machine used for tensile testing, including the load frame, load cell, crosshead, and output devices. It then provides details on conducting a tensile test, including specimen preparation, loading procedure, and data collection. Examples are given demonstrating how load and elongation data can be used to determine material properties and how finite element analysis can simulate the effects of strain hardening.Universal Testing Machine



Universal Testing MachineAshish Kholia
Ėý
The document proposes the design and development of a universal testing machine with a load capacity of 0.3kN. The applicants, Aashish Kholiya and Ravi Teja, are undergraduate students at IIT Ropar who are undertaking this project under the guidance of Dr. Dhiraj Mahajan. They plan to design components like the ball screw, base plate, select appropriate sensors and a stepper motor. Their objectives are to develop a low-cost machine with high accuracy. They have conducted preliminary work on component design and sensor selection. A work plan with timeline is also provided to complete the project in 6 months. If approved, the project will be executed at IIT Ropar.UTM



UTMmaneeb
Ėý
A universal testing machine is used to perform tensile, compressive, and shear strength tests on materials and structures. It consists of a loading unit, control panel, and control devices. The document outlines the components, working principle, and procedures for conducting compressive, tensile, and shear tests using a universal testing machine. Specimens are gripped firmly and loaded incrementally until the yield point is reached, then the maximum load is recorded to determine the material's strength. Proper specimen dimensions and a uniform loading rate without jerks are important to get accurate test results. torsion testing machine



torsion testing machineShantaEngg Rege
Ėý
Shanta Engineering is incepted in the year 1978. We are one of the leading manufacturers and exporters of a wide range of products. We manufacture a wide range of Testing Instruments and Equipment's that are used to test rubber, plastic, cables and allied materials. Our product range includes Laboratory Testing Equipment, Cable Testing Instruments, Testing Machine and machines for agricultural application.
Our range of products are manufactured as per different standards used by our customers and also sometimes they are customized to satisfy special requirements of our customers.
With a group of experienced & talented personnel together our design & development of machine / instruments are at par with the imported testing equipment's on quality basis. Although we are offering the highest quality level to match with the imported Testing Equipment's, we always see to it that the cost of our all equipment's are affordable to a small budget buyers. We export our product to Indian Subcontinent country.Lab 8 tensile testing 



Lab 8 tensile testing elsa mesfin
Ėý
This document provides instructions for conducting a tensile test to determine the mechanical properties of polymers. A tensile test involves gripping a dogbone-shaped polymer specimen at both ends and pulling it at a constant rate until failure. Key points:
- Stress-strain curves are generated from the test, showing properties like elastic modulus, yield point, and toughness.
- Properties depend on factors like crystallinity, molecular weight, and glass transition temperature. Brittle polymers have steeper stress-strain curves.
- The test procedure involves preparing specimens to standards, setting up the tensile testing machine and software to control displacement rate and record data, calibrating load cells, gripping the specimen, and conducting the0 exp no.4 bending test experiment



0 exp no.4 bending test experimentMuhammed Fuad Al-Barznji
Ėý
Muhammed Fuad Rashid
Petroleum Engineering Department at Koya University
Mechanics of material Laboratory
2020Instron machine



Instron machineBrijesh Kumar Bajpai
Ėý
The document discusses an Instron machine, which is a versatile instrument used to measure load from 2 grams to 100 kgs using a strain gauge principle. It consists of a load cell containing metal beams with strain gauges that measure the resistance change when a load is applied. This allows the machine to generate a load-elongation curve on a chart. The Instron machine can test properties of yarns, cords, fibers and other materials under tension, and has applications in measuring tensile, flexural, friction and tear properties. It operates over a wide range of speeds and loads with interchangeable load cells and grips.ENGINEERING SYSTEM DYNAMICS-TAKE HOME ASSIGNMENT 2018



ENGINEERING SYSTEM DYNAMICS-TAKE HOME ASSIGNMENT 2018musadoto
Ėý
1. Read Chapter 4 â System Dynamics for Mechanical Engineers by Matthew Davies and Tony L. Schmitz and implement Examples 4.1 to 4.12 in Matlab.
2. Read Chapter 7 â System Dynamics for Mechanical Engineers by Matthew Davies and Tony L. Schmitz and implement Examples 7.1 to 7.11 in Matlab.
3. Read Chapter 9 â System Dynamics for Mechanical Engineers by Matthew Davies and Tony L. Schmitz and implement Examples 9.1 to 9.6 in Matlab.
4. Read Chapter 11 â System Dynamics for Mechanical Engineers by Matthew Davies and Tony L. Schmitz and implement Examples 11.1 to 11.7 in Matlab.
5. Read Chapter 2 - System Dynamics for Engineering Students: Concepts and Applications by Nicolae Lobontiu and attempt problem 2.18 (page 63).
6. Read Chapter 3 - System Dynamics for Engineering Students: Concepts and Applications by Nicolae Lobontiu and attempt problem 3.13 (pp 98 - 100).
7. Read Chapter 4 - System Dynamics for Engineering Students: Concepts and Applications by Nicolae Lobontiu and attempt problem 4.20 (page 146).
8. Read Chapter 5 - System Dynamics for Engineering Students: Concepts and Applications by Nicolae Lobontiu and attempt problems 5.15 (page 198), 5.21 (pp 199 - 200) and 5.27 (pp 201 â 202).
0 exp no.3 torsion



0 exp no.3 torsionMuhammed Fuad Al-Barznji
Ėý
Muhammed Fuad Rashid
Petroleum Engineering Department at Koya University
Mechanics of material Laboratory
2020exp: sample preparation for tensile test 



exp: sample preparation for tensile test Muhammed Fuad Al-Barznji
Ėý
This document describes an experiment on tensile testing of materials. It discusses preparing dog-bone shaped samples according to ASTM D638 standards. Tensile testing is done using a Shimadzu tensile testing machine to measure properties like stress and strain. Careful sample preparation and dimensions matching standards are needed to obtain accurate property values from the experiment. The conclusions emphasize getting the right sample dimension values according to standards to determine material properties correctly.Tensile



TensileAmmour Omrane
Ėý
This document provides an overview of tensile testing. It discusses tensile specimens, testing machines, stress-strain curves, and key mechanical properties measured by tensile tests such as strength, ductility, and elastic modulus. Tensile tests are used to select materials, ensure quality, compare new materials/processes, and predict behavior under other loads. Stress-strain curves are generated by applying tension to a specimen and recording the resulting force and elongation. Important aspects of the curves, like yield strength and plastic deformation, are defined.Design and development of horizontal tensile testing machine (5kN)



Design and development of horizontal tensile testing machine (5kN)Sajeed Mahaboob
Ėý
In this project, a portable horizontal tensile testing system involving the use of a specially designed tensile specimen is proposed. The system developed was designed to convert the rotation motion of a ball screw into the linear motion of specimen grips that apply a tensile load to the specimen. The frame contains an aligned linear motion guide for the movement of the specimen grips, ensuring the co-linearity of the travel axes. One side of the specimen is connected to a ball-screw block and the other side is connected to a load-cell (which is static) to detect the load magnitude.exp no.1 tensile test 



exp no.1 tensile test Muhammed Fuad Al-Barznji
Ėý
Muhammed Fuad Rashid
Petroleum Engineering Department at Koya University
Mechanics of material Laboratory
2020Examination of Tensile Test Specimens Produced in Three-Dimensional Printer: ...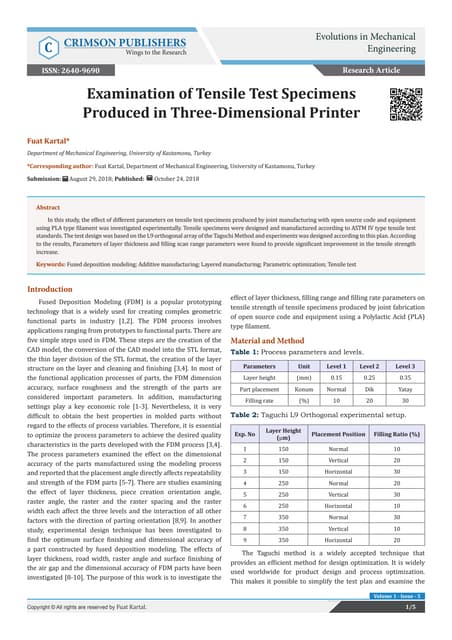



Examination of Tensile Test Specimens Produced in Three-Dimensional Printer: ...Crimsonpublishers-Mechanicalengineering
Ėý
Examination of Tensile Test Specimens Produced in Three-Dimensional Printer by Fuat Kartal* in Crimson Publishers: Applied mechanical engineering
In this study, the effect of different parameters on tensile test specimens produced by joint manufacturing with open source code and equipment using PLA type filament was investigated experimentally. Tensile specimens were designed and manufactured according to ASTM IV type tensile test standards. The test design was based on the L9 orthogonal array of the Taguchi Method and experiments was designed according to this plan. According to the results, Parameters of layer thickness and filling scan range parameters were found to provide significant improvement in the tensile strength increase
Lab report engineering materials lab - tensile test



Lab report engineering materials lab - tensile testMuhammad Yossi
Ėý
This is my Lab Report of Tensile Test when I was conducting engineering material lab in Sampoerna University. Feel free to download for a reference.
I know it is not a good report, but I hope this share might help you to find something you need.
Thank you.Shock Test Report 04



Shock Test Report 04Franco Pezza
Ėý
This report summarizes a shock test experiment on a cantilevered aluminum beam. A rotating hammer struck the beam at various angles, and a string gauge measured the resulting deformation. The maximum impact strain of 1876 Ξ-strain occurred at 20 degrees. Calculations determined the maximum stress on the beam was 18760.69 psi, which is 40.43% of the yield stress for aluminum. The energy loss in the system was approximately 28.5%, and the natural frequency of the beam was 76.92 Hz. The experiment verified relationships between impact energy, beam deformation, and impact angle.UTM Machine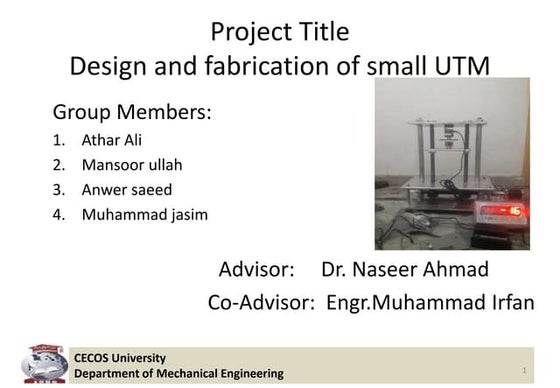



UTM Machine11chawki
Ėý
This document describes a project to design and fabricate a small universal testing machine (UTM) at CECOS University. A group of 4 students led the project under the guidance of an advisor and co-advisor. The UTM will be capable of performing tensile and compressive tests on soft materials like aluminum and plastics. It will weigh approximately 70kg and have dimensions of 80cm. The main components include steel plates, columns, power screws, sensors, and a stepper motor. Mild steel was selected as the primary material and fabrication involved various machining processes like cutting, drilling, grinding, and welding. The document outlines the subassemblies, final assembly, testing plans, and references used.Tensile testing experiment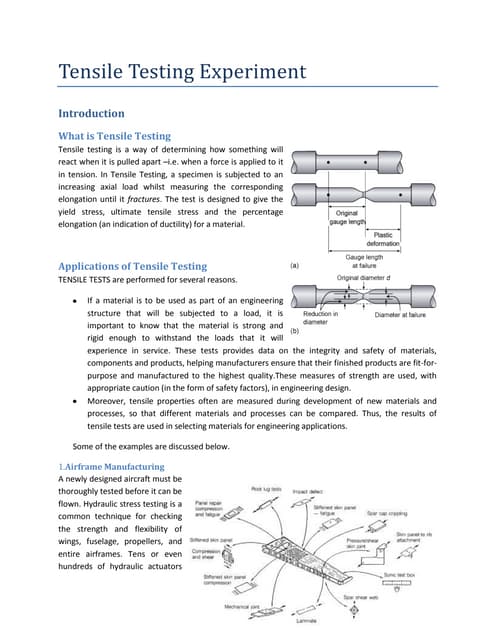



Tensile testing experimentSulaiman Dawood Barry
Ėý
Tensile testing is used to determine the strength and ductility of materials. A specimen is placed in grips and pulled apart under increasing tensile force while measuring elongation. The resulting stress-strain curve provides properties like yield strength, tensile strength, and Young's modulus. Tensile tests are important for engineering design and quality control by ensuring materials can withstand expected loads and comparing new materials. Common applications include testing aircraft components, bolts, and other loaded structures.Tensile test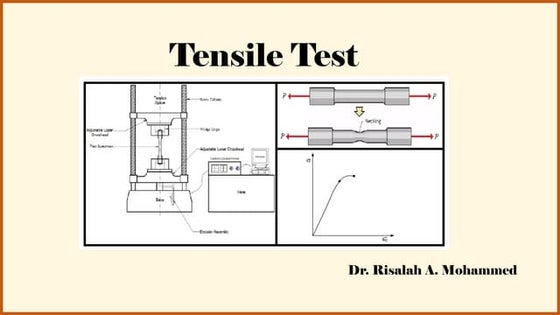



Tensile testDr.Risalah A. Mohammed
Ėý
Tensile testing subjects a material sample to controlled tension until failure to determine properties like ultimate tensile strength and elongation. The test uses a universal testing machine to apply tension to a standardized tensile specimen, measuring properties like modulus of elasticity, yield stress, and fracture stress. The test procedure involves securing the specimen in the machine and applying tension until failure while recording the stress-strain curve.Universal testing machines



Universal testing machinesGulfam Hussain
Ėý
The document discusses a presentation on a universal testing machine. It describes how the machine is used to apply tensile, compressive, and shear forces to test materials and measure their properties. It explains that the machine uses load cells, crossheads, and columns to grip specimens and apply and measure forces. The document outlines the working principle of the machine and procedures for tensile and compression tests.STRAIN MEASURING TECHNIQUES & APPLICATIONS 



STRAIN MEASURING TECHNIQUES & APPLICATIONS ASHIKA DILSHAN
Ėý
The document describes three experiments measuring strain on different surfaces using strain gauges:
1) Measuring strain on an I-beam under varying loads, finding theoretical strain values match measured values. Principal strains are calculated.
2) Measuring strain on a torsional cylindrical rod, calculating principal strains.
3) Measuring combined bending and torsion strain on a circular shaft, calculating principal strains using Mohr's circle.
The experiments aim to observe and compare measured and theoretical strain values using strain gauges and analytical calculations.DESIGN IMPROVISATION OF ELECTROMECHANICAL ACTUATORS FOR OPERATION AT SUB ZERO...



DESIGN IMPROVISATION OF ELECTROMECHANICAL ACTUATORS FOR OPERATION AT SUB ZERO...ijmech
Ėý
Control surface actuators are the key systems in any flight vehicle for enabling a strict control on the flight
parameters. The electromechanical actuator developed for an Unmanned aerial vehicle (UAV) is subjected
to sub-zero temperatures due to the altitude of operation. This paper discusses on how an actuator
developed is studied experimentally and improvised in design to ensure performance at -40oC. The
experimental observations are reasoned and supported by theoretical studies and remedial measures
incorporated to improve the actuator performance.LabReport2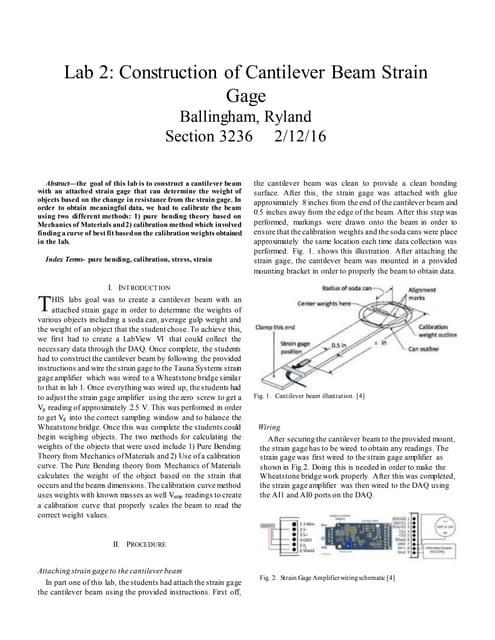



LabReport2Ryland Ballingham
Ėý
The document describes a lab experiment to construct a cantilever beam with an attached strain gage. This setup is used to determine the weights of various objects by measuring changes in resistance from the strain gage. The beam is calibrated using two methods: 1) pure bending theory based on mechanics principles and 2) a calibration curve method using known weights. Results show the calibration curve method has lower uncertainty and is therefore more accurate for determining unknown weights.Monitoring of strain and seismic vibrations in structures



Monitoring of strain and seismic vibrations in structureskalyanabooshnam
Ėý
This document discusses monitoring strain and seismic vibrations in structures through various approaches. It compares using electrical strain gauges, piezoelectric materials, and vibration monitoring to assess structural health. Experimental work was conducted on reinforced concrete beams to measure strain using electrical gauges under loading. Piezoelectric patches were also bonded to the beams to monitor vibrations from 100-250 kHz and detect changes caused by damage. The results showed the electrical strain gauges and vibration monitoring techniques can effectively monitor structures and detect damage.Polytechnic of namibia ...



Polytechnic of namibia ...Evandro Conceicao
Ėý
This lab report summarizes two experiments measuring resistance and voltage. In the first experiment, the resistance of three resistors was calculated from color codes and measured with a multimeter, finding errors within 5%. The second experiment measured voltages from a power supply at increasing levels, finding small discrepancies between the power supply and multimeter readings due to internal resistance converting energy. Overall, the lab demonstrated that calculating resistance from color codes is reasonably accurate and introduced concepts of resistance, voltage measurement, and energy conversion in circuits.Polytechnic of namibia ...



Polytechnic of namibia ...Evandro Conceicao
Ėý
This lab report summarizes two experiments measuring resistance and voltage. In the first experiment, the resistance of three resistors was calculated from their color codes and measured with a digital multimeter. The measured values were within 5% of the calculated values, validating the accuracy of using color codes. In the second experiment, the voltage output of a power supply was measured at increasing levels and found to be slightly lower than the power supply readings due to internal resistance dropping voltage. The experiments helped familiarize the student with lab equipment and electrical measurements.More Related Content
What's hot (20)
Lab 8 tensile testing 



Lab 8 tensile testing elsa mesfin
Ėý
This document provides instructions for conducting a tensile test to determine the mechanical properties of polymers. A tensile test involves gripping a dogbone-shaped polymer specimen at both ends and pulling it at a constant rate until failure. Key points:
- Stress-strain curves are generated from the test, showing properties like elastic modulus, yield point, and toughness.
- Properties depend on factors like crystallinity, molecular weight, and glass transition temperature. Brittle polymers have steeper stress-strain curves.
- The test procedure involves preparing specimens to standards, setting up the tensile testing machine and software to control displacement rate and record data, calibrating load cells, gripping the specimen, and conducting the0 exp no.4 bending test experiment



0 exp no.4 bending test experimentMuhammed Fuad Al-Barznji
Ėý
Muhammed Fuad Rashid
Petroleum Engineering Department at Koya University
Mechanics of material Laboratory
2020Instron machine



Instron machineBrijesh Kumar Bajpai
Ėý
The document discusses an Instron machine, which is a versatile instrument used to measure load from 2 grams to 100 kgs using a strain gauge principle. It consists of a load cell containing metal beams with strain gauges that measure the resistance change when a load is applied. This allows the machine to generate a load-elongation curve on a chart. The Instron machine can test properties of yarns, cords, fibers and other materials under tension, and has applications in measuring tensile, flexural, friction and tear properties. It operates over a wide range of speeds and loads with interchangeable load cells and grips.ENGINEERING SYSTEM DYNAMICS-TAKE HOME ASSIGNMENT 2018



ENGINEERING SYSTEM DYNAMICS-TAKE HOME ASSIGNMENT 2018musadoto
Ėý
1. Read Chapter 4 â System Dynamics for Mechanical Engineers by Matthew Davies and Tony L. Schmitz and implement Examples 4.1 to 4.12 in Matlab.
2. Read Chapter 7 â System Dynamics for Mechanical Engineers by Matthew Davies and Tony L. Schmitz and implement Examples 7.1 to 7.11 in Matlab.
3. Read Chapter 9 â System Dynamics for Mechanical Engineers by Matthew Davies and Tony L. Schmitz and implement Examples 9.1 to 9.6 in Matlab.
4. Read Chapter 11 â System Dynamics for Mechanical Engineers by Matthew Davies and Tony L. Schmitz and implement Examples 11.1 to 11.7 in Matlab.
5. Read Chapter 2 - System Dynamics for Engineering Students: Concepts and Applications by Nicolae Lobontiu and attempt problem 2.18 (page 63).
6. Read Chapter 3 - System Dynamics for Engineering Students: Concepts and Applications by Nicolae Lobontiu and attempt problem 3.13 (pp 98 - 100).
7. Read Chapter 4 - System Dynamics for Engineering Students: Concepts and Applications by Nicolae Lobontiu and attempt problem 4.20 (page 146).
8. Read Chapter 5 - System Dynamics for Engineering Students: Concepts and Applications by Nicolae Lobontiu and attempt problems 5.15 (page 198), 5.21 (pp 199 - 200) and 5.27 (pp 201 â 202).
0 exp no.3 torsion



0 exp no.3 torsionMuhammed Fuad Al-Barznji
Ėý
Muhammed Fuad Rashid
Petroleum Engineering Department at Koya University
Mechanics of material Laboratory
2020exp: sample preparation for tensile test 



exp: sample preparation for tensile test Muhammed Fuad Al-Barznji
Ėý
This document describes an experiment on tensile testing of materials. It discusses preparing dog-bone shaped samples according to ASTM D638 standards. Tensile testing is done using a Shimadzu tensile testing machine to measure properties like stress and strain. Careful sample preparation and dimensions matching standards are needed to obtain accurate property values from the experiment. The conclusions emphasize getting the right sample dimension values according to standards to determine material properties correctly.Tensile



TensileAmmour Omrane
Ėý
This document provides an overview of tensile testing. It discusses tensile specimens, testing machines, stress-strain curves, and key mechanical properties measured by tensile tests such as strength, ductility, and elastic modulus. Tensile tests are used to select materials, ensure quality, compare new materials/processes, and predict behavior under other loads. Stress-strain curves are generated by applying tension to a specimen and recording the resulting force and elongation. Important aspects of the curves, like yield strength and plastic deformation, are defined.Design and development of horizontal tensile testing machine (5kN)



Design and development of horizontal tensile testing machine (5kN)Sajeed Mahaboob
Ėý
In this project, a portable horizontal tensile testing system involving the use of a specially designed tensile specimen is proposed. The system developed was designed to convert the rotation motion of a ball screw into the linear motion of specimen grips that apply a tensile load to the specimen. The frame contains an aligned linear motion guide for the movement of the specimen grips, ensuring the co-linearity of the travel axes. One side of the specimen is connected to a ball-screw block and the other side is connected to a load-cell (which is static) to detect the load magnitude.exp no.1 tensile test 



exp no.1 tensile test Muhammed Fuad Al-Barznji
Ėý
Muhammed Fuad Rashid
Petroleum Engineering Department at Koya University
Mechanics of material Laboratory
2020Examination of Tensile Test Specimens Produced in Three-Dimensional Printer: ...



Examination of Tensile Test Specimens Produced in Three-Dimensional Printer: ...Crimsonpublishers-Mechanicalengineering
Ėý
Examination of Tensile Test Specimens Produced in Three-Dimensional Printer by Fuat Kartal* in Crimson Publishers: Applied mechanical engineering
In this study, the effect of different parameters on tensile test specimens produced by joint manufacturing with open source code and equipment using PLA type filament was investigated experimentally. Tensile specimens were designed and manufactured according to ASTM IV type tensile test standards. The test design was based on the L9 orthogonal array of the Taguchi Method and experiments was designed according to this plan. According to the results, Parameters of layer thickness and filling scan range parameters were found to provide significant improvement in the tensile strength increase
Lab report engineering materials lab - tensile test



Lab report engineering materials lab - tensile testMuhammad Yossi
Ėý
This is my Lab Report of Tensile Test when I was conducting engineering material lab in Sampoerna University. Feel free to download for a reference.
I know it is not a good report, but I hope this share might help you to find something you need.
Thank you.Shock Test Report 04



Shock Test Report 04Franco Pezza
Ėý
This report summarizes a shock test experiment on a cantilevered aluminum beam. A rotating hammer struck the beam at various angles, and a string gauge measured the resulting deformation. The maximum impact strain of 1876 Ξ-strain occurred at 20 degrees. Calculations determined the maximum stress on the beam was 18760.69 psi, which is 40.43% of the yield stress for aluminum. The energy loss in the system was approximately 28.5%, and the natural frequency of the beam was 76.92 Hz. The experiment verified relationships between impact energy, beam deformation, and impact angle.UTM Machine



UTM Machine11chawki
Ėý
This document describes a project to design and fabricate a small universal testing machine (UTM) at CECOS University. A group of 4 students led the project under the guidance of an advisor and co-advisor. The UTM will be capable of performing tensile and compressive tests on soft materials like aluminum and plastics. It will weigh approximately 70kg and have dimensions of 80cm. The main components include steel plates, columns, power screws, sensors, and a stepper motor. Mild steel was selected as the primary material and fabrication involved various machining processes like cutting, drilling, grinding, and welding. The document outlines the subassemblies, final assembly, testing plans, and references used.Tensile testing experiment



Tensile testing experimentSulaiman Dawood Barry
Ėý
Tensile testing is used to determine the strength and ductility of materials. A specimen is placed in grips and pulled apart under increasing tensile force while measuring elongation. The resulting stress-strain curve provides properties like yield strength, tensile strength, and Young's modulus. Tensile tests are important for engineering design and quality control by ensuring materials can withstand expected loads and comparing new materials. Common applications include testing aircraft components, bolts, and other loaded structures.Tensile test



Tensile testDr.Risalah A. Mohammed
Ėý
Tensile testing subjects a material sample to controlled tension until failure to determine properties like ultimate tensile strength and elongation. The test uses a universal testing machine to apply tension to a standardized tensile specimen, measuring properties like modulus of elasticity, yield stress, and fracture stress. The test procedure involves securing the specimen in the machine and applying tension until failure while recording the stress-strain curve.Universal testing machines



Universal testing machinesGulfam Hussain
Ėý
The document discusses a presentation on a universal testing machine. It describes how the machine is used to apply tensile, compressive, and shear forces to test materials and measure their properties. It explains that the machine uses load cells, crossheads, and columns to grip specimens and apply and measure forces. The document outlines the working principle of the machine and procedures for tensile and compression tests.STRAIN MEASURING TECHNIQUES & APPLICATIONS 



STRAIN MEASURING TECHNIQUES & APPLICATIONS ASHIKA DILSHAN
Ėý
The document describes three experiments measuring strain on different surfaces using strain gauges:
1) Measuring strain on an I-beam under varying loads, finding theoretical strain values match measured values. Principal strains are calculated.
2) Measuring strain on a torsional cylindrical rod, calculating principal strains.
3) Measuring combined bending and torsion strain on a circular shaft, calculating principal strains using Mohr's circle.
The experiments aim to observe and compare measured and theoretical strain values using strain gauges and analytical calculations.DESIGN IMPROVISATION OF ELECTROMECHANICAL ACTUATORS FOR OPERATION AT SUB ZERO...



DESIGN IMPROVISATION OF ELECTROMECHANICAL ACTUATORS FOR OPERATION AT SUB ZERO...ijmech
Ėý
Control surface actuators are the key systems in any flight vehicle for enabling a strict control on the flight
parameters. The electromechanical actuator developed for an Unmanned aerial vehicle (UAV) is subjected
to sub-zero temperatures due to the altitude of operation. This paper discusses on how an actuator
developed is studied experimentally and improvised in design to ensure performance at -40oC. The
experimental observations are reasoned and supported by theoretical studies and remedial measures
incorporated to improve the actuator performance.LabReport2



LabReport2Ryland Ballingham
Ėý
The document describes a lab experiment to construct a cantilever beam with an attached strain gage. This setup is used to determine the weights of various objects by measuring changes in resistance from the strain gage. The beam is calibrated using two methods: 1) pure bending theory based on mechanics principles and 2) a calibration curve method using known weights. Results show the calibration curve method has lower uncertainty and is therefore more accurate for determining unknown weights.Monitoring of strain and seismic vibrations in structures



Monitoring of strain and seismic vibrations in structureskalyanabooshnam
Ėý
This document discusses monitoring strain and seismic vibrations in structures through various approaches. It compares using electrical strain gauges, piezoelectric materials, and vibration monitoring to assess structural health. Experimental work was conducted on reinforced concrete beams to measure strain using electrical gauges under loading. Piezoelectric patches were also bonded to the beams to monitor vibrations from 100-250 kHz and detect changes caused by damage. The results showed the electrical strain gauges and vibration monitoring techniques can effectively monitor structures and detect damage.Examination of Tensile Test Specimens Produced in Three-Dimensional Printer: ...



Examination of Tensile Test Specimens Produced in Three-Dimensional Printer: ...Crimsonpublishers-Mechanicalengineering
Ėý
Viewers also liked (7)
Polytechnic of namibia ...



Polytechnic of namibia ...Evandro Conceicao
Ėý
This lab report summarizes two experiments measuring resistance and voltage. In the first experiment, the resistance of three resistors was calculated from color codes and measured with a multimeter, finding errors within 5%. The second experiment measured voltages from a power supply at increasing levels, finding small discrepancies between the power supply and multimeter readings due to internal resistance converting energy. Overall, the lab demonstrated that calculating resistance from color codes is reasonably accurate and introduced concepts of resistance, voltage measurement, and energy conversion in circuits.Polytechnic of namibia ...



Polytechnic of namibia ...Evandro Conceicao
Ėý
This lab report summarizes two experiments measuring resistance and voltage. In the first experiment, the resistance of three resistors was calculated from their color codes and measured with a digital multimeter. The measured values were within 5% of the calculated values, validating the accuracy of using color codes. In the second experiment, the voltage output of a power supply was measured at increasing levels and found to be slightly lower than the power supply readings due to internal resistance dropping voltage. The experiments helped familiarize the student with lab equipment and electrical measurements.Elc pre lab 2 complete



Elc pre lab 2 completeEvandro Conceicao
Ėý
The document discusses a pre-lab assignment for an Electrical Engineering laboratory on Ohm's Law, voltage dividers, and current dividers. It contains the student's responses to 4 questions about power dissipation in resistors, definitions of voltage dividers and current dividers, and applying knowledge of series and parallel circuits to calculate equivalent resistances. The student explained that power dissipated in a resistor depends on resistance and current squared, defined voltage and current dividers, and correctly calculated total resistances for the series and parallel circuits in the questions.Report 3



Report 3Evandro Conceicao
Ėý
This lab report summarizes experiments conducted to study Ohm's Law and circuit behavior. The experiments involved measuring currents and voltages in circuits with single and dual power supplies connected to various resistor configurations. The results showed that resistance decreases in parallel circuits as predicted by Ohm's Law. Kirchhoff's Laws and the conservation of energy were also verified. Overall, the experimental findings supported the theoretical understanding of how voltage, current and resistance relate in electrical circuits.Mom 3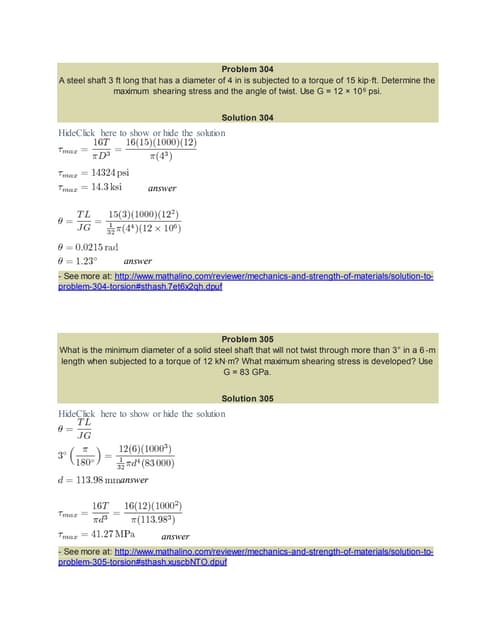



Mom 3Evandro Conceicao
Ėý
The document contains solutions to 325 problems related to torsion and torsional stress. The problems involve determining shear stresses, angles of twist, and diameters of circular or hollow shafts subjected to various torque loads. The solutions show calculations of shear stresses and angles of twist using the appropriate torsion equations and given material properties like shear modulus.Lab report 2



Lab report 2Evandro Conceicao
Ėý
This document summarizes an experiment to verify Ohm's law and analyze resistive circuits. The experiment has two parts: 1) Develop a voltage-current characteristic curve for a resistor to verify Ohm's law. Measure voltage and current at increasing voltage levels and plot the relationship. 2) Determine voltages and currents in series and parallel resistor circuits using voltage and current divider rules. Measure voltages across individual resistors in series to verify calculations match measurements.Similar to Mom lab revised (20)
Static Strain Measurement (Over Hanging Beam).pptx



Static Strain Measurement (Over Hanging Beam).pptxAshok Banagar
Ėý
This document outlines an experiment to measure strain on a cantilever beam using resistance strain gauges. It includes an introduction explaining strain measurement using strain gauges, objectives of learning how to use strain indicators and apply uncertainty analysis. The methodology section details the equipment used including a cantilever beam, strain gauges, weights and amplifier. The experimentation section provides steps to mount the beam, zero the amplifier, record strain measurements at different beam lengths and weight amounts. The results section shows tables of strain values measured. Finally, the conclusions note that strain increased with increasing beam length and load amount as expected.Strength of materials_laboratory



Strength of materials_laboratorysudheerchekka1
Ėý
This experiment aims to determine the modulus of rigidity of a mild steel or cast iron specimen through a torsion test. The torsion test will be conducted using a torsion testing machine and angle of twist measuring attachment. Observations of the maximum twisting torque and angle of twist will be recorded. These values along with the specimen's length and polar moment of inertia will be used in the torsion equation to calculate the modulus of rigidity. Precautions such as ensuring the specimen remains within its elastic limit will be followed to obtain accurate results.Sample preparation for tensile test 2



Sample preparation for tensile test 2Muhammed Fuad Al-Barznji
Ėý
This document describes an experiment on tensile testing of materials. It discusses preparing dog-bone shaped samples according to ASTM D638 standards. Tensile testing is done using a Shimadzu tensile testing machine to measure properties like stress and strain. Careful sample preparation and dimensions matching standards are needed to obtain accurate property values from the experiment. The conclusions emphasize getting the right sample dimension values according to standards to determine material properties correctly.exp no.0 sample preparation for tensile test 



exp no.0 sample preparation for tensile test Muhammed Fuad Al-Barznji
Ėý
Muhammed Fuad Rashid
Petroleum Engineering Department at Koya University
Mechanics of material Laboratory
2020Strength of material lab, Exp 3&4: Compression and impact tests



Strength of material lab, Exp 3&4: Compression and impact testsOsaid Qasim
Ėý
- Utilization the UTM machine and know the different
ways that could test the materialâs properties.
2- Knowing the different types of failure in the compression.
3- Determining Young's modulus âEâ and Passionâs ratio
âÏ
â and Yield/Proof stress Ï y.
1. Finding the impact load effect on the materials.
2. Finding the relative toughness of the different materials.
3. Distinguish between static and dynamic loads and how differently they
effect in the material.
4.Knowing the different methods to preform the impact test (Charpy,
IZOD, Impact tensile).exp no.2 compressive test



exp no.2 compressive testMuhammed Fuad Al-Barznji
Ėý
Muhammed Fuad Rashid
Petroleum Engineering Department at Koya University
Mechanics of material Laboratory
2020fdocuments.in_tensile-test-presentation-55845f0fef46d.ppt



fdocuments.in_tensile-test-presentation-55845f0fef46d.pptRanjithAk38
Ėý
This document provides an overview of a tensile testing lab, including the basic principles and terminology of tensile testing, the objectives and procedures of the lab, and an example tensile test simulation. The lab aims to determine material properties through uniaxial tensile testing according to ASTM standards and analyze stress-strain curves and strain hardening behavior. Finite element analysis is used to simulate the deformation under tensile loading for different materials.Instrumentation Lab. Experiment #6 Report: Strain Measurements 1



Instrumentation Lab. Experiment #6 Report: Strain Measurements 1mohammad zeyad
Ėý
This file contains a report for the 6th experiment in the Lab "Instrumentation and Dynamic Systems", taken by mechanical engineering students. Analysis of Lifting Machine



Analysis of Lifting MachineAhsen Parwez
Ėý
This project report summarizes the design of a transporter machine used to lift heavy machinery between floors. The machine uses pneumatic pistons and chain-driven pulleys. It is divided into 3 subsystems: 1) Pneumatics & Pulley, 2) Mainframe, and 3) Carriage. Each subsystem is analyzed in detail to check for failure against criteria like rupture, buckling, yielding, etc. The analyses ensure each component can withstand a critical design load of 3 tonnes, which is the desired lifting capacity of the machine. The report also provides design recommendations and addresses potential design shortcomings.Tensile test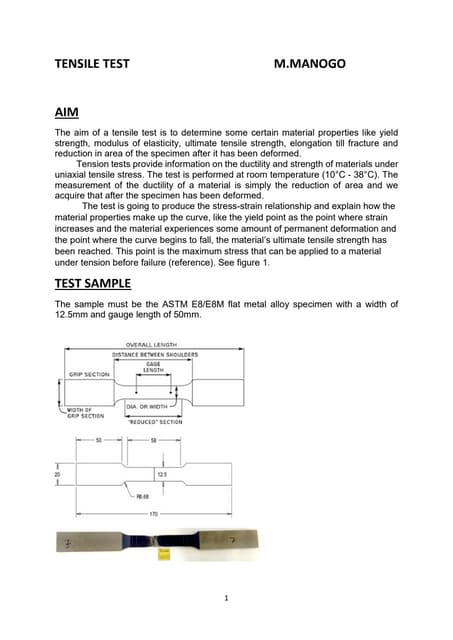



Tensile testMoetiManogo
Ėý
Tensile test research and accurate data for academics and professional engineers. Engineers use tensile tests to solve most fracture problems.1-tension.pdf



1-tension.pdfssuserf48c97
Ėý
The static tension test determines the strength of a material when subjected to stretching. A standard test specimen is pulled slowly until failure using a testing machine. The shape is usually round, square, or rectangular. Dimensions depend on standards but the gage length must have a uniform cross-section. The stress-strain diagram is analyzed to determine properties like yield stress, tensile strength, elongation, modulus of elasticity, and toughness. True stress and true strain consider changes in cross-sectional area during plastic deformation.Mechanics of solids_1_lab_report 



Mechanics of solids_1_lab_report HammadShoaib4
Ėý
Hammad Shoaib submitted a lab report for the Mechanics of Solids course to determine various mechanical properties of materials through tensile and bend tests. The report describes procedures to develop a stress-strain curve for steel rebar and determine its yield strength, ultimate strength, modulus of elasticity, and percentage elongation. Additional experiments include a bend test to examine ductility and a tensile test on wood to find compressive strengths parallel and perpendicular to the grain.Diploma 3 Strength_of_Materials.pdf



Diploma 3 Strength_of_Materials.pdfGokarnaMotra1
Ėý
This document provides instructions for conducting a tensile test experiment using a Universal Testing Machine. The aim is to determine properties like elastic limit, yield strength, ultimate strength, Young's modulus of elasticity, percentage elongation, and percentage reduction in area of a mild steel specimen. The procedure involves fixing a specimen in the grips of the UTM and applying a tensile load until failure. Measurements are taken from the load-extension graph to calculate the material properties.Design of a testing bench, statistical and reliability analysis of some mecha...



Design of a testing bench, statistical and reliability analysis of some mecha...IAEME Publication
Ėý
This document describes the design and manufacturing of a testing bench to determine mechanical properties of materials and stiffness of springs or absorption factors of shock absorbers simultaneously. The testing bench uses combinations of test specimens and springs or shock absorbers. Statistical analysis is conducted on the results to determine mean values and standard deviations of the spring stiffness or material properties such as resilience or tensile strength. Specifically, the testing of ebony wood samples and a spring determined the spring stiffness and wood resilience. Testing of glass samples and a spring or shock absorber yielded the spring/absorber properties and glass tensile strength.strenght of materials lab R18A0383.pdf



strenght of materials lab R18A0383.pdfGokarnaMotra1
Ėý
This document describes an experiment to determine the deflection and bending stress of a cantilever beam. A cantilever beam is clamped at one end and free at the other. Deflection measurements are taken at the free end as loads are applied. The deflection values are used to calculate the beam's Young's modulus and bending strength based on equations that relate deflection to the beam's properties and loading. Proper measurement techniques and safety precautions are outlined to ensure accurate results. The experiment is designed to analyze beam behavior under bending loads.Determination of Elastic Behavior of RCC Section by Experimentation and Valid...



Determination of Elastic Behavior of RCC Section by Experimentation and Valid...IJERA Editor
Ėý
Experimental and Analytical study is conducted to check the elastic behavior of RCC and Fiber reinforced RCC
section. Standard size and shapes of specimen s are casted with combination of RCC with and without steel
fibers. Percentage of FRC is varied from 0.5% to 1.5% in RCC specimen. Elastic behavior of the specimen
tested and studied for different loading conditions (Axial and Flexural). Observed results are plotted in different
formats and validated using FEA. Mech CE6315 som lab_manual



Mech CE6315 som lab_manualKIT-Kalaignar Karunanidhi Institute of Technology
Ėý
This document contains instructions and procedures for conducting various strength of materials experiments in a laboratory. It includes experiments like tensile testing, torsion testing, impact testing using Izod and Charpy methods, and hardness testing. The document provides the aims, required equipment, procedures, formulas, observations tables and sample calculations for determining properties like yield stress, modulus of rigidity, and impact strength from the experimental results. It is a manual intended to guide students through conducting various experiments and analyzing the data in a strength of materials laboratory class.Mom lab revised
- 1. Polytechnic of Namibia Fluid Mechanics 610S1 Bernoulliâs Experiment [Type here] 9/11/2013 Group members 1. E.J.N Conceicao 212086561 2. 3. 4. 5. 6. 7. 8. 9. 10. Lecturer: Mr Okorie Maduako E. B.Eng. (Mech.), M. Eng.(Mech.) Lab. Technician: Isabel Bishi (Mrs), B Tech, Mech. Eng.
- 2. Introduction The flow of a fluid has to be in agreement with a bunch of scientific principles and theories which follow the conservation of mass and energy. The first of these principles is that a liquid flowing through a conduit requires a steady flow therefore the velocity is constant and will be inversely proportional to the flow area. Secondly the theory states that if a velocity increases then the pressure must decrease. Both Bernoulliâs equation and the continuity equation are essential analytical tools for the design of fluid carrying devices such as (pipes, machines) that is in the subject of fluid mechanics. Aim of practical Therefore the purpose of this lab is to verify Bernoulliâs equation by making use of the P6231 Bernoulliâs apparatus which demonstrates both of the principles stated above which can also examine the turbulence in an accelerating fluid stream. Theory If a load is statically changed with time and applied slowly over a cross-sectional area of a member, its change in behaviour may change and this will produce the graphs in figures 3, 4. These testes are normally conducted at room temperature and the ways in which this load may be applied are as follows, tension which is the method used in this experiment, compression and shear. Tension is the most widely used method to determine the change in the mechanical behaviour of a material during loading. Most of the tension tests are carried out according to the ASTM standard (Standard Test Methods for Tension Testing of Metallic Materials). Equations to be familiar with for the understanding of the mathematical section of the report. ï· To calculate the stressâĶ Force/area (Equation 1) ï· Cross Sectional area= ((Ï/4)*D^2)) (Equation 2) ï· Change in elongation = strain*Gauge length (Equation 3) ï· Tensile Strength (UTS) = Maximum load/Area (Equation 4) ï· Modulus of elasticity= Stress/ Strain (Equation 5) ï· To calculate strain= Change in length/original length (Equation 6) Hypothesis As the tensile load is gradually increased axially the elongation of the specimen is first proportional to the load applied to the material is said to be elastic. However as one keeps on increasing the load, the elastic limit of the specimen is exceeded and it starts yielding. At some point the specimen will reach the plastic region where by the material keeps on elongating without a considerable loading. By continuously increasing the load the material starts to strain harden, whereby changes in the internal structure occur, so the material will not elongate that much as one increases the load. This will cause the specimen to fracture with little application of the load.
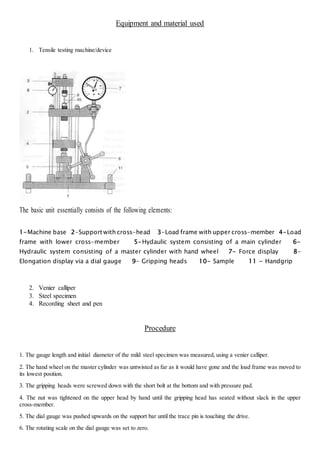
- 3. Equipment and material used 1. Tensile testing machine/device The basic unit essentially consists of the following elements: 1-Machine base 2-Support with cross-head 3-Load frame with upper cross-member 4-Load frame with lower cross-member 5-Hydaulic system consisting of a main cylinder 6- Hydraulic system consisting of a master cylinder with hand wheel 7- Force display 8- Elongation display via a dial gauge 9- Gripping heads 10- Sample 11 - Handgrip 2. Venier calliper 3. Steel specimen 4. Recording sheet and pen Procedure 1. The gauge length and initial diameter of the mild steel specimen was measured, using a venier calliper. 2. The hand wheel on the master cylinder was untwisted as far as it would have gone and the load frame was moved to its lowest position. 3. The gripping heads were screwed down with the short bolt at the bottom and with pressure pad. 4. The nut was tightened on the upper head by hand until the gripping head has seated without slack in the upper cross-member. 5. The dial gauge was pushed upwards on the support bar until the trace pin is touching the drive. 6. The rotating scale on the dial gauge was set to zero.
- 4. 7. The maximum pointer on the force display was also set to zero. 8. The specimen was slowly and constantly loaded by rotating the hand wheel. 9. The interval was increased by 0.1mm after each complete revolution on the dial gauge. 10. The force from the force display was recorded every 0.1mm. From 1mm extension, the reading was extended to 0.2mm and after another 1mm extension it was extended to 0.3mm and so on. 11. The sample was monitored and noted for constriction. 12. The maximum test force was read from the maximum pointer and noted. 13. After fracture the sample was removed from the heads. 14. The hand wheel on the master cylinder was twisted back as far as it has gone and the load frame was moved down. Safety pre-cautions and issues to note during the tensile test: ï· -Wearing of safety boots is mandatory when performing the test. ï· -Lab overalls should be worn. ï· -Avoiding sudden, jerky force application. ï· -Avoiding being startled during the fracturing moment as it may set off quite a bang. - Observations 1. The steel specimen was placed on the tensile testing machine. 2. As the load was continuously applied to the steel specimen, it started to neck around the upper part of the gauge length. Figure: 1 3. After some time the steel specimen fractured.
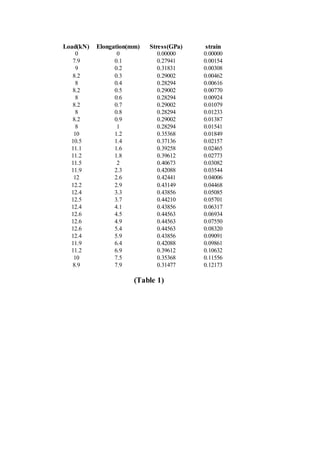
- 5. Figure :2 Results To calculate the stressâĶ Force/area To calculate the strainâĶ change in length/original length Cross Sectional area ((Ï/4)*6^2)) = 28.27433388mm2 Gauge length 64.9mm
- 6. Load(kN) Elongation(mm) Stress(GPa) strain 0 0 0.00000 0.00000 7.9 0.1 0.27941 0.00154 9 0.2 0.31831 0.00308 8.2 0.3 0.29002 0.00462 8 0.4 0.28294 0.00616 8.2 0.5 0.29002 0.00770 8 0.6 0.28294 0.00924 8.2 0.7 0.29002 0.01079 8 0.8 0.28294 0.01233 8.2 0.9 0.29002 0.01387 8 1 0.28294 0.01541 10 1.2 0.35368 0.01849 10.5 1.4 0.37136 0.02157 11.1 1.6 0.39258 0.02465 11.2 1.8 0.39612 0.02773 11.5 2 0.40673 0.03082 11.9 2.3 0.42088 0.03544 12 2.6 0.42441 0.04006 12.2 2.9 0.43149 0.04468 12.4 3.3 0.43856 0.05085 12.5 3.7 0.44210 0.05701 12.4 4.1 0.43856 0.06317 12.6 4.5 0.44563 0.06934 12.6 4.9 0.44563 0.07550 12.6 5.4 0.44563 0.08320 12.4 5.9 0.43856 0.09091 11.9 6.4 0.42088 0.09861 11.2 6.9 0.39612 0.10632 10 7.5 0.35368 0.11556 8.9 7.9 0.31477 0.12173 (Table 1)
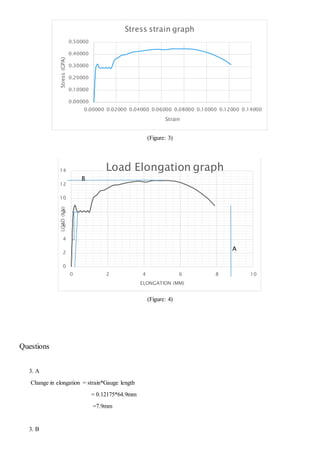
- 7. Stress strain graph (Figure: 3) Load Elongation graph (Figure: 4) Questions 3. A 0.50000 0.40000 0.30000 0.20000 0.10000 14 12 10 8 6 4 2 Change in elongation = strain*Gauge length = 0.12175*64.9mm =7.9mm 3. B 0.00000 0.00000 0.02000 0.04000 0.06000 0.08000 0.10000 0.12000 0.14000 Stress (GPA) Strain 0 0 2 4 6 8 10 LOAD (KN) ELONGATION (MM) B A
- 8. Tensile Strength (UTS) = Maximum load/Area =12.6kN/28.27433388mm2 =0.445GPa 3. C 0.2%*original length = 0.1298mm Therefore o.2% proof stress = 8kN/28.27433388mm2 =0.282GPa 3. D Modulus of elasticity= Stress/ Strain =0.279405345(GPa)/0.00154 =181.43 GPa 4. Since the calculated Modulus of elasticity according to the experiment is 181.43GPa, and the theoretical value for mild steel is 200. The range of these modulus for all steels is between (180&200), therefore it is safe to assume that this experiment represents the way mild steel behaves under tension. 5 CONCLUSION A tensile load was applied to the specimen and the elongation was measured on the dial gauge. It was observed that at just over 8 KN the increase in length was no longer proportional to the increase in load. At this point the test had exceeded the elastic limit of the specimen. The maximum load applied was 12 KN and at this point 'necking' was observed. Even though the load was reduced, elongation continued until the specimen fractured at load of 8.9 KN .The specimen was removed and its broken ends fitted together so the gauge length could be measured at the moment of fracture and gave the reading of 72.8mm. Therefore according to the results we can conclude that the hypothesis is correct.



