Moorey to mealy conversion
- 1. Prof.Neeraj Bhargava Abhishek Kumar Department of Computer Science School of Engineering & System Sciences, MDS, University Ajmer, Rajasthan, India 1
- 2. ïFinite State Machine (FSM) ïMoore Machine ïMealy Machine ïMoorey to Mealy Conversion ïExample 2
- 3. ï― A finite state machine is a machine that has many states and has a logical way of changing from one state to the other under guiding rules. 3
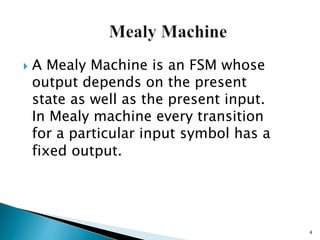
- 4. ï― A Mealy Machine is an FSM whose output depends on the present state as well as the present input. In Mealy machine every transition for a particular input symbol has a fixed output. 4
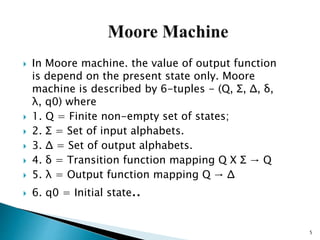
- 5. ï― In Moore machine. the value of output function is depend on the present state only. Moore machine is described by 6-tuples - (Q, ÎĢ, Î, Îī, Îŧ, q0) where ï― 1. Q = Finite non-empty set of states; ï― 2. ÎĢ = Set of input alphabets. ï― 3. Î = Set of output alphabets. ï― 4. Îī = Transition function mapping Q X ÎĢ â Q ï― 5. Îŧ = Output function mapping Q â Î ï― 6. q0 = Initial state.. 5
- 6. ï― Input: Moore Machine ï― Output: Mealy Machine ï― Step 1: Take a blank Mealy Machine transition table format. ï― Step 2: Copy all the Moore Machine transition states into this table format.. ï― Step 3: Check the present states and their corresponding outputs in the Moore Machine state table; if for a state Qi output is m, copy it into the output columns of the Mealy Machine state table wherever Qi appears in the next state.. 6
- 7. 7
- 8. 8
- 9. 9
- 10. 10