Parallel, Perpendicular, or Neither?
- 1. PARALLEL, PERPENDICULAR, OR NEITHER Practice Problems
- 2. REMEMBER THE CHARACTERISTICS: Characteristic Parallel Lines Perpendicular Lines Slope Same or Equal Opposite Reciprocals Y-Intercepts Different Same or Different
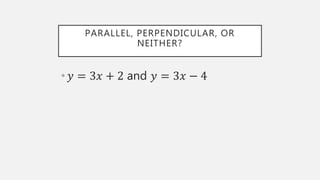
- 3. PARALLEL, PERPENDICULAR, OR NEITHER? ŌĆó Øæ” = 3Øæź + 2 and Øæ” = 3Øæź ŌłÆ 4
- 4. THESE LINES ARE PARALLEL BECAUSE THEY HAVE THE SAME SLOPE, BUT DIFFERENT Y-INTERCEPTS. The first line has a slope of 3 and a y-intercept of 2. The second line has a slope of 3 and a y-intercept of -4.
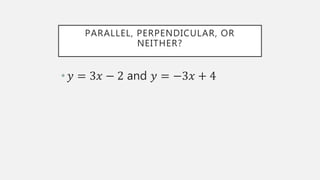
- 5. PARALLEL, PERPENDICULAR, OR NEITHER? ŌĆó Øæ” = 3Øæź ŌłÆ 2 and Øæ” = ŌłÆ3Øæź + 4
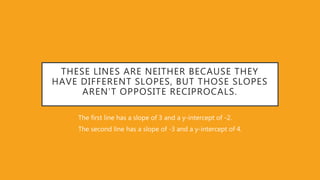
- 6. THESE LINES ARE NEITHER BECAUSE THEY HAVE DIFFERENT SLOPES, BUT THOSE SLOPES ARENŌĆÖT OPPOSITE RECIPROCALS. The first line has a slope of 3 and a y-intercept of -2. The second line has a slope of -3 and a y-intercept of 4.
- 7. PARALLEL, PERPENDICULAR, OR NEITHER? ŌĆó Øæź + 3Øæ” = 12 and 6Øæź ŌłÆ 2Øæ” = 12
- 8. IT WOULD BE EASIER TO DO THIS PROBLEM IF THE EQUATIONS WERE IN SLOPE-INTERCEPT FORM, SO BEGIN BY REWRITING THEM. ŌĆó Øæź + 3Øæ” = 12 ŌĆó Subtract x on each side: ŌĆó 3Øæ” = ŌłÆØæź + 12 ŌĆó Divide each term by 3: ŌĆó Øæ” = ŌłÆ 1 3 Øæź + 4 ŌĆó This line has a slope of ŌłÆ 1 3 and a y- intercept of 4. ŌĆó 6Øæź ŌłÆ 2Øæ” = 12 ŌĆó Subtract 6x on each side: ŌĆó ŌłÆ2Øæ” = ŌłÆ6Øæź + 12 ŌĆó Divide each term by -2: ŌĆó Øæ” = 3Øæź ŌłÆ 6 ŌĆó This line has a slope of 3 and a y- intercept of -6.
- 9. THESE LINES ARE PERPENDICULAR BECAUSE THEY HAVE OPPOSITE RECIPROCAL SLOPES. The first line has a slope of -1/3. The second line has a slope of 3/1.