polynomials ┬®shivam saxena
- 2. 2 x 3 ŌĆō 3 x + 4= 2( 2 ) 3 ŌĆō 3 ( 2 ) + 4
- 3. Monomial: A number, a variable or the product of a number and one or more variables. Polynomial: A monomial or a sum of monomials. Binomial: A polynomial with exactly two terms. Trinomial: A polynomial with exactly three terms. Coefficient: A numerical factor in a term of an algebraic expression.
- 4. Degree of a monomial: The sum of the exponents of all of the variables in the monomial. Degree of a polynomial in one variable: The largest exponent of that variable. Standard form: When the terms of a polynomial are arranged from the largest exponent to the smallest exponent in decreasing order.
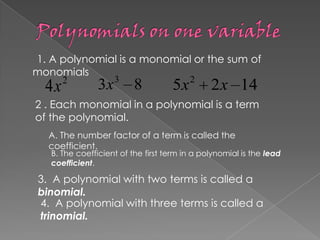
- 5. 1. A polynomial is a monomial or the sum of monomials 2 4x 83 3 x 1425 2 xx 2 . Each monomial in a polynomial is a term of the polynomial. A. The number factor of a term is called the coefficient. B. The coefficient of the first term in a polynomial is the lead coefficient. 3. A polynomial with two terms is called a binomial. 4. A polynomial with three terms is called a trinomial.
- 6. Write the polynomials in standard form. 745 24 xxx 4 4x 2 x x5 7 )7552(1 234 xxxx 4 x 3 2x 2 5x x5 7 Remember: The lead coefficient should be positive in standard form. To do this, multiply the polynomial by ŌĆō1 using the distributive property 243 5572 xxxx 4 x 3 2x 2 5x x5 7
- 7. Write the polynomials in standard form and identify the polynomial by degree and number of terms. 23 237 xx xx 231 2
- 8. 23 237 xx 3 3x 2 2x 7 7231 23 xx 723 23 xx This is a trinomial. The trinomialŌĆÖs degree is 3.
- 9. Add (x2 + x + 1) to (2x2 + 3x + 2) You might decide to add like terms as the next slide demonstrates.
- 10. x2 + x + 1+ 2x2 + 3x + 2= 3x2+ 4x+3 Or you could add the trinomials in a column
- 11. Dividing a polynomial by a polynomial other than a monomial uses a ŌĆ£long divisionŌĆØ technique that is similar to the process known as long division in dividing two numbers, which is reviewed on the next slide.