Ppt on polynomial
- 1. Power Point Presentation on POLYNOMIALS By : Tushar Thapliyal Class : IX â , ROLL NO . .
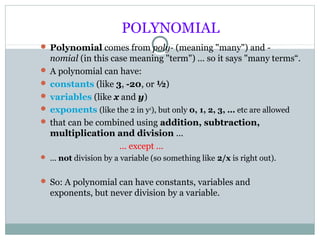
- 2. POLYNOMIAL ï Polynomial comes from poly- (meaning "many") and - nomial (in this case meaning "term") ... so it says "many termsâ. ï A polynomial can have: ï constants (like 3, -20, orÂ Â―) ï variables (like x and y) ï exponents (like the 2 in y2 ), but only 0, 1, 2, 3, ... etc are allowed ï that can be combined using addition, subtraction, multiplication and division ...                                      ... except ... ï ... not division by a variable (so something like 2/x is right out). ï So: A polynomial can have constants, variables and exponents, but never division by a variable.
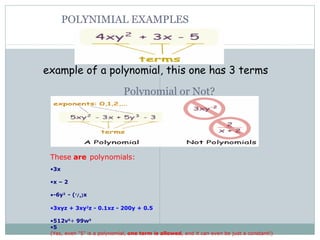
- 3. Polynomial or Not? POLYNIMIAL EXAMPLES example of a polynomial, this one has 3 terms These are polynomials: âĒ3x âĒx â 2 âĒ-6y2  - (7 /9 )x âĒ3xyz + 3xy2 z - 0.1xz - 200y + 0.5 âĒ512v5 + 99w5 âĒ5 (Yes, even "5" is a polynomial, one term is allowed, and it can even be just a constant!)
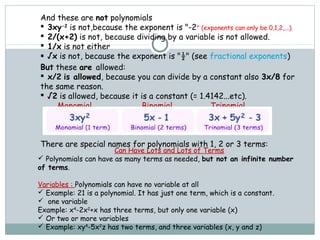
- 4. And these are not polynomials ï§ 3xy-2  is not,because the exponent is "-2" (exponents can only be 0,1,2,...). ï§ 2/(x+2) is not, because dividing by a variable is not allowed. ï§ 1/x is not either ï§ âx is not, because the exponent is "Â―" (see fractional exponents) But these are allowed: ï§ x/2 is allowed, because you can divide by a constant also 3x/8 for the same reason. ï§ â2 is allowed, because it is a constant (= 1.4142...etc). Monomial, Binomial, Trinomial There are special names for polynomials with 1, 2 or 3 terms: Can Have Lots and Lots of Terms ïž Polynomials can have as many terms as needed, but not an infinite number of terms. Variables : Polynomials can have no variable at all ïž Example: 21 is a polynomial. It has just one term, which is a constant. ïž one variable Example: x4 -2x2 +x has three terms, but only one variable (x) ïž Or two or more variables ïž Example: xy4 -5x2 z has two terms, and three variables (x, y and z)
- 5. See how nice and smooth the curve is? The Degree is 3 (the largest exponent of x) What is Special About Polynomials? Because of the strict definition, polynomials are easy to work with. For example we know that: âĒ If you add polynomials you get a polynomial âĒ If you multiply polynomials you get a polynomial ï So you can do lots of additions and multiplications, and still have a polynomial as the result. ï Also, polynomials of one variable are easy to graph, as they have smooth and continuous lines. ïExample: x4 -2x2 +x                               You can also divide polynomials (but the result may not be a polynomial). Degree : The degree of a polynomial with only one variable is the largest exponent of that variable. Example:                  Standard Form : The Standard Form for writing a polynomial is to put the terms with the highest degree first. Example: Put this in Standard Form: 3x2  - 7 + 4x3  + x6 The highest degree is 6, so that goes first, then 3, 2 and then the constant last: x6  + 4x3  + 3x2  - 7 You don't have to use Standard Form, but it helps.
- 6. âĒ Degreeof polynomial :- thehighest power of the variablein apolynomial istermed asthedegreeof polynomial. âĒ Constant polynomial :- A polynomial of degreezero is called constant polynomial. âĒ Linear polynomial :- A polynomial of degreeone. âĒ E.g. :-9x + 1 âĒ Quadratic polynomial :- A polynomial of degree two. E.g. :-3/2yÂē -3y + 3 âĒ Cubic polynomial :- A polynomial of degreethree. âĒ E.g. :-12xÂģ -4xÂē + 5x +1 âĒ Bi â quadratic polynomial :- A polynomial of degree four. âĒ E.g. :- 10x â 7x Âģ+ 8xÂē -12x + 20
- 7. âĒ . Standard Form âĒ The Standard Form for writing apolynomial isto put thetermswith thehighest degreefirst. âĒ Example: Put this in Standard Form: 3x2  - 7 + 4x3  + x6 Thehighest degreeis6, so that goesfirst, then 3, 2 and then theconstant last: x6  + 4x3  + 3x2  - 7
- 8. ZERO OF POLYNOMIAL ï It is a solution to the polynomial equation, P(x) = 0. It is that value of x that makes the polynomialequal to 0. In other words, the number r is a root of a polynomial P(x). ï We say that is a root or zero of a polynomial, P(x) , if P(r)=0 . In other words, x=r is a root or zero of a polynomial if it is a solution to the equation P(x)=0 . ï Letâs first find the zeroes for . To do this we simply solve the following equation. ï So, this second degree polynomial has two zeroes or roots. ï Now, letâs find the zeroes for . That will mean solving, ï So, this second degree polynomial has a single zero or root. Also, recall that when we first looked at these we called a root like this a double root. Ex: ï Weâve also got a product of three terms in this polynomial. However, since the first is now an x this will introduce a third zero. The zeroes for this polynomial are, because each of these will make one of the terms, and hence the whole polynomial, zero.
- 9. Multiplying Two Polynomials Examples: ( ) ( )2 5 10 3x x x+ + â = ( )( )2 4 5 3 4x x x+ + â = 3 x 2 10x+ 3xâ 2 5x+ 50x+ 15â 3 x 2 15x+ 47x+ 15â 3 12x 2 16xâ 2 3x+ 4xâ 15x+ 20â = 3 12x 2 13xâ 11x+ 20â Polynomials and Polynomial Functions Multiplication
- 10.  Dividing Polynomials ï In this section weâre going to take a brief look at dividing polynomials. This is something that weâll be doing off and on throughout the rest of this chapter and so weâll need to be able to do this. ï Letâs do a quick example to remind us how long division of polynomials works. ï Example 1  Divide by . ï Solution ï Letâs first get the problem set up ï Recall that we need to have the terms written down with the exponents in decreasing order and to make sure we donât make any mistakes we add in any missing terms with a zero coefficient. ï ï Now we ask ourselves what we need to multiply to get the first term in first polynomial. In this case that is . So multiply by and subtract the results from the first polynomial. ï The new polynomial is called the remainder. We continue the process until the degree of the remainder is less than the degree of the divisor, which is in this case . So, we need to continue until the degree of the remainder is less than 1. ï Recall that the degree of a polynomial is the highest exponent in the polynomial. Also, recall that a constant is thought of as a polynomial of degree zero. Therefore, weâll need to continue until we get a constant in this case. ï Here is the rest of the work for this example. ï Okay, now that weâve gotten this done, letâs remember how we write the actual answer down. The answer is,
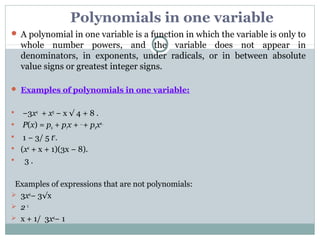
- 11. Polynomials in one variable ï A polynomial in one variable is a function in which the variable is only to whole number powers, and the variable does not appear in denominators, in exponents, under radicals, or in between absolute value signs or greatest integer signs. ï Examples of polynomials in one variable: ï§ â3x4 + x3 â x â 4 + 8 . ï§ P(x) = p0 + p1x + ... + pnxn. ï§ 1 â 3/ 5 t7 . ï§ (x2 + x + 1)(3x â 8). ï§ 3 . Examples of expressions that are not polynomials: ï 3x2 â 3âx ï 2 x ï x + 1/ 3x4 â 1
- 12. âĒ Let p(x) beany polynomial of degree greater than or equal to oneand let a beany real number. If p(x) isdivided by linear polynomial x-athen the reminder isp(a). âĒ Proof :- Let p(x) beany polynomial of degreegreater than or equal to 1. suppose that when p(x) isdivided by x-a, the quotient isq(x) and thereminder isr(x), i.g; p(x) = (x-a) q(x) +r(x) Remindertheorem
- 13. Sincethedegreeof x-ais1 and thedegreeof r(x) isless than thedegreeof x-a,thedegreeof r(x) = 0. Thismeansthat r(x) isaconstant .say r. So , for every valueof x, r(x) = r. Therefore, p(x) = (x-a) q(x) + r In particular, if x = a, thisequation givesus p(a) =(a-a) q(a) + r Which provesthetheorem.
- 14. Example: ï 2x2 -5x-1 divided by x-3 ï f(x) is 2x2 -5x-1 ï g(x) is x-3 ï After dividing we get the answer 2x+1, but there is a remainder of 2 ï q(x) is 2x+1 ï r(x) is 2 ï In the style f(x) = g(x)·q(x) + r(x) we can write: ï 2x2 -5x-1 = (x-3)(2x+1) + 2
- 15. Let p(x) beapolynomial of degree n > 1 and let abeany real number. If p(a) = 0 then (x-a) isafactor of p(x). PROOF:-By thereminder theorem , p(x) = (x-a) q(x) + p(a). FactorTheorem
- 16. 1. If p(a) = 0,then p(x) = (x-a) q(x), which showsthat x-aisafactor of p(x). 2. Sincex-aisafactor of p(x), p(x) = (x-a) g(x) for samepolynomial g(x). In thiscase, p(a) = (a-a) g(a) =0
- 17. factorization of polynomialsFactor 12y2  â 5y. ïIn this case, no number is a common factor between the two terms (specifically, the 12 and the5 share no common numerical factor), but I can still divide out a common variablefactor of "y" from each of the two terms. 12y2  â 5y = y(         ) ïIn the first term, I have the "12" and the other "y" factor left over: 12y2  â 5y = y(12y    ) (This is because 12y2  means 12ÃyÃy, so taking the 12 and one of the y's out front leaves the second y behind.) In the second term, I have the "5" left over: 12y2  â 5y = y(12y â 5) Factor x2  + 4x â x â 4. This polynomial has four terms with no factor common to all four, so I'll try to factor "in pairs": x2  + 4x â x â 4 = x(x + 4) â 1(x + 4) = (x + 4)(x â 1) In the second line above, I factored a "1" out. Why? If "nothing" factors out, a "1" factors out Factor x2  â 4x + 6x â 24. I'll try to factor "in pairs": Copyright ÂĐ Elizabeth Stapel 2002-2011 All Rights Reserved x2  â 4x + 6x â 24 = x(x â 4) + 6(x â 4) = (x â 4)(x + 6)
- 19. THANK YOU