�ݺ�ߣ

Free Vibration Of Plate
Under Free-Free Condition
By
Dipak Prasad (Exam roll: 111204020)
Under the guidance of
Prof. Chaitali Ray
INDIAN INSTITUTE OF ENGINEERING SCIENCE AND TECHNOLOGY
SHIbpUR, HOwRAH- 711103
MAY 2016

INTRODUCTION
 Vibration is the major unacceptable property associated
with any solid material by virtue of its stiffness.
 Vibration is capable of destroying any structure within its
design life due to its feature of high amplitude in
resonance stage.
 Here only free vibration of plate is concerned under free-
free condition.
 As the title suggests no external exciting force is acting on
plate.

OBJECTIVE
Another notable feature of project is free-free condition i.e. each element is free to displaced in
all 6 directions(3 translational and 3 rotational).
Free-freevibrational analysisisused for determination of behavior of airplanesand ships.
Analysishelpsin determination of frequency of different modesof vibration which would help in
designing structuressafefrom view of resonance.

AUTHENTICITY OF RESULT
 Free-free vibration analysis is the simplest technique for checking
authenticity of different software’s results regarding any plate
vibration. Correlation between experimental & theoretical modal
shapes can be done by calculation of MAC. If MAC value lies
between 0.9 to 1, both resultsaresaid to in good correlation.
 Thus it helps in updation of simple FE assumptions regarding
structural geometry, boundary conditions, material propertiesetc.

NUMERICAL ANALYSIS
Numerical analysis is done with help of ANSYS Mechanical APDL
Dimension of sample plate is 270mm×270mm.
Thickness of plate is 5 mm.
Modulus of elasticity of plate is 2x1011 N/m2 and Poisson’s ratio is 0.3.
For free-free condition degree of freedom assumed per node is 6.
Thus element is selected properly which could satisfy the criteria stated above.

ELEMENTPROPERTIES
 Element selected for analysis is shell
8node281.
 8node281 shell element has 8 nodes as shown

ELEMENTPROPERTIES
Shell elements are typically planarelements.
They are used to model thin structures which will experience bending.
Element features: -
6 DOFpernode (3 translations and 3 rotations) for3-Delements.
Bending modes are included.
More than 1 stress at each point on the element.

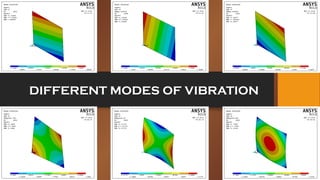
MESHING AND SOLVER
 Plate is divided into 10X10 meshes.
 Mode is extracted by Block Lanczos method.
 No of modes extracted is 12.
 Now solution is done with help of solver.
 Results are obtained by plotting different modes of vibration pattern in general prospect.
 Here first three modes of vibration shows zero frequency and after that consecutive
three modes are showing frequency of 222.79,326.35,404.07 respectively.

RAYLEIGH DAMPING
 [M][+[C][+[K][x]=[0]
 [+2 []+ 2[x]=[0]ξω ω
 [C]= [M]+ [K]α β
 2 = + 2ξω α ω β
 = 0.5[1/ ]ξ ω ω
 [ = 0.5[
 METHOD OF MODE ExTRACTION QR-DAMPED

EXPERIMENTAL SET-UP
An Impact hammer
(B&K type – 8206)
Unidirectional piezoelectric CCLD accelerometer
(B&K type – 4507)
B & K modal analysis set up

EXPERIMENTAL SET-UP
B & K Data acquisition
system (Photon plus)
Freely hanging plate with copper wire Modal analysis software RT pro

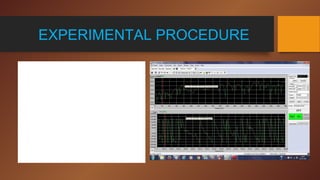
EXPERIMENTAL PROCEDURE
 Here wave pulse shows response of B&K hammer
and plate via CCLD accelerometer after hammering
at a particular node of plate.
 Hammering is said to be perfect if only one peak is
obtained in hammer response.
 Only perfect hammering data is accepted.
 For each node five hammering is done.

EXPERIMENTAL PROCEDURE
 Here wave pulse shows the actual plate
vibration pattern.
 Peak is studied near to frequency
obtained in numerical analysis.
 Only those peaks are valid where phase
angle is 90 or -90
 Data is taken by hammering at various
nodes.

DATA ACQUISITION
Proper dataafter hammering for each nodeisstored in form of
.UNV file.
Experiment on 5 mm thick plateisdoneby dividing it into 5X5
meshesi.e. it consistsof 36 nodes. Henceafter wholeexperiment
36 unv filesarecreated.
All thesedatafilesaregiven asinput in pulse-reflex which in turn
providesnatural frequencies, damping, +z displacement of each
nodefor different modes.

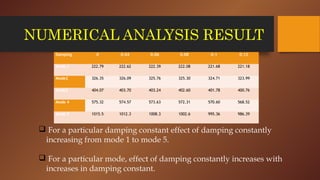
NUMERICAL ANALYSIS RESULT
Damping 0 0.04 0.06 0.08 0.1 0.12
Mode 1 222.79 222.62 222.39 222.08 221.68 221.18
Mode2 326.35 326.09 325.76 325.30 324.71 323.99
Mode3 404.07 403.70 403.24 402.60 401.78 400.76
Mode 4 575.32 574.57 573.63 572.31 570.60 568.52
Mode 5 1015.5 1012.3 1008.3 1002.6 995.36 986.39
 For a particular damping constant effect of damping constantly
increasing from mode 1 to mode 5.
 For a particular mode, effect of damping constantly increases with
increases in damping constant.

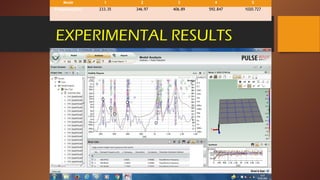
EXPERIMENTAL RESULTS
Mode 1 2 3 4 5
Frequency(Hz) 233.35 346.97 406.89 592.847 1020.727

COMPARISON OF RESULTS
 =( . -Hence error observed for 1st mode of vibration 233 35
. )/ . = . %222 79 222 79X100 4 74
However it is impossible to get exact results from both numerical
.as well as experimental analysis
 % .An error of 10 is permitted
 ,Probable reasons of error may be manual mistake in hammering
improper implementation of exact boundary conditions and
.negligence of damping character of instruments

MODAL ASSURANCE CRITERION
The criteria to be fulfilled by experimental modal
vectors are MAC.
The experimental mode shape should be compatible
with the numerical mode shapes so that they are
consistent in nature.
MAC values provides a quantitative confidence factor
whether the mode shapes obtained experimentally are
in good correlation with those obtained numerically

MAC CALCULATION
MACij =
{Φi}  experimental mode shape vectors
{Ψj}  numerical analysis mode shape vectors

value of MAC varies from 0 to 1.
For good correlation it should lie between 0.9 to 1.

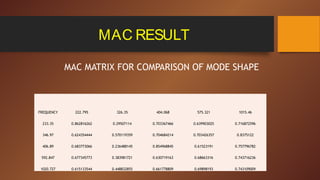
MAC RESULT
MAC MATRIX FOR COMPARISON OF MODE SHAPE
FREQUENCY 222.795 326.35 404.068 575.321 1015.46
233.35 0.862816262 0.29507114 0.703367466 0.639903025 0.716872596
346.97 0.624354444 0.570119359 0.704684214 0.703426357 0.8375122
406.89 0.683773066 0.236488145 0.854968845 0.61523191 0.757796782
592.847 0.677345773 0.383981721 0.630719163 0.68663316 0.743716236
1020.727 0.615133544 0.448832855 0.661778809 0.69898193 0.743109009

OBSERVATIONS
One of the major observation which can be drawn is
that first three modes of vibration shows zero frequency
in numerical analysis.
The probable reason behind such phenomena is
representing the rigid body motion of plate where no
vibration occurred.
Based on available data error observed is 4.74%.

OBSERVATIONS
Best MAC values had been observed for 1st and 3rd mode of
vibration with 0.863 and 0.855 respectively.
Worst MAC value had been observed for 2nd mode of
vibration with 0.57.
Here it should be noticed that inspite of very less error in
frequency values of all five modes, experimental and FE model
can’t be said to be in good correlation from mode shape point
of view.

FUTURE ASPECTS
 Further verification of results is necessary. Experiment should
be revised again and compared with numerical results.
If MAC values are not proper than assumptions regarding
structural geometry, boundary conditions made for numerical
analysis should be revised.
 It is very much necessary that analytical results would be a true
representation of actual behavior of structure not only in view of
frequency but also deflections, bending moment, shear force.

REFERENCES
 https://en.wikipedia.org/wiki/Br%C3%BCel_%26_Kj%C3%A6r
 https://www.researchgate.net/post/which_is_the_best_element_
choice_for_multilayered_FRP_material_in_ANSYS
 https://
www.researchgate.net/post/what_is_the_application_of_freefree_modal_analysis_and_constrained_modal_analysis
 The Modal Assurance Criterion – twenty years of use and abuse, Randall J Allemang,
university of Ohio
 Application of MAC in frequency domain, D. Fotsch and D.J. Ewins, dynamic
section, Mechanical engineering department, Imperial college of Science, Medicine
and Technology, United Kingdom
 Modal Assurance Criterion, Miroslav Pastor, Michal Binda, Tomas Harcarik, Technical
University of Kosice, Faculty of Mechanical Engineering

�ݺ�ߣ

Presentation1 -

More Related Content

Presentation1 -