Root Locus Example
- 1. By Prof. Hitesh Dholakiya Root Locus Example Control Engineering E n g i n e e r i n g F u n d a Engineering Funda Android App Control System YT Playlist
- 2. Root Locus plot Asymptotes Angle of Departure Break away point Outlines of Session Intersection to imaginary axis E n g i n e e r i n g F u n d a Engineering Funda Android App Control System YT Playlist
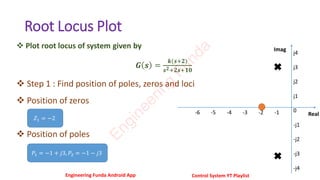
- 3. Root Locus Plot ? Plot root locus of system given by ? ? = ?(?+?) ??+??+?? ? Step 1 : Find position of poles, zeros and loci ? Position of zeros ? Position of poles ?1 = ?2 ?1 = ?1 + ?3, ?2 = ?1 ? ?3 Imag j4 j3 j2 j1 0 -j1 -j2 -j3 -j4 Real -6 -5 -4 -3 -2 -1 E n g i n e e r i n g F u n d a Engineering Funda Android App Control System YT Playlist
- 4. Asymptotes ? Find Number of Asymptotes, Centroid of Asymptotes and Angle of Asymptotes ? Number of Asymptotes ? Centroid of Asymptotes ? Angle of Asymptotes Imag j4 j3 j2 j1 0 -j1 -j2 -j3 -j4 Real -6 -5 -4 -3 -2 -1 Number of Asymptotes = P ĻC Z = 2 ĻC 1 = 1 ? ? = ?(? + ?) ?? + ?? + ?? ?1 = ?2 ?1 = ?1 + ?3, ?2 = ?1 ? ?3 ?? = ĶŌ ???? ????? ?? ????? ? ĶŌ ???? ????? ?? ????? ? ? ? ?? = ?1 ? 1 ? (2) 2 ? 1 = 0 ? = (2? + 1) ? ? ? ĄÁ 1800 = 1800 ?? = 0 ? =180 E n g i n e e r i n g F u n d a
- 5. Angle of Departure ? Angle of Departure Imag j4 j3 j2 j1 0 -j1 -j2 -j3 -j4 Real -6 -5 -4 -3 -2 -1 ?? = 0 ? =180 ?? = 180 ? ? ????? ?? ???? ? ? ????? ?? ????? ?? = 180 ? ??1 ? ??1 = 180 ? 90 ? 71 = 161 ???= 90 ???= 71 E n g i n e e r i n g F u n d a
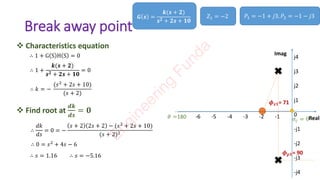
- 6. Break away point ? Characteristics equation ? Find root at ?? ?? = ? Imag j4 j3 j2 j1 0 -j1 -j2 -j3 -j4 Real -6 -5 -4 -3 -2 -1 ?? = 0 ? =180 ???= 90 ???= 71 Ąā 1 + G S H S = 0 ? ? = ?(? + ?) ?? + ?? + ?? ?1 = ?2 ?1 = ?1 + ?3, ?2 = ?1 ? ?3 Ąā 1 + ?(? + ?) ?? + ?? + ?? = 0 Ąā ? = ? (?2 + 2? + 10) (? + 2) Ąā ?? ?? = 0 = ? ? + 2 2? + 2 ? (?2 + 2? + 10) (? + 2)2 Ąā 0 = ?2 + 4? ? 6 Ąā ? = 1.16 Ąā ? = ?5.16 E n g i n e e r i n g F u n d a
- 7. Inter section to imaginary axis ? As per Diagram You donĄŊt need to plot Intersection to imaginary axis E n g i n e e r i n g F u n d a