Run diff pp update1
- 1. Why Does a Team Outperform its Run Differential? Greg Ackerman Syracuse University Sabermetrics Club
- 2. SU Sabermetrics Club âĒ SABR Student Group Affiliate âĒ Justin Mattingly âĒ Joey Weinberg âĒ Colby Conetta âĒ Ray Garzia âĒ Mallory Miller âĒ Zack Potter âĒ Marcus Shelmidine âĒ Brandon Love âĒ Matt Tanenbaum âĒ Bryan Kilmeade âĒ Justin Moritz âĒ Stephen Marciello âĒ Kyle OâConnor âĒ Willie Kniesner âĒ Michael Rotondo âĒ Matt Russo âĒ Sam Fortier âĒ Matt Filippi âĒ Isaac Nelson âĒ Zack Albright âĒ John Van Ermen âĒ Colton Smith âĒ Chris Karasinski âĒ Zach Tornabene
- 3. Basic Premise âĒ Explain the Difference between Actual Win Percentage and Expected Win Percentage based on Run Differential (Expected Win Percentage based upon Pythagenpat formula from run differential) âĒ X = ((runs scored + runs against)/games)^.285 âĒ If achieve run differential to possibly put team in playoffs â do not want to squander it âĒ If borderline run differential for playoffs â could be difference in attaining playoff spot âĒ Will focus on 3 key factors that may influence teams outperforming or underperforming their run differential âĒ Performance of Bench âĒ Relief Pitching âĒ Pitching Depth âĒ Part II â Add managerial decisions to the model âĒ Pinch Hitters Used âĒ Defensive Substitutions âĒ Relievers Used âĒ Etc.
- 4. Charts âĒ Average of (Actual Win % - Expected Win %) âĒ Standard Deviation of (Actual Win % - Expected Win %) âĒ Variables calculated from www.baseball-reference.com âĒ Team Examples of Difference in Actual Win % - Expected Win % âĒ San Francisco Giants âĒ St. Louis Cardinals âĒ New York Yankees âĒ Toronto Blue Jays âĒ Colorado Rockies
- 5. -0.02 -0.015 -0.01 -0.005 0 0.005 0.01 0.015 0.02 0.025 Average of Actual Win% - Expected Win% - By Team - 2000-2013
- 6. 0 0.005 0.01 0.015 0.02 0.025 0.03 0.035 0.04 Standard Deviation of Actual Win% - Exp. Win% - By Team â 2000-2013
- 7. -0.02 -0.01 0 0.01 0.02 0.03 0.04 0.05 2000 2001 2002 2003 2004 2005 2006 2007 2008 2009 2010 2011 2012 San Francisco Giants - Difference in Actual and Expected Win Percentage
- 8. -0.04 -0.03 -0.02 -0.01 0 0.01 0.02 0.03 0.04 0.05 2000 2001 2002 2003 2004 2005 2006 2007 2008 2009 2010 2011 2012 2013 St. Louis Cardinals - Difference in Actual and Expected Win Percentage
- 9. -0.04 -0.02 0 0.02 0.04 0.06 0.08 2000 2001 2002 2003 2004 2005 2006 2007 2008 2009 2010 2011 2012 2013 New York Yankees - Difference in Actual and Expected Win Percentage
- 10. -0.08 -0.06 -0.04 -0.02 0 0.02 0.04 0.06 2000 2001 2002 2003 2004 2005 2006 2007 2008 2009 2010 2011 2012 2013 Toronto Blue Jays â Difference in Actual and Expected Win Percentage
- 11. -0.06 -0.05 -0.04 -0.03 -0.02 -0.01 0 0.01 0.02 0.03 2000 2001 2002 2003 2004 2005 2006 2007 2008 2009 2010 2011 2012 2013 Colorado Rockies - Difference in Actual and Expected Win Percentage
- 12. Measures of Bench (Hitters) Performance âĒ OPS+ - On-Base Average plus Slugging Percentage â Adjusted for Park and League âĒ HR â Home Runs âĒ SB â Stolen Bases âĒ CS â Caught Stealing âĒ Calculated from Baseball Reference â using only bench players listed for each team â weighted average based upon plate appearances of each player âĒ Ultimately, only included OPS+ âĒ Other variables did not add statistical value to the regression model beyond OPS+
- 13. Measure of Relief/Depth Pitcher Performance âĒ FIP â Fielding Independent Pitching âĒ ERA+ - Earned Run Average adjusted for ballpark âĒ SO/W â strike out to walk ratio âĒ Calculated as a weighted average based upon innings pitched âĒ Calculated for group of ârelieversâ noted on Baseball-Reference â includes closer and top 4 used relievers âĒ Calculated for group of âdepthâ pitchers noted on Baseball Reference- includes pitchers not included in âstartersâ or ârelieversâ categories
- 14. Regression Model I âĒ After different regression model incarnations â settled upon the following to illustrate results: âĒ (Actual â Expected Win %)i = Îą0 +Îē1 (Bench OPS+) + Îē2 (Relief variable) + Îē3 (Pitching Depth variable) + Îĩi
- 15. Regression Results â (Actual Win% - Pyth Win%) on Bench and Relief Pitching Performance I II III Intercept 0.0206 (1.4307) Intercept 0.0071 (0.5750) Intercept -0.0102 (-1.0729) OPS+ - Bench 0.00001 (0.1104) OPS+ - Bench 0.00002 (0.1911) OPS+ - Bench 0.00002 (0.1795) FIP â Relief -0.0044** (-1.9130) ERA â Relief -0.0026 (-1.5358) KBB â Relief 0.0014 (1.3735) FIP â Depth -0.0009 (-0.4956) ERA â Depth 0.0001 (0.0756) KBB â Depth 0.0007 (0.7328)
- 16. Results âĒ Variables have expected signs for bench (hitter) performance, relief pitching, and pitching depth âĒ Only statistically significant result is for relief pitching performance âĒ Specifically â FIP âĒ FIP has a negative and significant impact on (Actual Win Percentage â Exp. Win Percentage) âĒ As FIP increases â has negative impact on dependent variable âĒ More likely to underperform run differential âĒ As FIP decreases â has positive impact on dependent variable âĒ More likely to outperform run differential
- 17. Sample Bench OPS+ Relief FIP Depth FIP top 10% Seasons - Outperform Run Diff 81.56304 3.66215 4.691264 Bottom 10% Seasons - Underperform Run Diff 81.63799 3.981814 4.769715 % Differential Between Samples -0.09% -8.03% -1.64%
- 18. 65 70 75 80 85 90 Average of Bench OPS+
- 19. 0 1 2 3 4 5 6 FIP - Relief and Pitching Depth FIP-Relief FIP-Depth
- 20. Managerial Decisions âĒ For Next Step: Added Managerial Decisions to the Data Set âĒ To measure managerial decisions â used the Bill James Handbook âĒ Attempt to measure the impact of various managerial decisions on the ability to outperform (underperform) a teamâs run differential
- 21. Managerial Decision Variables âĒ Pinch Hitters Used âĒ Pinch Runners Used âĒ Defensive Substitutions âĒ Relief Pitchers: Innings Pitched âĒ Stolen Bases Attempted âĒ Sacrifices Attempted âĒ Pitch Outs Ordered
- 22. Regression Model II âĒ (Actual â Expected Win %)i = Îą0 +Îē1 (Bench OPS+) + Îē2 (Relief FIP) + Îē3 (Pitching Depth FIP) + Îē4 (Pinch Hitters) + Îē5 (Pinch Runners) + Îē6 (Defensive Substitutions) + Îē7 (Relief Innings) + Îē8 (SB Attempts) + Îē9 (SAC Attempts) + Îē10 (Pitch Outs) + Îĩi
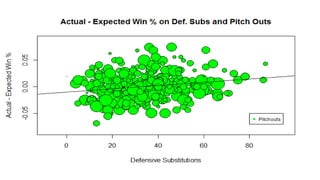
- 23. Variable Coefficient Variable Coefficient Intercept 0.0200 (0.9657) Defensive Substitutions 0.0003*** (3.5602) OPS+ - Bench -0.00003 (-0.2786) Relief Innings Pitched -0.00004 (-1.4258) FIP â Relief -0.0023 (-0.9656) Stolen Bases Attempted 0.000007 (0.3458) FIP â Depth 0.0012 (0.5889) Sacrifices Attempted -0.00002 (-0.2871) Pinch Hitters Used -0.000004 (-0.1621) Pitch Outs Ordered -0.0002** (-2.0751) Pinch Runners Used 0.00004 (0.4072)
- 24. Results âĒ Two statistically significant managerial variables: âĒ Defensive Substitutions â (+) â significant at the 1% level âĒ Pitchouts Ordered â (-) â significant at the 5% level âĒ Defensive Substitutions â more defensive substitutions used â greater likelihood to outperform run differential âĒ Part is managerial decision âĒ Part is roster flexibility âĒ Pitchouts Ordered â more pitchouts ordered â greater likelihood to underperform run differential âĒ Part is wasting a pitch âĒ Part is lack of faith in catcher/pitcher âĒ Likely a proxy for risk averse behavior on part of manager
- 25. Results âĒ Relief Pitcher Innings Pitched â (-) but not quite statistically significant (15% level) âĒ When Managerial Statistics included â impact of FIP-Relievers is lessened as well â no longer statistically significant âĒ Tried including one or the other â not quite statistically significant âĒ Appears to still have some marginal effect on ability to outperform/underperform run differential
- 26. Sample Defensive Substitutions Relievers Used Pitch Outs top 10% Seasons - Outperform Run Diff 36.0732 444.0732 17.2927 Bottom 10% Seasons - Underperform Run Diff 26.1892 458.7222 21.5000 % Differential Between Samples 37.74% -0.03% 19.57%
- 28. 0 5 10 15 20 25 30 35 40 45 50 Average of Defensive Substitutions Used
- 29. 0 5 10 15 20 25 30 35 40 Average of Pitchouts Ordered
- 30. Conclusions âĒ Aimed to determine why teams outperform/underperform run differential âĒ Is it just luck? â or are there factors that contribute to its explanation? âĒ Without Manager Data â it appears that Relief Pitcher Performance (measured by FIP) plays an important role âĒ Increase in FIP by Relievers â more likely to underperform âĒ Decrease in FIP by Relievers â more likely to outperform âĒ With Manager Data âĒ Defensive Substitutions â more defensive subs â more likely to outperform âĒ Pitchouts â likely proxy for risk aversion (poor catching performance?) â more pitchouts â more likely to underperform run differential âĒ Starting point of our research â hope to learn more in future â open to different variables/approaches to help determine answers
Editor's Notes
- #4: We are not looking at starting pitching and starting lineup, rather we are looking at performance of bench, relief pitching, and pitching depth.
- #6: Talk about the Yankees, Giants, and Cardinals having high averages and have been outperforming their run differential between 2000 and 2013, while teams like the Blue Jays, Rockies, and
- #7: One interesting thing here is that the Giants have the lowest standard deviation between 2000 and 2013
- #8: 0 is what we would expect, so the Giants have regularly outperformed their run differential
- #12: Last of team group
- #13: The key to this is that we used a weighted average for each team amongst bench hitters only, according to baseball-reference. We are really only going to be focusing on OPS+ because it is the most promising of the bench statistics.
- #14: This is a weighted average of FIP, ERA+, and SO/BBW. We used
- #15: The dependent variable here is actual â expected win percentage. The independent variables are bench OPS+, and a variable amongst FIP, ERA, or strikeout to walk ratio amongst the top five relievers and depth pitching.
- #16: These are all very small numbers because we are dealing with trying to explain a very small number, which is the difference between actual and expected win percentage.
- #17: This makes sense because if you are not pitching well in late innings, you are likely to underperform your run differential, while if you have good Fielding Independent Pitching amongst relievers, they are more likely to outperform their run differential.
- #18: This slide shows the best seasons and worst seasons in terms of outperforming and overperforming run differential. The only place we have a sizeable difference between the top 10% and bottom 10% of seasons is in relief FIP, as there is a 8.03% dropoff.
- #19: This just gives an idea of the average bench OPS+ amongst the team.
- #20: This is the difference between relief and depth FIP.