Schk
Download as ppt, pdf0 likes313 views
The document defines and describes several types of numbers: Natural numbers (N) are the counting numbers 1, 2, 3, etc. with no greatest number. Whole numbers (W) include 0. Integers (Z) include both positive and negative numbers. Rational numbers (Q) are numbers that can be written as p/q where p and q are integers. Irrational numbers cannot be written as p/q. Real numbers (R) include all rational and irrational numbers. The number line represents all real numbers as unique points. Ancient Greek mathematicians like Pythagoras, Cantor and Dedekind studied properties of rational and irrational numbers and their representation on the number line. Decimal expans
![NATURAL NUMBERS
The set of natural numbers is denoted by
N. Therefore N=[1,2,3]
The least number is 1.
The greatest number is Infinite.
Natural numbers are also called counting
numbers.](https://image.slidesharecdn.com/schk-130327085440-phpapp02/85/Schk-4-320.jpg)
![WHOLE NUMBERS
The set of whole numbers is denoted by W.
Therefore W=[0,1,2,3]
The least number is 0.
The greatest number is Infinite.](https://image.slidesharecdn.com/schk-130327085440-phpapp02/85/Schk-5-320.jpg)
![INTEGERS
The set of integers is denoted by I or Z.
Z=[-3,-2,-1,01,2,3...]
It is the collection of positive and negative
numbers.](https://image.slidesharecdn.com/schk-130327085440-phpapp02/85/Schk-6-320.jpg)
![RATIONAL NUMBERS
The numbers which are in the form of p / q.
(q is not equal to 0) were p , q are integers
are called rational numbers.
It is denoted by Q. therefore Q=[p / q (p
not equal to 0)].EX:3/4,1/2,-3/2.](https://image.slidesharecdn.com/schk-130327085440-phpapp02/85/Schk-7-320.jpg)
Schk
- 4. NATURAL NUMBERS The set of natural numbers is denoted by N. Therefore N=[1,2,3] The least number is 1. The greatest number is Infinite. Natural numbers are also called counting numbers.
- 5. WHOLE NUMBERS The set of whole numbers is denoted by W. Therefore W=[0,1,2,3] The least number is 0. The greatest number is Infinite.
- 6. INTEGERS The set of integers is denoted by I or Z. Z=[-3,-2,-1,01,2,3...] It is the collection of positive and negative numbers.
- 7. RATIONAL NUMBERS The numbers which are in the form of p / q. (q is not equal to 0) were p , q are integers are called rational numbers. It is denoted by Q. therefore Q=[p / q (p not equal to 0)].EX:3/4,1/2,-3/2.
- 8. IRRATIONAL NUMBERS A number S is called Irrational , if it cannot be written in the form of p / q (q is not equal to 0,were p and q are integers . There are Infinite many Irrational numbers. Examples-(0.1011011101111)
- 9. REAL NUMBERS It is the collection of rational and irrational numbers. Every real number is represented by a unique point on the number line. Also every point on the number line represents a unique real number.
- 10. NUMBER LINE -5 -4 -3 -2 -1 0 1 2 3 4 5 Number line is the collection of positive and negative numbers.
- 11. PYTHAGORAS(569BC-479BC) The Pythagoras in Greece, followers of the famous mathematician and philosopher Pythagoras, were the first to discover the numbers which were not rational, around 400BC these numbers are called irrational numbers, because they cannot be written in the form of a ratio of integers
- 12. In the 1870s two German mathematicians , Cantor and Dedekind , showed that corresponding to every real number , there is a point on the real number line, and corresponding to every point on the DEDEKIND.R CANTOR.G number line , there exists a unique real number
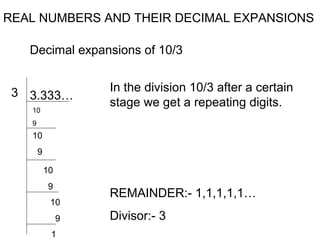
- 13. REAL NUMBERS AND THEIR DECIMAL EXPANSIONS Decimal expansions of 10/3 3 3.333 In the division 10/3 after a certain stage we get a repeating digits. 10 9 10 9 10 9 REMAINDER:- 1,1,1,1,1 10 9 Divisor:- 3 1
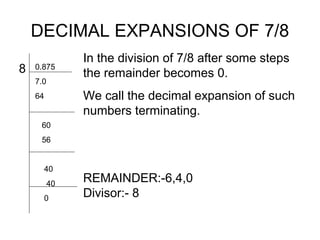
- 14. DECIMAL EXPANSIONS OF 7/8 In the division of 7/8 after some steps 8 0.875 the remainder becomes 0. 7.0 64 We call the decimal expansion of such numbers terminating. 60 56 40 40 REMAINDER:-6,4,0 0 Divisor:- 8
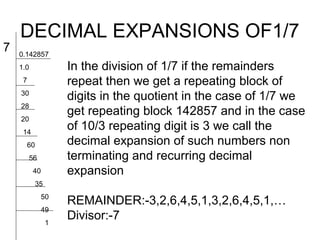
- 15. DECIMAL EXPANSIONS OF1/7 7 0.142857 1.0 In the division of 1/7 if the remainders 7 repeat then we get a repeating block of 30 digits in the quotient in the case of 1/7 we 28 get repeating block 142857 and in the case 20 14 of 10/3 repeating digit is 3 we call the 60 decimal expansion of such numbers non 56 terminating and recurring decimal 40 expansion 35 50 REMAINDER:-3,2,6,4,5,1,3,2,6,4,5,1, 49 1 Divisor:-7
- 16. REPRESENTING THE REAL NUMBERS ON THE NUMBER LINE
- 17. OPERATIONS ON REAL NUMBERS The sum or difference of a rational number and an irrational number is a irrational . The product or quotient of a non-zero rational number with an irrational number is irrational If we add or subtract, multiply or divide two irrationals, the result may be irrational or rational.
- 18. ARCHIMEDES The Greek genius Archimedes was the first to compute digits in the decimal expansion of 22/7 he showed 3.140845 <22/7<30142857.aryabhat ta , the great mathematician, found the value of 22/7 correct to four decimal places (3.1416).