�ݺ�ߣ

Selected problems of
maritime traffic risk modelling
Pentti Kujala, Professor
Jakub Montewka, Ph.D., Chief Mate
Aalto University, Finland

Przemysław Krata, Ph.D.
Maritime University of Gdynia, Poland

Stockholm, 28-29 January 2010

Agenda

Risk modelling - outline

Probability of an accident

Consequences

A case study

Selected problems of maritime traffic risk modelling

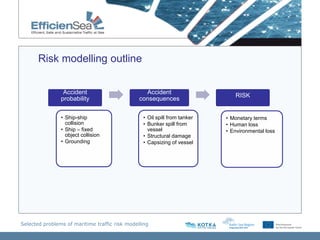
Risk modelling outline

P C R
P – accident‟s probability
C – accident‟s consequences
R – risk


Risk modelling outline

Accident Accident
RISK
probability consequences

• Ship-ship • Oil spill from tanker • Monetary terms
collision • Bunker spill from • Human loss
• Ship – fixed vessel • Environmental loss
object collision • Structural damage
• Grounding • Capsizing of vessel


Accident‟s probability assessment
Ship-ship collision Ship-fixed object Grounding models
models collision models

Fujii, Macduff, Fujii, Macduff,
1974 Gluver&Olsen „98 1974

Kite – Powell,
Pedersen, 1995 U. Kunz, 1998 1999

MDTC based
M. Knott, 1998 Fowler, 2000
model, 2010

Z. Prucz, 1998 Quy, 2007

Gravity model,
2010


Collision probability assessment – MDTC based model

Figure
The relationships between
MDTC, safe passing
distance, and collision

Figure
Representation of vessels
as discs and definition of
collision situation.


Collision probability assessment – MDTC based model
MDTC (LOA)

Tanker_Tanker
5 Tankers_cd
Tanker_Pass
Tanker_Pass_cd
4 Pass_Cont
Pass_Cont_cd
Cont_diff
3 Cont_diff_cd

MDTC (LOA)
RoRo_RoRo 6
RoRo_cd
2 Cont_Cont
Cont_cd 5
Tankers_diff
1
Tankers_diff_cd 4
Pass_Pass
0 Pass_Pass_cd
3
10 30 50 70 90 110 130 150 170
Angle of intersection (deg) 2

Figure 1
Values of MDTC obtained for all meeting scenarios,
with corresponding values of collision diameters. 0
0 20 40 60 80 100 120 140 160 180
Angle of intersection (deg)
1_port_2_stb Both_to_port CD
Figure
MDTC and CD’s values computed at 95%
confidence level by use of Monte Carlo simulations.


Collision probability assessment – causation factor


Grounding probability assessment – gravity model
The field of characteristics of ships location:

S S (T( j ,i ) , R, d ( R,e,m) )
T - maximum draught of a ship,
R - turning circle radius,
d - coefficient of the effective distance of obstruction detecting
e - coefficient describing a technical equipment of a ship,
m - coefficient of ship‟s manoeuvrability,
j, i - denotes coordinates of ship.

The field of characteristics of the obstructions:

P P( H ( j ',i ') , b( j ',i ') , s( j ',i ') , c( j ',i ') )
H - water depth,
s - coefficient of soundings accuracy,
b - coefficient of ship‟s hull destruction when contacted with the seabed,
c - coefficient of soundings position accuracy.


The grounding threat intensity at any arbitrarily chosen point of the space
containing any number of sources of a threat (eg.: shallows) can be obtained as
a vector sum of grounding threat intensities coming from every single obstruction
according to the formula:

np
E ( j, i) Ek
k 1

Ē(j,i) - is a grounding threat intensity field in the point (j, i),
Ē k - is a grounding threat intensity vector generated by k-numbered obstruction,
np - is a number of obstructions located in considered area.


A spatial distribution of values of the grounding threat intensity vectors.
A shape of a safety contour (blue) depends on the assumption regarding the acceptable value of
the grounding threat intensity vectors in the closest point of shallow approach.
The critical value adjustment was performed on the basis of a minimum under keel clearance
(UKC) requirement.

Blue means safety Centre of fairway


Accident‟s consequences assessment

Quantity of oil spill Cost of oil spill Structural damage Ship capsizing

IMO methodology
Munif et al.
MEPC 117(52) 2004 Etkin, 2000 Pedersen, 1994
2005
MEPC 110(49) 2003

Skjong et al. 2005 Bulian et al.
Smailys & Česnauskis, Brown, 2002
2009
2006 in SAFEDOR
project

In house build model Zhang, Hinz, 2010
based on the two
above mentioned,
2009 Yasuhira, 2009
In house build
model, based on
Zhang‟s approach
and AIS data2010


Size of an oil outflow due to collision and grounding considering there is a spill as
a function of cargo deadweight as calculated by IMO probabilistic methodology for
double hull tankers only.

Collision

Grounding


Accident‟al oil outflow model for double hull tankers in the Gulf of Finland
Number of ships

Monthly tanker traffic profiles

Length (m)
350
600
300
500
250

400 200

300 150

200 100

100 50

0 0
Gas Crude oil Oil products Chemical mode max min
Tanker Gas Crude oil Oil products Chemical
Winter Summer
DWT (tons)

Tanker's DWT as a function of her length
180000

160000

140000

120000 y = 0,0015x3,3008
2
R = 0,9577
100000

80000

60000

40000

20000

0
50 70 90 110 130 150 170 190 210 230 250 270 290
Length (m)


Accident‟al oil outflow model for double hull tankers in the Gulf of Finland

X > 21125
Pareto2(9009.10; 1.90) Shift=+3.04 X > 34485 Pareto2(49459; 8.4) Shift=-3.16
5.0%
5.0%
Probability

2,0E-04

Probability
1,5E-04

1,5E-04

1,0E-04
1,0E-04

5,0E-05
5,0E-05

0,0E+00 0,0E+00
0 10000 20000 30000 40000 50000 0 10000 20000 30000 40000 50000
Spill size [t] Spill size [t]

The probability of an oil spill from the tankers operating in the Gulf of Finland in case of collision,
estimated by Pareto2 distributions for summer (to left) and winter traffic (to right).


A case study
Block diagram of risk assessment process applied in the study.


A case study
1. Helsinki-Tallinn crossing for summer and winter traffic.

2. Approach to oil terminal in Sköldvik


A case study
Cumulative density functions of risk due to tankers collisions in the Helsinki-
Tallinn crossing for summer and winter traffic.

X <0.43
Lognorm(123682; 246804) Shift=-1123.4 X > 444368
95% 5.0%

Probability
1
Probability

1
Mean = 122559
0,8 Summer 0,8
Mean=0.19
0,6 0,6
Winter
0,4 Mean=0.14 0,4

0,2 0,2

0 0
0 0,25 0,5 0,75 1 0 0,1 0,2 0,3 0,4 0,5 0,6
RISK [USD*Million] RISK [USD*Millions]


A case study
The safety contours of the analyzed fairway to Sköldvik (red and green curves)
and the fairway centre line (black straight line).

60,15
Latitude [deg N]

60,14
Histograms of tankers' lateral distribution on fairway
60,13 leading to Sköldvig

Probability
0,0020
60,12
0,0015
60,11
0,0010
60,1

60,09 0,0005

60,08 0,0000
-750 -500 -250 0 250 500 750 1000 1250
60,07 Distance from waterway center [m]
S_bound N_bound
60,06
25,5 25,52 25,54 25,56 25,58 25,6 25,62
Longitude [deg E]
Two histograms of tankers‟ lateral distribution on the fairway to
Sköldvig, red line represents north bound traffic whereas black
line is south bound traffic
The safety contours


A case study
Probability and cumulative density functions of variable “risk” in case of grounding in
the Sköldvik harbour approach, summer traffic.

Lognorm(123682; 246804) Shift=-1123.4 X > 444368 Lognorm(123682; 246804) Shift=-1123.4 X > 444368
5,0% 5.0%
Probability

Probability
1,2E-05 1
Mean = 122559
1,0E-05
Mean = 122559 0,8

8,0E-06
0,6
6,0E-06
0,4
4,0E-06

2,0E-06 0,2

0,0E+00 0
0 0,1 0,2 0,3 0,4 0,5 0,6 0 0,1 0,2 0,3 0,4 0,5 0,6
RISK [USD*Millions] RISK [USD*Millions]


Thank you for your attention


�ݺ�ߣ

Selected Problems Of Marine Traffic Risk Modelling

More Related Content

Selected Problems Of Marine Traffic Risk Modelling