Singular matrix
?
1 like?173 views
A matrix is singular if its determinant is equal to 0. A 3x3 matrix is provided as an example of a singular matrix, where the calculation of the determinant equals 0. Therefore, this matrix is singular. A second example shows that if a 2x1 matrix is pre-multiplied by a singular 2x2 matrix, then the value of x must be 0.
Singular matrix
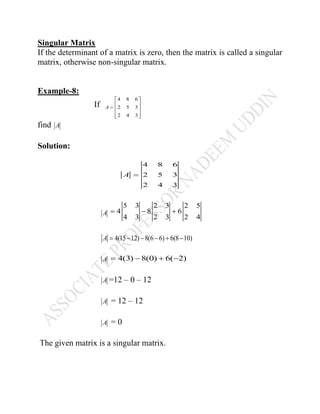
- 2. Singular Matrix If the determinant of a matrix is zero, then the matrix is called a singular matrix, otherwise non-singular matrix. Example-8: If 4 8 6 2 5 3 2 4 3 A = ? ? ? ? ? ? ? ?? ? find A Solution: 4 8 6 2 5 3 2 4 3 A = A 5 3 2 3 2 5 4 8 6 4 3 2 3 2 4 = ? + 4(15 12) 8(6 6) 6(8 10)A = ? ? ? + ? A 4(3) 8(0) 6( 2)= ? + ? A =12 ¨C 0 ¨C 12 A = 12 ¨C 12 A = 0 The given matrix is a singular matrix.
- 3. Example-9: 5 5 a singular matrix then find the value of x 2 IF A is x ? ? = ? ? ? ? Solution: 5 5 2 10 5 0 10 5 5 10 10 5 2 A x A x x x x x = = ? = ? = = =