Solving quadratic equations
Download as PPTX, PDF
0 likes435 views
A quadratic equation is an equation of the form ax^2 + bx + c, where a cannot equal 0. There are three main methods for solving quadratic equations: factoring, completing the square, and using the quadratic formula. Factoring works when the equation can be rewritten as a product of two binomial expressions. Completing the square is used when factoring is not possible. It involves rearranging terms in the equation into a perfect square trinomial. The quadratic formula, ax^2 + bx + c = 0, can be used to solve any quadratic equation by substituting the values of a, b, and c.
Solving quadratic equations
- 2. What is a quadratic equation? The name quadratic zome from squad meaning square, the variable get squared (over 2) The standard form of a quadratic equation is: ax卒2 + bx + c The assumptions are: a, b and c are known values. a can't be 0. "x" is the variable or unknown (you don't know it yet).
- 4. How to solve quadratic equations There are 3 ways to find the solutions: 1. You can Factor the Quadratic (find what to multiply to make the Quadratic Equation) 2. You can Complete the Square 3. You can use the special Quadratic Formula:
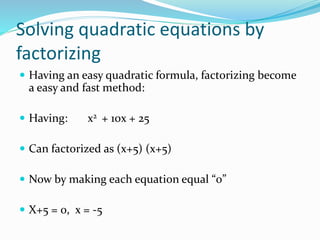
- 5. Solving quadratic equations by factorizing Having an easy quadratic formula, factorizing become a easy and fast method: Having: x2 + 10x + 25 Can factorized as (x+5) (x+5) Now by making each equation equal 0 X+5 = 0, x = -5
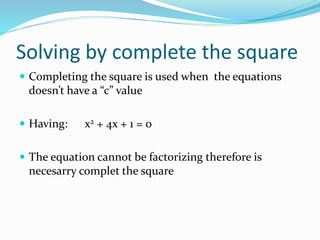
- 6. Solving by complete the square Completing the square is used when the equations doesnt have a c value Having: x2 + 4x + 1 = 0 The equation cannot be factorizing therefore is necesarry complet the square
- 7. Step 1: Passing to the other side the c value x2 + 4x = -1 Step 2: Using the formula (B/2)2 obtain the real c value (4/2)2 = 4 Step 3 : Sum the c value to both sides of the equation. x2 + 4x + 4 = -1+ 4 Step 4: Factorize the trinomial equation (x+2)2 = 3 Solve the quation by square root
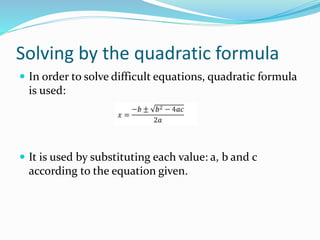
- 8. Solving by the quadratic formula In order to solve difficult equations, quadratic formula is used: It is used by substituting each value: a, b and c according to the equation given.
- 9. *Completing the Square (Completing the Square) http://www.mathsisfun.com/algebra/completing-square.html *Quadratic equations. (2000, January 1). . Retrieved May 29, 2014, from http://mathworld.wolfram.com/QuadraticEquation.html