Spinner velosity tool
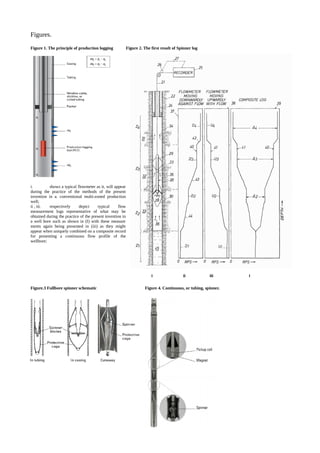
- 1. STID 92250744 Velocity Tools in Production Logging. A. Nasirisavadkouhi, International Campus of Sharif University of Technology Winter 2015 This paper was prepared for the advanced well-log course held by Dr Saeed Shad at SUT_international Campus. The template of the paper is based on the latest SPE standards therefore the pictures can be found at the end of the paper. Abstract Production logs are a record of one or more in-situ measurements that describe the nature and behavior of fluids in or around the borehole during production or injection. The instrument ''spinner'' which is a device for measuring in situ the velocity of fluid flow in a production or injection well based on the speed of rotation of an impeller, was introduced in this paper. Its history and its types were mentioned. Then I discussed some concepts of its working and the reasons behind it. Eventually a method of graphical spinner log interpretation was explained. Fundamentals of Production Logging The aim of production logging is to determine where the oil and gas and water are coming from in a producing well or to determine where the gas or water is going to in an injection well. Because the radial influx (or outflux) of these phases into the borehole cannot be directly measured, production logging looks for intervals of stable or unchanging flow rate (q) and then calculates the differences between adjacent stable intervals. From a few basic sensors, production logging tools have evolved to a family of tools each with sensors designed to make measurements that, once interpreted together, provide accurate flow rates for multiple phases and determine precisely where the various fluids are entering (or exiting) the borehole. This development and application of new production logging technologies is much needed, as well trajectories continue to grow in complexity, progressing from vertical to deviated and horizontal and posing new challenges in completion design and flow assurance. Downhole separators and positive displacement meters are not used to measure the stable downhole flow rates; instead, velocities, areas, void fractions, and other indirect attributes of the flow rate must be measured. Most of the measurements available have limited ranges within which a calibrated response can be expected. It would be fair to say that the task of converting downhole measurements into a multiphase flow rate and then accurately determining where the various fluids are entering the borehole presents a challenge, a nontrivial task, or to use plain, noneuphemistic language, a problem. The objective of this paper is to familiarize readers with this tool and moreover to help them address the difficulties production logging faces in this area. Therefore, this paper reviews the concepts with regards to the function of the Spinner. The History and Evolution of the Spinner As described in Bonet (Pat. US3,630,078) one of the most successful techniques presently employed for determining the flow rate of fluids flowing in a well bore is to successively pass a so-
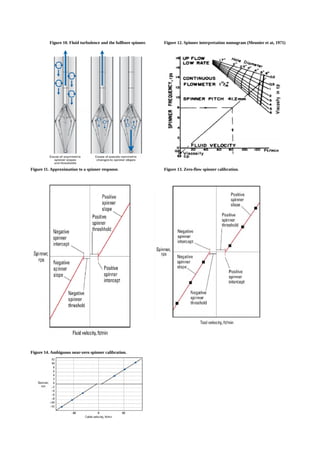
- 2. called "spinner-type" flowmeter tool at selected constant speeds through the fluid-filled well bore. By successively recording the resulting rotational speeds of the flowmeter spinner at the corresponding depth locations of the tool, a continuous flow survey or fluid-velocity log will be obtained from which the flow rates of the well bore fluids at different depth intervals of the well bore can be readily determined. Thus, where the well being surveyed is a production well having two or more producing intervals, the resulting log will clearly indicate the respective velocities or flow rates of the connate fluids which are being produced from each of the several producing intervals. On the other hand, where the well is an injection well in which fluids are being injected simultaneously into two or more formation intervals, the resulting flow survey will show what portion of the injection fluid is entering each formation interval. Those skilled in the art will, of course, appreciate that the rotational speed of the spinner in a flowmeter of this nature is simply a linear function of the apparent or relative velocity of the well bore fluids in relation to the tool. Thus, at low flow rates, the spinner will inherently turn at correspondingly-low speeds so long as the well bore fluids are moving at a sufficient velocity to still turn the spinner. It is apparent, therefore, that for a given spinner, the minimum "operating threshold" for such a flowmeter is directly related to the amount of rotational friction which must be overcome before the spinner can turn. Accordingly, as fully explained in the Bonnet, it was found that the unique magnetic suspension arrangement shown there significantly reduced the static and dynamic friction affecting the rotation of the flowmeter spinner. Although flowmeters arranged in accordance with the Bonnet patent have met with considerable success, it has been found nevertheless that their loose-fitting magnetically-suspended spinners become unreliable, if not ineffective, in substantially deviated or non-vertical well bore interval. Attempts to use bushings or bearings of different types for maintaining these spinner shafts coaxially centered in the tool have not been too successful, however, in view of the additional friction. Moreover, fluid-borner debris such as sand grains or other solid particles will often enter the clearance spaces around or in such bearings and impair, if not totally disrupt, the operation of these bearings as well as other closely fitted movable elements of the flow meter. Eventually innovations were introduced by (Anderson et al. US3,954,006) the first results are shown in (Fig 2) . Nowadays turbine, or spinner, flowmeters are used extensively within industry with small to negligible errors, the situation is very different in oilfield applications. The fullbore spinner does not, as its name suggests, cover the entire pipe cross section (Fig. 3). Typically a fullbore spinner sweeps only about 40% of the casings cross-sectional area. In addition, the blades do not have a progressive pitch (as on a ship's propellers and in gas turbines) because the requirement to collapse down to a diameter of 1 11/16 in precludes anything more complicated than a flat spinner blade. The continuous, or tubing, spinner has a progressive-pitch spinner, which is more effective at extracting energy from the well fluids (and therefore reducing the spinner threshold, which is the minimum velocity needed to start the spinner turning) (Fig.4). Unfortunately, the much reduced spinner diameter more than negates the effect of the improved blade profile, and the threshold velocity of a standard tubing spinner is about 3 times higher than that of a fullbore spinner. The Flow Scanner mini spinner (Fig.5) is used in an array of five mini-spinners recording velocities on the vertical axis of the pipe, from the bottom to the top. This arrangement is discussed in detail for the Flow Scanner horizontal and deviated well production logging system (see the "Fundamantals of production logging'' Ch.12.Flow Scanner Interpretation, Schlumberger). A change of bearing technology together with the progressive pitch of a tubing spinner delivers a spinner threshold comparable to that of a fullbore spinner. Theoretical spinner response model Consider the response of an ideal spinner flowmeter (Fig.6). The spinner speed in revolutions per second (rps) is directly proportional to the fluid velocity passing through the spinner. The slope of the response curve, measured in rps/ft/min [rps/m/min],comes directly from the spinner pitch measured in inches [centimeters]. Once friction in the bearings is included, the response becomes a little more complicated, with two
- 3. response lines, one for positive spinner readings and a second for negative spinner readings. There is now a range of low fluid velocities where the spinner does not turn because the spinner torque is smaller than the bearing friction. Close inspection of the response lines in Fig.6 shows a small curve at low spinner speeds owing to the action of static friction (stiction), viscosity effects, or both. To avoid the complications arising from nonlinear spinner response, near-zero spinner readings are discarded if they look at all suspicious. Increasing the viscosity of the fluid passing through the spinner produces some unusual results. The threshold first increases and then decreases while the spinner slope changes by about a factor of 5 or more. Figures.7 and .8 show the results of previously unpublished experimental data from the Schlumberger Gould Research Center. Changing the density of the fluid passing through the spinner (e.g., from liquid to gas) also increases the size of the low-velocity region where the spinner does not turn but should not significantly change the spinner response slope (Fig. 9). However, changing the fluid from liquid to gas causes a big change in the pseudo-Reynolds number (created using the tool velocity) and makes the creation of turbulence and vortices much easier, thus leading to the situation of (Fig. 10). NŌä£ pseudo = Žü.v .d ╬╝ where: v: tool velocity, m/s Žü=gasdensity ,kg/m 3 d=hydraulic diameter ,m ╬╝=dynamic viscosity ,Pa.s On the left of (Fig. 10) is an upward-moving tool (equivalent to a negative fluid velocity) generating vortices that travel down and confuse the spinner. Behavior like this creates asymmetric spinner slopes and thresholds. The effect can be expected to be bigger in gas wells, but it is still present in water and the lighter oils. In the middle and on the right of (Fig. 10) is the effect of the spinner cage stirring up the flow. To a first-order approximation the effects seem to be equal for flow from above and flow from below. Practical spinner response model The theoretical spinner response model described in the previous section is too complicated for everyday use. Instead, some approximations are introduced until the model of (Fig. 11) is reached. This shows a spinner in an unknown fluid as having a positive and negative spinner slope and a positive and negative spinner threshold. Because these four parameters can change with velocity, fluid density, fluid viscosity, casing diameter, and other conditions, the model needs judicious updating over an interpretation interval. Spinner interpretation ŌĆöInitial laboratory characterization The first approach (Meunier et ah, 1971) to calibrating the spinner involved laboratory characterization and the creation of interpretation nomograms (Fig. 12). The laboratory-determined spinner threshold and spinner pitch were combined with a stationary spinner reading, velocity profile viscosity model, and the pipe internal diameter to deliver a downhole flow rate. Unfortunately, the log analyst did not often have reliable downhole viscosity information, so measurements of the spinner threshold in the laboratory were rarely representative of the field, and logs versus depth could not easily be processed. Since tool speed cannot be measured downhole, as a proxy the cable velocity at surface is used. Typically cable speeds of 30, 60, and 90 ft/min [10, 20, and 30 m/min] are used, but for unstable wells additional speeds are added to average out the variations. For high-velocity gas wells, top speeds of 120-150 ft/min [40- 50 m/min] are used to better define the positive spinner intercept. In situ spinner calibration Because it is impractical to vary the downhole fluid velocity while measuring the spinner rps, the problem of spinner characterization (usually known as calibration) is approached from a different direction. Instead of varying the fluid velocity, the tool is moved up and down at different speeds
- 4. and the spinner rps plotted versus tool speed. In the absence of any kind of downhole velocity or downhole depth measurement, the tool velocity is inferred from the cable velocity as it leaves the drum at surface. Fortunately, under steady-state conditions the velocity at surface is very close to the downhole tool velocity. However, under transient conditions as the tool accelerates from rest or slows to a halt, the tool velocity cannot be expected to accurately match the cable velocity at surface. As a rule of thumb, traversing a 30-ft [10-m] interval above the top perforation and below the deepest perforation allows the tool to achieve a steady velocity before logging the zone of interest. In a zero-flow environment it is possible to determine the positive and negative spinner slopes and the positive and negative spinner thresholds (Fig. 13). But where does a zero-flow environment exist? Shutting in the well at surface does not guarantee zero flow because there may be cross flow between zones. Above the top perforation, after the well has been shut in for many hours, there should be no flow, but in the time available for production logging there may be wellbore storage (unlikely) or liquid fallback from the tubing that extends to surface. Although it is safer to assume that there is no flow below the deepest perforation (in the absence of casing plugs isolating deeper zones), the fluid density and viscosity below the deepest perforation are often unrepresentative of the fluid density and viscosity flowing in the well (the fluid for which the spinner calibration is intended). Usually the best way to identify a zero-flow region is to refer to the temperature log and identify what appears to be a geothermal gradient because geothermal gradients are incompatible with fluid movement. The spinner calibration in (Fig. 14) corresponds to a small positive velocity (down flow) but this analysis can be delivered with confidence only after inspecting the temperature log in (Fig. 15). The log in (Fig. 15) shows cable velocity, depth, spinner rps, the extent of the perforated intervals in red, spinner calibration zone in yellow, rate calibration zones in gray, pressure, temperature, and density (indicating a water-filled borehole). The steep change in temperature at about 3,900 ft shows where the cooler cross flowing water is lost into a perforation (indicated in red) and the geothermal gradient is recovered. Recirculation and the spinner In anywhere from 5┬░ to 75┬░ deviation, a low mixture velocity combined with a high slip velocity produces gravity segregation of the phases, giving rise to large high-side velocities, significant shear between light and heavy phases in the pipe, and finally a downflow of the heavy phase on the low side of the pipe (Fig. 16 ). A centered spinner tries to average the mixture of velocities passing through the swept area of the blades but generally returns a velocity that is too low if not actually negative. A poorly centralized spinner, lying closer to the low side of the pipe, returns an answer still more heavily weighted for heavy-phase recirculation. Increasing the diameter of the spinner blades reduces the size of the errors and is the simplest way of improving data quality under these conditions. While recirculation normally occurs with gas or oil bubbling up through water, the inverse situation, of water falling back through oil or gas, is often seen when a well has just been shut in. In these cases the spinner sees an erroneous uphole flow rate. Diverter flowmeters Another approach to handling the problems of recirculation is use of the diverter flowmeter. Because recirculation requires a low mixture velocity, the diverter, or petal basket (Fig. 17), flowmeter funnels the flow of the well through a 1- to 1 ┬Į in diameter tube in which a spinner velocity is recorded. If run in a 7-in completion the diverter flowmeter increases the velocity by about 20 times and therefore reduces the poorly defined threshold for recirculation effects to one twentieth. However, once the diverter is deployed the diverter flowmeter tool can only be used to make stationary measurements. This means that the accelerated flow passing through the diverter must exceed the spinner threshold (which is of the order of 15 ft/min for the small spinner used). In addition, the in situ spinner calibration of the slope and threshold must be made with the flowmeter
- 5. closed and in a different flow regime from that being used for the station measurements. Nevertheless, the diverter flowmeter is currently the least bad approach to measuring velocity in the presence of recirculation. Graphical interpretation techniques In the past, graphical techniques for interpreting spinner data were very popular. One elegant approach is still seen in production log interpretation manuals. In (Fig.18), after the various stable interval responses are plotted together with a zero-flow response, the reading line for the zero tool velocity spinner is projected right, across to the zero-flow line, from which a vertical line is dropped down to the horizontal axis, which delivers the fluid velocity. Increasing the slope of the zero-flow line by the reciprocal of the velocity profile correction factor automatically corrects for the velocity profile across the pipe. This technique assumes that the zero-flow spinner response slope and threshold are applicable to all the stable intervals above. In practice this is true only for well- behaved monophasic wells with a clean, uncontaminated zero-flow region at the bottom. This plotting technique is no longer used commercially. References Colin Whittaker ''Fundamantals of production logging'' ,2012, Schlumberger Technology Corporation. Jean-Loup Bonnet, ''MAGNETIC SUSPENSION FLOWMETER'' Verrieres-le-Buisson, France, Schlumberger Technology Corporation, Oct. 31, 1969, United States Patent Nu.3,630,078 Meunier, D., Tixier, M.P., and Bonnet, J.L.: "The Production Combination Tool, A New System for Production Monitoring," Journal of Petroleum Technology (May 1971), 603-613. Ronald A. Anderson; James J. Smolen, both of Houston, Tex. ''methods for determining velocities and flow rates of fluids flowing in well bore'', Schlumberger Technology Corporation, May 4, 1976,United States Patent Nu.3,954,006.
- 6. Figures. Figure 1. The principle of production logging Figure 2. The first result of Spinner log i. shows a typical flowmeter as it, will appear during the practice of the methods of the present invention in a. conventional multi-zoned production well; ii , iii. respectively depict typical flow measurement logs representative of what may he obtained during the practice of the present invention in a well bore such as shown in (I) with these measure ments again being presented in (iii) as they might appear when uniquely combined on a composite record for presenting a continuous flow profile of the wellbore; i ii iii i Figure.3 Fullbore spinner schematic Figure 4. Continuous, or tubing, spinner.
- 7. Figure 5. Flow Scanner minispinner. Figure 6. Spinner response with friction and stiction. Figure 7. Spinner response to increasing viscosity. Figure 9. Spinner response to decreasing density. Figure 8. Spinner response to increasing viscosity, amplified scale
- 8. Figure 10. Fluid turbulence and the fullbore spinner. Figure 12. Spinner interpretation nomogram (Meunier et at, 1971) Figure 11. Approximation to a spinner response. Figure 13. Zero-flow spinner calibration. Figure 14. Ambiguous near-zero spinner calibration.
- 9. Figure 15. Near-zero spinner calibration plot data. In the absence of any kind of downhole velocity or downhole depth measurement, the tool velocity is inferred from the cable velocity as it leaves the drum at surface. Fortunately, under steady-state conditions the velocity at surface is very close to the downhole tool velocity. However, under transient conditions as the tool accelerates from rest or slows to a halt, the tool velocity cannot be expected to accurately match the cable velocity at surface. As a rule of thumb, traversing a 30-ft [10-m] interval above the top perforation and below the deepest perforation allows the tool to achieve a steady velocity before logging the zone of interest.
- 10. Figure 16. Three-dimensional axial velocity distribution in recirculation. Figure 16. Diverter, or petal basket, flowmeter Figure 17. Graphical techniques for computing the mixture velocity.


![called "spinner-type" flowmeter tool at selected constant speeds through the fluid-filled well bore.
By successively recording the resulting rotational speeds of the flowmeter spinner at the
corresponding depth locations of the tool, a continuous flow survey or fluid-velocity log will be
obtained from which the flow rates of the well bore fluids at different depth intervals of the well
bore can be readily determined. Thus, where the well being surveyed is a production well having
two or more producing intervals, the resulting log will clearly indicate the respective velocities or
flow rates of the connate fluids which are being produced from each of the several producing
intervals. On the other hand, where the well is an injection well in which fluids are being injected
simultaneously into two or more formation intervals, the resulting flow survey will show what
portion of the injection fluid is entering each formation interval. Those skilled in the art will, of
course, appreciate that the rotational speed of the spinner in a flowmeter of this nature is simply a
linear function of the apparent or relative velocity of the well bore fluids in relation to the tool.
Thus, at low flow rates, the spinner will inherently turn at correspondingly-low speeds so long as
the well bore fluids are moving at a sufficient velocity to still turn the spinner. It is apparent,
therefore, that for a given spinner, the minimum "operating threshold" for such a flowmeter is
directly related to the amount of rotational friction which must be overcome before the spinner can
turn. Accordingly, as fully explained in the Bonnet, it was found that the unique magnetic
suspension arrangement shown there significantly reduced the static and dynamic friction affecting
the rotation of the flowmeter spinner. Although flowmeters arranged in accordance with the Bonnet
patent have met with considerable success, it has been found nevertheless that their loose-fitting
magnetically-suspended spinners become unreliable, if not ineffective, in substantially deviated or
non-vertical well bore interval. Attempts to use bushings or bearings of different types for
maintaining these spinner shafts coaxially centered in the tool have not been too successful,
however, in view of the additional friction. Moreover, fluid-borner debris such as sand grains or
other solid particles will often enter the clearance spaces around or in such bearings and impair, if
not totally disrupt, the operation of these bearings as well as other closely fitted movable elements
of the flow meter. Eventually innovations were introduced by (Anderson et al. US3,954,006) the
first results are shown in (Fig 2) . Nowadays turbine, or spinner, flowmeters are used extensively
within industry with small to negligible errors, the situation is very different in oilfield applications.
The fullbore spinner does not, as its name suggests, cover the entire pipe cross section (Fig. 3).
Typically a fullbore spinner sweeps only about 40% of the casings cross-sectional area. In addition,
the blades do not have a progressive pitch (as on a ship's propellers and in gas turbines) because the
requirement to collapse down to a diameter of 1 11/16
in precludes anything more complicated than a
flat spinner blade. The continuous, or tubing, spinner has a progressive-pitch spinner, which is more
effective at extracting energy from the well fluids (and therefore reducing the spinner threshold,
which is the minimum velocity needed to start the spinner turning) (Fig.4). Unfortunately, the much
reduced spinner diameter more than negates the effect of the improved blade profile, and the
threshold velocity of a standard tubing spinner is about 3 times higher than that of a fullbore
spinner.
The Flow Scanner mini spinner (Fig.5) is used in an array of five mini-spinners recording velocities
on the vertical axis of the pipe, from the bottom to the top. This arrangement is discussed in detail
for the Flow Scanner horizontal and deviated well production logging system (see the
"Fundamantals of production logging'' Ch.12.Flow Scanner Interpretation, Schlumberger). A change
of bearing technology together with the progressive pitch of a tubing spinner delivers a spinner
threshold comparable to that of a fullbore spinner.
Theoretical spinner response model
Consider the response of an ideal spinner flowmeter (Fig.6). The spinner speed in revolutions per
second (rps) is directly proportional to the fluid velocity passing through the spinner. The slope of
the response curve, measured in rps/ft/min [rps/m/min],comes directly from the spinner pitch
measured in inches [centimeters].
Once friction in the bearings is included, the response becomes a little more complicated, with two](https://image.slidesharecdn.com/6eb09f96-1a0a-44e5-82ec-18a4f90683cd-150309061704-conversion-gate01/85/Spinner-velosity-tool-2-320.jpg)
![response lines, one for positive spinner readings and a second for negative spinner readings. There
is now a range of low fluid velocities where the spinner does not turn because the spinner torque is
smaller than the bearing friction. Close inspection of the response lines in Fig.6 shows a small curve
at low spinner speeds owing to the action of static friction (stiction), viscosity effects, or both. To
avoid the complications arising from nonlinear spinner response, near-zero spinner readings are
discarded if they look at all suspicious. Increasing the viscosity of the fluid passing through the
spinner produces some unusual results. The threshold first increases and then decreases while the
spinner slope changes by about a factor of 5 or more. Figures.7 and .8 show the results of
previously unpublished experimental data from the Schlumberger Gould Research Center.
Changing the density of the fluid passing through the spinner (e.g., from liquid to gas) also
increases the size of the low-velocity region where the spinner does not turn but should not
significantly change the spinner response slope (Fig. 9).
However, changing the fluid from liquid to gas causes a big change in the pseudo-Reynolds number
(created using the tool velocity) and makes the creation of turbulence and vortices much easier, thus
leading to the situation of (Fig. 10).
NŌä£ pseudo
=
Žü.v .d
╬╝
where: v: tool velocity, m/s Žü=gasdensity ,kg/m
3
d=hydraulic diameter ,m ╬╝=dynamic viscosity ,Pa.s
On the left of (Fig. 10) is an upward-moving tool (equivalent to a negative fluid velocity)
generating vortices that travel down and confuse the spinner. Behavior like this creates asymmetric
spinner slopes and thresholds. The effect can be expected to be bigger in gas wells, but it is still
present in water and the lighter oils. In the middle and on the right of (Fig. 10) is the effect of the
spinner cage stirring up the flow. To a first-order approximation the effects seem to be equal for
flow from above and flow from below.
Practical spinner response model
The theoretical spinner response model described in the previous section is too complicated for
everyday use. Instead, some approximations are introduced until the model of (Fig. 11) is reached.
This shows a spinner in an unknown fluid as having a positive and negative spinner slope and a
positive and negative spinner threshold. Because these four parameters can change with velocity,
fluid density, fluid viscosity, casing diameter, and other conditions, the model needs judicious
updating over an interpretation interval.
Spinner interpretation ŌĆöInitial laboratory characterization
The first approach (Meunier et ah, 1971) to calibrating the spinner involved laboratory
characterization and the creation of interpretation nomograms (Fig. 12).
The laboratory-determined spinner threshold and spinner pitch were combined with a stationary
spinner reading, velocity profile viscosity model, and the pipe internal diameter to deliver a
downhole flow rate. Unfortunately, the log analyst did not often have reliable downhole viscosity
information, so measurements of the spinner threshold in the laboratory were rarely representative
of the field, and logs versus depth could not easily be processed. Since tool speed cannot be
measured downhole, as a proxy the cable velocity at surface is used. Typically cable speeds of 30,
60, and 90 ft/min [10, 20, and 30 m/min] are used, but for unstable wells additional speeds are
added to average out the variations. For high-velocity gas wells, top speeds of 120-150 ft/min [40-
50 m/min] are used to better define the positive spinner intercept.
In situ spinner calibration
Because it is impractical to vary the downhole fluid velocity while measuring the spinner rps, the
problem of spinner characterization (usually known as calibration) is approached from a different
direction. Instead of varying the fluid velocity, the tool is moved up and down at different speeds](https://image.slidesharecdn.com/6eb09f96-1a0a-44e5-82ec-18a4f90683cd-150309061704-conversion-gate01/85/Spinner-velosity-tool-3-320.jpg)
![and the spinner rps plotted versus tool speed.
In the absence of any kind of downhole velocity or downhole depth measurement, the tool velocity
is inferred from the cable velocity as it leaves the drum at surface. Fortunately, under steady-state
conditions the velocity at surface is very close to the downhole tool velocity. However, under
transient conditions as the tool accelerates from rest or slows to a halt, the tool velocity cannot be
expected to accurately match the cable velocity at surface. As a rule of thumb, traversing a 30-ft
[10-m] interval above the top perforation and below the deepest perforation allows the tool to
achieve a steady velocity before logging the zone of interest.
In a zero-flow environment it is possible to determine the positive and negative spinner slopes and
the positive and negative spinner thresholds (Fig. 13). But where does a zero-flow environment
exist? Shutting in the well at surface does not guarantee zero flow because there may be cross flow
between zones. Above the top perforation, after the well has been shut in for many hours, there
should be no flow, but in the time available for production logging there may be wellbore storage
(unlikely) or liquid fallback from the tubing that extends to surface. Although it is safer to assume
that there is no flow below the deepest perforation (in the absence of casing plugs isolating deeper
zones), the fluid density and viscosity below the deepest perforation are often unrepresentative of
the fluid density and viscosity flowing in the well (the fluid for which the spinner calibration is
intended). Usually the best way to identify a zero-flow region is to refer to the temperature log and
identify what appears to be a geothermal gradient because geothermal gradients are incompatible
with fluid movement. The spinner calibration in (Fig. 14) corresponds to a small positive velocity
(down flow) but this analysis can be delivered with confidence only after inspecting the temperature
log in (Fig. 15).
The log in (Fig. 15) shows cable velocity, depth, spinner rps, the extent of the perforated intervals in
red, spinner calibration zone in yellow, rate calibration zones in gray, pressure, temperature, and
density (indicating a water-filled borehole). The steep change in temperature at about 3,900 ft
shows where the cooler cross flowing water is lost into a perforation (indicated in red) and the
geothermal gradient is recovered.
Recirculation and the spinner
In anywhere from 5┬░ to 75┬░ deviation, a low mixture velocity combined with a high slip velocity
produces gravity segregation of the phases, giving rise to large high-side velocities, significant
shear between light and heavy phases in the pipe, and finally a downflow of the heavy phase on the
low side of the pipe (Fig. 16 ). A centered spinner tries to average the mixture of velocities passing
through the swept area of the blades but generally returns a velocity that is too low if not actually
negative. A poorly centralized spinner, lying closer to the low side of the pipe, returns an answer
still more heavily weighted for heavy-phase recirculation.
Increasing the diameter of the spinner blades reduces the size of the errors and is the simplest way
of improving data quality under these conditions. While recirculation normally occurs with gas or
oil bubbling up through water, the inverse situation, of water falling back through oil or gas, is often
seen when a well has just been shut in. In these cases the spinner sees an erroneous uphole flow
rate.
Diverter flowmeters
Another approach to handling the problems of recirculation is use of the diverter flowmeter.
Because recirculation requires a low mixture velocity, the diverter, or petal basket (Fig. 17),
flowmeter funnels the flow of the well through a 1- to 1 ┬Į in diameter tube in which a spinner
velocity is recorded. If run in a 7-in completion the diverter flowmeter increases the velocity by
about 20 times and therefore reduces the poorly defined threshold for recirculation effects to one
twentieth. However, once the diverter is deployed the diverter flowmeter tool can only be used to
make stationary measurements. This means that the accelerated flow passing through the diverter
must exceed the spinner threshold (which is of the order of 15 ft/min for the small spinner used). In
addition, the in situ spinner calibration of the slope and threshold must be made with the flowmeter](https://image.slidesharecdn.com/6eb09f96-1a0a-44e5-82ec-18a4f90683cd-150309061704-conversion-gate01/85/Spinner-velosity-tool-4-320.jpg)


![Figure 15. Near-zero spinner calibration plot data.
In the absence of any kind of downhole velocity or downhole depth measurement, the tool velocity is inferred from the cable velocity as it
leaves the drum at surface. Fortunately, under steady-state conditions the velocity at surface is very close to the downhole tool velocity.
However, under transient conditions as the tool accelerates from rest or slows to a halt, the tool velocity cannot be expected to accurately
match the cable velocity at surface. As a rule of thumb, traversing a 30-ft [10-m] interval above the top perforation and below the deepest
perforation allows the tool to achieve a steady velocity before logging the zone of interest.](https://image.slidesharecdn.com/6eb09f96-1a0a-44e5-82ec-18a4f90683cd-150309061704-conversion-gate01/85/Spinner-velosity-tool-9-320.jpg)
