Test for independence
1 like197 views
The document describes the steps for conducting a chi-squared test of independence using a sports team example. It tests the claim that the team's results are independent of the weather using a 1% significance level. The chi-squared calculated value of 6.956 is less than the critical value of 9.21 so the null hypothesis that the results are independent of weather is accepted.
Test for independence
- 2. Procedure Test Concerning for independence 1. Hypothesis H0: The two attributes are not associated. H1: The two attributes are associated. 2. Level of significance 留 = 1% or 5% or any given value. 3. Test of statistic Where o = observed value e = estimated value 4. Critical region 2 留,(r-1)(c-1) 5. Computation 6. Conclusion: If the calculated value of test statistic falls in the area of rejection, we reject the null hypothesis otherwise accept it. ( ) 2 2 0 e e =
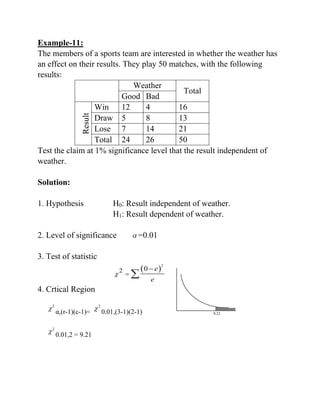
- 3. Example-11: The members of a sports team are interested in whether the weather has an effect on their results. They play 50 matches, with the following results: Weather Total Good Bad Result Win 12 4 16 Draw 5 8 13 Lose 7 14 21 Total 24 26 50 Test the claim at 1% significance level that the result independent of weather. Solution: 1. Hypothesis H0: Result independent of weather. H1: Result dependent of weather. 2. Level of significance =0.01 3. Test of statistic 4. Crtical Region 2 留,(r-1)(c-1)= 2 0.01,(3-1)(2-1) 2 0.01,2 = 9.21 ( ) 2 2 0 e e = 9.21
- 4. 5. Computation 6. Conclusion: Accept H0. And conclude that the teams result are independent of the weather. Good Bad Total Win 12 { 24 16 50 = 7.68 4 { 26 16 50 = 8.32 16 Draw 5 { 13 24 50 = 6.24 8 { 26 13 50 = 6.76 13 Loose 7 { 24 21 50 = 10.08 14 { 26 21 50 = 10.92 21 Total 24 26 50 O e 12 7.68 2.43 5 6.24 0.246 7 10.08 0.941 4 8.32 2.243 8 6.76 0.227 14 10.92 0.868 ( ) 2 o e e 2 6.956cal =