Theories of columns
- 2. ï― ï― ï― ï― ï― ï― ï― A member of a structure which carries an axial compressive load is called a strut A vertical strut which is liable for failure due to buckling or bending is called a column Failure of a STRUT occurs due to any one of the following stresses set up in the column Direct stress- Due to compressive stress (for short columns) Buckling stress or crippling stress- Due to lateral bending of columns Combined direct compressive stress and buckling stresses
- 3. ï― ï― ï― ï― ï― ï― ï― Column is initially straight and the load is applied axially Cross section of the column is uniform through out its length The column material is isotropic and homogenous Length of the column is very large compared to its lateral dimensions Direct stress (compressive stress) is very small compared to buckling stress The column will fail by buckling alone Self weight of the column is negligible
- 6. M=EI dÂēy/dxÂē ïĻ EI dÂēy/dxÂē= -Py ïĻdÂēy/dxÂē + (P/EI).y=0 ï― ï― ï― Solution of the differential equation, dÂēy/dxÂē + mÂēy=0 is y= Acosmx + Bsinmx Hence solution of DE dÂēy/dxÂē + (P/EI).y=0 is y=Acosâ(P/EI)x + Bsinâ(P/EI)x
- 7. Boundary conditions are ïĻ (i) When x=0, y=0 ïĻ (ii) When x=L, y=0 ï― Using BC (i), A=0 ï― Hence y= Bsin â(P/EI)x ï― Using BC (ii), B sinâ(P/EI)L=0 ïĻâ(P/EI)L=nÎ , where n=1,2,3...... ïĻ Simplest case is when n=1, â(P/EI)L=Î ï― P=Î ÂēEI/LÂē ï―
- 9. ï― y= Bsin â(P/EI)x; This means y is a sin function of x. Hence, we can plot various column failure modes as follows:
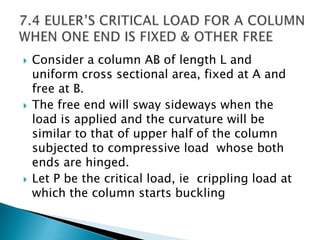
- 10. ï― ï― ï― Consider a column AB of length L and uniform cross sectional area, fixed at A and free at B. The free end will sway sideways when the load is applied and the curvature will be similar to that of upper half of the column subjected to compressive load whose both ends are hinged. Let P be the critical load, ie crippling load at which the column starts buckling
- 11. ï― Let y be the deflection at a section which is x distance away from A.
- 12. ï― Moment due to crippling load at the section, M= P(a-y) M=EI dÂēy/dxÂē ïĻ EI dÂēy/dxÂē= -P(y-a) ïĻdÂēy/dxÂē + (P/EI).y - Pa=0 ï (1) ï― Let Îŧ = (P/EI).y â Pa; ïĻ d Îŧ/dx= (P/EI).dy/dx ï―
- 13. ïĻdÂē Îŧ/dxÂē= (P/EI).dÂēy/dxÂē ïĻdÂēy/dxÂē= (EI/P). dÂē Îŧ/dxÂē Eqn (1) becomes (EI/P). dÂē Îŧ/dxÂē + Îŧ=0 ïĻ dÂē Îŧ/dxÂē + Îŧ.(P/EI)=0 ï― Îŧ= Acosâ(P/EI)x + Bsinâ(P/EI)x ïĻ (P/EI).y â Pa= Acosâ(P/EI)x + Bsinâ(P/EI)x ïĻ y=(EI/P){ Acosâ(P/EI)x + Bsinâ(P/EI)x+Pa} ï―
- 14. Boundary conditions: I) At x=0, y=0 II) At x=L, dy/dx=0 ï― Using condition (i), y=0=A + Pa; A=-Pa ï― y= (EI/P){-Pacosâ(P/EI)x + Bsinâ(P/EI)x+Pa} ï― Using condition (ii), dy/dx=0= Bâ(P/EI).cos â(P/EI).L ï― â(P/EI).L=(2n-1) Î /2, n=1,2,3.... ï― Simplest case is when n=1; â(P/EI).L= Î /2 ï― P=Î ÂēEI/4LÂē ï―
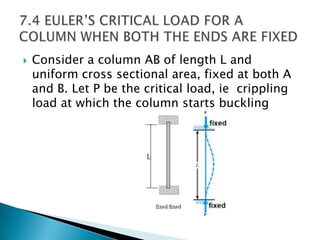
- 15. ï― Consider a column AB of length L and uniform cross sectional area, fixed at both A and B. Let P be the critical load, ie crippling load at which the column starts buckling
- 16. ï― ï― ï― Let y be the deflection at a section which is x distance away from B. Moment due to crippling load at the section, M= Mo-Py M=EI dÂēy/dxÂē
- 17. EI dÂēy/dxÂē= Mo-Py ïĻdÂēy/dxÂē + (P/EI).y â Mo/EI=0 ï (1) ï― Let Îŧ = (P/EI).y â Mo/EI; ïĻ d Îŧ/dx= (P/EI).dy/dx ïĻdÂē Îŧ/dxÂē= (P/EI).dÂēy/dxÂē ïĻdÂēy/dxÂē= (EI/P). dÂē Îŧ/dxÂē Eqn (1) becomes (EI/P). dÂē Îŧ/dxÂē + Îŧ=0 ï―
- 18. dÂē Îŧ/dxÂē + Îŧ.(P/EI)=0 ï― Îŧ= Acosâ(P/EI)x + Bsinâ(P/EI)x ïĻ(P/EI).y â Mo= Acosâ(P/EI)x + Bsinâ(P/EI)x ï― y=(EI/P){ Acosâ(P/EI)x + Bsinâ(P/EI)x+Mo} ï― Boundary conditions: a) At x=0, y=0 b) At x=L, y=0 c) At x=0, dy/dx=0 ï― Using condition (a), y=0=A + Mo; A=-Mo ï―
- 19. y= (EI/P){-Mocosâ(P/EI)x + Bsinâ(P/EI)x+Mo} ï― Using condition (c),B=0 ïĻy= (EI/P){-Mocosâ(P/EI)x + Mo) ï― Using condition (b), -Mocosâ(P/EI)L + Mo=0 ïĻcosâ(P/EI)L =1 ïĻâ(P/EI)L=2nÎ , n=1,2,3,..... ï― Simplest case is when n=1, â(P/EI)L=2Î ï― P= 4Î ÂēEI/LÂē ï―
- 20. ï― ï― ï― ï― ï― Consider a column AB of length L and uniform cross sectional area, fixed at end A and hinged at end B Let P be the critical load, ie crippling load at which the column starts buckling At the fixed end of the column, there will be a fixed end moment=Mo This Mo will tend to make the slop of deflection at fixed end zero. Inorder to balance the moment a reaction force H will be generated at B
- 21. ï― Let y be the deflection at a section which is x distance away from A.
- 22. ï― Hence the moment at section at a distance x from fixed end, Moment M=-Py + H(L-x)
- 23. M=EI dÂēy/dxÂē ïĻ EI dÂēy/dxÂē= -Py + H (L-x) ïĻdÂēy/dxÂē + (P/EI).y âH (L-x)=0 ï (1) ï― Let Îŧ = (P/EI).y âH (L-x); ïĻd Îŧ/dx=(P/EI)dy/dx + H ïĻd ÂēÎŧ/dxÂē=(P/EI)dÂēy/dxÂē ïĻ dÂēy/dxÂē= (EI/P) d ÂēÎŧ/dxÂē ï―
- 24. Hence eqn (1) becomes (EI/P) d ÂēÎŧ/dxÂē + Îŧ=0 ïĻÎŧ= A cos â(P/EI) x + B sinâ(P/EI) x ïĻ(P/EI).y âH (L-x)= A cos â(P/EI) x + B sinâ(P/EI) x ï― Boundary conditions: a) At x=0, y=0 b) At x=L, y=0 c) At x=0, dy/dx=0 ï―
- 25. ï― ï― ï― ï― Using condition (a) A = -HL Using condition (b), 0= -HL cos {â(P/EI) L} + B sin{â(P/EI) L}ï (2) Using condition (c), -H= â(P/EI)Bï (3) Using condition eqns (2) and (3),â(P/EI)Lcos{â(P/EI)L} + sinâ(P/EI)L=0
- 26. ï― Tan â(P/EI)L=â(P/EI)L Solution of tanÎļ= Îļ is Îļ= 4.5 radian ïĻâ(P/EI)L=4.5 ïĻP/EI=20.25/LÂē; 2ÏÂē=20.25 ï― P=2ÏÂēEI/LÂē ï―
- 27. ï― ï― ï― The effective length (Le) of a given column with given end conditions is the length of an equivalent column of same material and cross section hinged at its either ends and having the value of Eulerâs crippling load equal to that of the column. Crippling load for any type of end conditions is given by, P=ÏÂēEI/LeÂē The moment of inertia in the equation in Eulerâs equation is the least among Ixx and Iyy
- 29. Moment of inertia I =AkÂē, where A is the cross sectional area and k is the least radius of gyration. ï― Crippling load P=ÏÂēEI/LeÂē; ï― Substituting I=AkÂē, P= ÏÂēE (AkÂē)/LeÂē ïĻP= ÏÂēE (A)/(Le/k)Âē ïĻP/A= ÏÂēE /(Le/k)Âē ï― Le/k = Equivalent length/least radius of gyration=Slenderness ratio ï―
- 30. ï― ï― It is an empirical formula which is applicable to all columns whether short or long 1/P=1/Pc + 1/PE - P= Crippling load - Pc= Crushing load= Ïc x A - PE= Eulerâs crippling load= ÏÂēEI/ LeÂē - Ïc= Ultimate crushing stress (=330 MPa for Mild steel) - A= Cross sectional area
- 31. P=Pc.PE/(Pc + PE) OR P=Pc/{(Pc/PE) + 1} ï― P= Ïc.A/( Ïc.A.LeÂē/Î ÂēEI + 1) ïĻ P= Ïc.A/( Ïc /Î ÂēE x (Le/k)Âē + 1) ï― Ïc /Î ÂēE=Îą=Rankineâs constant ï― Hence P= Ïc.A/( Îą x (Le/k)Âē + 1) ï― Case-1:- If a column is short Pc>>Pe; Hence PâPc ï― Case-2:- If a column is long Pe>>Pc; Hence PâPE ï―
- 32. SL No Material Crushing stress in MPa Îą 1 Wrought iron 250 1/9000 2 Cast iron 550 1/1600 3 Mild steel 330 1/7500 4 Timber 50 1/750
- 33. ï― ï― ï― Consider a column AB of length L and uniform cross section fixed at A and free at B subjected to a compressive load P at an eccentricity of amount e Let a be the lateral deflection at free end Consider a section at a distance x from A where the lateral displacement is y.
- 34. ï― ï― ï― Bending moment at the section, M= P(a+e-y) EI dÂēy/dxÂē= P(a+e-y) dÂēy/dxÂē + (P/EI) x y â P (a + e)/EI=0
- 35. ï― Let Îŧ=(P/EI) x y â P (a + e)/EI Then dÂē Îŧ/dxÂē=(P/EI) x dÂē y/dxÂē ïĻ(EI/P) dÂē Îŧ/dxÂē + Îŧ= 0 ïĻÎŧ= A cos {â(P/EI)x} + B sin {â(P/EI)x} ï― ï― (P/EI).y â P(a+e)/EI= A cos {â(P/EI)x} + B sin {â(P/EI)x}
- 36. Boundary conditions are A) x=0, y=0 B) x=0,yâ=0 C) x=L, y=a ï― Condition (A) ï A= -P(a+e)/EI ï― Condition (B) ï B=0 ï―
- 37. Condition (c) ï e= (a + e) x cos{â(P/EI)L} ï― (a+e)= e x sec {â(P/EI)L} ï― Maximum stress induced at a section ï― Maximum stress at any section= crushing stress + bending stress ï― Crushing stress= P/A ï― Bending stress= M/Z; where Z is the section modulus ï― M= P (a+e)= P x e x sec {â(P/EI)L} ï― Hence Ïmax= P/A + P x e x sec {â(P/EI)L} ï―