thermodynamics lecture 1 sengel bkkkkkook
- 2. TodayŌĆÖs lectureŌĆ”. ŌĆó Composition of non reacting gases. ŌĆó Mole fraction. ŌĆó Mass fraction. ŌĆó Volume fraction. ŌĆó DeltonŌĆÖs Law of partial pressure. ŌĆó AmagatŌĆÖs Law
- 3. Composition of a gas mixture ŌĆó The composition of a gas mixture refers to the relative amounts or proportions of different gases that make up the overall mixture. This composition is often expressed using various metrics such as mole fraction, mass fraction, or volume fraction. Let's explore an example to illustrate the concept of gas mixture composition: ŌĆó Air is a common example of a gas mixture. It is composed of several gases, with nitrogen (NŌéé) and oxygen (OŌéé) being the primary components. Other gases, such as carbon dioxide (COŌéé), argon (Ar), and trace amounts of other gases, are also present.
- 4. Composition of a gas mixture
- 5. Perfect gases ŌĆó Perfect gases generally refers to an idealized model of gases that follows ideal gas laws. In the ideal gas model, gases are assumed to consist of particles (atoms or molecules) that are in constant, random motion, and the interactions between these particles are negligible. ØæĘØæĮ = ØÆÅØæ╣Øæ╗ ŌĆó It's important to note that real gases deviate from ideal behavior under certain conditions, such as at high pressures or low temperatures. ŌĆó In reality, no gas is truly perfect according to the ideal gas laws under all conditions.
- 6. Properties of an ideal gas mixture ŌĆó Each gas in the mixture behaves independently of the others. ŌĆó The total pressure of the ideal gas mixture is the sum of the partial pressures of each gas. PtotalŌĆŗ = P1+ ŌĆŗ P2 + P3 +ŌĆ”ŌĆ”..+ Pn ŌĆó The total volume of the ideal gas mixture is the sum of the volumes that each gas would occupy individually at the same temperature and pressure. ŌĆó Ideal gas mixtures assume that there are no intermolecular forces or interactions between gas molecules. ŌĆó This implies that the gases do not condense into liquids or exhibit deviations from ideal behavior.
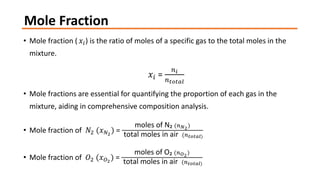
- 7. Mole Fraction ŌĆó Mole fraction (ŌĆŗ ØæźØæ¢) is the ratio of moles of a specific gas to the total moles in the mixture. ØæźØæ¢ = ØæøØæ¢ ØæøØæĪØæ£ØæĪØæÄØæÖ ŌĆó Mole fractions are essential for quantifying the proportion of each gas in the mixture, aiding in comprehensive composition analysis. ŌĆó Mole fraction of Øæü2 (ØæźØæü2 ) = moles of NŌéé (ØæøØæü2) total moles in air (ØæøØæĪØæ£ØæĪØæÄØæÖ) ŌĆó Mole fraction of Øæé2 (ØæźØæé2 ) = moles of OŌéé (ØæøØæé2) total moles in air (ØæøØæĪØæ£ØæĪØæÄØæÖ)
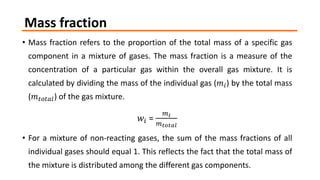
- 8. Mass fraction ŌĆó Mass fraction refers to the proportion of the total mass of a specific gas component in a mixture of gases. The mass fraction is a measure of the concentration of a particular gas within the overall gas mixture. It is calculated by dividing the mass of the individual gas (ØæÜØæ¢) by the total mass (ØæÜØæĪØæ£ØæĪØæÄØæÖ) of the gas mixture. ØæżØæ¢ = ØæÜØæ¢ ØæÜØæĪØæ£ØæĪØæÄØæÖ ŌĆó For a mixture of non-reacting gases, the sum of the mass fractions of all individual gases should equal 1. This reflects the fact that the total mass of the mixture is distributed among the different gas components.
- 9. Volume Fraction: ŌĆó The volume fraction is the ratio of the volume of each gas to the total volume of the mixture. ŌĆó In the case of air, the approximate composition by volume is often given as about 78% nitrogen, 21% oxygen, 0.04% carbon dioxide, and trace amounts of other gases.
- 10. Dalton's Law of Partial Pressures ŌĆó According to Dalton's Law, the total pressure exerted by a mixture of non-reacting gases is the sum of the partial pressures of individual gases. PtotalŌĆŗ = P1+ ŌĆŗ P2 + P3 +ŌĆ”ŌĆ”..Pn ŌĆó This law is particularly useful when dealing with gas mixtures, providing a straightforward method to calculate the contribution of each gas to the total pressure.
- 11. Example ŌĆó Suppose you have a container that contains a mixture of three non- reacting gases: nitrogen (NŌéé), oxygen (OŌéé), and carbon dioxide (COŌéé). Each gas exerts its own pressure within the container. ŌĆó According to Dalton's Law: Ptotal=PNŌééŌĆŗ+ POŌééŌĆŗ+ PCOŌééŌĆŗ PNŌééŌĆŗ= 400 kPa; POŌééŌĆŗ = 300 kPa; PCOŌééŌĆŗŌĆŗ= 300 kPa Ptotal= 400 kPa + 300 kPa + 300 kPa = 1000 kPa
- 12. Problem:1 ŌĆó You have a container containing a mixture of two non-reacting gases: oxygen (OŌéé) and nitrogen (NŌéé). The total pressure in the container is 800 kPa, and the mass fraction of oxygen in the mixture is 0.4. Determine: ŌĆó The mass fraction of nitrogen in the mixture? ŌĆó The mass of oxygen in the container if the total mass of the mixture is 5 kg?
- 13. Solution ŌĆó Mass Fraction of Nitrogen (ØæżØæü2 ): ØæżØæü2 + ØæżØæé2 = 1 ØæżØæü2 ŌĆŗ= 1ŌłÆ0.4 = 0.6 ŌĆó Mass of Oxygen in the Container: ØæżØæé2 = ØæÜØæé2 ØæÜØæĪØæ£ØæĪØæÄØæÖ ØæÜØæé2 = 0.4├Ś5
- 14. Problem:2 ŌĆó You have a container containing a mixture of three non-reacting gases: hydrogen (HŌéé), carbon dioxide (COŌéé), and nitrogen (NŌéé). The partial pressures of these gases are as follows: PHŌééŌĆŗ= 150kPa; PCOŌéé= 200 kPa; PNŌéé= 100 kPa. The molar masses of the gases are: MHŌéé = 2 g/mol; MCOŌéé= 44 g/mol; MNŌéé = 28 g/mol. if the container volume is 5 L and the temperature is 300 K. Calculate: ŌĆó The mole fraction of each gas in the mixture. ŌĆó The mass fraction of each gas in the mixture.
- 15. Solution ØæźØÉ╗2 = ØæøØÉ╗2 ØæøØæĪØæ£ØæĪØæÄØæÖ ŌĆó Use the ideal gas law to find the moles of each gas (niŌĆŗ): ØæøØæ¢ŌĆŗ= ØæāØæ¢Øæē ØæģØæć ŌĆó Where R=8.314 J/(mol K) Is the ideal gas constant. ØæżØÉ╗2 = ØæÜØÉ╗2 ØæÜØæĪØæ£ØæĪØæÄØæÖ ØæÜØæ¢ = ØæøØæ¢ ├Ś ØæĆØæ¢
- 16. Amagat's law ŌĆó The volume occupied by a mixture of non-reacting gases is equal to the sum of the volumes of the individual gases at the same temperature and pressure as the mixture. ØæēØæĪØæ£ØæĪØæÄØæÖ ŌĆŗ = Øæ¢=1 Øæø ØæēØæ¢ ŌĆó This law is applicable on the non-reacting gases where there are no significant intermolecular forces between the gas molecules. ŌĆó It's essential to note that Amagat's law is most accurate under conditions where gases behave ideally, and it becomes less precise under extreme conditions (high pressure, low temperature) or when gases have significant interactions with each other.
- 17. Example ŌĆó Let's say you have a container of nitrogen gas (NŌéé) and a container of argon gas (Ar). According to Amagat's law, the total volume of the gas mixture, assuming ideal behavior, would be the sum of the volumes of nitrogen and argon individually. ØæĮØÆĢØÆÉØÆĢØÆéØÆŹ = ØæĮØæĄØ¤É + ØæĮØæ©ØÆō ŌĆó It's essential to note that Amagat's law is most accurate under conditions where gases behave ideally, and it becomes less precise under extreme conditions (high pressure, low temperature) or when gases have significant interactions with each other.
- 18. Adiabatic mixture of perfect gases ŌĆó The adiabatic mixture of perfect gases refers to a scenario where a mixture of perfect gases undergoes an adiabatic process. An adiabatic process is one in which there is no heat exchange with the surroundings. ØæāØæēØøŠ = ØÉČØæ£ØæøØæĀØæĪØæÄØæøØæĪ ŌĆó ØøŠ = ØÉČØæē ØÉČØæā represents the ratio of the specific heat at constant pressure to the specific heat at constant volume. ŌĆó For a mixture of perfect gases, each gas component in the mixture follows its own adiabatic process, and the overall behavior is determined by the combined effect of these individual processes.
- 19. ŌĆó The adiabatic process equations are commonly used to analyze the changes in temperature, pressure, and volume of a gas mixture when no heat is transferred to or from the system. ŌĆó This can be relevant in various applications, such as in the analysis of processes in internal combustion engines, compressors, and other thermodynamic systems where heat exchange with the surroundings is minimal. ŌĆó It's worth noting that the adiabatic process assumes that the system is insulated from its surroundings, and any changes in internal energy are solely due to work done on or by the system.
- 20. Adiabatic Compression in a Compressor: ŌĆó Initial State: ŌĆó Air enters the compressor at a certain temperature, pressure, and volume. ŌĆó The compressor compresses the air adiabatically, meaning that no heat is exchanged with the surroundings. ŌĆó Compression: ŌĆó As the air is compressed, its volume decreases, and its pressure and temperature increase. ŌĆó The adiabatic compression process can be described by the adiabatic equation ØæāØæēØøŠ = ØÉČØæ£ØæøØæĀØæĪØæÄØæøØæĪ, where P is the pressure, V is the volume, and ╬│ is the ratio of specific heats. ŌĆó Final State: ŌĆó The air exits the compressor at a higher pressure, temperature, and potentially a different volume.