Translating standard form into vertex form if a=1
- 1. Translating Quadratic Function from Standard Form into Vertex Form if ØÆé = ؤŠMathematics 9
- 2. Standard From and Vertex Form of Quadratic Function ’é┤Standard Form Øæ” = ØæÄØæź2 + ØæÅØæź + ØæÉ ’é┤Vertex Form Øæ” = ØæÄ Øæź ŌłÆ ŌäÄ 2 + Øæś
- 3. ’é┤To translate quadratic function from standard form to vertex form, you need to know the following: 1. Completing the Square Method 2. Factoring
- 4. Standard to Vertex Form ’é┤ Steps for translating quadratic function from standard to vertex form if ØÆé = ؤŠStep 1: Since the equation is in the standard form Øæ” = ØæÄØæź2 + ØæÅØæź + ØæÉ, and we want to convert it into the form of Øæ” = ØæÄ Øæź ŌłÆ ŌäÄ 2 + Øæś, then the first thing that we need to do is transpose ØæÉ to the other side of equal sign. Example 1: Øæ” = Øæź2 + 4Øæź + 7 Øæ” ŌłÆ 7 = Øæź2 + 4Øæź
- 5. Standard to Vertex Form ’é┤ Steps for translating quadratic function from standard to vertex form ØÆé = ؤŠStep 2: Perform completing the square. The goal of this method is to make a perfect square trinomial and it will only happen if the coefficient of Øæź2 or the value of a is equal to 1. Since the coefficient of Øæź2 in the example below is equal to 1, then we can immediately perform completing the square. For this situation, we will going to add ØÆā Ø¤É Ø¤É to the both sides of equation. Øæ” ŌłÆ 7 + ØÆā Ø¤É Ø¤É = Øæź2 + 4Øæź + ØÆā Ø¤É Ø¤É ØÆÜ ŌłÆ ؤĢ + Ø¤Æ = ØÆÖ Ø¤É + ؤÆØÆÖ + Ø¤Æ Since ØæÅ = 4, then Ø¤Æ Ø¤É Ø¤É = 4
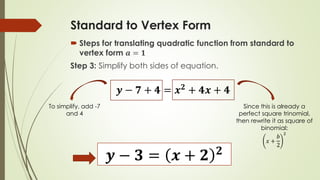
- 6. Standard to Vertex Form ’é┤ Steps for translating quadratic function from standard to vertex form ØÆé = ؤŠStep 3: Simplify both sides of equation. ØÆÜ ŌłÆ ؤĢ + Ø¤Æ = ØÆÖ Ø¤É + ؤÆØÆÖ + Ø¤Æ To simplify, add -7 and 4 Since this is already a perfect square trinomial, then rewrite it as square of binomial: Øæź + ØæÅ 2 2 ØÆÜ ŌłÆ ؤæ = ØÆÖ + Ø¤É Ø¤É
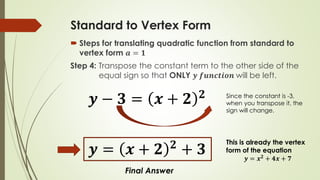
- 7. Standard to Vertex Form ’é┤ Steps for translating quadratic function from standard to vertex form ØÆé = ؤŠStep 4: Transpose the constant term to the other side of the equal sign so that ONLY ØÆÜ ØÆćØÆ¢ØÆÅØÆäØÆĢØÆŖØÆÉØÆÅ will be left. ØÆÜ ŌłÆ ؤæ = ØÆÖ + Ø¤É Ø¤É Since the constant is -3, when you transpose it, the sign will change. ØÆÜ = ØÆÖ + Ø¤É Ø¤É + ؤæ This is already the vertex form of the equation ØÆÜ = ØÆÖ Ø¤É + ؤÆØÆÖ + ؤĢ Final Answer
- 8. More Examples Translating Standard Form into Vertex Form when ØæÄ = 1
- 9. Example 1 ØÆÜ = ØÆÖ Ø¤É + ؤöØÆÖ + ؤÅØ¤Ä Quadratic in Standard Form ØÆÜ ŌłÆ ؤÅØ¤Ä = ØÆÖ Ø¤É + ؤöØÆÖ Transpose 10 to the left ØÆÜ ŌłÆ ؤÅØ¤Ä + _____ = ØÆÖ Ø¤É + ؤöØÆÖ + _____ Completing the square: Since ØæÅ = 6, the ØæÅ 2 2 = 6 2 2 = 9 ØÆÜ ŌłÆ ؤÅØ¤Ä + ؤŚ = ØÆÖ Ø¤É + ؤöØÆÖ + ؤŚ Add 9 to both sides of equation ØÆÜ ŌłÆ ؤŠ= ØÆÖ + ؤæ Ø¤É Transpose -1 so that only y will be left. ØÆÜ = ØÆÖ + ؤæ Ø¤É + ؤŠFinal Answer Find the vertex form of the function Øæ” = Øæź2 + 6Øæź + 10.
- 10. Example 2 ØÆÜ = ØÆÖ Ø¤É + ؤÉØÆÖ ŌłÆ ؤæ Quadratic in Standard Form ØÆÜ + ؤæ = ØÆÖ Ø¤É + ؤÉØÆÖ Transpose -3 to the left ØÆÜ + ؤæ + _____ = ØÆÖ Ø¤É + ؤÉØÆÖ + _____ Completing the square: Since ØæÅ = 2, the ØæÅ 2 2 = 2 2 2 = 1 ØÆÜ + ؤæ + ؤŠ= ØÆÖ Ø¤É + ؤÉØÆÖ + ؤŠAdd 1 to both sides of equation ØÆÜ + Ø¤Æ = ØÆÖ + Ø¤Å Ø¤É Transpose 4 so that only y will be left. ØÆÜ = ØÆÖ + Ø¤Å Ø¤É ŌłÆ Ø¤Æ Final Answer Find the vertex form of the function Øæ” = Øæź2 + 2Øæź ŌłÆ 3.