Translating vertex form into standard form when a is not equal to 1
- 1. Translating Quadratic Function from Vertex Form into Standard Form if ØÆé ŌēĀ ؤŠMathematics 9
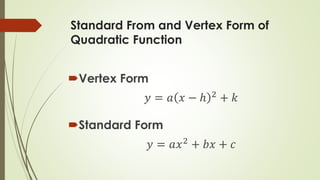
- 2. Standard From and Vertex Form of Quadratic Function ’é┤Vertex Form Øæ” = ØæÄ Øæź ŌłÆ ŌäÄ 2 + Øæś ’é┤Standard Form Øæ” = ØæÄØæź2 + ØæÅØæź + ØæÉ
- 3. ’é┤To translate quadratic function from vertex form back to standard form, all we need to do is to simplify and you should know the following: 1. FOIL Method 2. Distributive Property
- 4. Vertex Form into Standard Form ’é┤ Steps for translating quadratic function from vertex back to standard form if ØÆé ŌēĀ ؤŠStep 1: Since the vertex form of quadratic equation is written in the form of Øæ” = ØæÄ Øæź ŌłÆ ŌäÄ 2 + Øæś, and we have a square of binomial which is Øæź ŌłÆ ŌäÄ 2, then we can replace it by (Øæź ŌłÆ ŌäÄ)(Øæź ŌłÆ ŌäÄ). See the example below. Example 1: Øæ” = 2 Øæź ŌłÆ 3 2 + 6 Øæ” = 2 Øæź ŌłÆ 3 Øæź ŌłÆ 3 + 6
- 5. Vertex Form into Standard Form ’é┤ Steps for translating quadratic function from vertex back to standard form if ØÆé ŌēĀ ؤŠStep 2: Now, we have two binomials and the operation is multiplication, and to get the product of two binomials, we need to use the FOIL method. Example 1: Øæ” = 2(Øæź ŌłÆ 3)(Øæź ŌłÆ 3) + 6 ØæŁ ØæČ Øæ░ Øæ│ F = ØÆÖ ØÆÖ = ØÆÖ Ø¤É O = ØÆÖ ŌłÆؤæ = ŌłÆؤæØÆÖ I = ŌłÆؤæ ØÆÖ = ŌłÆؤæØÆÖ L = ŌłÆؤæ ŌłÆؤæ = ؤŚ Øæ” = 2(Øæź2 ŌłÆ 3Øæź ŌłÆ 3Øæź + 9) + 6 Do not forget to put parenthesis because the product of the two binomials are still multiplied by 2.
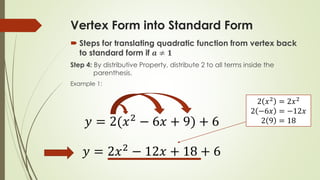
- 6. Vertex Form into Standard Form ’é┤ Steps for translating quadratic function from vertex back to standard form if ØÆé ŌēĀ ؤŠStep 3: Simplify the terms inside the parenthesis by combining like terms Example 1: Øæ” = 2(Øæź2 ŌłÆ 3Øæź ŌłÆ 3Øæź + 9) + 6 ŌłÆ6Øæź Øæ” = 2(Øæź2 ŌłÆ 6Øæź + 9) + 6
- 7. Vertex Form into Standard Form ’é┤ Steps for translating quadratic function from vertex back to standard form if ØÆé ŌēĀ ؤŠStep 4: By distributive Property, distribute 2 to all terms inside the parenthesis. Example 1: Øæ” = 2(Øæź2 ŌłÆ 6Øæź + 9) + 6 Øæ” = 2Øæź2 ŌłÆ 12Øæź + 18 + 6 2 Øæź2 = 2Øæź2 2 ŌłÆ6Øæź = ŌłÆ12Øæź 2 9 = 18
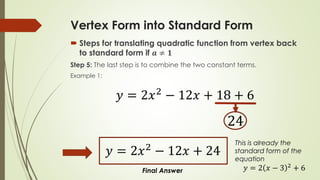
- 8. Vertex Form into Standard Form ’é┤ Steps for translating quadratic function from vertex back to standard form if ØÆé ŌēĀ ؤŠStep 5: The last step is to combine the two constant terms. Example 1: Øæ” = 2Øæź2 ŌłÆ 12Øæź + 18 + 6 24 Øæ” = 2Øæź2 ŌłÆ 12Øæź + 24 Final Answer This is already the standard form of the equation Øæ” = 2 Øæź ŌłÆ 3 2 + 6
- 9. More Examples Translating Vertex into Standard Form when ØæÄ ŌēĀ 1
- 10. Example 1 ØÆÜ = ŌłÆ ØÆÖ ŌłÆ ؤ¢ Ø¤É + Ø¤É Quadratic in Vertex Form ØÆÜ = ŌłÆ ØÆÖ ŌłÆ ؤ¢ ØÆÖ ŌłÆ ؤ¢ + Ø¤É Square of binomial ØæÄ + ØæÅ 2 = (ØæÄ + ØæÅ(ØæÄ + ØæÅ) ØÆÜ = ŌłÆ ØÆÖ Ø¤É ŌłÆ ؤ¢ØÆÖ ŌłÆ ؤ¢ØÆÖ + ؤöØ¤Æ + Ø¤É FOIL Method ØÆÜ = ŌłÆ(ØÆÖ Ø¤É ŌłÆ ؤÅؤöØÆÖ + ؤöؤÆ) + Ø¤É Simplify the result of FOIL ØÆÜ = ŌłÆØÆÖ Ø¤É + ؤÅؤöØÆÖ ŌłÆ ؤöØ¤Æ + Ø¤É Distributive Property ØÆÜ = ŌłÆØÆÖ Ø¤É + ؤÅؤöØÆÖ ŌłÆ ؤöØ¤É Combine like terms ØÆÜ = ŌłÆØÆÖ Ø¤É + ؤÅؤöØÆÖ ŌłÆ ؤöØ¤É Final Answer Find the standard form of the function ØÆÜ = ŌłÆ ØÆÖ ŌłÆ ؤ¢ Ø¤É + ؤÉ.
- 11. Example 2 ØÆÜ = ؤæ ØÆÖ + Ø¤É Ø¤É ŌłÆ ؤō Quadratic in Vertex Form ØÆÜ = ؤæ ØÆÖ + Ø¤É ØÆÖ + Ø¤É ŌłÆ ؤō Square of binomial ØæÄ + ØæÅ 2 = (ØæÄ + ØæÅ(ØæÄ + ØæÅ) ØÆÜ = ؤæ ØÆÖ Ø¤É + ؤÉØÆÖ + ؤÉØÆÖ + Ø¤Æ ŌłÆ ؤō FOIL Method ØÆÜ = ؤæ(ØÆÖ Ø¤É + ؤÆØÆÖ + ؤÆ) ŌłÆ ؤō Simplify the result of FOIL ØÆÜ = ؤæØÆÖ Ø¤É + ؤÅؤÉØÆÖ + ؤÅØ¤É ŌłÆ ؤō Distributive Property ØÆÜ = ؤæØÆÖ Ø¤É + ؤÅؤÉØÆÖ + ؤĢ Combine like terms ØÆÜ = ؤæØÆÖ Ø¤É + ؤÅؤÉØÆÖ + ؤĢ Final Answer Find the standard form of the function ØÆÜ = ؤæ ØÆÖ + Ø¤É Ø¤É ŌłÆ ؤō.