Trigonometry maths methodology
- 2. AIMS AND OBJECTIVES ŌĆó The aim of the lesson: ŌĆó To teach learners how to simplify trigonometric expressions using the CAST diagram. ŌĆó To teach learners how to simplify trigonometric expressions using identities and Co-ratios
- 3. PRIOR KNOWLEDGE ŌĆó In grade you learnt about the theorem of Pythagoras, the trigonometric ratios and the names of the sides on a right angle. ŌĆó Recap Questions 1. State the theorem of Pythagoras 2. Define sin x, cos x and tan x in terms of their sides 3. Name the sides of the triangle.
- 4. PRIOR KNOWLEDGE Solve for x using the theorem of Pythagoras. Revision Theorem of Pythagoras: Øæź2 + Øæ”2 = Øæ¤2 , where c is the hypotenuse. ØæåØæ¢ØæøØæź = Øæ£ØæØØæØØæ£ØæĀØæ¢ØæĪØæÆ ŌäÄØæ”ØæØØæ£ØæĪØæÆØæøØæóØæĀØæÆ = Øæ” Øæ¤
- 5. PRIOR KNOWLEDGE ŌĆó ØÉČØæ£ØæĀØæź = ØæÄØææØæŚØæÄØæÉØæÆØæøØæĪ ŌäÄØæ”ØæØØæ£ØæĪØæÆØæøØæóØæĀØæÆ = Øæź Øæ¤ ŌĆó ØæĪØæÄØæøØæź = Øæ£ØæØØæØØæ£ØæĀØæ¢ØæĪØæÆ ØæÄØææØæŚØæÄØæÉØæÆØæøØæĪ = Øæ” Øæź
- 6. THE CAST DIAGRAM ŌĆó This CAST diagram helps us to identify whether our trigonometric ratios are positive or negative.
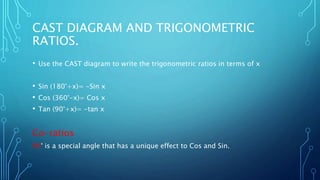
- 7. CAST DIAGRAM AND TRIGONOMETRIC RATIOS. ŌĆó Use the CAST diagram to write the trigonometric ratios in terms of x ŌĆó Sin (180┬░+x)= -Sin x ŌĆó Cos (360┬░-x)= Cos x ŌĆó Tan (90┬░+x)= -tan x Co-ratios 90┬░ is a special angle that has a unique effect to Cos and Sin.
- 8. CO-RATIOS ŌĆó ØæåØæ¢Øæø (90┬░ŌłÆx) =Cos x ŌĆó ØÉČØæ£ØæĀ 90┬░ + Øæź = ŌłÆ sin Øæź This effect is applicable with the angle 270┬░ as well. What will be the answer: ØæåØæ¢Øæø (90┬░+x) = ?? ØæåØæ¢Øæø (270┬░ ŌłÆ Øæź)= ??
- 9. TRIGONOMETRIC IDENTITIES ØæĪØæÄØæøØæź = ØæĀØæ¢ØæøØæź ØæÉØæ£ØæĀØæź ØæĀØæ¢Øæø2 Øæź + ØæÉØæ£ØæĀ2 Øæź = 1 Therefore, ØæĀØæ¢Øæø2 Øæź= 1- ØæÉØæ£ØæĀ2 Øæź And ØæÉØæ£ØæĀ2 Øæź= 1- ØæĀØæ¢Øæø2 Øæź
- 11. CLASSWORK 1. In which Quadrants are the following positive: ŌĆó Sin ╬Ė ŌĆó Cos ╬Ė ŌĆó Tan ╬Ė ŌĆó 2. Express the following in terms of ╬Ė: ŌĆó Sin (90┬░+ ╬Ė) ŌĆó Tan (180┬░- ╬Ė) ŌĆó Cos (360┬░- ╬Ė)
- 12. CLASSWORK ŌĆó 3. Simplify the expressions ŌĆó ØÆöØÆŖØÆŠؤŚØ¤Ä┬░ ŌłÆ x cos 360┬░ ŌłÆ x tan 180┬░ ŌłÆ x ├Ę cos(180┬░ ŌłÆ x) The end.