UNIT 4 - Proportion and modular structures (Book unit 9)
- 1. PROPORTION & MODULAR STRUCTURES UNIT 4 (BOOK UNIT 9)
- 2. ’üĄ Proportions are built from ratios. A "ratio" is just a comparison between two different things. ’üĄ We can express the ratio with different notations: ’üĄ in words: 5 to 10 There are two other notations for this "15 to 20" ratio: ’üĄ odds notation: 5 : 10 ’üĄ fractional notation: 5/10 ’üĄ With cociente 0.5 ’üĄ With percentage 50% ’üĄ If figures have the same shape, we can say that there is a constant ratio. ’üĄ Proportions we will see on this Unit are Equality, Symmetry and Similarity. Ratios
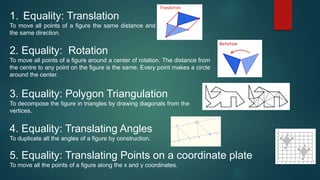
- 3. Equality ’üĄ When two figures have the same size, the same shape and the same orientation they are Equal. ’üĄ Equal figures are also Congruent. Congruency happens when you can place one figure over the other and they fit exactly. ’üĄ Two equal figures are always on a 1:1 ratio ’üĄ We can make equal figures with the next methods.
- 4. 1. Equality: Translation To move all points of a figure the same distance and the same direction. 2. Equality: Rotation To move all points of a figure around a center of rotation. The distance from the centre to any point on the figure is the same. Every point makes a circle around the center. 3. Equality: Polygon Triangulation To decompose the figure in triangles by drawing diagonals from the vertices. 4. Equality: Translating Angles To duplicate all the angles of a figure by construction. 5. Equality: Translating Points on a coordinate plate To move all the points of a figure along the x and y coordinates.
- 5. Symmetry ’üĄ When two figures have the same size and the same shape but different orientation they are Symmetric. ’üĄ Symmetric figures are also Congruent. Congruency happens when you can place one figure over the other and they fit exactly. ’üĄ We can make symmetric figures with the next methods.
- 6. 1. Symmetry : Reflection Also known as mirror or axial symmetry. To move all points of a figure the same distance from an axis (also called mirror line) but in the opposite direction. One half of the figure Will be the reflection of the other. 2. Symmetry : Point Symmetry To move all points of a figure the same distance from a central point but in the opposite direction. The figure will looks the same Upside Down. 3. Symmetry : Bilateral Symmetry As in the reflection symmetry, but when we have volumen. The axis is in this case a plane and the 2D shape will be here a 3D shape. All points of our volumen will be at the same distance from a plane-axis but in the opposite direction.
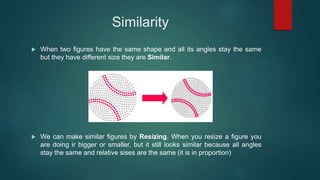
- 7. Similarity ’üĄ When two figures have the same shape and all its angles stay the same but they have different size they are Similar. ’üĄ We can make similar figures by Resizing. When you resize a figure you are doing ir bigger or smaller, but it still looks similar because all angles stay the same and relative sises are the same (it is in proportion)
- 8. 1. Similarity : Resize from a vertex 2. Similarity : Resize from a outer point