FR8695_IndProj
0 likes294 views
The document compares the fatigue life of solder balls in completely filled and incompletely filled flip-chip packages. It finds that a fully filled model has a much higher fatigue cycle sustaining capacity than an incompletely filled model, which shows the possibility of earlier failure. Finite element analysis is used to calculate the fatigue life of both models based on strain values and the Coffin-Manson equation. The results show that the completely filled model has a higher fatigue life than the incompletely filled model.
1 of 12
Download to read offline




Recommended
Group8 presentation



Group8 presentationNilesh Raj
Ėý
1) The document presents the process and mechanical design of a packed bed extractor to extract 96% of benzene from a feed mixture of 78% 1-hexene and 22% benzene using tetra-methylene sulphone as the solvent.
2) Key steps in the process design include determining the equilibrium curve, mass flow rates, minimum solvent rate, dispersed and continuous phase properties, flooding velocity, column diameter, mass transfer coefficient, number of transfer units, and column height.
3) Key steps in the mechanical design include determining the shell and head thickness, stresses from various loads, internal packing support, and distributor specifications. Lessing rings are identified as the optimum packing material.Tutorial 9



Tutorial 9gauraviist
Ėý
This document provides a summary of topics covered in a Mechanics of Solids tutorial, including pressure vessels, combined loadings, stress and deflection analysis problems. Problems 1-3 cover pressure vessels and determining stresses in pipes under various internal pressures. Problems 4-7 analyze combined loadings on beams, bands and links, determining stresses, deflections, and using moment area theorems.Stresses in bolts & nuts



Stresses in bolts & nutsshivanisingh204784
Ėý
The document discusses stresses in bolts and nuts when they are tightened. It provides two examples: (1) A steel bolt connecting a copper tube that is tightened by 1/4 turn, (2) A steel bolt and tubular piece tightened by 1/4 turn and then subjected to an external tensile load. It calculates the stresses induced in the bolt and tube for each example, accounting for both the tightening and any external loads based on principles of static equilibrium and compatibility. The final stresses are provided for the bolt and tube in each example.10 main body of thesis chap 6



10 main body of thesis chap 6Miftakhu Zaim
Ėý
This section calculates the hoop stress on a winch drum under pulling and braking loads using Hampe's solution method. It finds that:
1) The maximum hoop stress occurs at the 0.8 position from the near end of the drum for both pulling (348.4 MPa) and braking (370.7 MPa) loads.
2) The calculated maximum stresses are 19% and 42% lower than design code values for pulling and braking loads respectively.
3) It is proposed to reinforce the drum thickness only in the area around the 0.8 position to resist the higher stresses, rather than using a uniform thickness.CIVE 801 - Course Project Report (Teal)



CIVE 801 - Course Project Report (Teal)Cyle Teal
Ėý
The document describes a course project analyzing the dynamics of a 375-foot concrete bridge modeled as a single-degree-of-freedom system. Key aspects included:
1) Designing the bridge frame and multi-girder cross section, then calculating the total mass as 108.2 kip-sec^2/ft.
2) Computing the natural frequency as 15.4 rad/sec and natural period as 0.41 sec based on the system stiffness of 25,800 kip/ft and mass.
3) Finding the undamped free vibration response to two sets of initial conditions involved sinusoidal functions of the natural frequency and time.
4) Determining the damped freeSide_Impact_Van_MDB



Side_Impact_Van_MDBSiddhesh Ozarkar
Ėý
The document discusses a simulation of an FMVSS 214 side impact test on a van.
The objectives were to analyze the van's performance, calculate injury metrics like HIC and CSI, compare to FMVSS 214 standards, and explore structural reinforcements.
The initial base run showed high Y-displacement of 772mm and high chest deflection. Reinforcements like added beam members reduced displacement to 600mm. However, injury values like CSI of 318 and pelvic acceleration of 589g still exceeded standards. Further improvements like side airbags and strengthened A/B pillars were proposed.Roof Crush



Roof CrushSiddhesh Ozarkar
Ėý
This document summarizes the results of a simulation analyzing the structural performance of a truck undergoing roof crush. The simulation shows the roof experiencing over 142mm of maximum deflection on impact, with outward bulging of the external door panels. Using high-strength steel for structural members in a subsequent simulation reduced the deflection. The total energy during impact initially spikes then gradually increases, and the simulation ends with displacement of the A-B pillars and roof cross members, and buckling observed.Zero Turn Radius Presentation - Team Panache



Zero Turn Radius Presentation - Team PanacheSiddhesh Ozarkar
Ėý
The document describes the design of a zero-turn radius system for a John Deere 5065E tractor. The system uses hydraulic tie rods attached to a double-acting cylinder to enable the rear wheels to rotate independently, allowing the tractor to turn within its own width. Calculations are shown for sizing the steering and tie rod cylinders and rods. The design also incorporates a hydrostatic transmission system to provide infinitely variable speed control for the rear wheels to facilitate zero-turn maneuvers. Performance simulations and a cost analysis are presented to evaluate the technical feasibility and cost-effectiveness of the zero-turn design.Reliability of Flip-Chip Packages



Reliability of Flip-Chip PackagesPratik Saxena
Ėý
Developed 2D CAD model of flip chip package using Hypermesh
Fatigue analysis using ABAQUS CAE was done, predicting the life of solder balls in completely-filled clean and non-clean flip-chip packages
Assessed and identified the fatigue life of a completely clean model is higher than that of incomplete non-clean model
G012235155



G012235155IOSR Journals
Ėý
This document summarizes a numerical investigation and fatigue life estimation of a conventional diesel engine piston. A finite element analysis was conducted using ANSYS to determine thermal and stress distributions in the piston under operating loads. The piston geometry was meshed and assigned material properties of an aluminum alloy. Temperature loads from 250°C to 650°C and pressure loads from 1 to 60 bar were applied. The results from the finite element analysis were then used to estimate the fatigue life of the piston according to ASME standards. The analysis determined a fatigue life of 868,587 cycles with a peak stress of 69.8 MPa.A Study on Stochastic Thermal Characterization of Electronic Packages



A Study on Stochastic Thermal Characterization of Electronic PackagesIJERA Editor
Ėý
Insofar as the electronics can be found now in several applications of multiple domains, we have tried to
highlight in this study that, those systems must be based on unquestionable reliability and meet the needs of the
external environment. Starting from the unit "°c / w" concerning the thermal resistance from the gap between
junction temperature and a reference temperature, we have tried to compare the thermal performance of
electronic packages taking into consideration the thermal management. Our approach is based on the Monte
Carlo simulation and the stochastic characterization of the QFN. From the norm of normalization, we have
obtained standardized data sheets allowing accurate comparisons of the thermal performance of electronic
packages as produced by different manufacturers. Our numerical model through simulation, prototyping
concerning the design involves the JEDEC recommendations, which we consider a very interesting alternative.
Through the deterministic analysis, we conducted an analysis from the Matlab program parameters, which
control the Ansys software, the results were processed by statistical techniques to evaluate the times of the
thermal resistance of the QFN. That is why we must consider the electronic package (encapsulating the
integrated circuit), through the printed circuit board (PCB) to ensure the junction temperature maintenance and
avoid the dissipation of the heat. Also our process was based on the union of the finite element method to the
Monte Carlo simulation and stochastic characterization of the QFN.
Keywords: Electronic package; Finite element method; printed Paper id 27201447



Paper id 27201447IJRAT
Ėý
This document summarizes a study on predicting creep life for gas turbine discs. Creep is an important design consideration for gas turbine components operating at high temperatures. The study models a typical gas turbine disc and analyzes temperature distribution, stresses, and creep strains using finite element analysis. An Inconel 718 disc is modeled and analyzed to determine creep life and emphasize creep considerations in gas turbine disc design. Thermal, stress, and creep analyses are performed to evaluate creep life and strains at different stress levels over time.IJCER (www.ijceronline.com) International Journal of computational Engineerin...



IJCER (www.ijceronline.com) International Journal of computational Engineerin...ijceronline
Ėý
The document discusses the modeling and analysis of stresses in a steam turbine casing under transient operating conditions. It presents the finite element modeling process for the casing, including assumptions made and software used. The modeling considers thermal loading during startup and shutdown cycles. Heat transfer and thermal analyses are conducted to determine temperature distributions and thermal stresses within the casing. High stresses are found at locations like corners, welds and nozzle fits, which can lead to damage like cracking over the turbine's lifetime. The analysis aims to better understand casing behavior under transient conditions and improve design for increased reliability and life.FEA Project-Pressure Vessel & Heat Loss Analysis



FEA Project-Pressure Vessel & Heat Loss AnalysisMostafa Fakharifar
Ėý
This document summarizes a finite element analysis of a pressure vessel subjected to internal pressure and temperature gradients. A one-eighth model was analyzed in ABAQUS for three load cases: internal pressure only, internal temperature only, and combined internal pressure and temperature. Mesh convergence studies showed the medium-fine mesh provided accurate results efficiently. For internal pressure only, the maximum von-Mises stress was 504.8 MPa. For internal temperature only, the maximum stress was lower at 123.1 MPa. The combined case produced the highest stresses and strains, with a maximum von-Mises stress of 555.1 MPa occurring at the top center and fillet.Thermal stresses



Thermal stressesrawaabdullah
Ėý
Thermal stresses occur when a material experiences a change in temperature that causes it to expand or contract. This document discusses thermal stresses, providing examples of how temperature gradients and differences in thermal expansion coefficients between materials can lead to stresses. It also outlines the background and methods for experimentally measuring thermal stress fields in a composite-metal bonded joint, including using infrared cameras to measure temperature fields and grid analysis to measure displacement fields. The results provide insight into how temperature changes affect the stress distribution in the bonded joint.IRJET-Finite Element Analysis of Glazed Surface



IRJET-Finite Element Analysis of Glazed SurfaceIRJET Journal
Ėý
Raghunath .D. Deshpande, Gayatri Chiniwal, Laxmi Golsangi, "Finite Element Analysis of Glazed Surface", International Research Journal of Engineering and Technology (IRJET), Vol2,issue-01 March 2015. e-ISSN:2395-0056, p-ISSN:2395-0072. www.irjet.net
Abstract
In the present work finite element analysis of a glazed surface of a structure is performed. Considering particular location, topography and terrain and considering glass panel of a particular type, size and thickness, analysis is performed for wind pressure and suction acting on the surface. Different cases are considered â (i) varying truss widths, (ii) varying cable position and (iii) varying number of cables. The results of this work show that displacement, stress and weight of the steel of structure vary linearly with the variation in truss width. Displacement is found to be least for a certain range of truss width. Stresses decrease with increase in truss width and the weight of steel increases with increase in truss width. Having known this, one can choose economical and efficient steel supporting structure to support the glazing.01 kern's method.



01 kern's method.Naveen Kushwaha
Ėý
This document provides an overview of Kern's method for designing shell-and-tube heat exchangers. It begins with objectives and an introduction to Kern's method. It then outlines the design procedure algorithm and provides an example application. The example involves designing an exchanger to sub-cool methanol condensate using brackish water as the coolant. The document walks through each step of the Kern's method design process for this example, including calculating properties, determining duties, selecting tube/shell parameters, and estimating heat transfer coefficients.QUENCHING CRACK ANALYSIS OF BIG SIZE FORGING BY FE ANALYSIS



QUENCHING CRACK ANALYSIS OF BIG SIZE FORGING BY FE ANALYSISIAEME Publication
Ėý
Heat treatment crack is one of the main defects of big size forging manufacturing
process. In this research finite element method has been applied to predict crack
initiation by mass effect during water agitation cooling. Cooling curve of specimens
was measured water quenching test and convective coefficients were calculated by
inverse method based on finite element method. Water quenching processes were
analyzed by Deform software and Jmatpro. Crack initiation has been predicted by
comparison of stress distribution and strength on the temperature basisPortfolio Po-Chun Kang



Portfolio Po-Chun KangPO-CHUN (Andy) KANG
Ėý
This document summarizes a student project analyzing the effectiveness of different fin designs on a CPU heatsink. It includes the design of a base heatsink model with 30 fins and details simulations varying the number of fins, fin height, and rotation speed. The results show that increasing the number of fins from 30 to 50 lowers the maximum surface temperature by 5.4°C, while further increases do not impact temperature as much.Giancarlo Lombardi_Summer2014_NASA_GSFC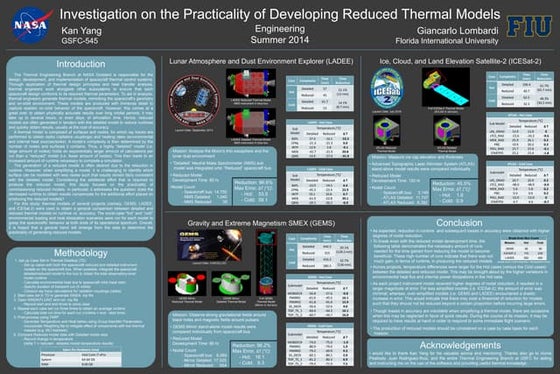



Giancarlo Lombardi_Summer2014_NASA_GSFCGiancarlo Lombardi
Ėý
- Thermal models were reduced by decreasing the number of nodes to decrease runtime, but this came at the cost of reduced accuracy. Across different spacecraft models, reduced models showed larger temperature differences and errors compared to detailed models, especially for more simplified models.
- Runtime was decreased by up to 61.7% for reduced models, but the development time required to create the reduced models was high. Break even points where runtime savings outweighed development costs required many repeated model runs, indicating reduced models may not be practical for most cases.04 - Army-MPR and Stevens Collaboration to Design and Build the Smallest Twin...



04 - Army-MPR and Stevens Collaboration to Design and Build the Smallest Twin...SEHER OZKAN
Ėý
The document describes the design and development of the world's smallest twin screw extruder for processing nanoparticles into nanoenergetics and nanocomposites. The extruder was designed using mathematical modeling and finite element analysis to optimize screw configuration and processing parameters for a propellant material. Key features include three sealed mixing and vacuum zones, temperature control zones, and a slit die. The design addresses challenges of conserving nanoparticle size and selecting proper binders for mixing nanoparticles at very small processing rates. The extruder will support Army research on developing highly energetic and less sensitive explosives.8 iiste photo 7



8 iiste photo 7Alexander Decker
Ėý
This document describes a photoelastic stress analysis of the bending strength of a helical gear. The analysis involved creating a 3D photoelastic model of the gear, subjecting it to loading, and freezing the stresses. Slices were cut from the model and observed under polarized light to determine stress distributions. Maximum bending stresses were calculated for different slices and scaled up to prototype values. Finite element analysis was also performed and showed good agreement with experimental results, with less than 2% variation in maximum stress values. The analysis found that helical gears experience higher peak bending stresses than spur gears due to their point contact loading.SHEAR CHAR STRENGTH OF TPUNS



SHEAR CHAR STRENGTH OF TPUNSJake Lewis
Ėý
This document summarizes research on evaluating the shear strength of the char layer formed on thermoplastic polyurethane elastomer nanocomposites (TPUNs) used as ablative materials. TPUN samples reinforced with carbon nanofibers and carbon nanotubes were tested using an oxy-acetylene test bed to simulate the extreme heating of a solid rocket motor. Infrared cameras and sensors monitored the sample surface temperature and behavior during testing. A shear strength sensor was used to apply a transverse load to the charred samples until rupture, measuring the reaction force. The maximum force and energy required to fracture the samples were evaluated to determine which material's char layer had the best shear strength performance for insulation applicationsFinite Element Analysis of shear wall and pipe interestion problem



Finite Element Analysis of shear wall and pipe interestion problemUniversity of Southern California
Ėý
This document summarizes a finite element analysis course project involving the analysis of a shear wall and shell intersection model. Key aspects include:
- Node and element label plots are provided for the shear wall under shear and thermal loading, and for the shell model.
- Deformation plots from ABAQUS and MATLAB are shown for the shear wall under shear and thermal loading, and for the shell model under pressure loading.
- Results from ABAQUS and MATLAB are compared and percentage errors discussed.
- Stress plots like von Mises and principal stresses from ABAQUS and MATLAB are presented for the shear wall and shell models.
- Codes written for creating shape functions, stiffness matrices, and performing other finite elementInternational Journal of Engineering Research and Development (IJERD)



International Journal of Engineering Research and Development (IJERD)IJERD Editor
Ėý
We would send hard copy of Journal by speed post to the address of correspondence author after online publication of paper.
We will dispatched hard copy to the author within 7 days of date of publicationPlanar pcb design guide(ferroxcube) no pw



Planar pcb design guide(ferroxcube) no pwJaeseong Cho
Ėý
This document provides a procedure for designing planar power transformers with low profiles and good thermal characteristics. The key steps are:
1) Calculate the maximum flux density based on allowed core losses and temperature rise using empirical formulas.
2) Determine the number of primary and secondary turns based on design formulas for the transformer topology (e.g. flyback, forward).
3) Distribute the winding turns across available PCB layers considering track width, spacing and insulation requirements.
4) Estimate the temperature rise in the PCB caused by AC currents using charts relating current, conductor dimensions and frequency.
An example is provided for a flyback transformer design meeting given specifications. Primary turns are distributed across 2-Structural modeling and meshing of blast furnace using TCL/Tk scripting.



Structural modeling and meshing of blast furnace using TCL/Tk scripting.debiprasadghosh
Ėý
The document describes modeling and meshing of a blast furnace structure using HyperMesh and finite element analysis software. Key steps include:
1. Creating the blast furnace geometry in HyperMesh using solid modeling techniques.
2. Generating a mesh and applying material properties, boundary conditions, and loads representing the blast furnace's operation.
3. Exporting the model to ANSYS and using Tcl/Tk scripts to automate running iterative simulations with modified parameters.
4. Viewing stress results in HyperView to evaluate design changes and optimize the blast furnace structure.Paper id 28201451



Paper id 28201451IJRAT
Ėý
This document summarizes a numerical study that examines the effects of fin spacing, fin material, and jet velocity on the heat transfer performance of plate fin heat sinks cooled by impinging air jets. The study considers fin spacings of 2mm, 3mm, and 4mm, and fin materials of aluminum, copper, and steel. Jet velocities of 5m/s, 10m/s, and 15m/s are examined. The results show that heat transfer rate increases with decreasing fin spacing, higher thermal conductivity fin materials like copper, and increasing jet velocity. Copper fins achieved the highest heat transfer rates but are heavier and more expensive than aluminum. A fin spacing of 2mm with aluminum fins and a jet velocity of 15Plug-In Hybrid Simulation



Plug-In Hybrid SimulationSiddhesh Ozarkar
Ėý
This document discusses the energy requirements and powertrain design for a plug-in hybrid electric vehicle (PHEV). It first estimates the energy needed at the wheels by simulating the vehicle's performance on various drive cycles. It then sizes the engine and electric motor based on these energy needs. For the engine, it selects a 100kW gasoline engine similar to that in the 2010 Toyota Prius. It models the Prius powertrain configuration and calculates performance metrics like acceleration and top speed. Finally, it evaluates the vehicle's emissions and fuel economy on the drive cycles to validate that it meets targets.FRONTAL FIXED 40 % OFFSET BARRIER TEST



FRONTAL FIXED 40 % OFFSET BARRIER TESTSiddhesh Ozarkar
Ėý
- A vehicle collision simulation was conducted using LS-Dyna to analyze the vehicle's response to a 10m/s frontal collision with a rigid barrier.
- The vehicle model included significant interior components like the seat, steering, and a dummy model. The seat was constrained to move with the vehicle.
- The simulation showed the vehicle's kinetic energy decreasing and internal energy increasing as the vehicle absorbed the impact energy through deformation. Dummy motion and stresses/displacements were within acceptable limits.
- Underbody structure reinforcement was suggested to better distribute loads and prevent engine intrusion into the passenger compartment under heavy impact loads.More Related Content
Similar to FR8695_IndProj (20)
Reliability of Flip-Chip Packages



Reliability of Flip-Chip PackagesPratik Saxena
Ėý
Developed 2D CAD model of flip chip package using Hypermesh
Fatigue analysis using ABAQUS CAE was done, predicting the life of solder balls in completely-filled clean and non-clean flip-chip packages
Assessed and identified the fatigue life of a completely clean model is higher than that of incomplete non-clean model
G012235155



G012235155IOSR Journals
Ėý
This document summarizes a numerical investigation and fatigue life estimation of a conventional diesel engine piston. A finite element analysis was conducted using ANSYS to determine thermal and stress distributions in the piston under operating loads. The piston geometry was meshed and assigned material properties of an aluminum alloy. Temperature loads from 250°C to 650°C and pressure loads from 1 to 60 bar were applied. The results from the finite element analysis were then used to estimate the fatigue life of the piston according to ASME standards. The analysis determined a fatigue life of 868,587 cycles with a peak stress of 69.8 MPa.A Study on Stochastic Thermal Characterization of Electronic Packages



A Study on Stochastic Thermal Characterization of Electronic PackagesIJERA Editor
Ėý
Insofar as the electronics can be found now in several applications of multiple domains, we have tried to
highlight in this study that, those systems must be based on unquestionable reliability and meet the needs of the
external environment. Starting from the unit "°c / w" concerning the thermal resistance from the gap between
junction temperature and a reference temperature, we have tried to compare the thermal performance of
electronic packages taking into consideration the thermal management. Our approach is based on the Monte
Carlo simulation and the stochastic characterization of the QFN. From the norm of normalization, we have
obtained standardized data sheets allowing accurate comparisons of the thermal performance of electronic
packages as produced by different manufacturers. Our numerical model through simulation, prototyping
concerning the design involves the JEDEC recommendations, which we consider a very interesting alternative.
Through the deterministic analysis, we conducted an analysis from the Matlab program parameters, which
control the Ansys software, the results were processed by statistical techniques to evaluate the times of the
thermal resistance of the QFN. That is why we must consider the electronic package (encapsulating the
integrated circuit), through the printed circuit board (PCB) to ensure the junction temperature maintenance and
avoid the dissipation of the heat. Also our process was based on the union of the finite element method to the
Monte Carlo simulation and stochastic characterization of the QFN.
Keywords: Electronic package; Finite element method; printed Paper id 27201447



Paper id 27201447IJRAT
Ėý
This document summarizes a study on predicting creep life for gas turbine discs. Creep is an important design consideration for gas turbine components operating at high temperatures. The study models a typical gas turbine disc and analyzes temperature distribution, stresses, and creep strains using finite element analysis. An Inconel 718 disc is modeled and analyzed to determine creep life and emphasize creep considerations in gas turbine disc design. Thermal, stress, and creep analyses are performed to evaluate creep life and strains at different stress levels over time.IJCER (www.ijceronline.com) International Journal of computational Engineerin...



IJCER (www.ijceronline.com) International Journal of computational Engineerin...ijceronline
Ėý
The document discusses the modeling and analysis of stresses in a steam turbine casing under transient operating conditions. It presents the finite element modeling process for the casing, including assumptions made and software used. The modeling considers thermal loading during startup and shutdown cycles. Heat transfer and thermal analyses are conducted to determine temperature distributions and thermal stresses within the casing. High stresses are found at locations like corners, welds and nozzle fits, which can lead to damage like cracking over the turbine's lifetime. The analysis aims to better understand casing behavior under transient conditions and improve design for increased reliability and life.FEA Project-Pressure Vessel & Heat Loss Analysis



FEA Project-Pressure Vessel & Heat Loss AnalysisMostafa Fakharifar
Ėý
This document summarizes a finite element analysis of a pressure vessel subjected to internal pressure and temperature gradients. A one-eighth model was analyzed in ABAQUS for three load cases: internal pressure only, internal temperature only, and combined internal pressure and temperature. Mesh convergence studies showed the medium-fine mesh provided accurate results efficiently. For internal pressure only, the maximum von-Mises stress was 504.8 MPa. For internal temperature only, the maximum stress was lower at 123.1 MPa. The combined case produced the highest stresses and strains, with a maximum von-Mises stress of 555.1 MPa occurring at the top center and fillet.Thermal stresses



Thermal stressesrawaabdullah
Ėý
Thermal stresses occur when a material experiences a change in temperature that causes it to expand or contract. This document discusses thermal stresses, providing examples of how temperature gradients and differences in thermal expansion coefficients between materials can lead to stresses. It also outlines the background and methods for experimentally measuring thermal stress fields in a composite-metal bonded joint, including using infrared cameras to measure temperature fields and grid analysis to measure displacement fields. The results provide insight into how temperature changes affect the stress distribution in the bonded joint.IRJET-Finite Element Analysis of Glazed Surface



IRJET-Finite Element Analysis of Glazed SurfaceIRJET Journal
Ėý
Raghunath .D. Deshpande, Gayatri Chiniwal, Laxmi Golsangi, "Finite Element Analysis of Glazed Surface", International Research Journal of Engineering and Technology (IRJET), Vol2,issue-01 March 2015. e-ISSN:2395-0056, p-ISSN:2395-0072. www.irjet.net
Abstract
In the present work finite element analysis of a glazed surface of a structure is performed. Considering particular location, topography and terrain and considering glass panel of a particular type, size and thickness, analysis is performed for wind pressure and suction acting on the surface. Different cases are considered â (i) varying truss widths, (ii) varying cable position and (iii) varying number of cables. The results of this work show that displacement, stress and weight of the steel of structure vary linearly with the variation in truss width. Displacement is found to be least for a certain range of truss width. Stresses decrease with increase in truss width and the weight of steel increases with increase in truss width. Having known this, one can choose economical and efficient steel supporting structure to support the glazing.01 kern's method.



01 kern's method.Naveen Kushwaha
Ėý
This document provides an overview of Kern's method for designing shell-and-tube heat exchangers. It begins with objectives and an introduction to Kern's method. It then outlines the design procedure algorithm and provides an example application. The example involves designing an exchanger to sub-cool methanol condensate using brackish water as the coolant. The document walks through each step of the Kern's method design process for this example, including calculating properties, determining duties, selecting tube/shell parameters, and estimating heat transfer coefficients.QUENCHING CRACK ANALYSIS OF BIG SIZE FORGING BY FE ANALYSIS



QUENCHING CRACK ANALYSIS OF BIG SIZE FORGING BY FE ANALYSISIAEME Publication
Ėý
Heat treatment crack is one of the main defects of big size forging manufacturing
process. In this research finite element method has been applied to predict crack
initiation by mass effect during water agitation cooling. Cooling curve of specimens
was measured water quenching test and convective coefficients were calculated by
inverse method based on finite element method. Water quenching processes were
analyzed by Deform software and Jmatpro. Crack initiation has been predicted by
comparison of stress distribution and strength on the temperature basisPortfolio Po-Chun Kang



Portfolio Po-Chun KangPO-CHUN (Andy) KANG
Ėý
This document summarizes a student project analyzing the effectiveness of different fin designs on a CPU heatsink. It includes the design of a base heatsink model with 30 fins and details simulations varying the number of fins, fin height, and rotation speed. The results show that increasing the number of fins from 30 to 50 lowers the maximum surface temperature by 5.4°C, while further increases do not impact temperature as much.Giancarlo Lombardi_Summer2014_NASA_GSFC



Giancarlo Lombardi_Summer2014_NASA_GSFCGiancarlo Lombardi
Ėý
- Thermal models were reduced by decreasing the number of nodes to decrease runtime, but this came at the cost of reduced accuracy. Across different spacecraft models, reduced models showed larger temperature differences and errors compared to detailed models, especially for more simplified models.
- Runtime was decreased by up to 61.7% for reduced models, but the development time required to create the reduced models was high. Break even points where runtime savings outweighed development costs required many repeated model runs, indicating reduced models may not be practical for most cases.04 - Army-MPR and Stevens Collaboration to Design and Build the Smallest Twin...



04 - Army-MPR and Stevens Collaboration to Design and Build the Smallest Twin...SEHER OZKAN
Ėý
The document describes the design and development of the world's smallest twin screw extruder for processing nanoparticles into nanoenergetics and nanocomposites. The extruder was designed using mathematical modeling and finite element analysis to optimize screw configuration and processing parameters for a propellant material. Key features include three sealed mixing and vacuum zones, temperature control zones, and a slit die. The design addresses challenges of conserving nanoparticle size and selecting proper binders for mixing nanoparticles at very small processing rates. The extruder will support Army research on developing highly energetic and less sensitive explosives.8 iiste photo 7



8 iiste photo 7Alexander Decker
Ėý
This document describes a photoelastic stress analysis of the bending strength of a helical gear. The analysis involved creating a 3D photoelastic model of the gear, subjecting it to loading, and freezing the stresses. Slices were cut from the model and observed under polarized light to determine stress distributions. Maximum bending stresses were calculated for different slices and scaled up to prototype values. Finite element analysis was also performed and showed good agreement with experimental results, with less than 2% variation in maximum stress values. The analysis found that helical gears experience higher peak bending stresses than spur gears due to their point contact loading.SHEAR CHAR STRENGTH OF TPUNS



SHEAR CHAR STRENGTH OF TPUNSJake Lewis
Ėý
This document summarizes research on evaluating the shear strength of the char layer formed on thermoplastic polyurethane elastomer nanocomposites (TPUNs) used as ablative materials. TPUN samples reinforced with carbon nanofibers and carbon nanotubes were tested using an oxy-acetylene test bed to simulate the extreme heating of a solid rocket motor. Infrared cameras and sensors monitored the sample surface temperature and behavior during testing. A shear strength sensor was used to apply a transverse load to the charred samples until rupture, measuring the reaction force. The maximum force and energy required to fracture the samples were evaluated to determine which material's char layer had the best shear strength performance for insulation applicationsFinite Element Analysis of shear wall and pipe interestion problem



Finite Element Analysis of shear wall and pipe interestion problemUniversity of Southern California
Ėý
This document summarizes a finite element analysis course project involving the analysis of a shear wall and shell intersection model. Key aspects include:
- Node and element label plots are provided for the shear wall under shear and thermal loading, and for the shell model.
- Deformation plots from ABAQUS and MATLAB are shown for the shear wall under shear and thermal loading, and for the shell model under pressure loading.
- Results from ABAQUS and MATLAB are compared and percentage errors discussed.
- Stress plots like von Mises and principal stresses from ABAQUS and MATLAB are presented for the shear wall and shell models.
- Codes written for creating shape functions, stiffness matrices, and performing other finite elementInternational Journal of Engineering Research and Development (IJERD)



International Journal of Engineering Research and Development (IJERD)IJERD Editor
Ėý
We would send hard copy of Journal by speed post to the address of correspondence author after online publication of paper.
We will dispatched hard copy to the author within 7 days of date of publicationPlanar pcb design guide(ferroxcube) no pw



Planar pcb design guide(ferroxcube) no pwJaeseong Cho
Ėý
This document provides a procedure for designing planar power transformers with low profiles and good thermal characteristics. The key steps are:
1) Calculate the maximum flux density based on allowed core losses and temperature rise using empirical formulas.
2) Determine the number of primary and secondary turns based on design formulas for the transformer topology (e.g. flyback, forward).
3) Distribute the winding turns across available PCB layers considering track width, spacing and insulation requirements.
4) Estimate the temperature rise in the PCB caused by AC currents using charts relating current, conductor dimensions and frequency.
An example is provided for a flyback transformer design meeting given specifications. Primary turns are distributed across 2-Structural modeling and meshing of blast furnace using TCL/Tk scripting.



Structural modeling and meshing of blast furnace using TCL/Tk scripting.debiprasadghosh
Ėý
The document describes modeling and meshing of a blast furnace structure using HyperMesh and finite element analysis software. Key steps include:
1. Creating the blast furnace geometry in HyperMesh using solid modeling techniques.
2. Generating a mesh and applying material properties, boundary conditions, and loads representing the blast furnace's operation.
3. Exporting the model to ANSYS and using Tcl/Tk scripts to automate running iterative simulations with modified parameters.
4. Viewing stress results in HyperView to evaluate design changes and optimize the blast furnace structure.Paper id 28201451



Paper id 28201451IJRAT
Ėý
This document summarizes a numerical study that examines the effects of fin spacing, fin material, and jet velocity on the heat transfer performance of plate fin heat sinks cooled by impinging air jets. The study considers fin spacings of 2mm, 3mm, and 4mm, and fin materials of aluminum, copper, and steel. Jet velocities of 5m/s, 10m/s, and 15m/s are examined. The results show that heat transfer rate increases with decreasing fin spacing, higher thermal conductivity fin materials like copper, and increasing jet velocity. Copper fins achieved the highest heat transfer rates but are heavier and more expensive than aluminum. A fin spacing of 2mm with aluminum fins and a jet velocity of 15Finite Element Analysis of shear wall and pipe interestion problem



Finite Element Analysis of shear wall and pipe interestion problemUniversity of Southern California
Ėý
More from Siddhesh Ozarkar (7)
Plug-In Hybrid Simulation



Plug-In Hybrid SimulationSiddhesh Ozarkar
Ėý
This document discusses the energy requirements and powertrain design for a plug-in hybrid electric vehicle (PHEV). It first estimates the energy needed at the wheels by simulating the vehicle's performance on various drive cycles. It then sizes the engine and electric motor based on these energy needs. For the engine, it selects a 100kW gasoline engine similar to that in the 2010 Toyota Prius. It models the Prius powertrain configuration and calculates performance metrics like acceleration and top speed. Finally, it evaluates the vehicle's emissions and fuel economy on the drive cycles to validate that it meets targets.FRONTAL FIXED 40 % OFFSET BARRIER TEST



FRONTAL FIXED 40 % OFFSET BARRIER TESTSiddhesh Ozarkar
Ėý
- A vehicle collision simulation was conducted using LS-Dyna to analyze the vehicle's response to a 10m/s frontal collision with a rigid barrier.
- The vehicle model included significant interior components like the seat, steering, and a dummy model. The seat was constrained to move with the vehicle.
- The simulation showed the vehicle's kinetic energy decreasing and internal energy increasing as the vehicle absorbed the impact energy through deformation. Dummy motion and stresses/displacements were within acceptable limits.
- Underbody structure reinforcement was suggested to better distribute loads and prevent engine intrusion into the passenger compartment under heavy impact loads.IPD



IPDSiddhesh Ozarkar
Ėý
This project aims to develop a re-designed and improved automated workstation to help increase employee productivity and efficiency. The workstation will save organizations space by occupying less area, allowing them to accommodate more employees without spending money on new buildings. It will also save the effort of maintaining productivity during renovation periods. The report discusses generating specifications for the workstation based on customer needs analysis. It addresses challenges in creating the product and its potential environmental impacts. The workstation aims to provide a more comfortable working atmosphere for employees to further boost productivity.Acceleration Modelling of EV1_FR8695



Acceleration Modelling of EV1_FR8695Siddhesh Ozarkar
Ėý
This document models and simulates the acceleration of a GM EV1 electric vehicle from 0 to 60 mph using MATLAB. It considers three mass scenarios: the base mass of 1560kg, a reduced mass of 1326kg, and an increased mass of 1794kg. The acceleration is modeled in two phases using differential equations. Simulation results show that acceleration decreases as mass increases, with times to reach 60 mph being 9.1, 7.8, and 10.5 seconds respectively. A mathematical solution to the differential equations is also derived and verified against MATLAB simulations, showing close matches.Casestudy3_TeamPulverisers5_MITPune - report



Casestudy3_TeamPulverisers5_MITPune - reportSiddhesh Ozarkar
Ėý
1) The document describes various design solutions to reduce deflection in the common pedal shaft of a tractor. It aims to simplify packaging and improve pedal efficiency.
2) The first solution adds a Plummer block support to the overhanging end of the shaft. The second solution uses an overhang reinforcement casing.
3) Other solutions reduce the overhang length by coupling the clutch and left brake pedals, using a button clutch with independent brake pedals, or a centrally located clutch pedal with latching brake pedals.Zero Turn Radius Report - Team Panache (1)



Zero Turn Radius Report - Team Panache (1)Siddhesh Ozarkar
Ėý
The document describes a proposed design for modifying a John Deere 5065E tractor to achieve zero turn or minimum turning radius capability. Key aspects of the design include:
1. Eliminating the gearbox transmission and differential, and incorporating braking and transmission systems into a hydraulic circuit using two independent hydraulic motors to enable wheels to rotate in the same or opposite directions.
2. Using hydraulically powered tie rods connected to the steering arms to enable the front wheels to turn in opposite directions during zero turn maneuvers while regular steering is maintained via power steering.
3. Employing directional control valves and pressure relief valves to manage fluid flow and pressures to different components including the steering, transmission, and tie rodsBAJA 2014 presentation



BAJA 2014 presentationSiddhesh Ozarkar
Ėý
The document provides technical details of a BAJA SAE vehicle designed by Team Piranha Racing from Maharashtra Institute of Technology, Pune. It includes dimensions, materials, and analysis results for components like the roll cage, suspension, transmission, brakes, and steering. Graphs show performance parameters like acceleration, traction forces, and steering characteristics. The document also outlines the project plan, workshop facilities, cost breakdown, and validation methods like a half-car model analysis of camber and roll center heights.FR8695_IndProj
- 1. Manufacturing Process Mechanics Individual Project (P2R2) NAME: SIDDHESH OZARKAR FR8695@WAYNE.EDU ï· DESIGNATION OF MODEL: P2R2 Model Number 2 with Radius of 0.015mm and 2nd order elements (CPE8) used
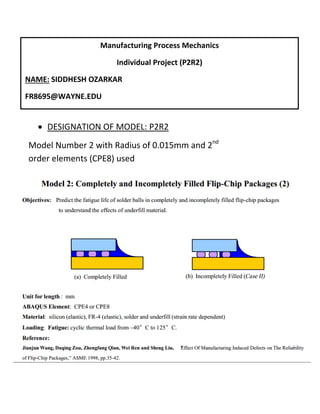
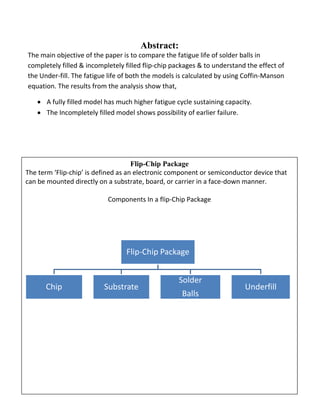
- 2. Abstract: The main objective of the paper is to compare the fatigue life of solder balls in completely filled & incompletely filled flip-chip packages & to understand the effect of the Under-fill. The fatigue life of both the models is calculated by using Coffin-Manson equation. The results from the analysis show that, ï· A fully filled model has much higher fatigue cycle sustaining capacity. ï· The Incompletely filled model shows possibility of earlier failure. Flip-Chip Package The term âFlip-chipâ is defined as an electronic component or semiconductor device that can be mounted directly on a substrate, board, or carrier in a face-down manner. Components In a flip-Chip Package Flip-Chip Package Chip Substrate Solder Balls Underfill
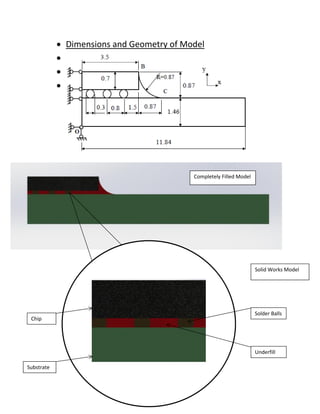
- 3. ï· Dimensions and Geometry of Model ï· ï· ï· Completely Filled Model Solid Works Model Chip Substrate Solder Balls Underfill
- 4. Chip Under-fill Solder-Balls Substrate Radius Given as per requirement.
- 5. Pre-Processing 1) The geometry of both the models completely filled and Incompletely Filled flip-chip packages is created as per the given dimensions in Solidworks. 2) Only surface geometry were created in Solidworks, the files were exported (.IGES) to Hypermesh 3) Meshing was performed on these models in Hypermesh. 4) Surfaces representing the various components were Independently Meshed so as to achieve finer mesh where required. 5) Finer mesh was created where potential high stress concentration was suspected like the solder balls. 6) Edges were set to equivalence after meshing. 7) Properties of the mesh ï· Element type â CPE8 ï· Smallest element size- 0.01mm 8) A solver deck containing the material properties for the various components of the package was imported in the Hypermesh file directory. This solver deck was assigned to the model so that respective elements have respective properties. 9) Boundary conditions were applied as follows - Constraint the left vertical side with DOF-1, bottom horizontal side with DOF-2, and intersection of both with DOF-1,2 . 10) All nodes were defined in one Entity set for the ease of utility. This entity set also contains the initial temperature condition of the model. 11) Create 4 load collectors were created as follows- ï· Constraint ï· Initial Temp(20C) ï· Temperature 233 K (â40°C) ï· Temperature 398 K (125°C) 12) Create Output block and 3 Load step. Step 1- Initial increment= 10 over a time period of 600 sec at temperature of 233 K Step 2- Increment= 10 over a time period of 900 sec at temperature of 398 K Step 3- Increment= 10 over a time period of 600 sec at temperature of 233 K 13) The file was then exported to Abaqus for Further Analysis.
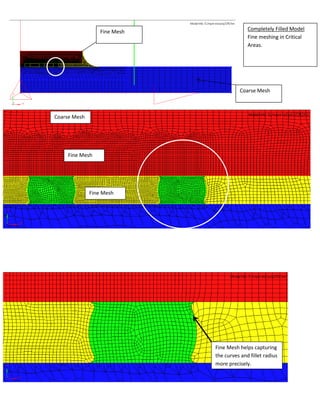
- 6. Completely Filled Model Fine meshing in Critical Areas. Coarse Mesh Fine Mesh Coarse Mesh Fine Mesh Fine Mesh Fine Mesh helps capturing the curves and fillet radius more precisely.
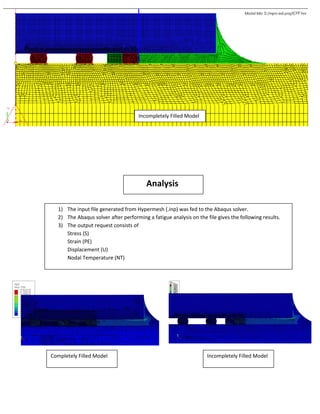
- 7. Incompletely Filled Model Analysis 1) The input file generated from Hypermesh (.inp) was fed to the Abaqus solver. 2) The Abaqus solver after performing a fatigue analysis on the file gives the following results. 3) The output request consists of Stress (S) Strain (PE) Displacement (U) Nodal Temperature (NT) Completely Filled Model Incompletely Filled Model
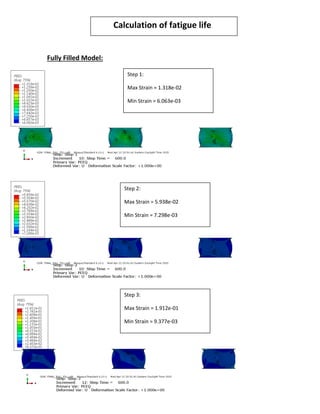
- 8. Fully Filled Model: Calculation of fatigue life Step 1: Max Strain = 1.318e-02 Min Strain = 6.063e-03 Step 2: Max Strain = 5.938e-02 Min Strain = 7.298e-03 Step 3: Max Strain = 1.912e-01 Min Strain = 9.377e-03
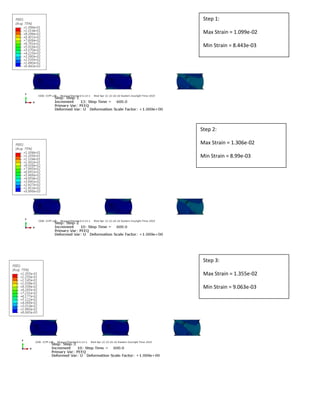
- 9. Step 1: Max Strain = 1.099e-02 Min Strain = 8.443e-03 Step 2: Max Strain = 1.306e-02 Min Strain = 8.99e-03 Step 3: Max Strain = 1.355e-02 Min Strain = 9.063e-03
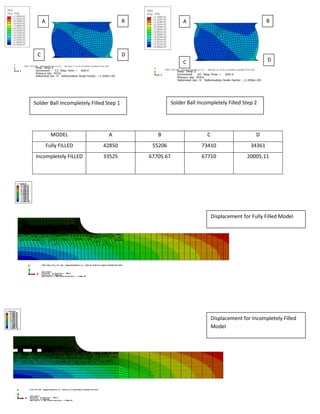
- 10. Fatigue Life of Solder Balls: According to the Coffin-Mansonâs equation, (Nf)Îē ÎÎģP = CP Where, Îē = Fatigue ductility exponent (Îē= 0.51) Nf = Fatigue life ÎÎģP = Applied plastic/inelastic strain range CP = Fatigue ductility coefficient. (CP= 1.14) Considering the rightmost solder ball for analysis: Model Step Number Max. Strain Min. Strain Fully Filled 1 1.318e-02 6.063e-03 - 2 5.938e-02 7.298e-03 - 3 1.912e-01 9.377e-03 Incompletely Filled 1 1.099e-02 8.443e-03 - 2 1.306e-02 8.991e-03 - 3 1.355e-02 9.063e-03 Solder Ball Fully Filled Step 1 Solder Ball Fully Filled Step 2 A A B A B A D C D C C
- 11. MODEL A B C D Fully FILLED 42850 55206 73410 34361 Incompletely FILLED 33525 67705.67 67710 20005.11 Solder Ball Incompletely Filled Step 1 Solder Ball Incompletely Filled Step 2 A AB A B A C D C D C C Displacement for Fully Filled Model Displacement for Incompletely Filled Model
- 12. CONCLUSION: ï· Fatigue life of completely filled model is higher than that of the incompletely filled model. ï· As compared to models with no radius present at the corners of the solder balls the ones with a radius present show higher fatigue sustaining capacity. ï· Due to the presence of radius the strain is more distributed and thus results in extended life of flip chip package.
