Lecture failure criteria
0 likes342 views
Thermoelastic stress analysis (TSA) measures small temperature changes in materials under load that correspond to stress levels. While failure criteria are not directly based on the sum of principal stresses measured by TSA, it can still predict failure locations. Both finite element analysis (FEA) and TSA are full-field techniques that provide spatial stress distributions. They complement each other, with FEA useful early in design and TSA confirming FEA models through experimental loading. Together, FEA and TSA provide a strong approach for predicting failure.
More Related Content
Viewers also liked (19)
Similar to Lecture failure criteria (20)
More from Hamdani Nurdin (6)
Lecture failure criteria
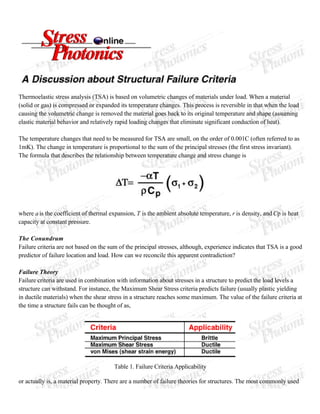
- 1. Thermoelastic stress analysis (TSA) is based on volumetric changes of materials under load. When a material (solid or gas) is compressed or expanded its temperature changes. This process is reversible in that when the load causing the volumetric change is removed the material goes back to its original temperature and shape (assuming elastic material behavior and relatively rapid loading changes that eliminate significant conduction of heat). The temperature changes that need to be measured for TSA are small, on the order of 0.001C (often referred to as 1mK). The change in temperature is proportional to the sum of the principal stresses (the first stress invariant). The formula that describes the relationship between temperature change and stress change is where a is the coefficient of thermal expansion, T is the ambient absolute temperature, r is density, and Cp is heat capacity at constant pressure. The Conundrum Failure criteria are not based on the sum of the principal stresses, although, experience indicates that TSA is a good predictor of failure location and load. How can we reconcile this apparent contradiction? Failure Theory Failure criteria are used in combination with information about stresses in a structure to predict the load levels a structure can withstand. For instance, the Maximum Shear Stress criteria predicts failure (usually plastic yielding in ductile materials) when the shear stress in a structure reaches some maximum. The value of the failure criteria at the time a structure fails can be thought of as, Table 1. Failure Criteria Applicability or actually is, a material property. There are a number of failure theories for structures. The most commonly used
- 2. ones are maximum stress, maximum shear stress, and von Mises stress. Each of these has their applicability, but the von Mises criteria is considered the most generally applicable. Table 2. TSA and Failure Criteria Formulas Finite Element Analysis Finite element analysis is a numerical modeling technique that combines structural geometry and loading information with material properties to predict stresses and strains in a structure. The technique is quite flexible and has wide applicability. FEA is often effectively used early in the design cycle to provide important information by reporting stresses in a structure full-field. However, FEA has some short comings. Modeling some particular, yet fairly common, types of loads and structural components with enough detail to provide good predictions can be difficult, and FEA is susceptible to incorrect information about material properties and loading conditions. Consequently, FEA is often used in combination with an experimental technique or some other confirming information. Failure Prediction with FEA and TSA Since finite element analysis (FEA) and thermoelastic stress analysis (TSA) are both full-field techniques it is also useful to think of failure criteria in full-field terms. Consider an image of the stresses near a hole in a strap loaded in pure tension for each of the formulations shown in Table 2. There are places around the hole where the stress state is biaxial, so it is expected that full-field presentations for each of the formulations would be different. One difference between the presentations would be the availability of sign information (see Table 3.). The sign is useful information to the stress analyst trying to visualize the stress flow through a structure. One could argue that shear stress has sign but it is not useful to think of it in this way. In terms of Mohr's circle shear stress is the diameter of the Mohr's circle, but a diameter cannot have a negative value.
- 3. Table 3. Full-Field Sign Information When Failure Theories Converge To look at the situation from another angle, consider that many cracks start on an edge of a structure. Fig. 1 describes the stress state at an edge. No stress is present in two perpendicular directions because they are open to the air. (Of course, this is not always true, the example of a round shaft can be sighted, but bear with me.) For this important state of stress all three failure criteria, and the sum of the principal stresses reduce to the same thing, s1. Therefore the stress concentration factor, Kt that is calculated by all the formulas is the same at the critical failure location. Fig. 3. State of stress near an edge must be uniaxial. Table 4. Calculating Kt with each of the formulas for the state of stress at the edge of a hole in a tensile field. The Case of Pure Shear Consider the most common case of pure shear stress, a uniform bar in torsion. Pure shear stress causes a material to shear only. Since there is no volumetric change, the sum of the principal stresses is zero. Consequently, TSA is often discounted as a useful measurement on this basis. As a practical matter there are few times when the section of shaft in pure shear is of interest structurally. This is because it is easy to calculate the stress levels in a shaft, failure theory holds well and consequently the design is usually well executed. The torque input and output couplings, however, are the likely causes of problems. Splines, keyways, shear pins, tapers, fillets, or notches are generally where problem initiate. In all of these cases the state of stress is a complicated one. One that challenges every experimental, numerical, and analytical technique. In difficult circumstances any information is welcome. Under circumstances like these FEA and TSA can work well
- 4. together to solve problems. TSA and FEA One of the most common uses of TSA is the confirmation and fine tuning of FEA models. The techniques have much in common, or maybe it is better to say " in complement". Both techniques are full-field. Both techniques have a spatial resolution dependent on the size of element (pixel) used. Both techniques are strong at showing relative stress in a structure in terms of location and magnitude. Both techniques require loading information and material property information for the accurate prediction of failure location and probability. For all their similarities, it is the differences that make the two techniques particularly strong when used together. FEA is most often used early in the design cycle. It is very useful in comparing design alternatives, but only if the model is accurate. If a prototype is available, even a plastic model, TSA can be used to confirm that the FEA model is accurate. While TSA requires the actual application of loads to the structure, this can bring into focus aspects of loading that are very difficult to predict or model. TSA of an actual prototype will not give false readings because of poor meshing, element choice, or inaccurate geometry modeling. Once confidence in the FEA model is established it can be used to check internal stresses, and to test design modifications without actually needing to be test a specimen. Sometimes structures are very complicated, or loading on the structure is so complicated that FEA is presented with nearly insurmountable challenges for a detailed analysis. Consider a highway bridge, where traffic loading, redundant supports, and interactions between concrete and steel set out a very difficult problem. Consider the fuel tank of a car, which is a complicated shape loaded with vibrations and road inputs, and filled with an infinite number of possible fuel levels. FEA is hardly useless in these cases, but only if loading inputs or initial stress states, or particular stress states can be verified. TSA will provide a stress pattern for any given condition, and it will always be the right stress pattern for that condition. By using TSA data as a starting point designers experiment with either real or numerical methods to see how structural conditions can be optimized. In Terms of Mohr's Circle Many failure theories are discussed in terms of Mohr's circle. The TSA measurement provides the sum of the principal stresses. Divide this by two and you have the location of the center of the Mohr's circle on the s axis. Photoelasticity results are proportional to the difference of the principal stresses, which is the diameter of Mohr's circle. (We gotta get these two together.) The full triaxial state of stress at any point can be represented as three circles. Compatibility dictates that each of the three circles must touch the other two along the s axis. The state of stress at the surface of a structure can only have a biaxial state of stress. In this case two of the three circles must go through the origin. Conclusion Like every experimental, numerical, and analytical stress analysis technique TSA has its advantages and disadvantages. We believe that an informed user of TSA technology can obtain interesting and useful results.
- 5. The engineers at Stress Photonics are always interested in discussing applications of TSA and other experimental techniques. We know that helping our customers (and potential customers) find effective and appropriate solutions to their problems must be our primary goal. We continue to develop the TSA technology so that it can be a tool to help solve an increasingly broader range of engineering problems. Fig 2. TSA image of stress near a hole in a strap loaded in tension.